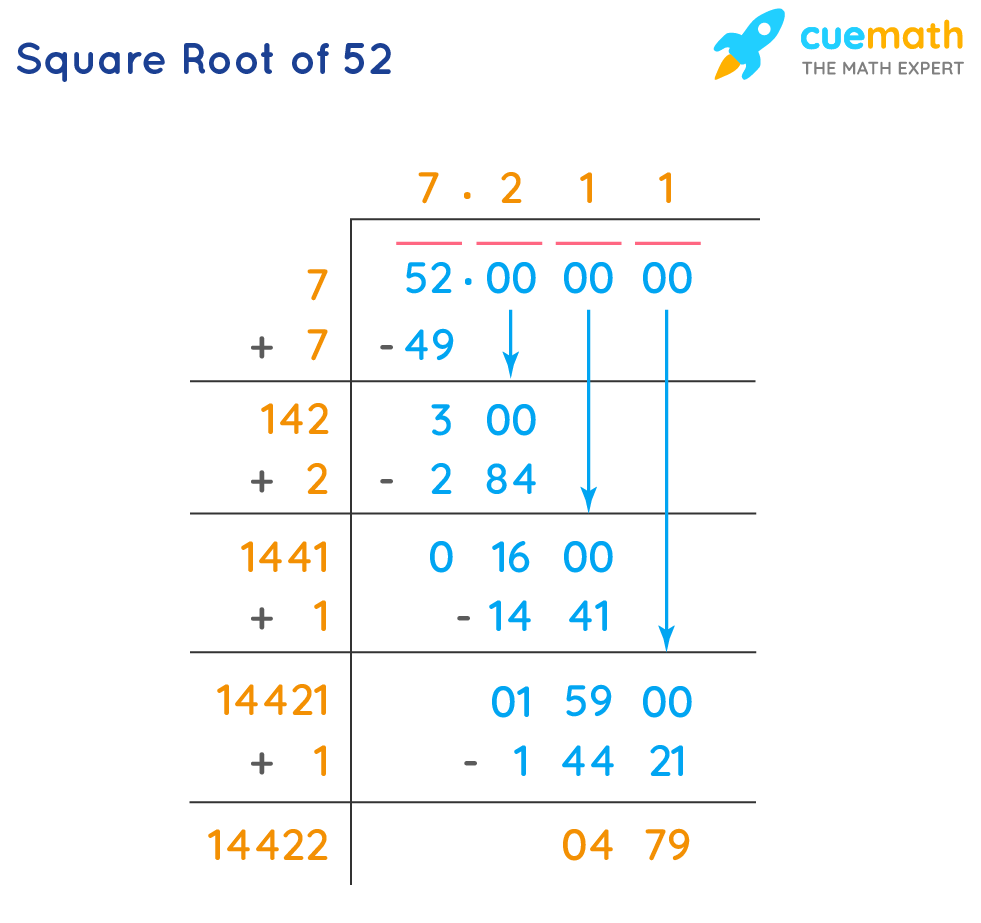
Topic square root of 25/49: The square root of 25/49 is a simple yet fascinating mathematical concept that offers insight into the world of fractions and roots. In this comprehensive guide, we'll explore what it means, how to calculate it, and its applications in various fields. Join us on this journey to master the square root of 25/49.
Table of Content
- Square Root of 25/49
- Introduction to Square Roots
- What is the Square Root of a Fraction?
- Understanding the Square Root of 25/49
- Step-by-Step Calculation
- Simplifying Fractions Before Finding Square Roots
- Examples of Similar Square Root Calculations
- Importance of Square Roots in Mathematics
- Applications of Square Roots in Real Life
- Common Mistakes to Avoid
- Advanced Topics: Irrational and Rational Numbers
- Conclusion and Summary
- YOUTUBE: Video hướng dẫn đơn giản hóa căn bậc hai của 25/49 bằng tiếng Việt, giúp người xem hiểu rõ hơn về quá trình tính toán và ứng dụng của nó.
Square Root of 25/49
The square root of a fraction can be found by taking the square root of the numerator and the denominator separately.
Calculation
Given the fraction:
The square root of the numerator (25) and the denominator (49) are calculated as follows:
- = 5
- = 7
Therefore, the square root of is:

READ MORE:
Introduction to Square Roots
The square root of a number is a value that, when multiplied by itself, gives the original number. It is represented by the radical symbol √. For example, the square root of 9 is 3 because \(3 \times 3 = 9\).
Square roots are essential in various areas of mathematics, including algebra, geometry, and calculus. They help simplify equations and solve quadratic equations. Understanding square roots is also crucial for grasping more advanced mathematical concepts.
When dealing with fractions, the square root can be applied to both the numerator and the denominator. For instance, the square root of a fraction \(\frac{a}{b}\) is \(\frac{\sqrt{a}}{\sqrt{b}}\). Let's explore this with the fraction \(\frac{25}{49}\).
- Numerator: \(25\)
- Denominator: \(49\)
We find the square roots of both parts:
- \(\sqrt{25} = 5\)
- \(\sqrt{49} = 7\)
Therefore, the square root of \(\frac{25}{49}\) is \(\frac{5}{7}\).
Square roots can also be irrational numbers, meaning they cannot be expressed as a simple fraction. Examples include \(\sqrt{2}\) and \(\sqrt{3}\). However, \(\sqrt{25/49}\) is a rational number, making it a straightforward example for understanding square roots.
Below is a summary table for quick reference:
| Expression | Result |
| \(\sqrt{25}\) | 5 |
| \(\sqrt{49}\) | 7 |
| \(\sqrt{\frac{25}{49}}\) | \(\frac{5}{7}\) |
What is the Square Root of a Fraction?
The square root of a fraction involves finding the square roots of both its numerator and denominator separately. This process can simplify understanding and calculating the square root of the entire fraction. Let's consider a fraction \(\frac{a}{b}\), where \(a\) and \(b\) are positive integers.
To find the square root of \(\frac{a}{b}\), follow these steps:
- Identify the numerator (\(a\)) and the denominator (\(b\)) of the fraction.
- Calculate the square root of the numerator: \(\sqrt{a}\).
- Calculate the square root of the denominator: \(\sqrt{b}\).
- Express the result as a new fraction: \(\frac{\sqrt{a}}{\sqrt{b}}\).
For example, let's find the square root of \(\frac{25}{49}\):
- Numerator: \(25\)
- Denominator: \(49\)
Next, we find the square roots of these values:
- \(\sqrt{25} = 5\)
- \(\sqrt{49} = 7\)
Therefore, the square root of \(\frac{25}{49}\) is \(\frac{5}{7}\).
This method can be applied to any fraction where both the numerator and the denominator are perfect squares. When they are not, the result might be an irrational number, which cannot be expressed as a simple fraction but can be approximated.
Here's a summary table for quick reference:
| Fraction | Square Root of Numerator | Square Root of Denominator | Result |
| \(\frac{25}{49}\) | 5 | 7 | \(\frac{5}{7}\) |
| \(\frac{16}{81}\) | 4 | 9 | \(\frac{4}{9}\) |
| \(\frac{9}{64}\) | 3 | 8 | \(\frac{3}{8}\) |
Understanding the Square Root of 25/49
The square root of \(\frac{25}{49}\) is an interesting and straightforward calculation that illustrates the concept of finding square roots of fractions. To understand this better, we need to break down the fraction and apply the square root to both the numerator and the denominator separately.
Let's start with the fraction:
- Numerator: \(25\)
- Denominator: \(49\)
Now, we find the square root of each part:
- Calculate the square root of the numerator: \(\sqrt{25} = 5\).
- Calculate the square root of the denominator: \(\sqrt{49} = 7\).
By placing these results into a fraction, we get:
\(\frac{\sqrt{25}}{\sqrt{49}} = \frac{5}{7}\)
This means that the square root of \(\frac{25}{49}\) is \(\frac{5}{7}\). This result can be understood and verified through the following steps:
- Identify that both \(25\) and \(49\) are perfect squares.
- Recognize that the square root of \(25\) is \(5\).
- Recognize that the square root of \(49\) is \(7\).
- Combine these results to form the fraction \(\frac{5}{7}\).
This fraction \(\frac{5}{7}\) is in its simplest form and is the exact square root of the original fraction \(\frac{25}{49}\).
For clarity, here's a summary table:
| Fraction | Square Root of Numerator | Square Root of Denominator | Result |
| \(\frac{25}{49}\) | 5 | 7 | \(\frac{5}{7}\) |
In conclusion, understanding the square root of \(\frac{25}{49}\) involves recognizing that the process requires taking the square root of both the numerator and the denominator. This results in a simplified fraction that accurately represents the square root of the original fraction.
Step-by-Step Calculation
Finding the square root of \(\frac{25}{49}\) involves a series of straightforward steps. By breaking down the process, we can understand how to approach the problem and apply similar methods to other fractions.
Here is a detailed, step-by-step calculation:
-
Identify the fraction:
We start with the fraction \(\frac{25}{49}\).
-
Find the square root of the numerator:
- The numerator is \(25\).
- The square root of \(25\) is \(5\) because \(5 \times 5 = 25\).
-
Find the square root of the denominator:
- The denominator is \(49\).
- The square root of \(49\) is \(7\) because \(7 \times 7 = 49\).
-
Combine the square roots:
Place the square roots of the numerator and denominator into a fraction:
\(\frac{\sqrt{25}}{\sqrt{49}} = \frac{5}{7}\)
-
Simplify the fraction (if necessary):
In this case, the fraction \(\frac{5}{7}\) is already in its simplest form.
-
Verify the result:
To ensure accuracy, multiply the result by itself:
\(\left( \frac{5}{7} \right)^2 = \frac{5 \times 5}{7 \times 7} = \frac{25}{49}\)
This confirms that \(\frac{5}{7}\) is indeed the square root of \(\frac{25}{49}\).
Here is a summary of the steps in a table format:
| Step | Operation | Result |
| 1 | Original Fraction | \(\frac{25}{49}\) |
| 2 | Square Root of Numerator | \(5\) |
| 3 | Square Root of Denominator | \(7\) |
| 4 | Combine | \(\frac{5}{7}\) |
| 5 | Simplify | \(\frac{5}{7}\) (already simplified) |
| 6 | Verification | \(\left( \frac{5}{7} \right)^2 = \frac{25}{49}\) |
By following these steps, we can accurately determine that the square root of \(\frac{25}{49}\) is \(\frac{5}{7}\).
Simplifying Fractions Before Finding Square Roots
Simplifying fractions before finding their square roots can make the process easier and ensure accuracy. When a fraction is in its simplest form, the calculation of its square root is more straightforward. Here is a step-by-step guide to simplifying fractions before finding their square roots:
-
Identify the greatest common divisor (GCD):
Find the GCD of the numerator and the denominator. The GCD is the largest number that divides both the numerator and the denominator without leaving a remainder.
- For example, in the fraction \(\frac{50}{100}\), the GCD of 50 and 100 is 50.
-
Divide both the numerator and the denominator by the GCD:
This step reduces the fraction to its simplest form.
- Continuing the example, \(\frac{50 \div 50}{100 \div 50} = \frac{1}{2}\).
-
Calculate the square root of the simplified fraction:
Once the fraction is simplified, find the square root of both the numerator and the denominator separately.
- For \(\frac{1}{2}\), calculate \(\sqrt{1} = 1\) and \(\sqrt{2}\), which is an irrational number (approximately 1.414).
Let’s apply this to another example: simplifying \(\frac{36}{64}\) before finding its square root.
- Identify the GCD of 36 and 64, which is 4.
- Divide both the numerator and the denominator by the GCD:
- \(\frac{36 \div 4}{64 \div 4} = \frac{9}{16}\)
- Now, find the square root of the simplified fraction \(\frac{9}{16}\):
- \(\sqrt{9} = 3\)
- \(\sqrt{16} = 4\)
- The square root of \(\frac{9}{16}\) is \(\frac{3}{4}\).
Here is a summary table for quick reference:
| Original Fraction | GCD | Simplified Fraction | Square Root of Simplified Fraction |
| \(\frac{50}{100}\) | 50 | \(\frac{1}{2}\) | \(\frac{1}{\sqrt{2}}\) |
| \(\frac{36}{64}\) | 4 | \(\frac{9}{16}\) | \(\frac{3}{4}\) |
By simplifying fractions before finding their square roots, we ensure that the calculations are easier and more precise. This method can be applied to any fraction to streamline the process of finding square roots.
Examples of Similar Square Root Calculations
To further understand how to find the square root of fractions, let's look at some similar examples. These examples will illustrate the step-by-step process of calculating the square root of different fractions.
-
Example 1: Square Root of \(\frac{16}{81}\)
- Identify the fraction: \(\frac{16}{81}\)
- Find the square root of the numerator: \(\sqrt{16} = 4\)
- Find the square root of the denominator: \(\sqrt{81} = 9\)
- Combine the results: \(\frac{\sqrt{16}}{\sqrt{81}} = \frac{4}{9}\)
-
Example 2: Square Root of \(\frac{9}{64}\)
- Identify the fraction: \(\frac{9}{64}\)
- Find the square root of the numerator: \(\sqrt{9} = 3\)
- Find the square root of the denominator: \(\sqrt{64} = 8\)
- Combine the results: \(\frac{\sqrt{9}}{\sqrt{64}} = \frac{3}{8}\)
-
Example 3: Square Root of \(\frac{4}{25}\)
- Identify the fraction: \(\frac{4}{25}\)
- Find the square root of the numerator: \(\sqrt{4} = 2\)
- Find the square root of the denominator: \(\sqrt{25} = 5\)
- Combine the results: \(\frac{\sqrt{4}}{\sqrt{25}} = \frac{2}{5}\)
-
Example 4: Square Root of \(\frac{49}{121}\)
- Identify the fraction: \(\frac{49}{121}\)
- Find the square root of the numerator: \(\sqrt{49} = 7\)
- Find the square root of the denominator: \(\sqrt{121} = 11\)
- Combine the results: \(\frac{\sqrt{49}}{\sqrt{121}} = \frac{7}{11}\)
Here is a summary table for quick reference:
| Fraction | Square Root of Numerator | Square Root of Denominator | Result |
| \(\frac{16}{81}\) | 4 | 9 | \(\frac{4}{9}\) |
| \(\frac{9}{64}\) | 3 | 8 | \(\frac{3}{8}\) |
| \(\frac{4}{25}\) | 2 | 5 | \(\frac{2}{5}\) |
| \(\frac{49}{121}\) | 7 | 11 | \(\frac{7}{11}\) |
These examples demonstrate how to find the square roots of various fractions by separately calculating the square roots of the numerators and denominators. With this method, you can confidently solve similar problems and understand the principles behind square root calculations.
Importance of Square Roots in Mathematics
The concept of square roots is fundamental in mathematics and appears frequently in various branches of this field. Here are several key reasons why square roots are important:
-
Basic Mathematical Operations:
Understanding square roots is essential for performing various mathematical operations. They are foundational for algebra, calculus, and other advanced math topics.
-
Solving Equations:
Square roots are often used to solve quadratic equations. For instance, the quadratic formula
x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}involves the square root of the discriminant. -
Geometry:
Square roots are crucial in geometry, particularly when dealing with right triangles and the Pythagorean theorem. For example, in a right triangle with sides of length \(a\), \(b\), and hypotenuse \(c\), the relationship is given by \(c = \sqrt{a^2 + b^2}\).
-
Real-World Applications:
Square roots are used in various real-world scenarios such as calculating areas, volumes, and in financial models. For example, the formula for the standard deviation in statistics includes the square root function.
-
Advanced Mathematics:
In more advanced mathematics, square roots are essential in complex number theory and certain algebraic structures. The concept of the square root of negative numbers leads to the introduction of imaginary numbers, defined as \(i = \sqrt{-1}\).
-
Scientific Calculations:
In science, square roots are often used in physics and engineering. For example, they appear in formulas for calculating energy, work, and other physical properties.
By mastering square roots, students and professionals can enhance their mathematical skills and apply these concepts in practical and theoretical problems effectively.
Applications of Square Roots in Real Life
Square roots play a crucial role in various real-life applications, ranging from everyday problem-solving to advanced scientific computations. Understanding how to apply square roots can help in multiple fields, including construction, finance, engineering, and physics. Here are some detailed examples of how square roots are used in practical scenarios:
- Construction and Architecture:
Square roots are essential in construction for calculating areas and determining the dimensions of materials. For instance, if an architect needs to design a square room with an area of 49 square meters, they can use the square root to find that each side of the room should be 7 meters long.
- Finance:
In finance, square roots are used in various calculations, such as determining the standard deviation in statistics. The standard deviation is a measure of the amount of variation or dispersion in a set of values. Calculating the square root of the variance gives the standard deviation, which is crucial for assessing investment risks.
- Engineering:
Engineers use square roots in formulas to determine stress, strain, and other mechanical properties of materials. For example, the calculation of the natural frequency of a structure involves taking the square root of the stiffness divided by the mass.
- Physics:
Square roots are widely used in physics, especially in formulas involving wave equations, quantum mechanics, and general relativity. For instance, the root mean square (RMS) value is used to calculate the effective voltage or current of an alternating current (AC) circuit.
- Medicine:
In medical imaging, such as MRI and CT scans, square roots are used to process signals and enhance image quality. The algorithms involved in these imaging techniques often require square root calculations to accurately reconstruct images from raw data.
- Everyday Life:
Square roots can also be seen in everyday activities such as calculating distances. For example, if you know the horizontal and vertical distances between two points, you can use the Pythagorean theorem to find the direct distance by calculating the square root of the sum of the squares of the two distances.

Common Mistakes to Avoid
Understanding and calculating the square root of a fraction such as can be tricky. Here are some common mistakes to avoid:
- Not Simplifying the Fraction First:
Before taking the square root, check if the fraction can be simplified. Simplifying the fraction first can make the process easier and reduce the chances of errors.
- Ignoring Perfect Squares:
Identify if the numerator and the denominator are perfect squares. For example, in the fraction , both 25 and 49 are perfect squares. Their square roots are 5 and 7, respectively, making the square root of the fraction equal to .
- Incorrectly Applying Square Roots:
When taking the square root of a fraction, apply the square root to both the numerator and the denominator separately. Do not combine them under a single square root symbol. For instance, should be calculated as , resulting in .
- Overlooking Rationalization:
In some cases, you may need to rationalize the denominator, especially if it is not a perfect square. This involves multiplying the numerator and the denominator by a value that makes the denominator a perfect square.
- Forgetting to Check the Final Answer:
Always simplify your final answer. After calculating the square root of a fraction, ensure that the result is in its simplest form. For example, if the fraction simplifies further, do so.
By being mindful of these common pitfalls, you can accurately and efficiently find the square roots of fractions.
Advanced Topics: Irrational and Rational Numbers
Understanding the distinction between rational and irrational numbers is fundamental in advanced mathematics. Let's explore these concepts in detail:
Rational Numbers
A rational number is any number that can be expressed as the quotient or fraction of two integers, where the numerator is an integer and the denominator is a non-zero integer. For example:
- \( \frac{5}{3} \)
- \( 5 \) (which can be written as \( \frac{5}{1} \))
- \( 0.75 \) (which can be written as \( \frac{3}{4} \))
Rational numbers include both terminating and repeating decimals.
Irrational Numbers
Irrational numbers cannot be expressed as a fraction of two integers. Their decimal representation is non-terminating and non-repeating. Examples include:
- \( \sqrt{2} \)
- \( \pi \)
- \( e \) (the base of natural logarithms)
Square Roots and Their Classification
Square roots play a crucial role in distinguishing between rational and irrational numbers. For example:
- \( \sqrt{25} = 5 \), a rational number
- \( \sqrt{49} = 7 \), a rational number
- \( \sqrt{2} \) is an irrational number because it cannot be simplified to a fraction of two integers
Properties and Operations
Let's delve into some properties and operations involving rational and irrational numbers:
- The sum of two rational numbers is always rational.
- The product of two rational numbers is always rational.
- The sum of a rational and an irrational number is always irrational.
- The product of a non-zero rational number and an irrational number is always irrational.
Practical Applications
Understanding rational and irrational numbers has practical applications in various fields such as engineering, physics, and finance. For example:
- Engineering: Calculations involving precise measurements often require rational numbers.
- Physics: Constants like \( \pi \) and \( e \) are irrational numbers used in formulas describing natural phenomena.
- Finance: Compound interest calculations can involve irrational numbers for continuous growth models.
Conclusion
Grasping the concepts of rational and irrational numbers enhances our ability to solve complex problems in mathematics and apply these solutions in real-world scenarios. Whether dealing with perfect squares or exploring the properties of surds, the distinction between these two types of numbers is essential for advanced mathematical understanding.
Conclusion and Summary
Understanding the square root of a fraction, such as \( \sqrt{\frac{25}{49}} \), is a fundamental mathematical skill with wide-reaching applications. By breaking down the process, we can simplify the fraction's square root as follows:
- Identify the square root of the numerator: \( \sqrt{25} = 5 \).
- Identify the square root of the denominator: \( \sqrt{49} = 7 \).
- Combine these results to get \( \sqrt{\frac{25}{49}} = \frac{5}{7} \).
This method can be applied to any fraction where both the numerator and the denominator are perfect squares. By simplifying each component separately, we can achieve the final simplified form easily.
In summary:
- The square root of a fraction is found by taking the square root of the numerator and the denominator separately.
- Simplifying fractions beforehand can make the process easier.
- Recognizing perfect squares can quickly guide you to the correct answer.
This principle is not only essential for academic purposes but also useful in various real-life applications where precise calculations are required. Mastering this skill builds a strong foundation for more advanced mathematical concepts.
With this understanding, you are now equipped to handle square roots of fractions confidently, avoiding common mistakes and applying these principles effectively in both theoretical and practical contexts.
Video hướng dẫn đơn giản hóa căn bậc hai của 25/49 bằng tiếng Việt, giúp người xem hiểu rõ hơn về quá trình tính toán và ứng dụng của nó.
Đơn giản hóa căn bậc hai của 25/49 || Căn 25/49
READ MORE:
Đơn Giản Hóa Căn Bậc Hai: sqrt(25/49)