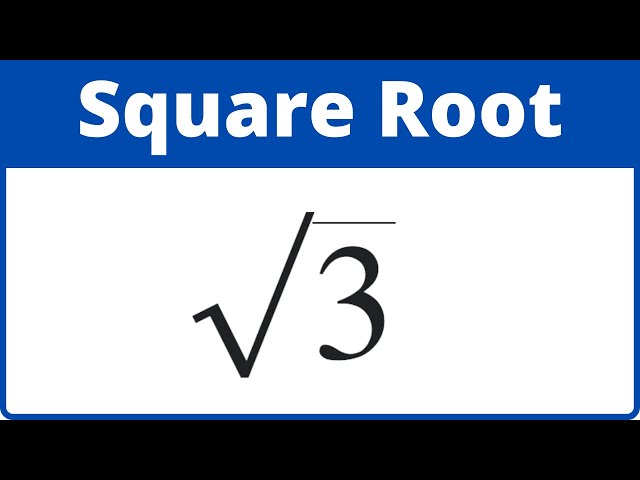
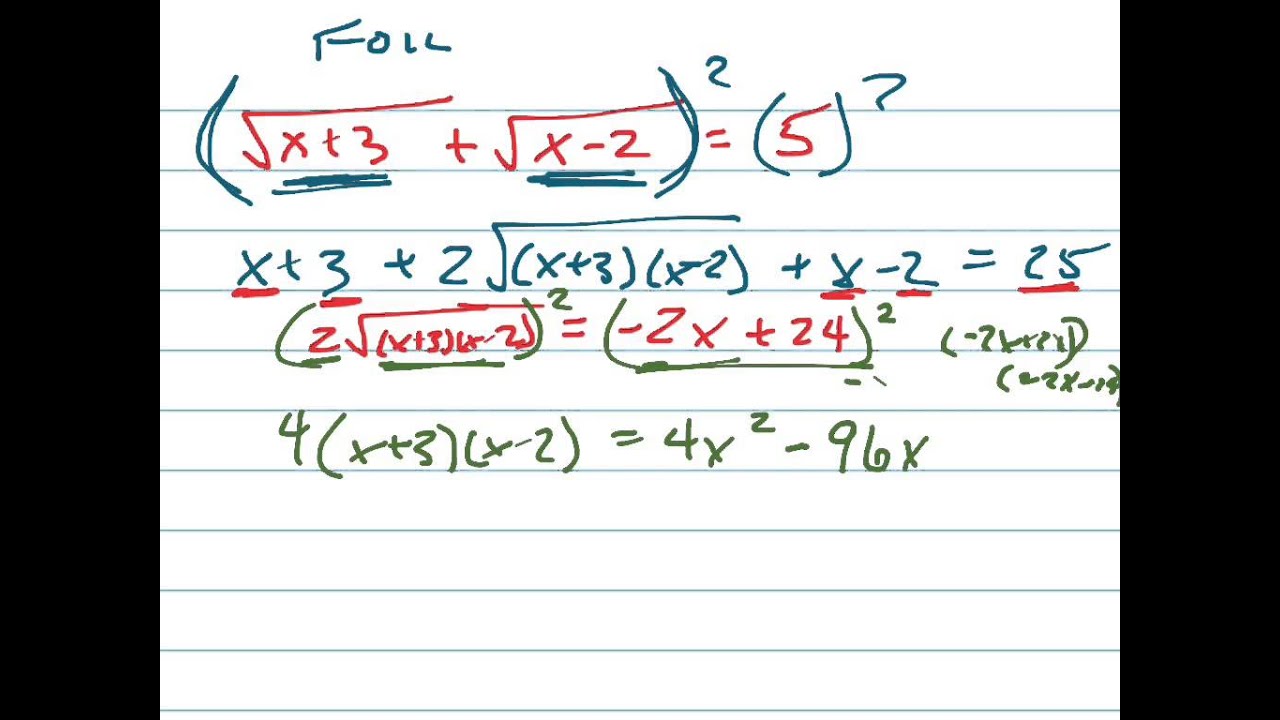Topic 3 square root: The square root of 3, often denoted as √3, is a fascinating and crucial number in mathematics. This article explores its properties, applications in geometry and science, and its significance in various fields. Whether you're a math enthusiast or curious about its real-world uses, discover the wonders of 3 square root with us!
Table of Content
- Understanding the Square Root of 3
- Introduction to the Square Root of 3
- Mathematical Properties and Significance
- Decimal Approximation and Rationality
- Applications in Geometry and Trigonometry
- Calculation Methods and Approximations
- Historical and Cultural Significance
- Role in Natural Phenomena and Structures
- Interesting Facts and Trivia
- Advanced Mathematical Contexts
- Practical Uses in Science and Engineering
- Summary and Key Takeaways
- YOUTUBE: Hướng dẫn chi tiết về cách tìm căn bậc hai của 3, với các phương pháp và ví dụ minh họa.
Understanding the Square Root of 3
The square root of 3, denoted as , is an important irrational number in mathematics. It is the positive real number that, when multiplied by itself, equals 3. In decimal form, it is approximately 1.73205.
Mathematical Properties
- The square root of 3 is represented as .
- It is an irrational number, meaning it cannot be expressed as a simple fraction.
- It has an infinite and non-repeating decimal expansion.
- It is often used in trigonometry, geometry, and calculus.
Decimal Approximation
The square root of 3 in decimal form is approximately 1.7320508075688772935. For practical purposes, it is often rounded to 1.732.
Applications in Geometry
The square root of 3 appears in various geometric contexts, such as:
- The height of an equilateral triangle with side length 2 is units, represented as in calculations.
- It is used to find the length of the diagonal of a cube.
- In trigonometry, it appears in the exact values of the tangent of 60 degrees and the sine of 60 degrees.
Calculation Methods
To find the square root of 3, several methods can be used:
- Using a calculator to directly compute the value.
- Using iterative methods such as the Newton-Raphson method to approximate it.
- Using series expansions to represent it as an infinite sum.
Fun Facts
Here are some interesting aspects of :
- It is part of the set of irrational square roots that include and .
- The square root of 3 is used in ancient architecture and art for its aesthetic proportions.
- It plays a role in the natural formation of hexagonal structures, such as in honeycombs and crystals.
Summary
The square root of 3 is a fundamental mathematical constant that appears in various fields from geometry to natural phenomena. Understanding its properties and applications can provide deeper insights into both theoretical and practical mathematics.

READ MORE:
Introduction to the Square Root of 3
The square root of 3, denoted as or √3, is a vital number in mathematics and its applications. This irrational number cannot be precisely expressed as a simple fraction, and its decimal form is approximately 1.73205. It frequently appears in various mathematical and scientific contexts, making it an essential concept to understand.
Here's a step-by-step exploration of the square root of 3:
- Definition: The square root of 3 is the number that, when multiplied by itself, equals 3. Symbolically, or .
- Decimal Representation: As an irrational number, √3 has an infinite and non-repeating decimal expansion. It begins as 1.7320508075688772935...
- Geometric Significance: √3 is crucial in geometry, particularly in the study of equilateral triangles and the Pythagorean theorem. For example:
- An equilateral triangle with sides of length 2 has a height of .
- The diagonal of a cube with unit side length is √3.
- Trigonometric Context: In trigonometry, √3 appears in the exact values of the sine and cosine functions at 30° and 60°. For instance:
- The tangent of 60° is or √3.
- Natural Occurrences: √3 is found in nature, such as in the structure of certain crystals and in the spacing of leaves and branches in plants.
Understanding the square root of 3 not only enriches one's knowledge of mathematics but also provides insights into its broader applications in science, engineering, and nature. Its pervasive presence across various disciplines highlights its importance and the beauty of mathematical concepts.
Mathematical Properties and Significance
The square root of 3, expressed as or √3, possesses several intriguing mathematical properties and plays a crucial role in various areas of mathematics and beyond. Below, we explore its properties and the significance it holds in different contexts:
- Irrationality:
The square root of 3 is an irrational number, which means it cannot be written as a simple fraction. Its decimal representation is non-terminating and non-repeating, approximately equal to 1.7320508075688772935... This property places it in the same category as other famous irrational numbers like √2 and π.
- Algebraic Properties:
- It satisfies the equation , meaning that squaring √3 gives 3: .
- In exponential form, it can be represented as .
- It is part of the solution set to quadratic equations of the form .
- Geometric Properties:
In geometry, √3 often appears in the context of equilateral triangles and hexagons. For instance:
- The height of an equilateral triangle with side length 2 is units, or .
- In a 30°-60°-90° triangle, the ratio of the sides opposite the 30° and 60° angles is .
- Trigonometric Significance:
√3 is significant in trigonometry, particularly in relation to certain angles:
- The tangent of 60° is equal to √3: .
- The sine of 60° is and the cosine of 30° is the same value.
- Presence in Mathematical Constants:
√3 is part of several mathematical constants and formulas, including:
- The volume of a tetrahedron with edge length 'a' is given by .
- It appears in the solution of the cubic equation .
- Application in Number Theory:
In number theory, √3 plays a role in various proofs and theorems, particularly those involving irrational numbers and their properties.
The square root of 3 is not just a number; it is a cornerstone in many mathematical theories and applications. Its properties and significance extend from pure mathematics into real-world applications, making it a subject worth exploring in depth.
Decimal Approximation and Rationality
The square root of 3, represented as or √3, is an important irrational number in mathematics. Understanding its decimal approximation and rationality provides insights into its unique nature and its role in various mathematical contexts.
- Decimal Approximation:
The square root of 3 is an irrational number, which means it cannot be expressed as a simple fraction and its decimal representation is infinite and non-repeating. The approximate value of √3 is:
- ≈ 1.7320508075688772935...
For practical purposes, it is often rounded to a few decimal places. Common approximations include:
- √3 ≈ 1.73 (rounded to two decimal places)
- √3 ≈ 1.732 (rounded to three decimal places)
- √3 ≈ 1.73205 (rounded to five decimal places)
- Methods of Approximation:
Various methods can be used to approximate the value of √3:
- Long Division Method: A manual technique to find square roots by iteratively estimating and refining the value.
- Newton-Raphson Method: An iterative numerical method that starts with an initial guess and refines it to approach the actual square root.
- Continued Fractions: Representing √3 as a continued fraction offers an exact way to approach its value:
- Rationality and Irrationality:
√3 is classified as an irrational number, which has significant implications:
- Definition: An irrational number cannot be written as a ratio of two integers. Thus, √3 cannot be expressed as , where p and q are integers.
- Proof of Irrationality: The irrationality of √3 can be proven through a method of contradiction, often involving the assumption that it can be expressed as a fraction, leading to a logical contradiction.
- Infinite Decimal Expansion: The non-repeating, non-terminating nature of its decimal expansion further confirms its irrationality.
- Significance in Mathematics:
The irrational nature and infinite decimal expansion of √3 are crucial in various mathematical applications:
- It plays a significant role in algebra, especially in solving quadratic equations.
- Its properties are essential in geometry, particularly in the study of triangles and circles.
- It appears in trigonometric functions and identities, enhancing our understanding of angles and their relationships.
Understanding the decimal approximation and irrationality of the square root of 3 reveals its complex and intriguing nature. This knowledge is fundamental in exploring deeper mathematical concepts and real-world applications where √3 plays a pivotal role.
Applications in Geometry and Trigonometry
The square root of 3, denoted as or √3, is a key value in both geometry and trigonometry. It appears in numerous fundamental structures and relationships within these fields, playing a crucial role in various calculations and theorems.
- Equilateral Triangles:
In an equilateral triangle, all sides are of equal length, and all angles are 60°. The height (or altitude) of such a triangle can be found using √3. For an equilateral triangle with side length 'a', the height 'h' is given by:
For example, if the side length is 2, the height will be:
, which simplifies to (approximately 1.732).
- 30°-60°-90° Triangles:
This special type of right triangle has sides in the ratio 1 : √3 : 2. Here’s how √3 comes into play:
- The side opposite the 30° angle (short leg) is half the length of the hypotenuse.
- The side opposite the 60° angle (long leg) is √3 times the length of the short leg.
For instance, if the hypotenuse is 2, the sides are 1 (short leg) and √3 (long leg).
- Hexagons:
In regular hexagons, which consist of six equilateral triangles, the distance from the center to a vertex (the radius) is the same as the side length. The distance between opposite vertices (the diameter) is 2√3 times the side length. This relationship is essential in understanding the symmetry and geometry of hexagons.
- Trigonometric Ratios:
√3 frequently appears in trigonometric functions associated with 30° and 60° angles:
- The tangent of 60° is √3:
- The sine of 60° is √3/2:
- The cosine of 30° is also √3/2:
- Distance and Diagonal Calculations:
√3 is also useful in finding distances and diagonals in geometric figures:
- In a cube with side length 'a', the space diagonal (the longest diagonal inside the cube) is √3 times the side length.
- In regular tetrahedrons, the distance between opposite vertices is √3 times the side length of the tetrahedron.
If the side length of the cube is 'a', the space diagonal 'd' is given by:
- Applications in Coordinate Geometry:
In coordinate geometry, √3 is used to calculate distances between points and to determine the slopes and angles of lines in relation to certain geometric shapes.
The square root of 3 is integral to understanding various geometric and trigonometric principles. Its recurring presence across different shapes and formulas highlights its importance and utility in mathematical problem-solving.

Calculation Methods and Approximations
The square root of 3, expressed as or √3, is an irrational number, meaning it cannot be represented as a simple fraction. However, there are several methods to calculate and approximate its value, each offering varying degrees of accuracy and complexity.
- Manual Calculation Methods:
- Long Division Method:
This traditional method involves a step-by-step division process to find the square root of a number. While it's time-consuming, it provides a good approximation by iteratively refining the result. Here's how it works:
- Pair the digits of the number from the decimal point.
- Find the largest number whose square is less than or equal to the first pair of digits.
- Subtract the square of this number from the first pair and bring down the next pair.
- Double the current quotient, and use it as a digit in the divisor, adjusting to find the next digit of the quotient.
- Repeat the process to achieve the desired precision.
- Prime Factorization Method:
Prime factorization involves breaking down the number into its prime factors. This method is useful for finding the square roots of perfect squares but can also provide insight into the roots of other numbers.
- Express 3 as the product of its prime factors (in this case, 3 itself).
- Pair the prime factors and take one number from each pair.
- For 3, since it is a prime number, this method shows it doesn't simplify neatly into pairs, indicating its irrationality.
- Long Division Method:
- Numerical Approximation Methods:
- Newton-Raphson Method:
This is an iterative method to approximate roots of real-valued functions. It's particularly efficient for finding the square roots of numbers. The method involves the following steps:
- Choose an initial guess . For √3, a reasonable start might be 1.5 or 2.
- Use the formula to find the next approximation.
- Repeat the process until the desired level of accuracy is reached. Typically, a few iterations will provide a very close approximation.
- Continued Fractions:
Continued fractions provide an exact representation of irrational numbers and offer a way to approximate them to any degree of accuracy. The continued fraction for √3 is:
By truncating this series at different points, one can get increasingly accurate approximations.
- Newton-Raphson Method:
- Common Approximations:
Several practical approximations of √3 are used in everyday calculations and estimations:
- ≈ 1.732 (rounded to three decimal places)
- ≈ 1.73205 (rounded to five decimal places)
- ≈ 1.732050807568877 (rounded to fifteen decimal places)
- Use of Calculators and Software:
Modern technology provides tools such as calculators and computer software to find the square root of 3 to many decimal places quickly and accurately. These tools use sophisticated algorithms to deliver results efficiently and are invaluable in both educational and professional settings.
Whether through manual methods or advanced numerical techniques, the calculation and approximation of √3 demonstrate the fascinating blend of simplicity and complexity in mathematics. Understanding these methods deepens our appreciation of this fundamental number.
Historical and Cultural Significance
The square root of 3, expressed as or √3, has intrigued mathematicians and scholars across various cultures and historical periods. Its unique properties and appearances in different fields have made it a symbol of mathematical beauty and complexity.
- Ancient Mathematics and Geometry:
√3 has been known and studied since ancient times. Its geometric significance, especially in the context of equilateral triangles and hexagons, made it a valuable number for early mathematicians:
- Egyptian and Babylonian Mathematicians:
Ancient civilizations such as the Egyptians and Babylonians used approximations of √3 in their architectural designs and astronomical calculations. Their ability to work with irrational numbers like √3 demonstrated advanced mathematical understanding.
- Greek Geometry:
Greek mathematicians, including Euclid, explored the properties of √3 in their studies of geometry. The Greeks' work on constructing geometric figures, including regular polygons, often involved the use of √3.
- Egyptian and Babylonian Mathematicians:
- Medieval Islamic Mathematics:
During the Islamic Golden Age, scholars made significant contributions to mathematics, including more precise calculations and approximations of √3. They developed methods that closely resemble modern algebra and trigonometry, where √3 plays a crucial role.
- Renaissance and Enlightenment Periods:
The Renaissance and Enlightenment periods saw a revival of interest in classical mathematics and the development of new mathematical theories. During this time, √3 was essential in the work of mathematicians who laid the foundations for modern calculus and number theory:
- Leonardo Fibonacci:
In his famous book "Liber Abaci," Fibonacci introduced the Western world to Hindu-Arabic numerals and included discussions on square roots, including √3, influencing the course of mathematical education in Europe.
- Isaac Newton:
Newton's development of the Newton-Raphson method provided a new way to approximate the square roots of numbers, including √3, more efficiently and accurately.
- Leonardo Fibonacci:
- Cultural Symbolism:
√3 has also found its place in cultural contexts, symbolizing harmony and balance in various traditions:
- Religious Symbolism:
In some cultures, the square root of 3 is associated with sacred geometry, which explores the mathematical principles that underlie natural patterns and structures.
- Art and Architecture:
Artists and architects have used the properties of √3 in their work to create aesthetically pleasing and structurally sound designs. For example, the proportions of certain elements in Gothic cathedrals are based on √3.
- Religious Symbolism:
- Modern Applications and Symbolism:
In contemporary times, √3 continues to be an important figure in both theoretical and applied mathematics. It also carries symbolic meanings in various fields:
- Mathematical Constants:
√3 is part of a group of fundamental mathematical constants that are critical in various scientific and engineering calculations. Its role in solving problems involving triangle geometry, particularly in physics and engineering, remains vital.
- Popular Culture:
In popular culture, √3 often appears in discussions about mathematical beauty and the elegance of numbers. It serves as a reminder of the interconnectedness of mathematics and the natural world.
- Mathematical Constants:
The square root of 3 is more than just a number; it is a symbol of the enduring quest for knowledge and the elegance of mathematical discovery. Its presence throughout history and across cultures underscores its profound impact and timeless relevance.
Role in Natural Phenomena and Structures
The square root of 3, denoted as or √3, is not only a critical mathematical constant but also a value that appears naturally in various phenomena and structures. Its unique properties contribute to the patterns and relationships found in nature.
- Crystal Lattices and Molecular Structures:
In crystallography, the geometric arrangement of atoms in crystals often involves the square root of 3. This relationship is crucial for understanding the structure and stability of different materials:
- Hexagonal Close Packing (HCP):
In HCP structures, which include metals like magnesium and titanium, the atoms are packed in layers where each atom touches three atoms in the layer above and three in the layer below. The distance between the centers of these atoms across the diagonal of the hexagon involves the factor √3.
- Graphene and Honeycomb Lattices:
Graphene, a single layer of carbon atoms arranged in a hexagonal lattice, demonstrates a repeating pattern where the distance between adjacent carbon atoms is related to √3. This structure gives graphene its remarkable strength and electrical properties.
- Hexagonal Close Packing (HCP):
- Biological Patterns:
√3 is found in various biological forms and structures, reflecting the inherent efficiency and symmetry in nature:
- Beehive Honeycombs:
Honeybees construct their hives using hexagonal cells. The hexagonal shape, which maximizes the use of space and minimizes material, relies on geometric properties involving √3. The distance between opposite sides of each hexagon is equal to √3 times the side length, creating an efficient and stable structure.
- DNA and Molecular Geometry:
The geometric configuration of the DNA double helix and other molecular structures often exhibit patterns where √3 plays a role. For instance, the angles and distances between atoms in certain molecular formations can be described using √3, contributing to the stability and function of these molecules.
- Beehive Honeycombs:
- Geometric Shapes and Natural Forms:
√3 appears in the proportions and dimensions of various natural shapes and forms, highlighting the deep connection between mathematics and nature:
- Hexagonal Patterns in Nature:
Hexagonal shapes are prevalent in natural phenomena, such as the pattern of basalt columns in the Giant's Causeway or the shape of snowflakes. The geometry of these hexagons involves the square root of 3, contributing to their stability and efficiency.
- Triangles and Tetrahedra:
Equilateral triangles and tetrahedral shapes, which are common in natural and man-made structures, often involve √3 in their calculations. The height of an equilateral triangle with side length 'a' is , and the spatial diagonal of a cube, formed by connecting opposite corners, is .
- Hexagonal Patterns in Nature:
- Acoustic and Wave Phenomena:
√3 also appears in the study of waves and sound. The relationship between wavelengths, frequencies, and the speed of sound or light can involve √3 in their calculations:
- Vibrations and Resonance:
In acoustics, the resonant frequencies of vibrating objects, such as strings or air columns, can be described using mathematical ratios that include √3. These relationships are essential for understanding musical tones and harmonics.
- Optical Phenomena:
In optics, the angles of refraction and diffraction, as well as the spacing of wavefronts in certain crystal structures, can involve √3, influencing the behavior of light and other electromagnetic waves.
- Vibrations and Resonance:
- Astrophysics and Space Geometry:
In the vast scales of astrophysics, √3 emerges in various contexts, from the geometry of celestial objects to the arrangement of matter in the universe:
- Triangular and Hexagonal Tessellations:
In the study of gravitational fields and cosmic structures, triangular and hexagonal tessellations, which often involve √3, help model the distribution and dynamics of matter in space.
- Orbital Mechanics:
The stability and resonance of planetary orbits can sometimes be described using ratios that include √3, offering insights into the natural order of celestial systems.
- Triangular and Hexagonal Tessellations:
The presence of √3 in natural phenomena and structures underscores the profound and ubiquitous role of mathematics in describing the world around us. Its appearance in diverse contexts highlights the inherent efficiency and beauty of mathematical relationships in nature.
Interesting Facts and Trivia
The square root of 3, often represented as √3, is an irrational number with several intriguing properties and applications. Here are some interesting facts and trivia about √3:
- Irrationality: The square root of 3 cannot be expressed as a simple fraction. Its decimal representation is non-repeating and non-terminating, approximately equal to 1.73205080757.
- Hexagon Side Length: In a regular hexagon inscribed in a unit circle, the length of each side is √3.
- Relationship with Equilateral Triangle: The height of an equilateral triangle with side length 2 is √3. This can be derived using the Pythagorean theorem.
- Approximation in Ancient Texts: The ancient Indian mathematician Aryabhata approximated √3 as 1.7320508, a remarkably accurate value for his time.
- Trigonometric Importance: The square root of 3 appears in various trigonometric identities and functions. For instance, tan(60°) = √3 and sin(60°) = (√3)/2.
- Engineering and Physics: The value of √3 is used in electrical engineering, especially in three-phase power systems where it relates to phase voltages and line voltages.
- Art and Architecture: The square root of 3 has been used in art and architecture to achieve aesthetically pleasing proportions and symmetry.
- Geometric Constructions: √3 is used in constructing certain geometric shapes and solving problems related to circles, triangles, and other polygons.
- Mathematical Constants: √3 is one of the few mathematical constants that is both a solution to a polynomial equation and a critical value in trigonometric calculations.
- Historical Calculations: Chinese mathematician Liu Hui calculated √3 to an accuracy of six decimal places in the 3rd century.
These facts illustrate the mathematical beauty and practical importance of the square root of 3, making it a fascinating topic in both theoretical and applied contexts.
Advanced Mathematical Contexts
The square root of 3 (\(\sqrt{3}\)) appears in various advanced mathematical contexts, highlighting its significance beyond basic arithmetic. Here are some key areas where \(\sqrt{3}\) plays a crucial role:
- Algebraic Structures: In algebra, \(\sqrt{3}\) is often involved in solving quadratic equations and in expressions where simplification of radicals is required. Its presence helps in understanding the properties of polynomials and algebraic equations.
- Geometry and Trigonometry:
- Equilateral Triangles: The height of an equilateral triangle with side length 1 is \(\frac{\sqrt{3}}{2}\). This relationship is fundamental in calculating the area and understanding the symmetry of equilateral triangles.
- Hexagons: The distance between opposite vertices (diameter of the circumcircle) in a regular hexagon with side length 1 is \(\sqrt{3}\). This is crucial for tessellation and in studying the geometric properties of hexagons.
- Trigonometric Functions: In trigonometry, \(\sqrt{3}\) is associated with the sine, cosine, and tangent functions of specific angles. For instance, \(\tan(60^\circ) = \sqrt{3}\).
- Calculus: \(\sqrt{3}\) appears in various calculus problems involving limits, derivatives, and integrals. For example, integrating functions that include \(\sqrt{3}\) helps in understanding more complex areas of motion and change.
- Complex Numbers: When extending to the complex plane, \(\sqrt{3}\) can be used in polar form representations of complex numbers, particularly in problems involving the cube roots of unity.
- Number Theory: In number theory, \(\sqrt{3}\) is studied in the context of irrational numbers. Its infinite, non-repeating decimal expansion exemplifies the nature of irrational numbers.
- Physics and Engineering: \(\sqrt{3}\) is used in physics and engineering, especially in three-phase electrical systems where the line voltage is \(\sqrt{3}\) times the phase voltage. This relationship is vital for the design and analysis of electrical systems.
- Optimization Problems: In optimization, \(\sqrt{3}\) helps in solving problems related to maximizing or minimizing areas and volumes. For instance, optimizing the shape of containers or structures often involves calculations using \(\sqrt{3}\).
These contexts demonstrate the broad application of \(\sqrt{3}\) in advanced mathematics and its importance in various scientific and engineering fields. Understanding \(\sqrt{3}\) not only enriches one's mathematical knowledge but also provides tools for practical problem-solving in diverse disciplines.
Practical Uses in Science and Engineering
The square root of 3, often represented as
1. Electrical Engineering
In electrical engineering,
The line-to-line voltage
\(V_{LL}\) is related to the line-to-neutral voltage\(V_{LN}\) by:\[ V_{LL} = \sqrt{3} \cdot V_{LN} \] This relationship is critical in ensuring proper voltage levels and efficient power distribution.
2. Mechanical Engineering
In mechanical engineering, the square root of 3 is used in the analysis of stress and strain in materials, particularly in the context of Mohr's Circle for plane stress.
Mohr's Circle uses
\(\sqrt{3}\) to determine principal stresses and maximum shear stresses.The transformation equations involve the factor
\(\sqrt{3}\) for calculating these critical stress values.
3. Structural Engineering
Structural engineers use
Equilateral triangles, which have sides of equal length, frequently appear in truss designs. The height (altitude) of an equilateral triangle is calculated using
\(\sqrt{3}\) :\[ h = \frac{\sqrt{3}}{2} \cdot a \] where
\(a\) is the length of a side.This property helps in determining the stability and load distribution in truss structures.
4. Chemistry
In chemistry, the square root of 3 appears in the study of molecular geometry, particularly in tetrahedral structures.
The bond angles in a perfect tetrahedron are approximately 109.5 degrees, which can be derived using
\(\sqrt{3}\) .This geometric understanding is crucial for predicting the shapes and angles of complex molecules.
5. Physics
In physics,
For example, in the analysis of standing waves on a string or in a cavity, the nodes and antinodes are separated by distances proportional to
\(\sqrt{3}\) .This constant also appears in the equations governing the resonance frequencies of these systems.
6. Computer Graphics
In computer graphics and geometric modeling, the square root of 3 is used in algorithms for rendering and mesh generation.
The subdivision surfaces and mesh refinement techniques often utilize
\(\sqrt{3}\) to achieve smoother and more accurate representations of 3D models.This leads to enhanced visual quality and realism in computer-generated imagery.
7. Architecture
In architecture, the aesthetic and structural properties of equilateral triangles, which involve
Domes, arches, and various structural elements incorporate the principles of equilateral triangles to distribute loads efficiently and achieve visually appealing designs.
The use of
\(\sqrt{3}\) in these designs contributes to both the functionality and beauty of architectural works.
Summary and Key Takeaways
The square root of 3, represented as
Irrational Number: The square root of 3 is an irrational number, which means its decimal representation is non-terminating and non-repeating. The value of
\(\sqrt{3}\) is approximately 1.73205.Mathematical Significance:
\(\sqrt{3}\) appears in various mathematical contexts, including geometry, trigonometry, and algebra. It is the positive solution of the equation\(x^2 = 3\) .Applications in Science and Engineering: The square root of 3 is crucial in fields like electrical engineering for three-phase power systems, mechanical engineering for stress analysis, and structural engineering for truss design. It is also used in chemistry for molecular geometry and in physics for wave phenomena.
Geometric Applications: In geometry,
\(\sqrt{3}\) is used to determine the height of equilateral triangles and the distances in tetrahedral structures. It also plays a role in calculating areas and volumes in various geometric shapes.Historical and Cultural Significance: Historically, the square root of 3 has been known since ancient times and has been studied by mathematicians from different cultures. It has appeared in various historical mathematical texts and applications.
Practical Uses: Beyond theoretical mathematics, the square root of 3 is used in practical scenarios such as computer graphics for mesh generation, architecture for structural designs, and other engineering calculations.
Approximation Methods: There are several methods to approximate
\(\sqrt{3}\) , including iterative methods like the Newton-Raphson method and long division. These methods provide increasingly accurate values for practical computations.
In summary, the square root of 3 is a vital mathematical constant with extensive applications in various scientific and engineering disciplines. Its unique properties and versatility make it an essential concept in both theoretical and practical contexts.
Hướng dẫn chi tiết về cách tìm căn bậc hai của 3, với các phương pháp và ví dụ minh họa.
Cách tìm Căn Bậc Hai của 3
READ MORE:
Video chứng minh rằng căn bậc hai của 3 là số vô tỉ, với các giải thích chi tiết và phương pháp logic.
Chứng Minh: Căn Bậc Hai của 3 là Số Vô Tỉ