Area and Perimeter of Triangles: Essential Formulas and Practical Examples

Triangles are one of the basic shapes in geometry. Understanding their properties, including area and perimeter, is fundamental in mathematics. Below are detailed explanations and formulas for calculating the area and perimeter of various types of triangles.

Perimeter of a Triangle

The perimeter of a triangle is the sum of the lengths of its sides. For a triangle with sides \( a \), \( b \), and \( c \), the perimeter \( P \) is given by:

\[
P = a + b + c
\]

Area of a Triangle

The area of a triangle can be calculated using different formulas depending on the information available. Here are the most commonly used methods:

1. Using Base and Height

If the base \( b \) and height \( h \) of the triangle are known, the area \( A \) is given by:

\[
A = \frac{1}{2} \times b \times h
\]

2. Using Heron's Formula

When the lengths of all three sides are known, Heron's formula can be used. First, calculate the semi-perimeter \( s \) of the triangle:

\[
s = \frac{a + b + c}{2}
\]

Then, the area \( A \) is given by:

\[
A = \sqrt{s \times (s - a) \times (s - b) \times (s - c)}
\]

3. Using Trigonometry

If two sides and the included angle are known, the area can be calculated using the formula:

\[
A = \frac{1}{2} \times a \times b \times \sin(C)
\]

where \( C \) is the angle between sides \( a \) and \( b \).

Types of Triangles and Their Properties

Triangles can be classified based on their sides and angles. Here are the main types:

• Equilateral Triangle: All sides are equal, and all angles are 60°.
• Isosceles Triangle: Two sides are equal, and the angles opposite these sides are equal.
• Scalene Triangle: All sides and angles are different.
• Right Triangle: One angle is 90°. The side opposite the right angle is the hypotenuse.

Example Problems

1. Perimeter Example

Calculate the perimeter of a triangle with sides 5 cm, 7 cm, and 10 cm:

\[
P = 5 + 7 + 10 = 22 \text{ cm}
\]

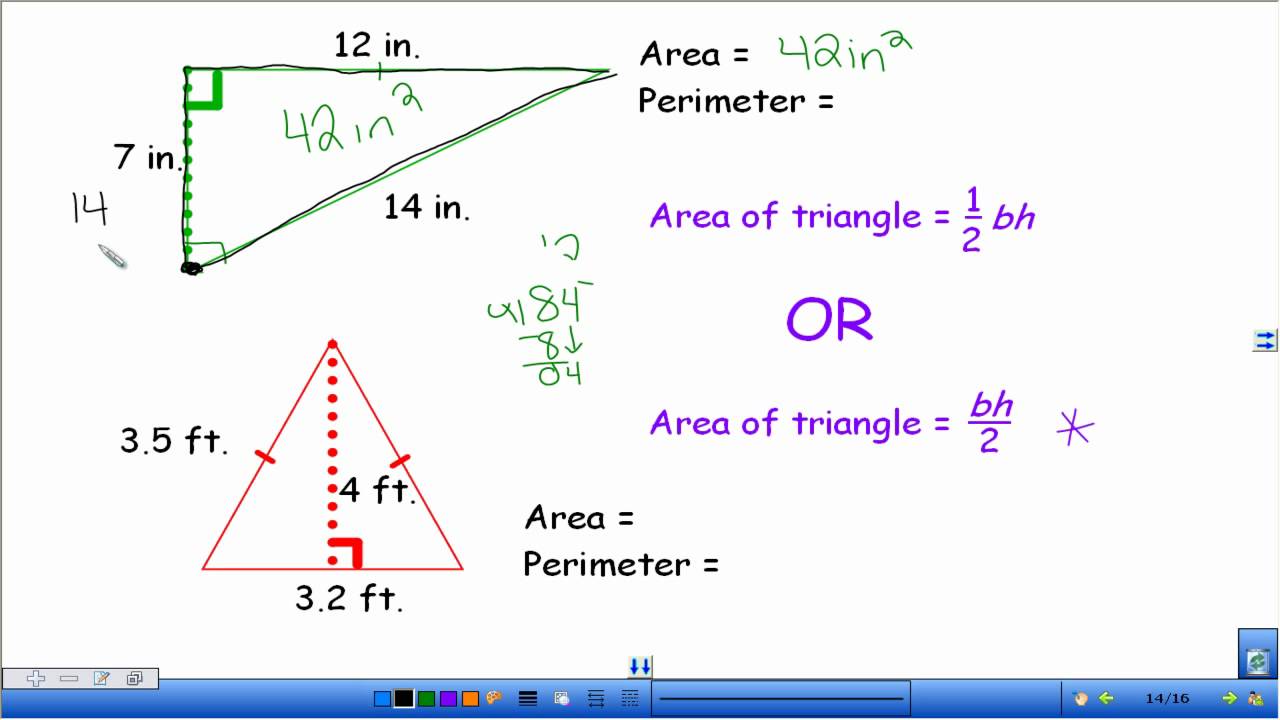
2. Area Example (Base and Height)

Calculate the area of a triangle with base 8 cm and height 5 cm:

\[
A = \frac{1}{2} \times 8 \times 5 = 20 \text{ cm}^2
\]

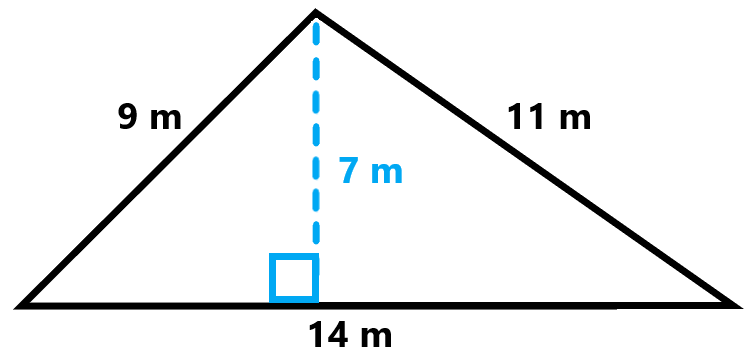
3. Area Example (Heron's Formula)

Calculate the area of a triangle with sides 6 cm, 8 cm, and 10 cm:

\[
s = \frac{6 + 8 + 10}{2} = 12
\]
\[
A = \sqrt{12 \times (12 - 6) \times (12 - 8) \times (12 - 10)} = \sqrt{12 \times 6 \times 4 \times 2} = \sqrt{576} = 24 \text{ cm}^2
\]

Conclusion

Understanding how to calculate the area and perimeter of triangles is essential in geometry. Using the correct formula based on the given information allows for accurate computations and a deeper appreciation of this fundamental shape.

Triangles are one of the simplest yet most fundamental shapes in geometry, characterized by having three sides, three vertices, and three angles. They are polygonal shapes that play a crucial role in various fields such as mathematics, engineering, architecture, and art. Understanding the properties of triangles is essential for solving many geometric problems and for the application of various mathematical concepts.

There are several key properties and types of triangles, each with unique characteristics:

• Equilateral Triangle: All three sides are of equal length, and all three interior angles are 60 degrees.
• Isosceles Triangle: Has at least two sides of equal length, and the angles opposite these sides are equal.
• Scalene Triangle: All sides and angles are of different lengths and measures.
• Right Triangle: One of the angles is exactly 90 degrees, which is the defining feature of this type of triangle.

Triangles are not only classified by their sides but also by their angles. Based on angles, triangles can be classified as:

• Acute Triangle: All three angles are less than 90 degrees.
• Obtuse Triangle: One of the angles is greater than 90 degrees.
• Right Triangle: One angle is exactly 90 degrees.

The study of triangles includes understanding concepts such as the perimeter, which is the sum of the lengths of all sides, and the area, which is the amount of space enclosed within the triangle. The basic formulas for calculating these are:

• Perimeter: \( P = a + b + c \) where \( a \), \( b \), and \( c \) are the lengths of the sides.
• Area (using base and height): \( A = \frac{1}{2} \times \text{base} \times \text{height} \)

Advanced methods like Heron's formula and trigonometric principles can also be used to determine the area when specific information about the triangle's sides and angles is known. This makes triangles versatile and widely applicable in various practical and theoretical problems.

A triangle is a three-sided polygon that has three edges and three vertices. It is one of the basic shapes in geometry and has several unique properties that distinguish it from other shapes. Understanding these properties is crucial for solving problems related to the area and perimeter of triangles.

Properties of Triangles

• Sum of Interior Angles: The sum of the interior angles of a triangle is always \(180^\circ\). This is a fundamental property of all triangles.
• Types of Triangles by Sides:
  • Scalene Triangle: A triangle with all sides of different lengths. Consequently, all three interior angles are different.
  • Isosceles Triangle: A triangle with two sides of equal length. The angles opposite these sides are also equal.
  • Equilateral Triangle: A triangle with all three sides of equal length. Each interior angle in an equilateral triangle is \(60^\circ\).
• Types of Triangles by Angles:
  • Acute Triangle: A triangle where all three interior angles are less than \(90^\circ\).
  • Right Triangle: A triangle with one interior angle equal to \(90^\circ\). The side opposite this angle is called the hypotenuse.
  • Obtuse Triangle: A triangle with one interior angle greater than \(90^\circ\).
• Perimeter: The perimeter of a triangle is the sum of the lengths of its sides. If a triangle has sides of lengths \(a\), \(b\), and \(c\), then the perimeter \(P\) is given by: \[ P = a + b + c \]
• Area: The area \(A\) of a triangle can be calculated using different formulas depending on the available information:
  • Using base \(b\) and height \(h\): \[ A = \frac{1}{2} \times b \times h \]
  • Using Heron's formula, when all side lengths \(a\), \(b\), and \(c\) are known: \[ s = \frac{a + b + c}{2} \] \[ A = \sqrt{s(s - a)(s - b)(s - c)} \]
  • Using trigonometry, when two sides and the included angle are known: \[ A = \frac{1}{2} \times a \times b \times \sin(C) \]

Triangles can be classified based on the lengths of their sides or the measures of their angles. Below are the main types of triangles:

Based on Sides

• Scalene Triangle: A triangle in which all three sides have different lengths. As a result, all three angles are also different.
• Isosceles Triangle: A triangle with two sides of equal length. The angles opposite these sides are also equal.
• Equilateral Triangle: A triangle in which all three sides are of equal length. Each of the internal angles in an equilateral triangle is 60°.

Based on Angles

• Acute Triangle: A triangle in which all three interior angles are less than 90°.
• Right Triangle: A triangle with one interior angle equal to 90°. The side opposite this angle is the hypotenuse, the longest side of the triangle.
• Obtuse Triangle: A triangle in which one of the interior angles is greater than 90°.

Each type of triangle has unique properties and can be found in various geometric problems and real-world applications. Understanding these types is fundamental to studying geometry and solving problems related to the area and perimeter of triangles.

An equilateral triangle is a special type of triangle in which all three sides are equal in length, and all three internal angles are equal to 60 degrees. This unique property makes it a regular polygon, meaning it is both equilateral (all sides are equal) and equiangular (all angles are equal).

Properties of Equilateral Triangles

• All sides are equal: \( a = b = c \)
• All internal angles are equal to 60 degrees: \( \angle A = \angle B = \angle C = 60^\circ \)
• It is a regular polygon with 3 sides.
• The centroid, orthocenter, circumcenter, and incenter are all the same point.
• The altitude, median, and angle bisector for each side are the same line.

Perimeter of an Equilateral Triangle

The perimeter of an equilateral triangle is calculated by adding all three sides:

\[
P = 3a
\]

Area of an Equilateral Triangle

The area of an equilateral triangle can be found using the formula:

\[
A = \frac{\sqrt{3}}{4} a^2
\]

Example Calculations

Example 1: Finding the Area

Find the area of an equilateral triangle with a side length of 6 cm.

1. Using the formula: \[ A = \frac{\sqrt{3}}{4} a^2 \]
2. Substitute \( a = 6 \): \[ A = \frac{\sqrt{3}}{4} (6)^2 = \frac{\sqrt{3}}{4} \times 36 = 9\sqrt{3} \, \text{cm}^2 \]

Example 2: Finding the Perimeter

Find the perimeter of an equilateral triangle with a side length of 8 inches.

1. Using the formula: \[ P = 3a \]
2. Substitute \( a = 8 \): \[ P = 3 \times 8 = 24 \, \text{inches} \]

Example 3: Finding the Side Length from Area

Find the side length of an equilateral triangle with an area of 16√3 cm².

1. Using the area formula: \[ A = \frac{\sqrt{3}}{4} a^2 \]
2. Rearrange to solve for \( a \): \[ a^2 = \frac{4A}{\sqrt{3}} \]
3. Substitute \( A = 16\sqrt{3} \): \[ a^2 = \frac{4 \times 16\sqrt{3}}{\sqrt{3}} = 64 \]
4. Taking the square root of both sides: \[ a = 8 \, \text{cm} \]

An isosceles triangle is a type of triangle that has two sides of equal length. These two equal sides are called the legs, and the third side is called the base. The angles opposite the equal sides are also equal.

Properties of Isosceles Triangles

• The two equal sides are called the legs.
• The angle between the two legs is called the vertex angle.
• The base angles, which are opposite the legs, are equal.
• The perpendicular bisector of the base bisects the vertex angle and is also the median and the altitude of the triangle.

Area of an Isosceles Triangle

The area of an isosceles triangle can be calculated using several methods depending on the known parameters:

• Using Base and Height: The formula is \( \text{Area} = \frac{1}{2} \times \text{base} \times \text{height} \). For example, if the base \( b \) is 10 cm and the height \( h \) is 17 cm, then the area is \( \frac{1}{2} \times 10 \times 17 = 85 \, \text{cm}^2 \).
• Using All Three Sides: The formula is \( \text{Area} = \frac{1}{2} \times b \times \sqrt{a^2 - \frac{b^2}{4}} \), where \( a \) is the length of the equal sides and \( b \) is the base.
• Using the Length of Two Sides and the Included Angle: The formula is \( \text{Area} = \frac{1}{2} \times a \times a \times \sin(\alpha) \), where \( a \) is the length of the equal sides and \( \alpha \) is the vertex angle.
• For an Isosceles Right Triangle: The formula is \( \text{Area} = \frac{a^2}{2} \), where \( a \) is the length of the equal sides.

Perimeter of an Isosceles Triangle

The perimeter of an isosceles triangle is the total length of all its sides. The formula is \( \text{Perimeter} = 2a + b \), where \( a \) is the length of the equal sides and \( b \) is the base.

• For example, if each of the equal sides \( a \) is 10 cm and the base \( b \) is 6 cm, then the perimeter is \( 2 \times 10 + 6 = 26 \, \text{cm} \).

Examples

1. Find the area of an isosceles triangle with a base of 24 cm and equal sides of 13 cm.
   • Using the formula \( \text{Area} = \frac{1}{2} \times b \times \sqrt{a^2 - \frac{b^2}{4}} \):
   • Area = \( \frac{1}{2} \times 24 \times \sqrt{13^2 - \frac{24^2}{4}} = \frac{1}{2} \times 24 \times \sqrt{169 - 144} = \frac{1}{2} \times 24 \times \sqrt{25} = \frac{1}{2} \times 24 \times 5 = 60 \, \text{cm}^2 \).
2. Find the perimeter of an isosceles triangle where the equal sides are 7 cm each, and the base is 10 cm.
   • Using the formula \( \text{Perimeter} = 2a + b \):
   • Perimeter = \( 2 \times 7 + 10 = 14 + 10 = 24 \, \text{cm} \).

Conclusion

Understanding the properties, area, and perimeter of isosceles triangles is crucial in geometry. These formulas and properties can be applied to solve various mathematical problems involving isosceles triangles.

A scalene triangle is a type of triangle where all three sides and all three angles are different. This means that no sides are of equal length and no angles are of equal measure. The unique properties of scalene triangles make them an interesting subject of study in geometry.

Properties of Scalene Triangles

• No equal sides.
• No equal angles.
• Does not have any lines of symmetry.
• The angles of a scalene triangle always add up to \(180^\circ\).
• It can be classified further into acute, obtuse, or right triangles depending on its angles.

Types of Scalene Triangles

• Acute Scalene Triangle: All three angles are less than \(90^\circ\).
• Obtuse Scalene Triangle: One angle is greater than \(90^\circ\) and the other two angles are less than \(90^\circ\).
• Right Scalene Triangle: One angle is exactly \(90^\circ\).

Formulas for Scalene Triangles

To calculate various properties of a scalene triangle, we use specific formulas:

Perimeter

The perimeter \(P\) of a scalene triangle is the sum of all its sides:

\[
P = a + b + c
\]
where \(a\), \(b\), and \(c\) are the lengths of the sides.

Area

The area \(A\) can be calculated using different methods, including the general triangle area formula and Heron's formula:

Using Base and Height

\[
A = \frac{1}{2} \times \text{base} \times \text{height}
\]

Using Heron's Formula

First, find the semi-perimeter \(s\):
\[
s = \frac{a + b + c}{2}
\]
Then, the area is:
\[
A = \sqrt{s(s-a)(s-b)(s-c)}
\]
where \(a\), \(b\), and \(c\) are the lengths of the sides of the triangle.

Example Calculations

Example 1: Perimeter

For a scalene triangle with sides 7 cm, 8 cm, and 10 cm, the perimeter is:
\[
P = 7 + 8 + 10 = 25 \text{ cm}
\]

Example 2: Area Using Heron's Formula

For a triangle with sides 5 cm, 6 cm, and 7 cm:
\[
s = \frac{5 + 6 + 7}{2} = 9 \text{ cm}
\]
\[
A = \sqrt{9(9-5)(9-6)(9-7)} = \sqrt{9 \times 4 \times 3 \times 2} = \sqrt{216} \approx 14.7 \text{ cm}^2
\]

A right triangle is a type of triangle that has one angle equal to 90 degrees. This angle is called the right angle. The sides of a right triangle have special names: the side opposite the right angle is the hypotenuse, and the other two sides are called the legs. Right triangles have unique properties that make them useful in various applications, including geometry, trigonometry, and real-world problem solving.

Properties of Right Triangles

• The sum of the two non-right angles in a right triangle is always 90 degrees.
• The hypotenuse is always the longest side of the right triangle.
• Pythagoras' Theorem is a fundamental relation in Euclidean geometry among the three sides of a right triangle.

Pythagoras' Theorem

Pythagoras' Theorem states that in a right triangle, the square of the length of the hypotenuse (c) is equal to the sum of the squares of the lengths of the other two sides (a and b).

The formula is:

$$

c2
=
a2
+
b2

Calculating the Perimeter of a Right Triangle

The perimeter of a right triangle is the sum of the lengths of all three sides. Using Pythagoras' Theorem, you can find the hypotenuse if the lengths of the legs are known.

Perimeter formula:

$$

P
=
a
+
b
+
c

Example of Perimeter Calculation

Given a right triangle with legs a = 3 units and b = 4 units, the hypotenuse c can be calculated as follows:

$$

32
+
42
=
c2

$$

9
+
16
=
c2

$$

25
=
c2

$$

c
=
25
=
5

Thus, the perimeter is:

$$

3
+
4
+
5
=
12
units

Calculating the Area of a Right Triangle

The area of a right triangle can be easily calculated by using the lengths of the two legs. The formula for the area is:

$$

Area
=


a
×
b

2

Example of Area Calculation

Using the same right triangle with legs a = 3 units and b = 4 units:

$$

Area
=


3
×
4

2

=

12
2

=
6
square units

The perimeter of a triangle is the sum of the lengths of its three sides. It is one of the most fundamental properties of a triangle and can be calculated easily if the side lengths are known.

General Formula:

The formula for the perimeter \(P\) of a triangle with side lengths \(a\), \(b\), and \(c\) is:

\[
P = a + b + c
\]

Step-by-Step Calculation:

1. Measure the lengths of all three sides of the triangle. Let's denote these lengths as \(a\), \(b\), and \(c\).
2. Apply the perimeter formula:

   
   \[
   P = a + b + c
   \]

3. Add the lengths of the sides together to find the perimeter.

Examples:

• Example 1: For a triangle with side lengths 7 cm, 10 cm, and 5 cm:

  
  \[
  P = 7 \, \text{cm} + 10 \, \text{cm} + 5 \, \text{cm} = 22 \, \text{cm}
  \]

• Example 2: For a triangle with side lengths 3.5 m, 4.2 m, and 5.8 m:

  
  \[
  P = 3.5 \, \text{m} + 4.2 \, \text{m} + 5.8 \, \text{m} = 13.5 \, \text{m}
  \]

• Example 3: For a triangle with side lengths 12 in, 15 in, and 18 in:

  
  \[
  P = 12 \, \text{in} + 15 \, \text{in} + 18 \, \text{in} = 45 \, \text{in}
  \]

Special Cases:

In some specific types of triangles, the formula can be applied directly with simplified forms:

• Equilateral Triangle: All three sides are equal. If the side length is \(a\), then:

  
  \[
  P = 3a
  \]

• Isosceles Triangle: Two sides are equal. If the equal sides are \(a\) and the base is \(b\), then:

  
  \[
  P = 2a + b
  \]

• Scalene Triangle: All three sides are different, and the general formula applies:

  
  \[
  P = a + b + c
  \]

Calculating the perimeter of a triangle involves summing the lengths of its three sides. Here are some detailed examples to illustrate the process:

Example 1: Scalene Triangle

Consider a scalene triangle with side lengths 5 cm, 7 cm, and 9 cm. The perimeter \(P\) is calculated as follows:

1. Identify the lengths of the sides: \(a = 5 \, \text{cm}\), \(b = 7 \, \text{cm}\), \(c = 9 \, \text{cm}\).
2. Apply the formula:

   
   \[
   P = a + b + c
   \]

3. Substitute the side lengths into the formula:

   
   \[
   P = 5 \, \text{cm} + 7 \, \text{cm} + 9 \, \text{cm} = 21 \, \text{cm}
   \]

Example 2: Equilateral Triangle

Consider an equilateral triangle with each side measuring 6 inches. The perimeter \(P\) is calculated as follows:

1. Identify the length of one side: \(a = 6 \, \text{in}\).
2. Since all sides are equal, apply the formula for an equilateral triangle:

   
   \[
   P = 3a
   \]

3. Substitute the side length into the formula:

   
   \[
   P = 3 \times 6 \, \text{in} = 18 \, \text{in}
   \]

Example 3: Isosceles Triangle

Consider an isosceles triangle with two equal sides of 8 meters each and a base of 5 meters. The perimeter \(P\) is calculated as follows:

1. Identify the lengths of the sides: \(a = 8 \, \text{m}\), \(b = 8 \, \text{m}\), \(c = 5 \, \text{m}\).
2. Apply the general perimeter formula:

   
   \[
   P = a + b + c
   \]

3. Substitute the side lengths into the formula:

   
   \[
   P = 8 \, \text{m} + 8 \, \text{m} + 5 \, \text{m} = 21 \, \text{m}
   \]

Example 4: Right Triangle

Consider a right triangle with side lengths 3 cm, 4 cm, and 5 cm. The perimeter \(P\) is calculated as follows:

1. Identify the lengths of the sides: \(a = 3 \, \text{cm}\), \(b = 4 \, \text{cm}\), \(c = 5 \, \text{cm}\).
2. Apply the perimeter formula:

   
   \[
   P = a + b + c
   \]

3. Substitute the side lengths into the formula:

   
   \[
   P = 3 \, \text{cm} + 4 \, \text{cm} + 5 \, \text{cm} = 12 \, \text{cm}
   \]

These examples illustrate how to calculate the perimeter for different types of triangles by simply adding the lengths of their sides.

One of the most common methods to calculate the area of a triangle is by using its base and height. This method is straightforward and applicable to a wide range of triangles.

The formula to find the area of a triangle using its base (\(b\)) and height (\(h\)) is:

$$\text{Area} = \frac{1}{2} \times \text{base} \times \text{height}$$

Here, the base refers to the length of the side of the triangle on which it stands, and the height is the perpendicular distance from the base to the opposite vertex.

To apply this formula, simply measure the length of the base and the corresponding perpendicular height, and plug the values into the formula. Then, multiply the base by the height and divide the result by 2 to obtain the area of the triangle.

This method is particularly useful when the triangle is right-angled, as the height can be easily determined by drawing a perpendicular line from the vertex opposite the base to the base itself.

Heron's formula provides an elegant method to calculate the area of a triangle when the lengths of all three sides are known. This formula is particularly useful in situations where the base and height of the triangle are not easily determinable.

The formula for the area of a triangle using Heron's formula is:

$$\text{Area} = \sqrt{s(s - a)(s - b)(s - c)}$$

where \(a\), \(b\), and \(c\) are the lengths of the triangle's sides, and \(s\) is the semiperimeter of the triangle given by \(s = \frac{a + b + c}{2}\).

To apply Heron's formula, first, calculate the semiperimeter (\(s\)) using the formula mentioned above. Then, substitute the values of \(a\), \(b\), \(c\), and \(s\) into the main formula. Finally, compute the square root of the resulting expression to obtain the area of the triangle.

While Heron's formula may involve more computation compared to other methods, it offers a versatile approach for finding the area of triangles with known side lengths, making it a valuable tool in geometry and practical applications.

Trigonometry provides another method to find the area of a triangle, especially when the measures of one angle and two side lengths are known. This approach is particularly useful when the triangle's base and height are not readily available.

The formula to calculate the area of a triangle using trigonometry is:

$$\text{Area} = \frac{1}{2} \times \text{side} \times \text{side} \times \sin(\text{angle})$$

Here, the side lengths (\(a\) and \(b\)) refer to the known sides of the triangle, and the angle (\(\theta\)) is the measure of the included angle between these sides.

To apply this formula, first, identify the known side lengths and the included angle. Then, use trigonometric functions to find the sine of the angle. Finally, multiply the product of the two side lengths and the sine of the angle by \( \frac{1}{2} \) to obtain the area of the triangle.

This method offers an alternative approach to finding the area of a triangle and can be particularly useful in situations where other methods are not applicable or convenient.

Here are some examples illustrating how to calculate the area of triangles using different methods:

1. Using Base and Height:

   Consider a triangle with a base of length \(6\) units and a height of \(4\) units. Using the formula \( \text{Area} = \frac{1}{2} \times \text{base} \times \text{height} \),

   $$\text{Area} = \frac{1}{2} \times 6 \times 4 = 12 \text{ square units}$$

2. Using Heron's Formula:

   Suppose we have a triangle with side lengths \(a = 5\), \(b = 7\), and \(c = 8\) units. First, calculate the semiperimeter \(s\):

   $$s = \frac{5 + 7 + 8}{2} = 10$$

   Then, apply Heron's formula:

   $$\text{Area} = \sqrt{10(10 - 5)(10 - 7)(10 - 8)} = \sqrt{10 \times 5 \times 3 \times 2} = \sqrt{300} \approx 17.32 \text{ square units}$$

3. Using Trigonometry:

   Consider a triangle with side lengths \(a = 9\) units, \(b = 12\) units, and an angle between them of \(60^\circ\). Use the formula \( \text{Area} = \frac{1}{2} \times \text{side} \times \text{side} \times \sin(\text{angle}) \),

   $$\text{Area} = \frac{1}{2} \times 9 \times 12 \times \sin(60^\circ) = \frac{1}{2} \times 9 \times 12 \times \frac{\sqrt{3}}{2} = 54 \times \frac{\sqrt{3}}{2} \approx 27.71 \text{ square units}$$

These examples demonstrate the versatility of different methods in calculating the area of triangles, offering flexibility based on the available information about the triangle.

The concepts of triangle area and perimeter have numerous real-world applications across various fields. Here are some examples:

1. Architecture and Construction:

   Architects and civil engineers use triangle area and perimeter calculations extensively in designing buildings, bridges, and other structures. These calculations help determine the amount of materials needed and ensure structural stability.

2. Land Surveying:

   Surveyors use triangle area calculations to measure and map land accurately. By dividing the land into triangles, they can calculate its total area and perimeter, which is crucial for property assessment and development planning.

3. Geometry and Mathematics:

   Triangle area and perimeter concepts are fundamental in geometry and mathematics education. Students learn about geometric principles and apply them to solve various problems, developing critical thinking and problem-solving skills.

4. Navigation and Cartography:

   In navigation and cartography, understanding triangle properties helps in determining distances and angles between locations on maps. This information is vital for navigation, route planning, and creating accurate maps.

5. Art and Design:

   Artists and designers often use geometric shapes, including triangles, in their creations. Understanding triangle properties allows them to create aesthetically pleasing compositions and structures in various art forms, from paintings to sculptures.

These applications highlight the importance of triangle area and perimeter calculations in diverse fields, showcasing their relevance and versatility in practical contexts.

Test your understanding of triangle area and perimeter with the following practice problems:

1. Problem 1:

   Calculate the area of a triangle with a base of \(8\) units and a height of \(6\) units.

2. Problem 2:

   Find the area of a triangle with side lengths \(5\), \(12\), and \(13\) units using Heron's formula.

3. Problem 3:

   Determine the area of a triangle with side lengths \(9\), \(10\), and \(12\) units and an included angle of \(60^\circ\), using trigonometry.

4. Problem 4:

   Given a triangle with perimeter \(30\) units and side lengths in the ratio \(3:4:5\), calculate its area.

5. Problem 5:

   A triangle has perimeter \(24\) units, and two of its sides measure \(6\) units and \(8\) units, respectively. Determine the area of the triangle.

These practice problems will help reinforce your understanding of triangle area and perimeter concepts, preparing you for various mathematical challenges.