Topic find the dimensions of a rectangle with perimeter: Discover how to find the dimensions of a rectangle with perimeter in this detailed guide. Learn the essential formulas, explore practical examples, and practice solving problems. Whether you're a student or simply curious, this article will provide you with the knowledge and tools to master this fundamental geometry concept.
Table of Content
- Finding the Dimensions of a Rectangle with a Given Perimeter
- Introduction to Rectangles
- Understanding Perimeter
- Basic Formulas and Definitions
- Calculating Dimensions from Perimeter
- Examples and Practice Problems
- Using Diagonals to Find Dimensions
- Special Cases and Advanced Problems
- Applications in Real Life
- Interactive Tools and Calculators
- Frequently Asked Questions
- YOUTUBE:
Finding the Dimensions of a Rectangle with a Given Perimeter
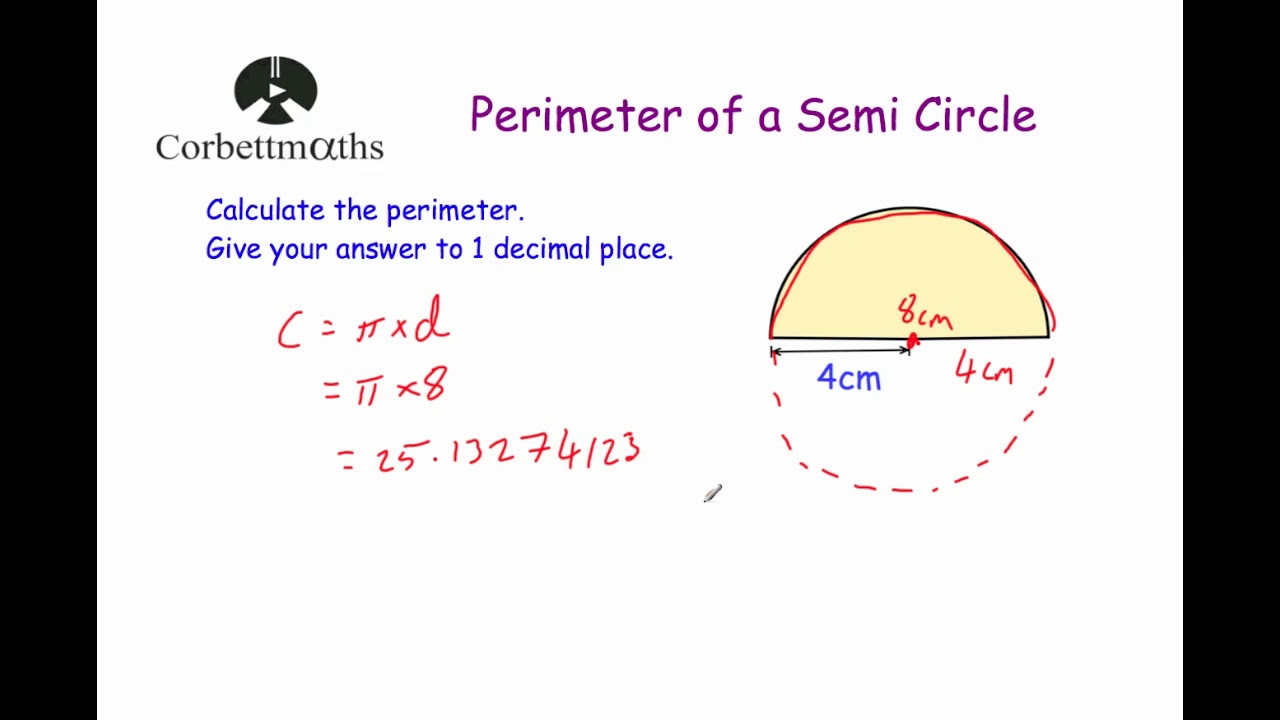
To find the dimensions of a rectangle when you know its perimeter, you can use the following steps and formulas:
Perimeter Formula
The perimeter of a rectangle is given by:
\[ P = 2(L + W) \]
where \( P \) is the perimeter, \( L \) is the length, and \( W \) is the width of the rectangle.
Finding the Length or Width
Given the perimeter and one of the dimensions (either length or width), you can find the other dimension using these formulas:
- Length: \[ L = \frac{P}{2} - W \]
- Width: \[ W = \frac{P}{2} - L \]
Example Calculation
Suppose the perimeter of a rectangle is 50 units and the width is 10 units. To find the length:
- Use the formula for length: \[ L = \frac{P}{2} - W \]
- Substitute the known values: \[ L = \frac{50}{2} - 10 \]
- Simplify the equation: \[ L = 25 - 10 = 15 \]
Therefore, the length of the rectangle is 15 units.
Applications and Examples
Here are some real-world applications and further examples:
- Calculating the fencing required for a rectangular garden (byjus.com).
- Determining the border length for an art project (splashlearn.com).
- Finding dimensions for construction plans such as a swimming pool (measureby.com).
Working with Diagonals
If you know the diagonal and one side of a rectangle, you can use the Pythagorean theorem to find the other side:
\[ D = \sqrt{L^2 + W^2} \]
where \( D \) is the diagonal. Rearranging to find the length:
\[ L = \sqrt{D^2 - W^2} \]
For example, if the diagonal is 13 units and the width is 5 units:
- Substitute the known values: \[ L = \sqrt{13^2 - 5^2} \]
- Calculate: \[ L = \sqrt{169 - 25} = \sqrt{144} = 12 \]
Thus, the length is 12 units.
Additional Resources
For more interactive learning and practice problems, you can explore:
- ChiliMath for practice problems and examples.
- Omni Calculator for various rectangle-related calculators.

READ MORE:
Introduction to Rectangles
A rectangle is a four-sided polygon with opposite sides that are equal in length and all angles are right angles (90 degrees). It is one of the most fundamental shapes in geometry, commonly found in everyday life, from books and screens to rooms and buildings.
The key properties of a rectangle include:
- Opposite Sides Equal: The opposite sides of a rectangle are of equal length.
- Right Angles: All four angles of a rectangle are 90 degrees.
- Diagonals: The diagonals of a rectangle are equal in length and bisect each other.
Understanding these properties helps in calculating the dimensions of a rectangle when certain measurements are known, such as the perimeter.
For instance, the perimeter \(P\) of a rectangle can be expressed as:
\[ P = 2(l + w) \]
where \(l\) is the length and \(w\) is the width of the rectangle. By rearranging this formula, you can find either the length or the width if the perimeter and the other dimension are known.
Let's dive deeper into the concept of perimeter in the next section.
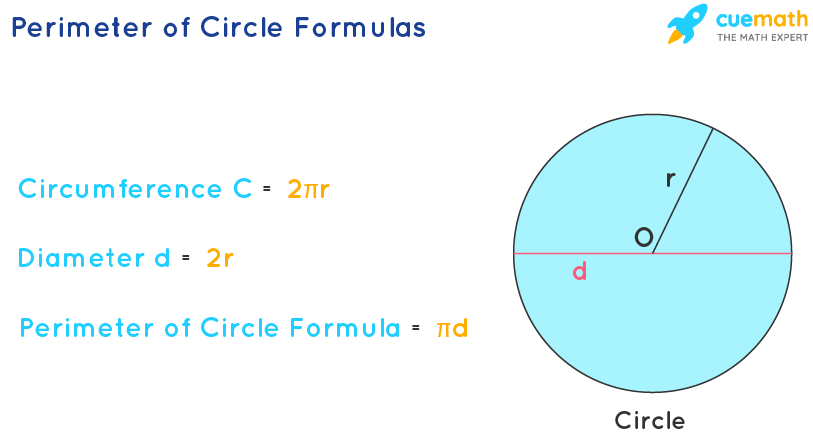
Understanding Perimeter
The perimeter of a rectangle is the total distance around the outside of the rectangle. It is a crucial measurement in geometry and is used in various practical applications, such as determining the amount of material needed to frame a picture or fence a yard.
To calculate the perimeter of a rectangle, you need to know the lengths of its sides. The formula for the perimeter \(P\) is:
\[ P = 2(l + w) \]
where \(l\) represents the length and \(w\) represents the width of the rectangle. This formula adds the lengths of all four sides, but since the opposite sides of a rectangle are equal, it simplifies to twice the sum of the length and the width.
Here is a step-by-step method to understand the calculation of the perimeter:
- Measure the Length: Measure the longer side of the rectangle. This is your length \(l\).
- Measure the Width: Measure the shorter side of the rectangle. This is your width \(w\).
- Sum the Dimensions: Add the length and the width together: \(l + w\).
- Double the Sum: Multiply the sum by 2 to get the perimeter: \(2(l + w)\).
For example, if a rectangle has a length of 8 units and a width of 5 units, the perimeter is calculated as follows:
\[ P = 2(8 + 5) = 2 \times 13 = 26 \text{ units} \]
Understanding the perimeter is fundamental to finding the dimensions of a rectangle when the perimeter is known. This forms the basis for more complex calculations and applications, which we will explore in the subsequent sections.
Basic Formulas and Definitions
To effectively find the dimensions of a rectangle using its perimeter, it's important to understand some basic formulas and definitions related to rectangles.
Definitions:
- Length (\(l\)): The longer side of the rectangle.
- Width (\(w\)): The shorter side of the rectangle.
- Perimeter (\(P\)): The total distance around the rectangle.
- Area (\(A\)): The amount of space inside the rectangle, calculated as \(A = l \times w\).
Basic Formulas:
- Perimeter Formula: The perimeter \(P\) of a rectangle can be calculated using the formula: \[ P = 2(l + w) \]
- Solving for Length or Width: If the perimeter and one dimension (length or width) are known, you can rearrange the perimeter formula to solve for the unknown dimension. \[ l = \frac{P}{2} - w \] \[ w = \frac{P}{2} - l \]
- Area Formula: The area \(A\) of a rectangle can be calculated using the formula: \[ A = l \times w \]
Let's go through a step-by-step example to illustrate these formulas:
- Given: A rectangle with a perimeter of 30 units and a width of 7 units.
- Find the Length: Use the perimeter formula and solve for \(l\). \[ P = 2(l + w) \] Substitute the given values: \[ 30 = 2(l + 7) \] Simplify and solve for \(l\): \[ 30 = 2l + 14 \] \[ 16 = 2l \] \[ l = 8 \]
With these basic formulas and definitions, you can now calculate various properties of a rectangle, including finding the dimensions when given the perimeter. These foundational concepts will aid in solving more complex problems and applications discussed in later sections.
Calculating Dimensions from Perimeter
To find the dimensions of a rectangle when given its perimeter, you can follow these steps:
- Understand the formula for the perimeter of a rectangle, which is P = 2(l + w), where P is the perimeter, l is the length, and w is the width.
- Identify the given perimeter of the rectangle.
- Use the perimeter formula to set up an equation involving the length and width of the rectangle.
- Solve the equation for one variable in terms of the other. For example, if you solve for length, you'll have an equation in terms of width, or vice versa.
- Substitute the value of the solved variable into either the length or width component of the perimeter formula.
- Calculate the dimensions of the rectangle using the substituted value and the known value of the other dimension.
For example, if the perimeter of a rectangle is 24 units and one of its dimensions is 6 units, you can follow these steps:
- Given the perimeter formula, P = 2(l + w), and the perimeter as 24 units.
- Substitute 24 for P.
- Solve the equation for one variable. Let's solve for length (l): 24 = 2(l + 6).
- Divide both sides by 2 to isolate (l + 6): 12 = l + 6.
- Subtract 6 from both sides to find the length: l = 6 units.
- Substitute l = 6 into the perimeter formula: 24 = 2(6 + w).
- Solve for width (w): 24 = 12 + 2w → 12 = 2w → w = 6 units.
So, the dimensions of the rectangle are length = 6 units and width = 6 units.

Examples and Practice Problems
In this section, we will go through examples and practice problems to help you understand how to find the dimensions of a rectangle given its perimeter.
Example 1
Given a rectangle with a perimeter of 24 units, find its possible dimensions.
- Start with the formula for the perimeter of a rectangle:
\[ P = 2(l + w) \]
- Substitute the given perimeter into the formula:
\[ 24 = 2(l + w) \]
- Simplify the equation to solve for \( l + w \):
\[ l + w = 12 \]
- Now, consider possible integer pairs \((l, w)\) that add up to 12:
- \( l = 1 \) and \( w = 11 \)
- \( l = 2 \) and \( w = 10 \)
- \( l = 3 \) and \( w = 9 \)
- \( l = 4 \) and \( w = 8 \)
- \( l = 5 \) and \( w = 7 \)
- \( l = 6 \) and \( w = 6 \)
- Verify each pair by calculating the perimeter:
- For \( l = 1 \) and \( w = 11 \):
\[ P = 2(1 + 11) = 24 \]
- For \( l = 2 \) and \( w = 10 \):
\[ P = 2(2 + 10) = 24 \]
- For \( l = 3 \) and \( w = 9 \):
\[ P = 2(3 + 9) = 24 \]
- For \( l = 4 \) and \( w = 8 \):
\[ P = 2(4 + 8) = 24 \]
- For \( l = 5 \) and \( w = 7 \):
\[ P = 2(5 + 7) = 24 \]
- For \( l = 6 \) and \( w = 6 \):
\[ P = 2(6 + 6) = 24 \]
- For \( l = 1 \) and \( w = 11 \):
Practice Problem 1
A rectangle has a perimeter of 30 units. Find its possible dimensions.
Example 2
Given a rectangle with a perimeter of 40 units, and the length is 5 units more than the width, find the dimensions of the rectangle.
- Start with the formula for the perimeter:
\[ P = 2(l + w) \]
- Substitute the given perimeter into the formula:
\[ 40 = 2(l + w) \]
- Simplify the equation to solve for \( l + w \):
\[ l + w = 20 \]
- Since the length is 5 units more than the width, substitute \( l = w + 5 \) into the equation:
\[ w + 5 + w = 20 \]
- Solve for \( w \):
\[ 2w + 5 = 20 \]
\[ 2w = 15 \]
\[ w = 7.5 \]
- Find \( l \):
\[ l = w + 5 = 7.5 + 5 = 12.5 \]
- Verify by calculating the perimeter:
\[ P = 2(12.5 + 7.5) = 40 \]
Practice Problem 2
A rectangle has a perimeter of 50 units, and the length is twice the width. Find its dimensions.
Using Diagonals to Find Dimensions
To find the dimensions of a rectangle using its diagonal, you can use the Pythagorean theorem. The diagonal of a rectangle divides it into two congruent right triangles. If the length of the rectangle is \( l \) and the width is \( w \), then the diagonal \( d \) can be found using the formula:
\[ d = \sqrt{l^2 + w^2} \]
Given the perimeter \( P \) and the diagonal \( d \), follow these steps to find the dimensions:
- Express the width \( w \) in terms of the length \( l \) and perimeter \( P \):
\[ w = \frac{P}{2} - l \]
- Substitute this expression into the diagonal formula:
\[ d = \sqrt{l^2 + \left( \frac{P}{2} - l \right)^2} \]
- Simplify and solve for \( l \):
\[ d^2 = l^2 + \left( \frac{P}{2} - l \right)^2 \]
\[ d^2 = l^2 + \left( \frac{P^2}{4} - P l + l^2 \right) \]
\[ d^2 = 2l^2 - P l + \frac{P^2}{4} \]
Rearrange into a quadratic equation:
\[ 2l^2 - P l + \left( \frac{P^2}{4} - d^2 \right) = 0 \]
Solve this quadratic equation for \( l \) using the quadratic formula:
\[ l = \frac{P \pm \sqrt{P^2 - 8d^2}}{4} \]
- Find \( w \) using the perimeter expression:
\[ w = \frac{P}{2} - l \]
Example:
Suppose the perimeter \( P \) is 30 units and the diagonal \( d \) is 13 units. To find the length \( l \) and width \( w \) of the rectangle, follow these steps:
- Calculate \( w \) in terms of \( l \):
\[ w = \frac{30}{2} - l = 15 - l \]
- Substitute into the diagonal formula and simplify:
\[ 13^2 = l^2 + (15 - l)^2 \]
\[ 169 = l^2 + 225 - 30l + l^2 \]
\[ 2l^2 - 30l + 56 = 0 \]
Solving this quadratic equation:
\[ l = \frac{30 \pm \sqrt{30^2 - 4 \cdot 2 \cdot 56}}{2 \cdot 2} \]
\[ l = \frac{30 \pm \sqrt{900 - 448}}{4} \]
\[ l = \frac{30 \pm \sqrt{452}}{4} \]
\[ l = \frac{30 \pm 21.26}{4} \]
\[ l \approx 12.82 \text{ or } 3.18 \]
- Find \( w \) for each \( l \):
If \( l = 12.82 \), then \( w = 15 - 12.82 \approx 2.18 \)
If \( l = 3.18 \), then \( w = 15 - 3.18 \approx 11.82 \)
Thus, the dimensions of the rectangle could be approximately 12.82 units by 2.18 units or 3.18 units by 11.82 units.
Special Cases and Advanced Problems
In this section, we will explore special cases and advanced problems related to finding the dimensions of a rectangle. These cases often involve additional constraints or require more advanced mathematical techniques. Let's dive in!
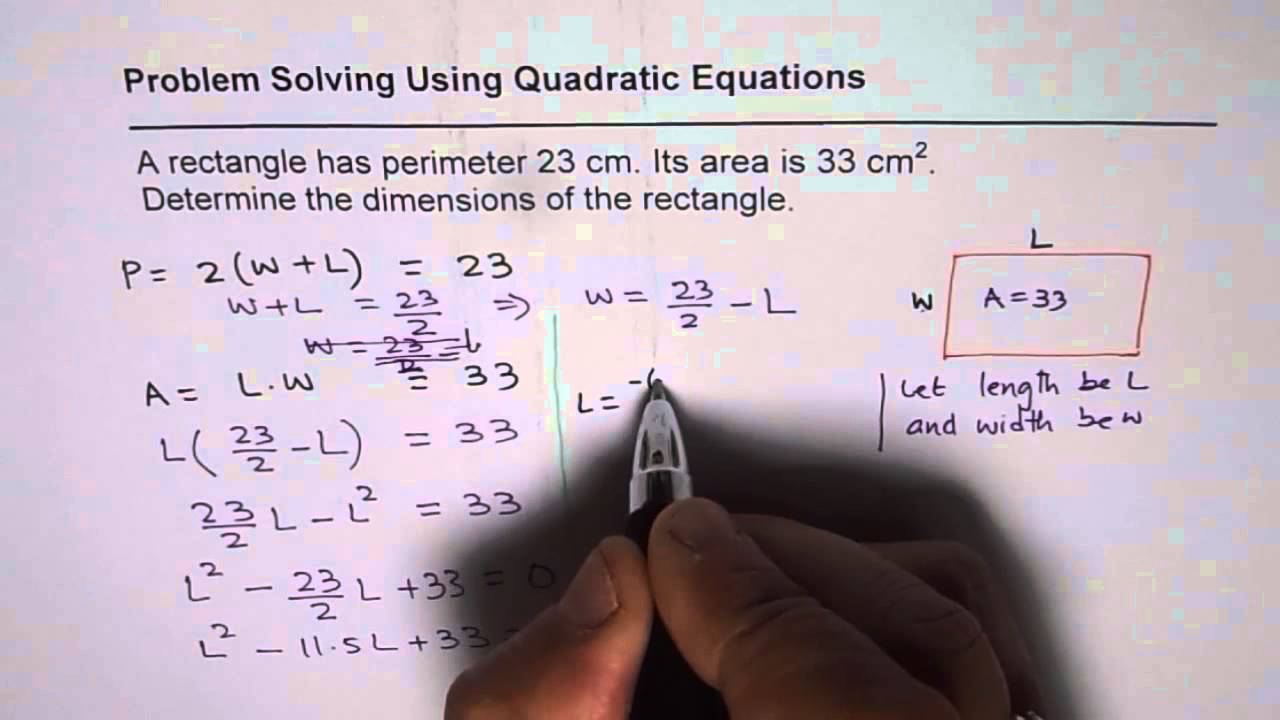
Case 1: Rectangle with a Given Perimeter and Area
Sometimes, you might be given both the perimeter and the area of a rectangle and need to find its dimensions.
- Given: Perimeter (P) and Area (A)
- Formulas:
- \( P = 2L + 2W \)
- \( A = L \times W \)
- Steps:
- Express one dimension in terms of the other using the perimeter formula: \[ L = \frac{P}{2} - W \]
- Substitute this expression into the area formula and solve the quadratic equation: \[ A = \left( \frac{P}{2} - W \right) \times W \] \[ A = \frac{P}{2} W - W^2 \] Rearrange and solve for W using the quadratic formula.
Example
Find the dimensions of a rectangle with a perimeter of 30 units and an area of 50 square units.
- Given:
- Perimeter (P) = 30 units
- Area (A) = 50 square units
- Using the perimeter formula: \[ L = \frac{30}{2} - W = 15 - W \]
- Substitute into the area formula: \[ 50 = (15 - W) \times W \] \[ 50 = 15W - W^2 \] \[ W^2 - 15W + 50 = 0 \]
- Solve the quadratic equation: \[ W = \frac{15 \pm \sqrt{15^2 - 4 \times 1 \times 50}}{2 \times 1} \] \[ W = \frac{15 \pm \sqrt{225 - 200}}{2} \] \[ W = \frac{15 \pm 5}{2} \] \[ W = 10 \text{ or } W = 5 \]
- Dimensions:
- If W = 10, then L = 15 - 10 = 5
- If W = 5, then L = 15 - 5 = 10
Case 2: Rectangle with Length Related to Width
Another common special case involves a relationship between the length and the width.
- Example: The length is twice the width plus 3 units.
- Formulas:
- \( L = 2W + 3 \)
- \( P = 2L + 2W \)
- Steps:
- Substitute the expression for L into the perimeter formula: \[ P = 2(2W + 3) + 2W \] \[ P = 4W + 6 + 2W \] \[ P = 6W + 6 \]
- Solve for W: \[ W = \frac{P - 6}{6} \]
- Use the relationship to find L: \[ L = 2W + 3 \]
Example
Find the dimensions of a rectangle with a perimeter of 42 units and where the length is twice the width plus 3 units.
- Given:
- Perimeter (P) = 42 units
- Relationship: \( L = 2W + 3 \)
- Using the perimeter formula: \[ 42 = 6W + 6 \] \[ 36 = 6W \] \[ W = 6 \]
- Finding L: \[ L = 2 \times 6 + 3 = 12 + 3 = 15 \]
- Dimensions:
- Width (W) = 6 units
- Length (L) = 15 units
Advanced Problem: Minimizing Perimeter for a Given Area
Sometimes, you need to find the dimensions of a rectangle that minimize the perimeter for a given area. This often involves calculus.
- Given: Area (A)
- Objective: Minimize \( P = 2L + 2W \) subject to \( A = L \times W \)
- Steps:
- Express one dimension in terms of the other using the area formula: \[ W = \frac{A}{L} \]
- Substitute into the perimeter formula: \[ P = 2L + 2\left(\frac{A}{L}\right) \] \[ P = 2L + \frac{2A}{L} \]
- Find the derivative of P with respect to L and set it to zero to find critical points: \[ \frac{dP}{dL} = 2 - \frac{2A}{L^2} = 0 \] \[ 2 = \frac{2A}{L^2} \] \[ L^2 = A \] \[ L = \sqrt{A} \]
- Using the relationship \( W = \frac{A}{L} \): \[ W = \frac{A}{\sqrt{A}} = \sqrt{A} \]
- Conclusion: The dimensions that minimize the perimeter for a given area are \( L = W = \sqrt{A} \), which makes the rectangle a square.
Applications in Real Life
Understanding the dimensions and perimeter of a rectangle has numerous practical applications in everyday life. Here are some detailed examples:
-
Building and Construction:
In construction, knowing the perimeter of a structure is essential for determining the amount of materials needed. For example, when building a wooden shed, the perimeter helps in calculating the total length of the lumber required for the walls.
-
Gardening:
When planning to enclose a garden with a fence, the perimeter measurement helps to determine how much fencing material is needed. For instance, if a garden is 20 feet long and 30 feet wide, the perimeter is calculated as:
\[ P = 2L + 2W = 2(20) + 2(30) = 100 \text{ feet} \]
-
Interior Design:
In interior design, the perimeter of a room is used to calculate the length of molding required for doors, windows, and baseboards. For a rectangular room measuring 15 feet by 20 feet, the perimeter is:
\[ P = 2L + 2W = 2(15) + 2(20) = 70 \text{ feet} \]
-
Picture Framing:
When creating custom picture frames, knowing the perimeter of the frame is crucial for cutting the correct length of framing material. For a picture measuring 8 inches by 10 inches, the perimeter is:
\[ P = 2L + 2W = 2(8) + 2(10) = 36 \text{ inches} \]
-
Surveying and Land Measurement:
In surveying, calculating the perimeter of a plot of land is essential for defining property boundaries. This is especially important for rectangular plots, where the perimeter provides a clear boundary line.
-
Sports Fields:
For designing sports fields, such as soccer or baseball fields, the perimeter is used to lay out the field dimensions and ensure proper boundary lines. For a rectangular soccer field measuring 100 meters by 70 meters, the perimeter is:
\[ P = 2L + 2W = 2(100) + 2(70) = 340 \text{ meters} \]
These examples highlight the importance of understanding how to calculate the dimensions and perimeter of a rectangle, demonstrating its wide range of applications in various fields and everyday scenarios.
Interactive Tools and Calculators
Finding the dimensions of a rectangle given its perimeter can be simplified using interactive tools and calculators available online. These tools allow you to input the perimeter and receive the possible dimensions instantly. Here are some steps and details about how to use these tools effectively:
- Visit an Online Calculator: There are several websites offering interactive calculators specifically for rectangles. You can start by visiting a trusted website.
- Input the Perimeter: Most calculators will have a field where you can enter the perimeter of the rectangle. Ensure you have this value ready.
- Choose Additional Parameters (if any): Some advanced calculators might ask for additional information such as the aspect ratio or one dimension to narrow down the possible results.
- Calculate: Click the calculate button to get the dimensions of the rectangle. The tool will use the perimeter formula \( P = 2(l + w) \) to compute the possible lengths and widths.
Here are some useful interactive tools and calculators:
These tools not only calculate the dimensions but often provide additional features such as:
- Graphical representation of the rectangle with labeled dimensions
- Step-by-step solutions explaining how the dimensions were calculated
- Options to switch between different units of measurement (e.g., inches, centimeters)
Using these interactive tools can save time and enhance understanding, especially when working with complex problems or verifying manual calculations.
| Calculator | Features | Link |
|---|---|---|
| Calculator Soup | Basic and advanced calculations, unit conversions | |
| Omni Calculator | Interactive graph, step-by-step solutions | |
| Math Is Fun | Simple interface, educational explanations |
Frequently Asked Questions
Here are some frequently asked questions about finding the dimensions of a rectangle with a given perimeter:
- What is the formula for the perimeter of a rectangle?
- How do I find the dimensions of a rectangle if I know the perimeter?
- Can I find the dimensions of a rectangle if I only know the perimeter?
- What if I know the area and the perimeter of the rectangle?
- How can I use a diagonal to find the dimensions of a rectangle?
- What is the golden rectangle and how does it relate to dimensions?
The formula for the perimeter \( P \) of a rectangle is:
\[
P = 2 \times (L + W)
\]
where \( L \) is the length and \( W \) is the width of the rectangle.
To find the dimensions of a rectangle when you know the perimeter \( P \), you need to have either the length or the width. The formulas are:
If the width \( W \) is known:
\[
L = \frac{P}{2} - W
\]
If the length \( L \) is known:
\[
W = \frac{P}{2} - L
\]
No, you need to know either the length or the width in addition to the perimeter to find the exact dimensions of the rectangle. Knowing only the perimeter does not provide enough information to determine both dimensions.
If you know both the area \( A \) and the perimeter \( P \) of the rectangle, you can use these values to find the dimensions. The formulas are:
1. Express the width in terms of the perimeter and length:
\[
W = \frac{P}{2} - L
\]
2. Substitute this into the area formula:
\[
A = L \times \left( \frac{P}{2} - L \right)
\]
3. Solve the resulting quadratic equation to find \( L \). Then, use \( W = \frac{P}{2} - L \) to find \( W \).
If you know the diagonal \( D \) and either the length or the width, you can use the Pythagorean theorem:
\[
D^2 = L^2 + W^2
\]
to find the unknown dimension.
For example, if you know the width \( W \) and the diagonal \( D \):
\[
L = \sqrt{D^2 - W^2}
\]
The golden rectangle is a special type of rectangle where the ratio of the length to the width is the golden ratio, approximately 1.618. This ratio is aesthetically pleasing and appears in various aspects of art and nature.
Tìm kích thước của một hình chữ nhật có chu vi 100 m và diện tích lớn nhất, hướng dẫn Calculus 1
READ MORE:
Tìm kích thước hình chữ nhật với chu vi và diện tích cho trước