What is the Square Root of 325: Comprehensive Guide to Calculation and Understanding

The square root of a number is a value that, when multiplied by itself, gives the original number. In this case, we are looking for the square root of 325.

The square root of 325 can be expressed as:

$$\sqrt{325}$$

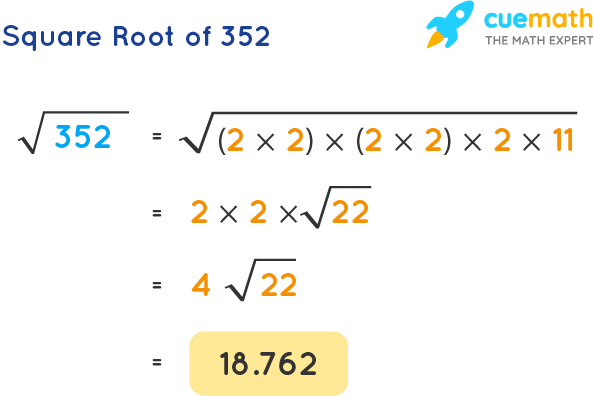
To simplify, we can factorize 325:

• 325 = 5^2 \times 13

Thus, the square root of 325 can be written as:

$$\sqrt{325} = \sqrt{5^2 \times 13}$$

By separating the factors inside the square root, we get:

$$\sqrt{325} = \sqrt{5^2} \times \sqrt{13}$$

Since the square root of \(5^2\) is 5, we have:

$$\sqrt{325} = 5 \sqrt{13}$$

Decimal Approximation

The decimal approximation of the square root of 325 is:

$$\sqrt{325} \approx 18.027756377319946$$

This value is approximate and can be used for practical calculations.

Conclusion

The exact form of the square root of 325 is \(5 \sqrt{13}\), and its decimal approximation is approximately 18.03.

The concept of a square root is fundamental in mathematics, representing a value that, when multiplied by itself, results in the original number. It is denoted by the radical symbol (√). For example, the square root of 9 is 3, since \(3 \times 3 = 9\).

Square roots can be categorized into two types:

• Perfect Squares: Numbers that have an integer as their square root. For example, 16 is a perfect square because its square root is 4 (\(4 \times 4 = 16\)).
• Non-Perfect Squares: Numbers that do not have an integer as their square root. For instance, 20 is not a perfect square, and its square root is an irrational number.

Calculating square roots can be done using several methods:

1. Prime Factorization: Breaking down the number into its prime factors to simplify the square root.
2. Long Division Method: A manual process similar to traditional division, which helps find square roots more accurately.
3. Using a Calculator: Modern calculators provide quick and precise square root calculations, which is particularly useful for non-perfect squares.

Understanding square roots is essential not only for academic purposes but also for solving real-world problems in engineering, physics, and finance. For example, they are used in calculating areas, solving quadratic equations, and analyzing financial models.

The square root of a number is a value that, when multiplied by itself, yields the original number. Mathematically, if \( x \) is the square root of \( y \), then \( x^2 = y \). This relationship is expressed using the radical symbol \( \sqrt{} \).

For example:

• The square root of 16 is 4, because \( 4 \times 4 = 16 \). This can be written as \( \sqrt{16} = 4 \).
• The square root of 25 is 5, because \( 5 \times 5 = 25 \). This is written as \( \sqrt{25} = 5 \).

Square roots can be classified into two main types:

1. Perfect Square Roots: These are square roots of perfect squares, where the result is an integer. For example, \( \sqrt{36} = 6 \), since 36 is a perfect square.
2. Non-Perfect Square Roots: These are square roots of numbers that are not perfect squares, resulting in irrational numbers. For instance, \( \sqrt{20} \approx 4.472 \), where 20 is not a perfect square and its square root is an irrational number.

In the case of 325, it is not a perfect square. Therefore, its square root is an irrational number. The exact value of the square root of 325 can be expressed as \( \sqrt{325} \). To simplify, 325 can be factored into prime factors:

325 = \( 5^2 \times 13 \)

Thus, the square root of 325 can be simplified to:

$$ \sqrt{325} = \sqrt{5^2 \times 13} = 5 \sqrt{13} $$

This simplifies the calculation and provides a clearer understanding of the square root of 325.

The square root of 325 is an interesting mathematical concept, as 325 is not a perfect square. This means that its square root is an irrational number, which cannot be expressed as a simple fraction and has a non-repeating, non-terminating decimal expansion.

To find the square root of 325, we start by using prime factorization:

• First, factorize 325 into its prime factors: \( 325 = 5^2 \times 13 \).

This allows us to simplify the square root expression:

$$ \sqrt{325} = \sqrt{5^2 \times 13} $$

We can separate the factors inside the square root:

$$ \sqrt{325} = \sqrt{5^2} \times \sqrt{13} $$

Since the square root of \( 5^2 \) is 5, we have:

$$ \sqrt{325} = 5 \sqrt{13} $$

Thus, the simplified form of the square root of 325 is \( 5 \sqrt{13} \).

To find the decimal approximation, we can use a calculator:

$$ \sqrt{13} \approx 3.605551275463989 $$

Multiplying by 5, we get:

$$ 5 \sqrt{13} \approx 5 \times 3.605551275463989 = 18.027756377319946 $$

Therefore, the decimal approximation of the square root of 325 is approximately 18.03.

This detailed understanding helps in various applications, from solving equations to understanding geometric properties. Recognizing the structure and steps involved in calculating the square root enhances comprehension and practical problem-solving skills.

Calculating the square root of 325 can be approached through various methods, each with its own advantages. Below are the most commonly used methods:

1. Prime Factorization Method:
• First, factorize 325 into its prime factors: \( 325 = 5^2 \times 13 \).
• Express the square root using these factors:

  $$ \sqrt{325} = \sqrt{5^2 \times 13} $$

• Simplify by separating the square root of the perfect square:

  $$ \sqrt{325} = \sqrt{5^2} \times \sqrt{13} $$

• Calculate the square root of \( 5^2 \) which is 5:

  $$ \sqrt{325} = 5 \sqrt{13} $$

• The simplified form is \( 5 \sqrt{13} \), which is the exact representation.
2. Long Division Method:
• Estimate a number that, when squared, is close to 325. In this case, 18 is a good starting point.
• Divide 325 by 18 to get an approximation: \( 325 \div 18 \approx 18.055 \).
• Take the average of 18 and 18.055: \( \frac{18 + 18.055}{2} = 18.0275 \).
• Repeat the process until the desired precision is achieved. This iterative method converges to the square root.
3. Using a Calculator:
• Modern calculators can quickly provide the square root of any number. Simply input 325 and use the square root function.
• The calculator gives an approximate value:

  $$ \sqrt{325} \approx 18.027756377319946 $$

Each method has its own use cases. The prime factorization method gives an exact form, the long division method provides a manual approach to understanding the process, and the calculator method is the quickest for practical purposes.

The prime factorization method is an effective way to simplify the calculation of the square root of a number by breaking it down into its prime factors. Here's a detailed step-by-step process for calculating the square root of 325 using this method:

1. Find the Prime Factors of 325:
• Start by dividing 325 by the smallest prime number, which is 5:

  $$ 325 \div 5 = 65 $$

• Next, factor 65 by dividing by the smallest prime number, which is again 5:

  $$ 65 \div 5 = 13 $$

• Finally, 13 is a prime number, so we have the prime factors of 325:

  $$ 325 = 5^2 \times 13 $$

2. Express the Square Root Using Prime Factors:
• Write the square root of 325 using its prime factorization:

  $$ \sqrt{325} = \sqrt{5^2 \times 13} $$

3. Simplify the Square Root:
• Separate the square root of the product into the product of the square roots:

  $$ \sqrt{325} = \sqrt{5^2} \times \sqrt{13} $$

• Calculate the square root of \( 5^2 \), which is 5:

  $$ \sqrt{5^2} = 5 $$

• Combine the results to simplify the expression:

  $$ \sqrt{325} = 5 \sqrt{13} $$

4. Approximate the Square Root of 13:
• To get a decimal approximation, use a calculator to find:

  $$ \sqrt{13} \approx 3.605551275463989 $$

• Multiply this approximation by 5 to get the decimal value:

  $$ 5 \times 3.605551275463989 \approx 18.027756377319946 $$

Therefore, the exact value of the square root of 325 is \( 5 \sqrt{13} \), and the decimal approximation is approximately 18.03. This method provides a clear and systematic approach to understanding and calculating the square root of non-perfect squares.

Finding the decimal approximation of the square root of 325 involves calculating a value that, when multiplied by itself, is as close as possible to 325. Here is a step-by-step process to find the decimal approximation:

1. Initial Estimate:
• Identify two perfect squares between which 325 lies. For instance, \( 18^2 = 324 \) and \( 19^2 = 361 \). Therefore, the square root of 325 lies between 18 and 19.
2. Refinement Using Division:
• Take an initial guess, say 18.1, and divide 325 by this guess:

  $$ \frac{325}{18.1} \approx 17.96 $$

• Average the initial guess with the result of the division:

  $$ \frac{18.1 + 17.96}{2} \approx 18.03 $$

3. Further Refinement:
• Take the new guess, 18.03, and repeat the process:

  $$ \frac{325}{18.03} \approx 18.03 $$

• The values are converging, indicating that the decimal approximation is accurate.
4. Using a Calculator for Precision:
• To achieve higher precision, use a scientific calculator to find:

  $$ \sqrt{325} \approx 18.027756377319946 $$

The decimal approximation of the square root of 325 is approximately 18.03. This method, known as iterative approximation, ensures a precise value for practical purposes.

For quick calculations, especially in practical applications, the use of a calculator is recommended for obtaining the most accurate result.

Calculating the square root of 325 step-by-step involves a systematic approach using both the prime factorization method and the long division method. Below is a detailed guide:

1. Prime Factorization Method:
• Start by breaking down 325 into its prime factors:

  $$ 325 = 5^2 \times 13 $$

• Write the square root expression using these factors:

  $$ \sqrt{325} = \sqrt{5^2 \times 13} $$

• Simplify the square root by separating the factors:

  $$ \sqrt{325} = \sqrt{5^2} \times \sqrt{13} $$

• Calculate the square root of \( 5^2 \):

  $$ \sqrt{5^2} = 5 $$

• Combine the results to get the simplified form:

  $$ \sqrt{325} = 5 \sqrt{13} $$

• For a decimal approximation, find \( \sqrt{13} \approx 3.605551275463989 \):

  $$ 5 \times 3.605551275463989 \approx 18.027756377319946 $$

2. Long Division Method:
• Estimate the initial number whose square is close to 325. We can start with 18.
• Divide 325 by the estimate:

  $$ \frac{325}{18} \approx 18.0556 $$

• Averaging the estimate and the result:

  $$ \frac{18 + 18.0556}{2} \approx 18.0278 $$

• Refine the process by repeating the steps with the new estimate:

  $$ \frac{325}{18.0278} \approx 18.0278 $$

• Continue this iterative process until the values converge. In this case, they converge quickly to approximately 18.0278.
3. Using a Calculator:
• For a quick and precise result, use a scientific calculator to find the square root of 325:

  $$ \sqrt{325} \approx 18.027756377319946 $$

By following these step-by-step methods, you can accurately determine the square root of 325, both in its simplified form and as a decimal approximation. This approach not only provides the exact value but also enhances understanding of the calculation process.

Calculating the square root of 325 using a calculator is a straightforward process. Follow these steps to find the result accurately:

1. Turn on your calculator and make sure it is in the standard calculation mode.
2. Locate the square root function on your calculator. This is usually represented by the symbol √ or may be accessed by pressing a secondary function key followed by the square root key.
3. Enter the number 325. This can be done by pressing the digits 3, 2, and 5 in sequence.
4. Press the square root button. This will instruct the calculator to compute the square root of the entered number.
5. Read the displayed result. The calculator should display an approximate value for the square root of 325.

For most calculators, the square root of 325 will be approximately:

\[
\sqrt{325} \approx 18.027756377319946
\]

Note that different calculators may display the result with varying degrees of precision. Ensure that your calculator is set to show an appropriate number of decimal places based on your need.

If your calculator includes a history or memory function, you can store this value for later reference or use it in further calculations without having to re-enter it.

Using these simple steps, you can quickly and accurately find the square root of 325 with a calculator.

Simplifying square roots involves expressing the square root in its simplest form. Here’s a detailed guide on how to simplify the square root of 325:

1. First, perform the prime factorization of 325. To do this, break down 325 into its prime factors:
   • 325 is divisible by 5: \(325 \div 5 = 65\)
   • 65 is divisible by 5: \(65 \div 5 = 13\)
   • 13 is a prime number and cannot be divided further.

   So, the prime factorization of 325 is:

   
   \[
   325 = 5^2 \times 13
   \]

2. Next, apply the property of square roots that allows you to separate the factors:

   
   \[
   \sqrt{325} = \sqrt{5^2 \times 13}
   \]

3. Since the square root of a squared number is the number itself, simplify:

   
   \[
   \sqrt{325} = \sqrt{5^2} \times \sqrt{13} = 5 \times \sqrt{13}
   \]

4. Thus, the simplified form of the square root of 325 is:

   
   \[
   \sqrt{325} = 5\sqrt{13}
   \]

By following these steps, you can simplify the square root of 325 to \(5\sqrt{13}\), which is its simplest form. This method can be applied to simplify the square roots of other numbers as well by finding their prime factors and simplifying accordingly.

Square roots have a wide range of applications in various fields of real life. Understanding these applications can help illustrate the importance of square roots in everyday activities and professional practices. Here are some key examples:

• Engineering and Construction:

  In engineering and construction, square roots are used to determine dimensions and measurements. For example, when calculating the length of the diagonal of a square or rectangle, the Pythagorean theorem is applied, which involves square roots. If a construction project requires determining the diagonal of a square plot with sides of 325 units, the calculation would involve the square root of 325.

• Physics and Science:

  Square roots are used in physics to solve equations related to motion, energy, and forces. For instance, in the equation for kinetic energy \(E_k = \frac{1}{2}mv^2\), where \(v\) is velocity, solving for velocity when energy and mass are known involves taking the square root. Similarly, many formulas in science require square root operations for accurate results.

• Finance:

  In finance, square roots are used to calculate the standard deviation and variance of investment returns, which are measures of risk. The formula for standard deviation involves taking the square root of the variance, helping investors understand the volatility and stability of their investments.

• Computer Graphics:

  Square roots play a significant role in computer graphics and animation. For example, when calculating the distance between two points in 2D or 3D space, the distance formula \(\sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2}\) involves square roots. This is essential for rendering images, modeling, and creating realistic animations.

• Medicine:

  In medicine, square roots are used in various calculations, such as determining dosages and interpreting medical test results. For example, the Body Mass Index (BMI) calculation involves a square root operation when adjusting for height and weight ratios.

These examples highlight just a few of the many ways square roots are applied in real life. Their use is essential for precise calculations and understanding complex relationships in numerous fields.

There are several common misconceptions about square roots that can lead to confusion. Understanding and correcting these misconceptions is crucial for a proper grasp of mathematical concepts. Here are some of the most frequent misunderstandings:

• Square Roots and Squaring are the Same:

  One common misconception is that taking the square root of a number is the same as squaring it. In reality, squaring a number means multiplying it by itself (e.g., \(5^2 = 25\)), while the square root of a number is the value that, when multiplied by itself, gives the original number (e.g., \(\sqrt{25} = 5\)).

• Negative Numbers Have No Square Roots:

  Another misconception is that negative numbers cannot have square roots. While it's true that there are no real square roots for negative numbers, they do have imaginary square roots. For instance, the square root of -1 is denoted as \(i\), where \(i\) is the imaginary unit.

• Only Perfect Squares Have Square Roots:

  Some people believe that only perfect squares (numbers like 1, 4, 9, 16, etc.) have square roots. In fact, every positive number has a square root, even if it is not a whole number. For example, \(\sqrt{325}\) is approximately 18.03, which is not an integer.

• Square Root of a Sum Equals Sum of Square Roots:

  A frequent error is assuming that the square root of a sum is the same as the sum of the square roots. This is incorrect: \(\sqrt{a + b} \neq \sqrt{a} + \sqrt{b}\). For instance, \(\sqrt{9 + 16} \neq \sqrt{9} + \sqrt{16}\) because \(\sqrt{25} = 5\), while \(\sqrt{9} + \sqrt{16} = 3 + 4 = 7\).

• Simplifying Square Roots Always Gives Whole Numbers:

  Another misconception is that simplifying square roots always results in whole numbers. While some square roots simplify to whole numbers, many do not. For example, the square root of 325 simplifies to \(5\sqrt{13}\), which is not a whole number.

By recognizing and addressing these common misconceptions, one can develop a clearer and more accurate understanding of square roots and their properties.

Here are some frequently asked questions (FAQs) about the square root of 325, along with detailed answers to help clarify common queries:

• What is the square root of 325?

  The square root of 325 is approximately 18.03. More precisely, it is \(\sqrt{325} \approx 18.027756377319946\).

• Is the square root of 325 a rational number?

  No, the square root of 325 is not a rational number. A rational number can be expressed as a fraction of two integers, but the square root of 325 is an irrational number because it cannot be expressed as an exact fraction.

• Can the square root of 325 be simplified?

  Yes, the square root of 325 can be simplified. The prime factorization of 325 is \(5^2 \times 13\), so:

  
  \[
  \sqrt{325} = \sqrt{5^2 \times 13} = 5\sqrt{13}
  \]

• How can I calculate the square root of 325 by hand?

  You can calculate the square root of 325 by hand using the long division method or by estimating and refining your estimate. For precise methods, using a calculator is recommended.

• What are the applications of knowing the square root of 325?

  The square root of 325 can be useful in various fields such as engineering, physics, and construction where precise measurements are required. It can also be helpful in solving mathematical problems involving quadratic equations and geometric calculations.

• Why is the square root of 325 important?

  Understanding the square root of 325 is important for solving problems in algebra, geometry, and real-life applications. It also helps in developing a deeper comprehension of the properties of numbers and their relationships.

• Is the square root of 325 used in the Pythagorean theorem?

  Yes, the square root of 325 can be used in the Pythagorean theorem to determine the length of a side of a right triangle when the other sides' lengths are known. For example, if one leg of a right triangle is 325 units, the hypotenuse can be calculated using \(\sqrt{325 + b^2}\).

These FAQs provide a comprehensive understanding of the square root of 325 and its significance in various contexts.