What is the Square Root of 180? Uncover the Mystery Behind This Number!

The square root of a number is a value that, when multiplied by itself, gives the original number. The square root of 180 can be expressed in various ways.

Exact Form

The exact form of the square root of 180 is:

\(\sqrt{180}\)

Simplified Radical Form

The square root of 180 can be simplified. Here are the steps to simplify it:

1. Factor 180 into its prime factors: \(180 = 2^2 \times 3^2 \times 5\)
2. Pair the prime factors: \(2^2\) and \(3^2\) can be taken out of the square root as single numbers.
3. Simplify the expression: \(\sqrt{180} = \sqrt{2^2 \times 3^2 \times 5} = 2 \times 3 \times \sqrt{5} = 6\sqrt{5}\)

Therefore, the simplified radical form of \(\sqrt{180}\) is:

\(6\sqrt{5}\)

Decimal Form

The decimal form of the square root of 180, rounded to three decimal places, is approximately:

\(\sqrt{180} \approx 13.416\)

Properties

• The square root of 180 is an irrational number.
• It cannot be expressed as a simple fraction.
• The decimal representation is non-terminating and non-repeating.

Calculation Methods

There are various methods to calculate the square root of 180, such as:

• Prime factorization method (as shown above).
• Using a calculator.
• Estimation and approximation techniques.

The concept of square roots is fundamental in mathematics. A square root of a number is a value that, when multiplied by itself, yields the original number. This concept is crucial for solving quadratic equations, understanding geometric properties, and various applications in science and engineering.

For example, the square root of 9 is 3 because \(3 \times 3 = 9\). Mathematically, this is represented as:

\(\sqrt{9} = 3\)

Square roots can be categorized into two types:

• Perfect Squares: Numbers whose square roots are whole numbers (e.g., \(\sqrt{16} = 4\)).
• Non-Perfect Squares: Numbers whose square roots are not whole numbers (e.g., \(\sqrt{20}\)).

In the case of non-perfect squares, the square roots can often be simplified into a radical form. For example, \(\sqrt{20}\) can be simplified to \(2\sqrt{5}\). Here's the step-by-step process:

1. Factor the number into its prime factors: \(20 = 2^2 \times 5\).
2. Pair the prime factors: \(2^2\) can be taken out of the square root as a single number.
3. Simplify the expression: \(\sqrt{20} = \sqrt{2^2 \times 5} = 2\sqrt{5}\).

The square root symbol (√) is used to denote the square root of a number. For instance, \(\sqrt{x}\) represents the square root of \(x\).

Square roots also have interesting properties:

• Non-negative: Square roots of non-negative numbers are always non-negative. For example, \(\sqrt{25} = 5\), not -5, even though \((-5) \times (-5) = 25\).
• Irrational Numbers: The square root of a non-perfect square is an irrational number, meaning it cannot be expressed as a simple fraction.

Understanding square roots is essential for advancing in various areas of mathematics, including algebra, geometry, and calculus. It also plays a significant role in real-world applications such as physics, engineering, and computer science.

The square root of a number is a value that, when multiplied by itself, gives the original number. In mathematical terms, if x is the square root of y, then x² = y. The square root is denoted by the radical symbol √. For example, the square root of 9 is 3, because 3 multiplied by itself equals 9.

Square roots can be classified into two main categories:

• Perfect Square Roots: These are square roots of perfect squares, which are numbers that can be expressed as the product of an integer with itself. For example, 16 is a perfect square because it is 4².
• Non-Perfect Square Roots: These are square roots of numbers that are not perfect squares. These roots are usually irrational numbers, which cannot be expressed as a simple fraction. An example is the square root of 2, which is approximately 1.414.

The process of finding a square root is the inverse operation of squaring a number. For example, if 5² = 25, then the square root of 25 is 5.

In symbolic form, the square root of a number a is written as √a or a^1/2. When dealing with non-negative real numbers, the principal square root is always taken as the positive root. For example, √25 = 5.

Square roots play a crucial role in various mathematical operations and are widely used in algebra, geometry, and calculus. They are fundamental in solving quadratic equations, optimizing functions, and analyzing geometrical shapes.

The square root of 180 is a number which, when multiplied by itself, equals 180. Mathematically, this can be expressed as:

\[\sqrt{180} = x \quad \text{such that} \quad x^2 = 180\]

Since 180 is not a perfect square, its square root is an irrational number. This means it cannot be expressed as a simple fraction and its decimal representation is non-terminating and non-repeating. The approximate value of the square root of 180 is 13.416:

\[\sqrt{180} \approx 13.416\]

To understand the square root of 180 better, let's break it down using different methods:

Simplified Radical Form

To simplify \(\sqrt{180}\), we can use the prime factorization method:

• Prime factorize 180: \(180 = 2^2 \times 3^2 \times 5\)
• Group the prime factors into pairs of squares: \(\sqrt{180} = \sqrt{2^2 \times 3^2 \times 5}\)
• Extract the square roots of the pairs: \(\sqrt{2^2 \times 3^2 \times 5} = 2 \times 3 \times \sqrt{5} = 6\sqrt{5}\)

Thus, the simplified radical form of the square root of 180 is:

\[\sqrt{180} = 6\sqrt{5}\]

Decimal Form

Using a calculator or the long division method, we find that the decimal form of \(\sqrt{180}\) is approximately 13.416. This is obtained through iterative approximation techniques or using advanced calculators.

Prime Factorization Method

Prime factorization involves breaking down 180 into its prime factors:

• Start with the smallest prime number, 2: \(180 \div 2 = 90\)
• Divide 90 by 2: \(90 \div 2 = 45\)
• Divide 45 by 3: \(45 \div 3 = 15\)
• Divide 15 by 3: \(15 \div 3 = 5\)
• Divide 5 by 5: \(5 \div 5 = 1\)

The prime factors of 180 are \(2 \times 2 \times 3 \times 3 \times 5\), and grouping the factors gives us:

\[\sqrt{180} = \sqrt{2^2 \times 3^2 \times 5} = 6\sqrt{5}\]

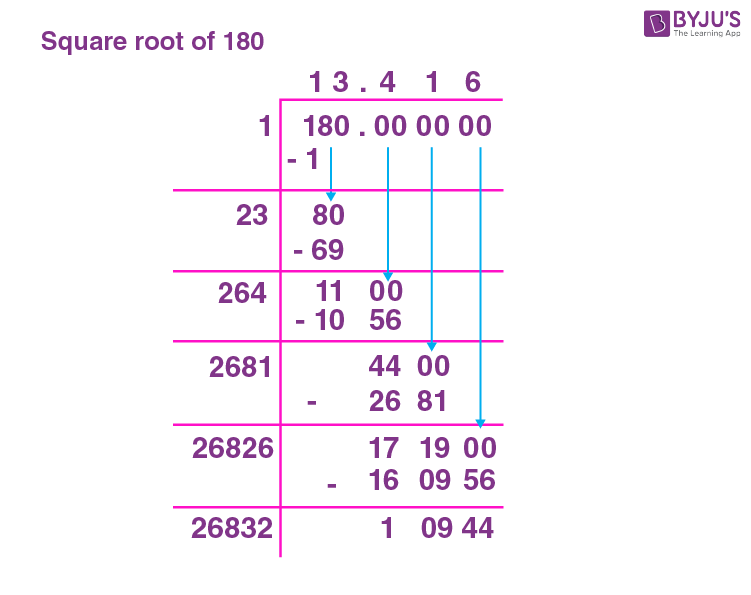
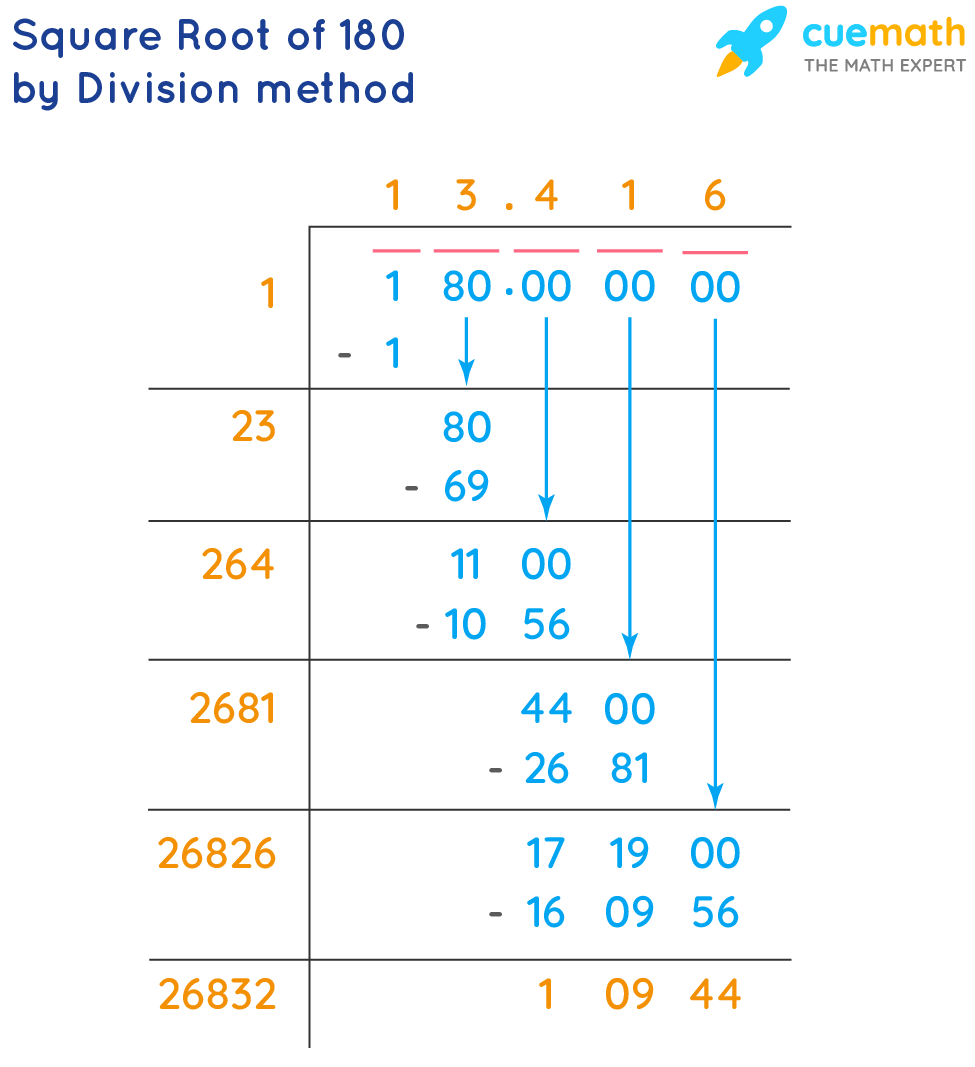
Long Division Method

The long division method provides a way to find the square root of a number to a high degree of accuracy. For 180:

1. Pair the digits from right to left: 1 and 80.
2. Find the largest number whose square is less than or equal to 1 (which is 1). Subtract 1 from 1 to get a remainder of 0.
3. Bring down the next pair (80), making the new number 80. Double the quotient obtained (1) to get 2. Determine how many times 2 fits into 80. Continue this process to find the square root.
4. Continue the process to obtain a more accurate value: \(\sqrt{180} \approx 13.416\).

Repeated Subtraction Method

Another method to understand square roots is by repeated subtraction of odd numbers. This method involves subtracting consecutive odd numbers from 180 until the result is zero or negative:

• 180 - 1 = 179
• 179 - 3 = 176
• 176 - 5 = 171
• ...continue this process until the result is less than or equal to zero.

If the subtraction never results in zero, the number is not a perfect square, reinforcing that \(\sqrt{180}\) is irrational.

Understanding these methods helps to see why \(\sqrt{180}\) is approximately 13.416 and confirms its irrational nature.

The exact form of the square root of 180 can be expressed in its simplified radical form. To find this, we need to factorize 180 and identify the perfect square factors.

First, let's find the prime factorization of 180:

• 180 ÷ 2 = 90
• 90 ÷ 2 = 45
• 45 ÷ 3 = 15
• 15 ÷ 3 = 5
• 5 ÷ 5 = 1

Thus, the prime factorization of 180 is:

180 = 2 × 2 × 3 × 3 × 5

We can group the prime factors into pairs of perfect squares:

180 = (2 × 2) × (3 × 3) × 5 = 2² × 3² × 5

Taking the square root of both sides, we get:

√180 = √(2² × 3² × 5)

Using the property of square roots that √(a × b) = √a × √b, we can simplify this to:

√180 = √(2²) × √(3²) × √5

Simplifying further, we get:

√180 = 2 × 3 × √5

Therefore, the exact form of the square root of 180 in simplified radical form is:

6√5

Simplifying the square root of 180 involves breaking it down into its prime factors and then simplifying the radical. Here are the detailed steps to simplify the square root of 180:

1. Find the prime factors of 180:

   Start by dividing 180 by the smallest prime number, which is 2, and continue dividing the quotient by prime numbers until you reach 1.

   180 ÷ 2 = 90

   90 ÷ 2 = 45

   45 ÷ 3 = 15

   15 ÷ 3 = 5

   5 ÷ 5 = 1

   So, the prime factors of 180 are \( 2^2 \times 3^2 \times 5 \).

2. Group the prime factors:

   Group the prime factors into pairs. Each pair will come out of the radical sign as a single number.

   \( 180 = (2^2) \times (3^2) \times 5 \)

3. Simplify the radical:

   Take the square root of each pair of prime factors and multiply them together outside the radical.

   \( \sqrt{180} = \sqrt{(2^2) \times (3^2) \times 5} \)

   \( \sqrt{180} = 2 \times 3 \times \sqrt{5} \)

   \( \sqrt{180} = 6\sqrt{5} \)

Therefore, the simplified radical form of the square root of 180 is \( 6\sqrt{5} \).

To simplify the square root of 180 using the prime factorization method, we follow these steps:

1. Find the prime factors of 180. Start by dividing 180 by the smallest prime number, which is 2.
• 180 ÷ 2 = 90
• 90 ÷ 2 = 45
• 45 ÷ 3 = 15
• 15 ÷ 3 = 5
• 5 ÷ 5 = 1
2. Write the prime factors of 180 as a product of prime numbers:

   \[180 = 2 \times 2 \times 3 \times 3 \times 5\]

3. Group the prime factors into pairs:

   \[180 = (2 \times 2) \times (3 \times 3) \times 5\]

4. Simplify the expression by taking the square root of each pair of prime factors:

   \[\sqrt{180} = \sqrt{(2 \times 2) \times (3 \times 3) \times 5} = 2 \times 3 \times \sqrt{5} = 6\sqrt{5}\]

Therefore, the simplified radical form of the square root of 180 is \(6\sqrt{5}\).

The square root of 180 can be expressed in its decimal form for a precise representation. This form is particularly useful when an exact value is needed in calculations or for a more understandable approximation in everyday contexts.

The square root of 180 in decimal form is approximately:

\[ \sqrt{180} \approx 13.416407865 \]

Here is a step-by-step method to understand how we arrive at this decimal value:

1. First, recognize that the square root function will give a number which, when multiplied by itself, results in 180.
2. Use a calculator or long division method to find the approximate value. The long division method is a traditional approach that can yield this result through manual calculations.
3. With modern tools, using a scientific calculator or an online calculator provides a quick and accurate decimal representation.

The decimal form is rounded to ten decimal places, giving us:

\[ \sqrt{180} \approx 13.416407865 \]

This representation shows that while the square root of 180 is an irrational number (it cannot be expressed as a simple fraction), we can still approximate it to a desired degree of accuracy for practical purposes.

To approximate the square root of 180, we can follow these steps:

1. Estimation: Begin by estimating the square root of numbers close to 180. For instance, √169 = 13 and √196 = 14, so √180 is likely slightly more than 13 and less than 14.
2. Linear Interpolation: To refine the estimate, use linear interpolation. Given that 180 is closer to 169 than 196, we can interpolate between √169 = 13 and √196 = 14. Calculate:

   √180 ≈ 13 + (180 - 169)/(196 - 169) * (14 - 13)

   √180 ≈ 13 + 11/27 ≈ 13.407

3. Newton's Method: For a more precise approximation, Newton's method can be employed. Start with a guess (e.g., 13.4) and iterate using the formula:

   x_n+1 = x_n - f(x_n) / f'(x_n)

   Where f(x) = x² - 180 and f'(x) = 2x.

These techniques provide increasingly accurate approximations of the square root of 180, catering to different levels of precision required in mathematical and real-world applications.

The square root of 180, denoted as √180, possesses the following properties:

1. Nature: √180 is an irrational number, meaning it cannot be expressed as a simple fraction.
2. Numerical Value: Approximated, √180 ≈ 13.4164.
3. Exact Form: In its exact radical form, √180 = √(36 * 5) = 6√5.
4. Factorization: The factorization of 180 influences its square root's properties, often used in mathematical derivations.
5. Decimal Representation: The decimal representation of √180 continues infinitely without repeating, affirming its irrational nature.
6. Applications: Used in geometry, physics, and engineering for calculations involving area, volume, and dynamics.

The square root of 180, denoted as √180, is an irrational number with the following characteristics:

• Non-Repeating Decimal: Its decimal representation continues indefinitely without any pattern.
• Non-Terminating: √180 cannot be expressed as a fraction of two integers.
• Proof of Irrationality: It has been proven through mathematical methods that √180 is irrational.
• Real-World Significance: The irrational nature of √180 makes it essential in various mathematical and scientific applications.
• Continued Use: Despite its irrationality, √180 is frequently used in calculations requiring precise measurements and in theoretical mathematics.

The decimal representation of the square root of 180, denoted as √180, is approximately 13.416407864998739...

This decimal continues indefinitely without repeating, confirming that √180 is an irrational number.

To find the square root of 180 using a calculator:

1. Turn on your calculator and ensure it is in standard mode.
2. Enter the number 180.
3. Locate the square root function (√) on your calculator.
4. Press the square root button to obtain the result.
5. The result displayed on the calculator screen is approximately 13.416407864998739...

Estimating and approximating the square root of 180 involves the following steps:

1. Initial Estimation: Begin with known square roots of nearby perfect squares. For instance, √169 = 13 and √196 = 14, suggesting √180 is slightly greater than 13 and less than 14.
2. Linear Interpolation: To refine the estimate, use linear interpolation between known values. Since 180 is closer to 169 than 196, interpolate between √169 = 13 and √196 = 14 to get a closer approximation.
3. Newton's Method: Use an iterative approach such as Newton's method to further refine the approximation. Start with an initial guess and iterate using the formula x_n+1 = x_n - f(x_n) / f'(x_n), where f(x) = x^2 - 180.
4. Calculator Approximation: For practical purposes, use a calculator to find √180 directly, providing a precise decimal approximation.

The square root of 180, denoted as √180, finds applications in various fields:

• Geometry: Used in calculating lengths of diagonals and sides in geometric shapes involving areas of 180 units.
• Physics: Utilized in mechanics and dynamics for determining forces, velocities, and accelerations in scenarios where the value 180 plays a role.
• Engineering: Essential in structural analysis and design, particularly in determining stress distributions and material requirements.
• Mathematics: Applied in mathematical modeling and computations involving areas, volumes, and complex equations where √180 appears as a fundamental component.
• Real-World Scenarios: Finds use in practical applications such as construction, architecture, and manufacturing where precise measurements and calculations are necessary.

In the realm of mathematics, the square root of 180, denoted as √180, plays various roles:

• Algebra: Used in solving quadratic equations involving √180 as part of the discriminant.
• Number Theory: Studied for its properties as an irrational number and its relationships with other mathematical constants.
• Geometry: Applied in geometric calculations where the value 180 is involved, such as in the areas and diagonals of specific shapes.
• Calculations: Utilized in practical mathematical computations requiring precise values for √180, both exact and approximate.
• Curriculum: Taught as part of mathematical education to illustrate concepts of irrational numbers, square roots, and their applications.

The square root of 180, √180, finds practical applications in everyday life:

• Measurement: Used in construction and carpentry to determine lengths and dimensions of materials.
• Finance: Applied in financial calculations for estimating returns on investments and loan repayments.
• Statistics: Utilized in statistical analysis to compute standard deviations and variability in data sets.
• Technology: Employed in computer science and engineering for programming algorithms and simulations.
• Healthcare: Utilized in medical imaging for calculating sizes and proportions of organs and tissues.

The square root of 180 can be expressed in its simplest radical form as \( 6 \sqrt{5} \). This is derived from the prime factorization of 180, which is \( 2^2 \times 3^2 \times 5 \). When simplified, \( \sqrt{180} = \sqrt{36 \times 5} = \sqrt{36} \times \sqrt{5} = 6 \sqrt{5} \).