180 Square Root Simplified: Easy Step-by-Step Guide

According to mathematical calculations and methods, the simplified square root of 180 is represented as follows: $$\sqrt{180} = 6 \sqrt{5}$$

Explanation:

To simplify the square root of 180, we first find its prime factors. 180 can be expressed as the product of 36 and 5. Since 36 is a perfect square, its square root is 6. Therefore, the simplified form of $$\sqrt{180}$$ is 6 times the square root of 5.

Verification:

Calculating further, $$6 \sqrt{5}$$ squared yields: $$(6 \sqrt{5})^2 = 36 \times 5 = 180$$ Thus, confirming that $$\sqrt{180} = 6 \sqrt{5}$$ is correct.

Conclusion:

Therefore, the simplified square root of 180 is $$\sqrt{180} = 6 \sqrt{5}$$

Square roots are a fundamental concept in mathematics, representing a value that, when multiplied by itself, gives the original number. For instance, the square root of 9 is 3, because \(3 \times 3 = 9\). Simplifying square roots involves finding the most reduced form of a square root expression.

Here are some key points to understand square roots better:

• The square root symbol is \(\sqrt{ }\).
• Every positive number has two square roots: one positive (principal) and one negative. For example, the square roots of 16 are 4 and -4.
• The square root of 0 is 0.
• Square roots of negative numbers are not real numbers; they are represented as imaginary numbers.

Let's break down the process of simplifying square roots with an example:

1. Find the prime factorization of the number under the square root.
2. Group the prime factors into pairs.
3. Move each pair of prime factors out of the square root.
4. Multiply the factors outside the square root together.
5. The result is the simplified form of the square root.

To illustrate, we will simplify the square root of 180:

Prime factorization of 180: \(180 = 2 \times 2 \times 3 \times 3 \times 5\).

Group the pairs: \(180 = (2 \times 2) \times (3 \times 3) \times 5\).

Move pairs outside the square root: \(\sqrt{180} = 2 \times 3 \times \sqrt{5}\).

Simplified form: \(\sqrt{180} = 6\sqrt{5}\).

Understanding these steps will help you simplify square roots efficiently and accurately. This guide will further explore the methods and applications of simplifying square roots, ensuring you gain a solid grasp of this essential mathematical concept.

Simplifying square roots involves expressing the square root in its simplest form. This process helps in reducing complex square root expressions, making them easier to work with. To simplify a square root, we need to factorize the number under the square root sign and extract the square factors.

Here's a detailed step-by-step approach to simplify square roots:

1. Find the Prime Factorization: Break down the number under the square root into its prime factors. For example, the prime factorization of 180 is \(180 = 2^2 \times 3^2 \times 5\).
2. Identify and Group Pairs of Factors: Group the prime factors into pairs. Each pair represents a square number. In the case of 180, we have pairs \(2^2\) and \(3^2\), and a single 5 left unpaired.
3. Extract the Pairs: Move each pair of factors out of the square root, placing them outside as a single factor. Each extracted pair simplifies to a single factor outside the radical. For \(2^2\) and \(3^2\), this results in \(2 \times 3\).
4. Simplify the Expression: Multiply the factors extracted from the pairs and place any remaining factors inside the square root. For 180, this means \(\sqrt{180} = 2 \times 3 \times \sqrt{5} = 6\sqrt{5}\).

The simplified form of \(\sqrt{180}\) is \(6\sqrt{5}\). Here’s a general example to illustrate the process:

• Consider \(\sqrt{72}\).
• Prime factorization: \(72 = 2^3 \times 3^2\).
• Group pairs: \((2^2) \times 2 \times (3^2)\).
• Extract pairs: \(2 \times 3 \times \sqrt{2}\).
• Simplified form: \(\sqrt{72} = 6\sqrt{2}\).

By following these steps, you can simplify any square root expression efficiently. This not only aids in solving mathematical problems but also enhances your understanding of number properties and factorization.

Simplifying square roots can be achieved through a few basic methods. These methods involve breaking down the number under the square root into its prime factors and then simplifying by extracting pairs of factors. Here are the most common methods:

1. Prime Factorization Method:
   1. Find the prime factorization of the number under the square root.
   2. Identify pairs of prime factors.
   3. Extract each pair of prime factors outside the square root as a single factor.
   4. Multiply the factors outside the square root together.
   5. Place any remaining factors inside the square root.

   Example:

   • Simplify \(\sqrt{180}\)
   • Prime factorization: \(180 = 2^2 \times 3^2 \times 5\)
   • Pairs: \(2^2\) and \(3^2\)
   • Extract pairs: \(2 \times 3 \times \sqrt{5}\)
   • Simplified form: \(\sqrt{180} = 6\sqrt{5}\)
2. Factor Tree Method:
   1. Create a factor tree to break down the number into its prime factors.
   2. Continue splitting each composite number until only prime numbers are left.
   3. Group the prime factors into pairs.
   4. Extract the pairs outside the square root.
   5. Multiply the factors outside the square root and leave any unpaired factors inside the square root.

   Example:

   • Simplify \(\sqrt{72}\)
   • Factor tree: \(72 \rightarrow 8 \times 9 \rightarrow 2^3 \times 3^2\)
   • Pairs: \(2^2\) and \(3^2\)
   • Extract pairs: \(2 \times 3 \times \sqrt{2}\)
   • Simplified form: \(\sqrt{72} = 6\sqrt{2}\)
3. Division Method:
   1. Divide the number under the square root by the smallest prime number (starting with 2).
   2. Continue dividing by the smallest prime until the result is a prime number.
   3. Group pairs of the same prime number.
   4. Extract the pairs outside the square root.
   5. Multiply the factors outside the square root together, leaving any remaining factors inside the square root.

   Example:

   • Simplify \(\sqrt{50}\)
   • Division: \(50 \div 2 = 25 \div 5 = 5\)
   • Pairs: \(2\) and \(5^2\)
   • Extract pairs: \(5 \times \sqrt{2}\)
   • Simplified form: \(\sqrt{50} = 5\sqrt{2}\)

By mastering these basic methods, you can simplify square roots efficiently and accurately, making it easier to handle complex mathematical problems.

Simplifying the square root of 180 involves breaking it down into its simplest radical form. Follow these detailed steps to simplify \(\sqrt{180}\):

1. Find the Prime Factorization:

   First, we need to find the prime factors of 180.

   • Divide 180 by 2 (the smallest prime number): \(180 \div 2 = 90\)
   • Divide 90 by 2: \(90 \div 2 = 45\)
   • Divide 45 by 3 (the next smallest prime number): \(45 \div 3 = 15\)
   • Divide 15 by 3: \(15 \div 3 = 5\)
   • 5 is a prime number, so we stop here.

   The prime factorization of 180 is \(2^2 \times 3^2 \times 5\).

2. Group the Prime Factors:

   Next, group the prime factors into pairs:

   • Pairs: \(2^2\) and \(3^2\)
   • Remaining factor: 5
3. Extract the Pairs:

   Each pair of prime factors can be moved outside the square root as a single factor:

   • \(\sqrt{2^2} = 2\)
   • \(\sqrt{3^2} = 3\)

   So, \(\sqrt{2^2 \times 3^2 \times 5} = 2 \times 3 \times \sqrt{5}\).

4. Simplify the Expression:

   Multiply the factors outside the square root:

   • \(2 \times 3 = 6\)

   Thus, the simplified form of \(\sqrt{180}\) is:

   \(\sqrt{180} = 6\sqrt{5}\)

By following these steps, you can simplify \(\sqrt{180}\) to its simplest form, \(6\sqrt{5}\). This method can be applied to simplify any square root by breaking down the number into its prime factors and extracting pairs.

The Prime Factorization Method is a reliable and straightforward approach to simplifying square roots. This method involves breaking down a number into its prime factors and then simplifying the square root by extracting pairs of factors. Follow these detailed steps to apply the Prime Factorization Method:

1. Find the Prime Factorization:

   Start by finding the prime factors of the number under the square root. For example, to simplify \(\sqrt{180}\), we find the prime factors of 180:

   • Divide 180 by 2 (the smallest prime number): \(180 \div 2 = 90\)
   • Divide 90 by 2: \(90 \div 2 = 45\)
   • Divide 45 by 3 (the next smallest prime number): \(45 \div 3 = 15\)
   • Divide 15 by 3: \(15 \div 3 = 5\)
   • 5 is a prime number, so we stop here.

   The prime factorization of 180 is \(2^2 \times 3^2 \times 5\).

2. Group the Prime Factors:

   Next, group the prime factors into pairs:

   • Pairs: \(2^2\) and \(3^2\)
   • Remaining factor: 5
3. Extract the Pairs:

   Each pair of prime factors can be moved outside the square root as a single factor:

   • \(\sqrt{2^2} = 2\)
   • \(\sqrt{3^2} = 3\)

   So, \(\sqrt{2^2 \times 3^2 \times 5} = 2 \times 3 \times \sqrt{5}\).

4. Simplify the Expression:

   Multiply the factors outside the square root:

   • \(2 \times 3 = 6\)

   Thus, the simplified form of \(\sqrt{180}\) is:

   \(\sqrt{180} = 6\sqrt{5}\)

Using the Prime Factorization Method ensures an accurate and efficient way to simplify square roots, making complex expressions more manageable and easier to understand.

To simplify the square root of 180, we need to break it down into its prime factors. Prime factorization involves expressing the number as a product of its prime numbers. Here’s a detailed step-by-step guide to breaking down 180 into its prime factors:

1. Start with the Smallest Prime Number:

   Begin by dividing 180 by the smallest prime number, which is 2.

   • \(180 \div 2 = 90\)
2. Continue Dividing by 2:

   Divide the quotient by 2 again:

   • \(90 \div 2 = 45\)
3. Move to the Next Prime Number:

   Since 45 is not divisible by 2, move to the next smallest prime number, which is 3:

   • \(45 \div 3 = 15\)
4. Continue Dividing by 3:

   Divide the quotient by 3 again:

   • \(15 \div 3 = 5\)
5. Identify the Remaining Prime Number:

   Since 5 is a prime number, we stop here.

Thus, the prime factorization of 180 is:

\(180 = 2 \times 2 \times 3 \times 3 \times 5\) or \(180 = 2^2 \times 3^2 \times 5\)

Breaking down 180 into its prime factors is an essential step in simplifying its square root. With these prime factors, we can now proceed to group them and simplify the square root effectively.

Once we have the prime factorization of 180, the next step is to group the prime factors. This process helps in simplifying the square root by identifying pairs of factors that can be taken outside the square root. Here’s how to group the prime factors step-by-step:

1. Write Down the Prime Factors:

   From the previous step, we have the prime factorization of 180 as \(180 = 2^2 \times 3^2 \times 5\).

2. Identify Pairs of Prime Factors:

   Look for pairs of the same prime factor:

   • We have a pair of 2s: \(2^2\)
   • We have a pair of 3s: \(3^2\)
   • The factor 5 is unpaired.
3. Group the Pairs:

   Group the pairs and list the remaining factors separately:

   • Pairs: \(2^2\) and \(3^2\)
   • Remaining factor: 5
4. Express the Grouped Factors:

   Rewrite the expression to show the grouped pairs:

   \(\sqrt{2^2 \times 3^2 \times 5}\)

By grouping the prime factors, we prepare the expression for the next step, which involves simplifying the square root by taking these pairs outside the radical. This step is crucial as it helps in reducing the complexity of the square root and makes it easier to handle in mathematical calculations.

Extracting square roots from prime factors involves breaking down the number into its prime components and then simplifying the square root by grouping the prime factors. Let's go through the process step by step for the number 180.

1. Prime Factorization of 180:

   First, we find the prime factors of 180. This can be done by dividing 180 by the smallest prime numbers until we are left with only prime numbers.

   180 can be divided by 2:

   \( 180 \div 2 = 90 \)

   90 can be divided by 2:

   \( 90 \div 2 = 45 \)

   45 can be divided by 3:

   \( 45 \div 3 = 15 \)

   15 can be divided by 3:

   \( 15 \div 3 = 5 \)

   5 is a prime number.

   So, the prime factorization of 180 is:

   \( 180 = 2 \times 2 \times 3 \times 3 \times 5 \)

2. Grouping the Prime Factors:

   Next, we group the prime factors in pairs. For each pair of the same prime factor, we can take one of those numbers out of the square root.

   • Pair of 2's: \( 2 \times 2 \)
   • Pair of 3's: \( 3 \times 3 \)
   • Unpaired 5: \( 5 \)
3. Extracting the Square Roots:

   We can now take the square root of each pair and move them outside the square root symbol:

   \( \sqrt{2 \times 2 \times 3 \times 3 \times 5} = \sqrt{(2 \times 2) \times (3 \times 3) \times 5} \)

   This simplifies to:

   \( 2 \times 3 \times \sqrt{5} \)

4. Simplifying the Result:

   Finally, we multiply the numbers outside the square root:

   \( 2 \times 3 = 6 \)

   So, the simplified form of \( \sqrt{180} \) is:

   \( 6\sqrt{5} \)

In conclusion, the process of extracting square roots from prime factors helps us simplify the square root of 180 to \( 6\sqrt{5} \).

After breaking down 180 into its prime factors and extracting the square roots, we have:

1. Prime factorization of 180 is \(2 \times 2 \times 3 \times 3 \times 5\).
2. Grouping the prime factors in pairs: \((2 \times 2) \times (3 \times 3) \times 5\).
3. Taking the square root of each group:
   • \(\sqrt{2 \times 2} = 2\)
   • \(\sqrt{3 \times 3} = 3\)

Since 5 does not form a pair, it remains under the square root. Thus, we have:

\[
\sqrt{180} = \sqrt{(2 \times 2) \times (3 \times 3) \times 5} = 2 \times 3 \times \sqrt{5} = 6\sqrt{5}
\]

Therefore, the simplified form of \(\sqrt{180}\) is \(6\sqrt{5}\).

To summarize:

• \(180 = 2^2 \times 3^2 \times 5\)
• \(\sqrt{180} = \sqrt{2^2 \times 3^2 \times 5}\)
• \(\sqrt{180} = 2 \times 3 \times \sqrt{5}\)
• \(\sqrt{180} = 6\sqrt{5}\)

This simplification process shows that \(\sqrt{180}\) in its simplest radical form is \(6\sqrt{5}\).

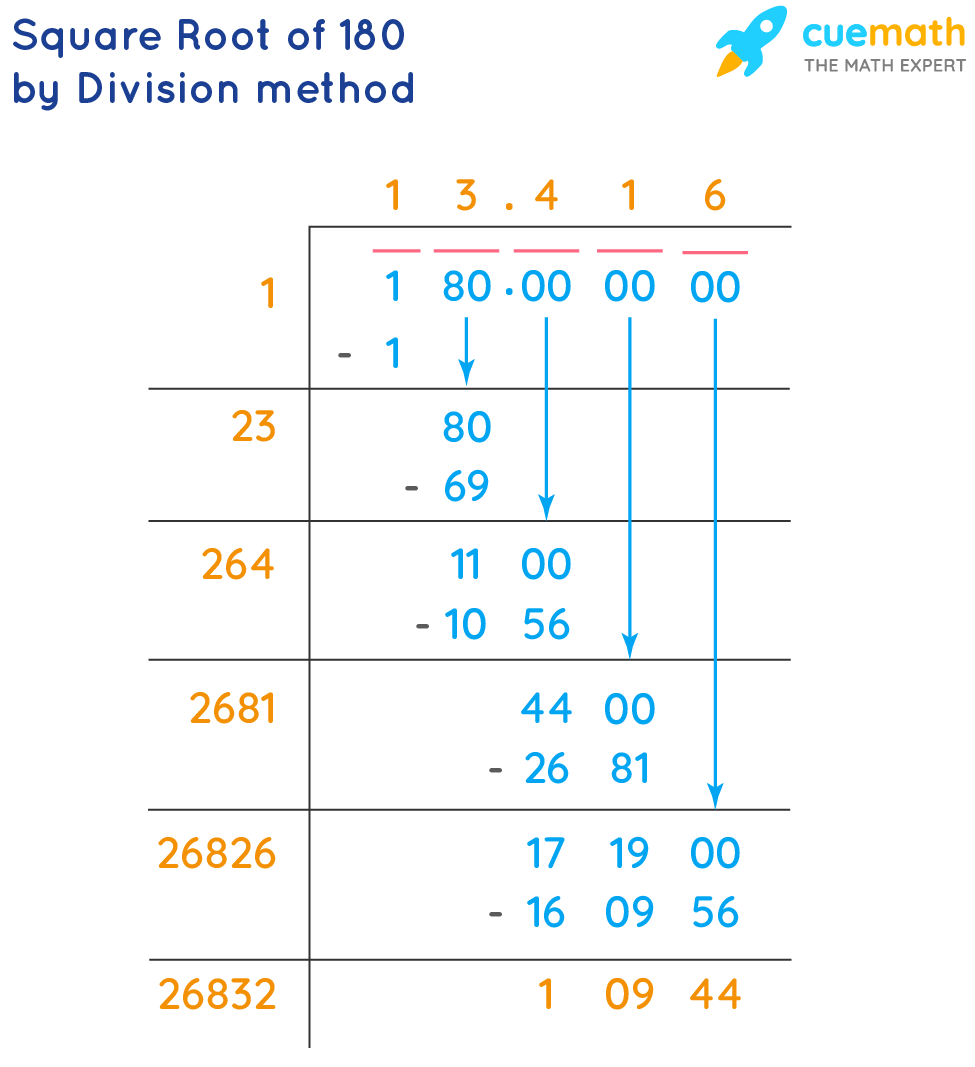
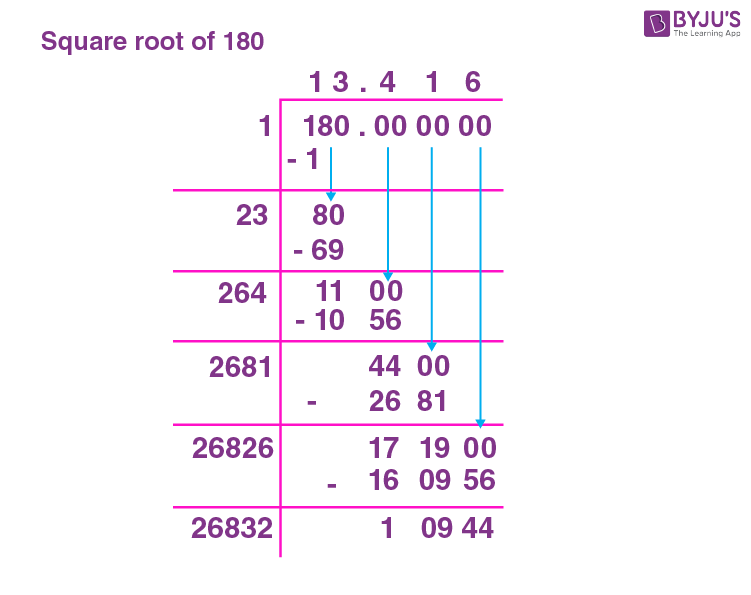
In addition to the prime factorization method, there are several alternative methods to simplify square roots. Here, we will discuss the long division method and the repeated subtraction method.

Long Division Method

The long division method is a systematic approach to find the square root of a number. Here’s how you can simplify the square root of 180 using this method:

1. Pair the digits: Starting from the decimal point, pair the digits of the number in twos. For 180, we consider 1 and 80.

2. Find the largest number: Find the largest number whose square is less than or equal to the first pair. For 1, the number is 1 (since 1² = 1).

3. Subtract and bring down the next pair: Subtract the square of the number found in the previous step from the first pair and bring down the next pair of digits. This gives us 80.

4. Double the quotient: Double the current quotient (1), which gives us 2, and find a digit x such that 2x multiplied by x is less than or equal to 80. The digit is 3 (since 23 x 3 = 69).

5. Repeat: Repeat the process for the remaining pairs. Continue this process to get a more accurate result.

The long division method can be used to find a more precise decimal approximation of square roots.

Repeated Subtraction Method

The repeated subtraction method involves subtracting successive odd numbers from the original number until you reach zero. Here’s how it works for the square root of 180:

1. Start with 180 and subtract the smallest odd number, which is 1:

   \( 180 - 1 = 179 \)

2. Subtract the next odd number, which is 3:

   \( 179 - 3 = 176 \)

3. Continue subtracting the next odd numbers (5, 7, 9, etc.):

   • \( 176 - 5 = 171 \)
   • \( 171 - 7 = 164 \)
   • \( 164 - 9 = 155 \)
   • \( 155 - 11 = 144 \)
   • \( 144 - 13 = 131 \)
   • \( 131 - 15 = 116 \)
   • \( 116 - 17 = 99 \)
   • \( 99 - 19 = 80 \)
   • \( 80 - 21 = 59 \)
   • \( 59 - 23 = 36 \)
   • \( 36 - 25 = 11 \)
   • \( 11 - 27 = -16 \)

Since the result is negative, 180 is not a perfect square, and thus the square root of 180 cannot be simplified to an integer. The method shows that the square root lies between 13 and 14.

These methods provide alternative ways to simplify square roots and understand their values in different contexts.

The factor tree method is a visual way of breaking down a number into its prime factors. Here is a step-by-step guide on how to simplify the square root of 180 using the factor tree method:

1. Start with the number 180 and begin by finding two factors of 180. For instance, 180 can be split into 18 and 10:

   $$
   
   180
   =
   18
   ×
   10
   
   

2. Next, factor each composite number (non-prime) further until all factors are prime:

   • 18 can be broken down into 2 and 9:

     $$
     
     18
     =
     2
     ×
     9
     
     

     9 can be broken down into 3 and 3:

     $$
     
     9
     =
     3
     ×
     3
     
     

   • 10 can be broken down into 2 and 5:

     $$
     
     10
     =
     2
     ×
     5
     
     

3. Now, list all the prime factors found:

   $$
   
   180
   =
   2
   ×
   2
   ×
   3
   ×
   3
   ×
   5
   
   

4. Group the prime factors into pairs to simplify the square root:

   $$
   
   
   
   180
   
   
   =
   
   
   2
   ×
   2
   ×
   3
   ×
   3
   ×
   5
   
   
   
   

   Since the square root of a product is the product of the square roots, we can simplify as follows:

   $$
   
   
   
   2
   ×
   2
   
   
   ×
   
   
   3
   ×
   3
   
   
   ×
   
   5
   
   =
   2
   ×
   3
   ×
   
   5
   
   =
   6
   
   5
   
   
   

Thus, the simplified form of the square root of 180 using the factor tree method is:

$$

6

5

Understanding the properties and rules of square roots is crucial for simplifying square roots like √180. Here are some fundamental properties and rules that can help:

• Property of Non-Negative Numbers: For any non-negative real number \(a\), the square root of \(a\) is non-negative. This is represented as \(\sqrt{a} \geq 0\).
• Square Root of a Product: The square root of a product is equal to the product of the square roots of each factor. Mathematically, this is written as:

  \[\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\]

  For example, \(\sqrt{180}\) can be broken down into \(\sqrt{36 \times 5} = \sqrt{36} \times \sqrt{5} = 6\sqrt{5}\).

• Square Root of a Quotient: The square root of a quotient is equal to the quotient of the square roots of the numerator and the denominator. This is given by:

  \[\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\]

  For instance, \(\sqrt{\frac{180}{4}} = \frac{\sqrt{180}}{\sqrt{4}} = \frac{\sqrt{180}}{2}\).

• Square Root of a Square: The square root of a number squared returns the absolute value of the original number. Expressed as:

  \[\sqrt{a^2} = |a|\]

  For example, \(\sqrt{6^2} = |6| = 6\).

• Distributive Property over Addition/Subtraction: Square roots do not distribute over addition or subtraction. This means:

  \[\sqrt{a + b} \ne \sqrt{a} + \sqrt{b}\]

  \[\sqrt{a - b} \ne \sqrt{a} - \sqrt{b}\]

  For example, \(\sqrt{25 + 9} = \sqrt{34}\), not \(5 + 3 = 8\).

Applying Square Root Properties to Simplify √180

Let's apply these properties to simplify \(\sqrt{180}\) step-by-step:

1. Factor 180 into its prime components: \(180 = 2^2 \times 3^2 \times 5\).
2. Use the product property: \(\sqrt{180} = \sqrt{2^2 \times 3^2 \times 5} = \sqrt{2^2} \times \sqrt{3^2} \times \sqrt{5}\).
3. Simplify the square roots: \(\sqrt{2^2} = 2\) and \(\sqrt{3^2} = 3\).
4. Combine the results: \(2 \times 3 \times \sqrt{5} = 6\sqrt{5}\).

Thus, the simplified form of \(\sqrt{180}\) is \(6\sqrt{5}\).

When simplifying square roots, it is easy to make mistakes that can lead to incorrect results. Here are some common pitfalls and how to avoid them:

• Misapplying the Product Property:

  It's crucial to correctly apply the property \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\). A common mistake is to assume this property holds for all forms of \(a\) and \(b\) without factoring properly.

  Example Mistake: Simplifying \(\sqrt{180}\) as \(\sqrt{18 \times 10}\) directly without checking for perfect squares.

  Correct Approach: Factor into perfect squares: \(\sqrt{180} = \sqrt{36 \times 5} = \sqrt{36} \times \sqrt{5} = 6\sqrt{5}\).

• Incorrectly Simplifying Non-Perfect Square Roots:

  A common error is to try to simplify a non-perfect square root incorrectly or assume it can be simplified further.

  Example Mistake: Assuming \(\sqrt{20}\) simplifies to \(\sqrt{10 \times 2}\) without recognizing \(\sqrt{20} = \sqrt{4 \times 5} = 2\sqrt{5}\).

  Correct Approach: Always look for the largest perfect square factor first: \(\sqrt{20} = \sqrt{4 \times 5} = 2\sqrt{5}\).

• Forgetting to Simplify Completely:

  Even after breaking down a square root, it's essential to check if further simplification is possible.

  Example Mistake: Stopping at \(\sqrt{180} = \sqrt{36 \times 5} = 6\sqrt{5}\), but not verifying if \(\sqrt{5}\) can be simplified (it can't, but always check).

  Correct Approach: Simplify step by step and check all factors for any further simplification possibilities.

• Misapplying the Quotient Property:

  The property \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\) only works correctly when both \(a\) and \(b\) are non-negative. Misapplication often happens with negative values under the square root or improper fractions.

  Example Mistake: Simplifying \(\sqrt{\frac{-9}{4}}\) as \(\frac{\sqrt{-9}}{\sqrt{4}} = \frac{i3}{2}\) without considering complex numbers context.

  Correct Approach: For real numbers, ensure both \(a\) and \(b\) are positive when using this property.

• Ignoring the Square Root of a Sum or Difference:

  Do not apply square root properties incorrectly to sums or differences. Square roots cannot be distributed over addition or subtraction.

  Example Mistake: Assuming \(\sqrt{25 + 9} = \sqrt{25} + \sqrt{9}\).

  Correct Approach: Evaluate inside the root first: \(\sqrt{25 + 9} = \sqrt{34}\), not \(5 + 3\).

• Missing the Simplification of Negative Square Roots:

  Forgetting that square roots of negative numbers involve imaginary units (\(i\)) can lead to incorrect simplification.

  Example Mistake: Treating \(\sqrt{-16}\) as simply \(4\).

  Correct Approach: Recognize the imaginary unit: \(\sqrt{-16} = 4i\).

Example of Avoiding Mistakes: Simplifying √180

Let's carefully simplify \(\sqrt{180}\) while avoiding common mistakes:

1. Factorize 180 into its prime factors: \(180 = 2^2 \times 3^2 \times 5\).
2. Use the product property correctly: \(\sqrt{180} = \sqrt{2^2 \times 3^2 \times 5} = \sqrt{2^2} \times \sqrt{3^2} \times \sqrt{5}\).
3. Simplify the perfect squares: \(\sqrt{2^2} = 2\) and \(\sqrt{3^2} = 3\).
4. Combine the results: \(2 \times 3 \times \sqrt{5} = 6\sqrt{5}\).

Thus, the simplified form of \(\sqrt{180}\) is \(6\sqrt{5}\), avoiding common errors in the process.

Simplifying square roots is not just an academic exercise; it has several practical applications across various fields. Here are some key areas where simplifying square roots is useful:

• Geometry and Trigonometry:

  Square roots often appear in geometric formulas, especially those involving triangles, circles, and areas. For example, simplifying \(\sqrt{180}\) can help in calculating the length of the sides in a right triangle where the hypotenuse is 180 units, simplifying distance calculations, and more.

  • Distance Formula: In a 2D coordinate system, the distance between two points \((x_1, y_1)\) and \((x_2, y_2)\) is given by:

    \[d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2}\]

    If the distance simplifies to \(\sqrt{180}\), knowing it simplifies to \(6\sqrt{5}\) can make further calculations easier.

  • Pythagorean Theorem: In right triangles, the lengths of the sides satisfy:

    \[a^2 + b^2 = c^2\]

    If \(a^2 + b^2 = 180\), the hypotenuse \(c\) would be \(\sqrt{180} = 6\sqrt{5}\).

• Physics and Engineering:

  Square roots are common in formulas that describe physical phenomena, such as the equations for kinetic energy, electrical circuits, and wave frequencies.

  • Wave Frequency: The formula for the frequency \(f\) of a wave is often proportional to the square root of some parameters. Simplifying the square root can make it easier to understand and predict wave behavior.
  • Electrical Circuits: In AC circuits, the RMS (Root Mean Square) voltage or current involves square roots. Simplifying these can aid in the analysis and design of circuits.
• Statistics and Probability:

  Square roots are fundamental in statistics, particularly in calculating standard deviations and variances. Simplifying these roots helps in interpreting and comparing data sets more efficiently.

  • Standard Deviation: The standard deviation of a data set measures the amount of variation or dispersion of a set of values. Simplifying square roots here helps in deriving meaningful insights.
  • Variance: Simplifying the square root in the variance formula makes it easier to understand the spread of data points around the mean.
• Algebra and Higher Mathematics:

  Simplified square roots are often easier to work with when solving algebraic equations, integrating functions, or working with complex numbers.

  • Simplifying Algebraic Expressions: Simplified forms are easier to use in equations. For instance, if \(x = \sqrt{180}\), using \(x = 6\sqrt{5}\) might simplify further algebraic manipulation.
  • Integration and Differentiation: In calculus, simplified square roots make the integration or differentiation of functions less complex and more manageable.
• Practical Daily Applications:

  Square roots simplified can also simplify practical calculations in everyday life, from computing areas and volumes to financial mathematics and even construction projects.

  • Construction: When determining the length of diagonal braces or when working with measurements, simplifying square roots helps in making accurate and quick calculations.
  • Finance: In compound interest calculations or when dealing with quadratic equations in financial models, simplifying square roots can provide clearer insights and easier computations.

Example Application: Calculating Diagonal Length

Imagine you are asked to find the diagonal length of a rectangular surface with side lengths 12 units and 15 units. Using the Pythagorean theorem:

1. Apply the formula: \[d = \sqrt{12^2 + 15^2}\]
2. Calculate the squares: \[12^2 = 144\] and \[15^2 = 225\]
3. Add and simplify the square root: \[d = \sqrt{144 + 225} = \sqrt{369}\]
4. Factorize 369 and simplify: \[369 = 3^2 \times 41\] so, \[\sqrt{369} = \sqrt{3^2 \times 41} = 3\sqrt{41}\]

Thus, the simplified form of the diagonal length is \(3\sqrt{41}\).

To master the process of simplifying square roots, it's essential to practice with a variety of examples. Here, we will provide detailed step-by-step examples and practice problems to reinforce your understanding of simplifying square roots, including \(\sqrt{180}\).

Example 1: Simplifying √180

1. Identify the prime factors of 180:

   First, factorize 180 into its prime factors:

   \[180 = 2^2 \times 3^2 \times 5\]

2. Apply the product property of square roots:

   Use the property \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\) to separate the factors:

   \[\sqrt{180} = \sqrt{2^2 \times 3^2 \times 5}\]

3. Simplify each square root:

   Simplify the square roots of the perfect squares:

   \[\sqrt{2^2} = 2\] and \[\sqrt{3^2} = 3\]

4. Combine the results:

   Multiply the simplified results with the remaining square root:

   \[2 \times 3 \times \sqrt{5} = 6\sqrt{5}\]

5. Conclusion:

   The simplified form of \(\sqrt{180}\) is \(6\sqrt{5}\).

Example 2: Simplifying √72

1. Identify the prime factors of 72:

   First, factorize 72 into its prime factors:

   \[72 = 2^3 \times 3^2\]

2. Apply the product property of square roots:

   Use the property \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\) to separate the factors:

   \[\sqrt{72} = \sqrt{2^3 \times 3^2}\]

3. Simplify each square root:

   Simplify the square roots of the perfect squares:

   \[\sqrt{3^2} = 3\]

   Since \(\sqrt{2^3}\) includes \(\sqrt{2^2} = 2\), we have:

   \[\sqrt{2^3} = \sqrt{2^2 \times 2} = 2\sqrt{2}\]

4. Combine the results:

   Multiply the simplified results with the remaining square root:

   \[3 \times 2\sqrt{2} = 6\sqrt{2}\]

5. Conclusion:

   The simplified form of \(\sqrt{72}\) is \(6\sqrt{2}\).

Practice Problems

Try to simplify the following square roots. Use the steps demonstrated in the examples above:

1. Simplify \(\sqrt{50}\)
2. Simplify \(\sqrt{98}\)
3. Simplify \(\sqrt{200}\)
4. Simplify \(\sqrt{125}\)
5. Simplify \(\sqrt{245}\)

Solutions to Practice Problems

Check your answers against these solutions:

1. \(\sqrt{50}\)
   1. Prime factorize 50: \(50 = 2 \times 5^2\)
   2. Apply the product property: \(\sqrt{50} = \sqrt{2 \times 5^2}\)
   3. Simplify: \(\sqrt{5^2} = 5\)
   4. Combine: \(5\sqrt{2}\)
   5. Answer: \(\sqrt{50} = 5\sqrt{2}\)
2. \(\sqrt{98}\)
   1. Prime factorize 98: \(98 = 2 \times 7^2\)
   2. Apply the product property: \(\sqrt{98} = \sqrt{2 \times 7^2}\)
   3. Simplify: \(\sqrt{7^2} = 7\)
   4. Combine: \(7\sqrt{2}\)
   5. Answer: \(\sqrt{98} = 7\sqrt{2}\)
3. \(\sqrt{200}\)
   1. Prime factorize 200: \(200 = 2^3 \times 5^2\)
   2. Apply the product property: \(\sqrt{200} = \sqrt{2^3 \times 5^2}\)
   3. Simplify: \(\sqrt{5^2} = 5\)
   4. Simplify \(\sqrt{2^3} = \sqrt{2^2 \times 2} = 2\sqrt{2}\)
   5. Combine: \(5 \times 2\sqrt{2} = 10\sqrt{2}\)
   6. Answer: \(\sqrt{200} = 10\sqrt{2}\)
4. \(\sqrt{125}\)
   1. Prime factorize 125: \(125 = 5^3\)
   2. Apply the product property: \(\sqrt{125} = \sqrt{5^3}\)
   3. Simplify \(\sqrt{5^3} = \sqrt{5^2 \times 5} = 5\sqrt{5}\)
   4. Combine: \(5\sqrt{5}\)
   5. Answer: \(\sqrt{125} = 5\sqrt{5}\)
5. \(\sqrt{245}\)
   1. Prime factorize 245: \(245 = 5 \times 7^2\)
   2. Apply the product property: \(\sqrt{245} = \sqrt{5 \times 7^2}\)
   3. Simplify: \(\sqrt{7^2} = 7\)
   4. Combine: \(7\sqrt{5}\)
   5. Answer: \(\sqrt{245} = 7\sqrt{5}\)

Understanding how to simplify square roots can raise several questions. Here, we address some of the most common queries to help clarify the process:

• What does it mean to simplify a square root?

  Simplifying a square root involves expressing it in its simplest form by factoring out perfect squares. For example, \(\sqrt{180}\) simplifies to \(6\sqrt{5}\) because 180 can be factored into \(36 \times 5\), where 36 is a perfect square.

• Why is it important to simplify square roots?

  Simplifying square roots can make mathematical expressions easier to understand and work with. It also helps in solving equations, comparing quantities, and making calculations more manageable.

• How do you simplify the square root of a number like 180?

  To simplify \(\sqrt{180}\), follow these steps:

  1. Factorize 180 into its prime factors: \(180 = 2^2 \times 3^2 \times 5\).
  2. Separate the perfect squares: \(\sqrt{180} = \sqrt{2^2 \times 3^2 \times 5}\).
  3. Simplify the square roots of the perfect squares: \(\sqrt{2^2} = 2\) and \(\sqrt{3^2} = 3\).
  4. Combine the results: \(2 \times 3 \times \sqrt{5} = 6\sqrt{5}\).

  Thus, the simplified form of \(\sqrt{180}\) is \(6\sqrt{5}\).

• Can all square roots be simplified?

  No, not all square roots can be simplified. Only square roots of numbers that have perfect square factors other than 1 can be simplified. For instance, \(\sqrt{5}\) cannot be simplified further because 5 is a prime number.

• What are the common mistakes to avoid when simplifying square roots?

  Some common mistakes include:

  • Not factoring the number completely.
  • Incorrectly applying the properties of square roots.
  • Ignoring negative square roots or imaginary numbers.
  • Not simplifying the square root fully.

  It's important to follow each step methodically to avoid these errors.

• How can I check if I’ve simplified a square root correctly?

  You can check your work by squaring your simplified answer. If you get back the original number under the square root, you’ve simplified it correctly. For example, \( (6\sqrt{5})^2 = 6^2 \times (\sqrt{5})^2 = 36 \times 5 = 180 \), which confirms that \(6\sqrt{5}\) is the correct simplification of \(\sqrt{180}\).

• What are some tips for simplifying complex square roots?

  Here are a few tips:

  • Break down the number into its prime factors.
  • Identify and separate perfect squares.
  • Use the properties of square roots correctly.
  • Practice with different numbers to become more familiar with the process.
• How do square roots apply to real-life problems?

  Simplifying square roots can be useful in various real-life scenarios, such as:

  • Calculating distances and lengths in geometry.
  • Solving problems in physics and engineering, like determining the magnitude of a vector or the frequency of a wave.
  • Interpreting statistical measures, such as standard deviation.
  • Performing financial calculations, such as computing interest rates.

Example Questions and Practice Problems

Here are some additional practice problems to help you reinforce your understanding of simplifying square roots:

1. What is the simplified form of \(\sqrt{144}\)?
2. Simplify \(\sqrt{50}\).
3. Find the simplified form of \(\sqrt{125}\).
4. Simplify \(\sqrt{72}\) and explain the steps.
5. What is \(\sqrt{225}\) in its simplest form?

Answers:

1. \(\sqrt{144} = 12\).
2. \(\sqrt{50} = 5\sqrt{2}\).
3. \(\sqrt{125} = 5\sqrt{5}\).
4. \(\sqrt{72} = 6\sqrt{2}\).
5. \(\sqrt{225} = 15\).

Simplifying square roots is a valuable skill that enhances our understanding and capability in mathematics. Throughout this guide, we've explored the detailed process of simplifying the square root of 180, and by extension, other square roots.

Key Points Recap

• Understanding the Concept:

  We started by understanding what it means to simplify a square root. Simplification involves expressing the square root in its simplest form by breaking it down into its prime factors and isolating the perfect squares.

• Step-by-Step Simplification of √180:

  We applied the process of simplification to \(\sqrt{180}\) by following these steps:

  1. Factorized 180 into prime factors: \(180 = 2^2 \times 3^2 \times 5\).
  2. Separated the perfect squares: \(\sqrt{180} = \sqrt{2^2 \times 3^2 \times 5}\).
  3. Simplified the perfect squares: \(\sqrt{2^2} = 2\) and \(\sqrt{3^2} = 3\).
  4. Combined the results: \(2 \times 3 \times \sqrt{5} = 6\sqrt{5}\).
  5. Final simplified form: \(\sqrt{180} = 6\sqrt{5}\).
• Common Mistakes to Avoid:

  We highlighted typical errors in simplifying square roots, such as failing to fully factorize the number or misapplying the properties of square roots.

• Applications and Practice:

  Simplifying square roots is not just an academic exercise; it has practical applications in fields like geometry, physics, and finance. Practice problems were provided to help solidify your understanding and proficiency.

Final Thoughts

Mastering the simplification of square roots equips you with a fundamental mathematical tool that simplifies complex calculations and problem-solving tasks. By consistently practicing and applying the methods outlined in this guide, you can enhance your confidence and accuracy in handling square roots.

Remember, the key to simplifying square roots effectively is understanding the properties and steps involved, and applying them meticulously. Whether you're simplifying \(\sqrt{180}\) or any other square root, the principles remain the same. Keep practicing, and soon simplifying square roots will become second nature!

We hope this guide has been helpful in your journey to mastering the simplification of square roots. Continue exploring and practicing, and you will find that these concepts will serve you well in various aspects of mathematics and beyond.