Topic square root of 18 in radical form: Discover how to simplify the square root of 18 into its radical form with ease. This guide walks you through the steps to break down √18 into \(3\sqrt{2}\), offering clear explanations and visual aids. Perfect for students and math enthusiasts looking to understand and apply radical simplification techniques effectively.
Table of Content
- Square Root of 18 in Radical Form
- Introduction to Square Roots and Radicals
- Understanding the Square Root of 18
- Step-by-Step Simplification of √18
- Prime Factorization of 18
- Applying Properties of Square Roots
- Expressing √18 in Simplest Radical Form
- Visualizing the Simplification Process
- Mathematical Explanation and Proof
- Real-Life Applications of √18
- Common Mistakes to Avoid
- FAQs about Square Roots and Radicals
- Advanced Concepts Related to Radicals
- Conclusion and Summary
- YOUTUBE: Hướng dẫn từng bước để đơn giản hóa căn bậc hai của 18, giúp bạn hiểu rõ hơn về cách làm việc với các căn bậc hai và dạng đơn giản nhất của chúng.
Square Root of 18 in Radical Form
The square root of 18 can be simplified into a radical form. This process involves expressing the square root in its simplest form by breaking it down into the product of prime factors and then simplifying the square root expression. Here's how we do it:
Steps to Simplify √18
- Find the prime factorization of 18.
- Express 18 as a product of its prime factors: \( 18 = 2 \times 3^2 \).
- Rewrite the square root of 18 using these factors: \( \sqrt{18} = \sqrt{2 \times 3^2} \).
- Apply the property of square roots: \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \).
- Simplify the expression: \( \sqrt{18} = \sqrt{2} \times \sqrt{3^2} = \sqrt{2} \times 3 \).
Therefore, the square root of 18 in its simplest radical form is:
\( \sqrt{18} = 3\sqrt{2} \)
Visual Representation
Below is a visual table to summarize the simplification process:
| Expression | Transformation |
|---|---|
| \( \sqrt{18} \) | Original expression |
| \( \sqrt{2 \times 3^2} \) | Breaking 18 into its prime factors |
| \( \sqrt{2} \times \sqrt{3^2} \) | Applying the property of square roots |
| \( \sqrt{2} \times 3 \) | Simplifying the square root of 9 to 3 |
| \( 3\sqrt{2} \) | Final simplified form |
This simplified form \( 3\sqrt{2} \) is the radical form of the square root of 18.

READ MORE:
Introduction to Square Roots and Radicals
Square roots and radicals are fundamental concepts in mathematics, used to find the original number that was squared to obtain a given value. Understanding these concepts is essential for solving equations, simplifying expressions, and exploring higher-level math. Let's delve into the basics of square roots and radicals and learn how to simplify them.
A square root of a number \( x \) is a number \( y \) such that \( y^2 = x \). For example, since \( 3^2 = 9 \), 3 is a square root of 9. We denote the square root of \( x \) as \( \sqrt{x} \). When dealing with positive numbers, the square root will also be positive.
Radicals, often called root expressions, extend beyond square roots. They include cube roots (\( \sqrt[3]{x} \)), fourth roots (\( \sqrt[4]{x} \)), and so on. The general form of a radical expression is \( \sqrt[n]{x} \), where \( n \) is the degree of the root. For square roots, \( n \) is typically 2 and often omitted, as in \( \sqrt{x} \).
Here's a step-by-step process to understand and simplify square roots:
- Identify the number under the square root symbol: This is called the radicand. For instance, in \( \sqrt{18} \), 18 is the radicand.
- Prime factorize the radicand: Break down the radicand into its prime factors. For 18, the prime factors are \( 2 \times 3 \times 3 \).
- Group the factors: Pair the prime factors in groups of two. For 18, we have \( 3 \times 3 \). Each pair can be simplified outside the radical.
- Simplify the radical expression: Move the pairs out of the radical as a single factor. For \( \sqrt{18} \), we can take out 3, resulting in \( 3\sqrt{2} \).
Let's summarize the steps with an example:
| Step | Example: Simplifying \( \sqrt{18} \) |
|---|---|
| Identify the radicand | 18 |
| Prime factorize 18 | \( 18 = 2 \times 3^2 \) |
| Group the factors | \( 18 = 2 \times (3 \times 3) \) |
| Simplify the radical | \( \sqrt{18} = \sqrt{2 \times 3^2} = 3\sqrt{2} \) |
By mastering these steps, you can simplify any square root or radical expression. Radicals are not only crucial for algebra but also form the basis for more complex mathematical concepts and applications.
Understanding the Square Root of 18
The square root of 18 is an intriguing number that can be simplified to a more concise radical form. This process involves breaking down the number under the square root, known as the radicand, into its prime factors. By understanding this simplification, we gain a clearer insight into the properties and applications of square roots.
Let's explore the square root of 18 step by step:
- Identify the Radicand:
The radicand in this case is 18. We are looking to simplify \( \sqrt{18} \).
- Prime Factorize the Radicand:
Break down 18 into its prime factors. Prime factorization involves expressing 18 as a product of prime numbers.
For 18, the prime factors are \( 2 \times 3 \times 3 \) or \( 2 \times 3^2 \).
- Group the Factors:
To simplify the square root, we group the factors in pairs. For the number 18, we have a pair of 3s.
This can be written as \( \sqrt{2 \times 3^2} \).
- Simplify the Expression:
Using the property of square roots \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \), we can simplify the expression.
For \( \sqrt{18} \), we simplify it as \( \sqrt{2 \times 3^2} \).
Since the square root of \( 3^2 \) is 3, we move 3 outside the radical:
\( \sqrt{2} \times 3 = 3\sqrt{2} \).
Thus, the square root of 18 simplifies to \( 3\sqrt{2} \). This is the most reduced form of the square root of 18, making it easier to work with in mathematical calculations.
To summarize the steps visually, here’s a quick overview:
| Step | Details |
|---|---|
| Identify the Radicand | 18 |
| Prime Factorize 18 | \( 18 = 2 \times 3^2 \) |
| Group the Factors | \( \sqrt{2 \times 3^2} \) |
| Simplify the Expression | \( \sqrt{2} \times 3 = 3\sqrt{2} \) |
By following these steps, you can simplify the square root of any number and understand the concept behind radical expressions more deeply.
Step-by-Step Simplification of √18
Simplifying the square root of 18, \( \sqrt{18} \), involves a series of steps that help break down the number into a more manageable and understandable form. Follow these detailed steps to transform \( \sqrt{18} \) into its simplest radical form.
- Identify the Radicand:
The radicand is the number under the square root symbol. Here, the radicand is 18. We start with \( \sqrt{18} \).
- Prime Factorize the Radicand:
Find the prime factors of 18. Prime factorization means breaking down the number into prime numbers that, when multiplied together, give the original number.
The prime factors of 18 are \( 2 \) and \( 3 \times 3 \). We can write this as \( 18 = 2 \times 3^2 \).
- Rewrite the Radicand using Prime Factors:
Express the radicand under the square root as a product of its prime factors. Using our prime factorization, we get:
\( \sqrt{18} = \sqrt{2 \times 3^2} \).
- Apply the Property of Square Roots:
Use the property \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \) to separate the factors inside the square root:
\( \sqrt{2 \times 3^2} = \sqrt{2} \times \sqrt{3^2} \).
- Simplify the Square Root:
Evaluate the square root of the perfect square (if any). Here, \( 3^2 \) is a perfect square, and \( \sqrt{3^2} = 3 \).
So, \( \sqrt{2} \times \sqrt{3^2} \) simplifies to \( \sqrt{2} \times 3 \).
- Combine the Results:
Multiply the numbers outside the square root to get the simplified form:
\( \sqrt{18} = 3 \times \sqrt{2} \) or \( 3\sqrt{2} \).
Therefore, the simplified radical form of \( \sqrt{18} \) is \( 3\sqrt{2} \).
To visualize this process, let's use a table to summarize each step:
| Step | Details |
|---|---|
| Identify the Radicand | \( \sqrt{18} \) |
| Prime Factorize | \( 18 = 2 \times 3^2 \) |
| Rewrite as a Product of Factors | \( \sqrt{18} = \sqrt{2 \times 3^2} \) |
| Apply the Property of Square Roots | \( \sqrt{2 \times 3^2} = \sqrt{2} \times \sqrt{3^2} \) |
| Simplify the Perfect Square | \( \sqrt{3^2} = 3 \) |
| Combine the Results | \( \sqrt{2} \times 3 = 3\sqrt{2} \) |
By following these steps, you can simplify any square root expression into its most understandable and usable form. This methodical approach not only simplifies calculations but also enhances your comprehension of radical expressions.
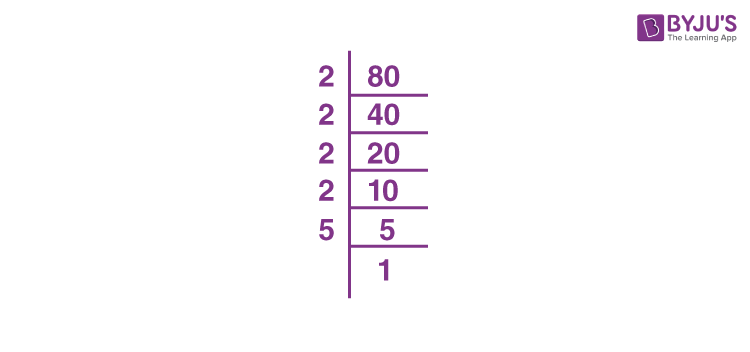
Prime Factorization of 18
Prime factorization is a crucial step in simplifying square roots and other mathematical operations. It involves breaking down a composite number into its prime number components, which are the building blocks of the number. Let's explore the prime factorization of 18 and how this process helps simplify the square root of 18.
The number 18 is a composite number, meaning it can be divided by numbers other than 1 and itself. The goal is to express 18 as a product of prime numbers. Here’s how we can find the prime factors of 18:
- Start with the smallest prime number:
Begin by dividing 18 by the smallest prime number, which is 2.
\( 18 \div 2 = 9 \)
This gives us the first prime factor: 2.
- Factor the resulting quotient:
Next, take the quotient obtained (9) and continue factorizing it by prime numbers.
Since 9 is not divisible by 2, move to the next smallest prime number, which is 3.
\( 9 \div 3 = 3 \)
We have another prime factor: 3.
- Factor until you reach a prime number:
The quotient now is 3, which is a prime number. Hence, we have factored 9 into prime numbers: \( 3 \times 3 \).
- Combine all the prime factors:
Now, list all the prime factors found in the process.
Combining all the prime factors, we have:
\( 18 = 2 \times 3 \times 3 \) or \( 18 = 2 \times 3^2 \).
Here’s a visual summary of the prime factorization of 18:
| Step | Details |
|---|---|
| Start with 18 | \( 18 \div 2 = 9 \) (2 is a prime factor) |
| Factorize 9 | \( 9 \div 3 = 3 \) (3 is a prime factor) |
| Prime Factorize 3 | 3 is already a prime number |
| Combine Prime Factors | \( 18 = 2 \times 3 \times 3 = 2 \times 3^2 \) |
With the prime factors of 18 identified as \( 2 \) and \( 3^2 \), we can use this breakdown to simplify the square root of 18 more effectively. The factorization into \( 2 \times 3^2 \) allows us to simplify \( \sqrt{18} \) by recognizing \( 3^2 \) as a perfect square, which simplifies to 3, leaving us with \( 3\sqrt{2} \).
Understanding the prime factorization of a number not only aids in simplifying square roots but also enhances your overall problem-solving skills in mathematics.
Applying Properties of Square Roots
The properties of square roots are powerful tools that simplify expressions and solve equations involving radicals. By understanding and applying these properties, we can efficiently work with square roots in various mathematical contexts. Let's explore these properties and apply them to the square root of 18.
Key properties of square roots include:
- Product Property:
The square root of a product is the product of the square roots of the factors:
\( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \)
For example, \( \sqrt{18} \) can be expressed as \( \sqrt{2 \times 9} = \sqrt{2} \times \sqrt{9} \).
- Quotient Property:
The square root of a quotient is the quotient of the square roots of the numerator and the denominator:
\( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \), where \( b \neq 0 \).
For instance, \( \sqrt{\frac{18}{4}} = \frac{\sqrt{18}}{\sqrt{4}} = \frac{3\sqrt{2}}{2} \).
- Simplifying Radical Expressions:
To simplify \( \sqrt{a} \), express \( a \) as the product of a perfect square and another number:
\( \sqrt{a \times b^2} = b \times \sqrt{a} \).
For \( \sqrt{18} \), rewrite 18 as \( 2 \times 9 \). Since \( 9 = 3^2 \), we simplify as:
\( \sqrt{18} = \sqrt{2 \times 3^2} = 3\sqrt{2} \).
- Addition and Subtraction of Radicals:
Only like radicals can be added or subtracted. Like radicals have the same radicand and index:
\( a\sqrt{b} + c\sqrt{b} = (a + c)\sqrt{b} \).
For example, \( 2\sqrt{18} - \sqrt{18} = (2 - 1)\sqrt{18} = \sqrt{18} = 3\sqrt{2} \).
To understand these properties better, let's see how they apply to the square root of 18 in a detailed step-by-step manner:
- Use the Product Property:
We start by using the product property of square roots to break down \( \sqrt{18} \).
Since \( 18 = 2 \times 9 \), we can write:
\( \sqrt{18} = \sqrt{2 \times 9} = \sqrt{2} \times \sqrt{9} \).
- Simplify the Perfect Square:
The number 9 is a perfect square (since \( 3^2 = 9 \)). We simplify \( \sqrt{9} \) to 3:
\( \sqrt{2} \times \sqrt{9} = \sqrt{2} \times 3 = 3\sqrt{2} \).
Let’s summarize the steps in a table for clarity:
| Step | Details |
|---|---|
| Rewrite the radicand | \( \sqrt{18} = \sqrt{2 \times 9} \) |
| Apply the Product Property | \( \sqrt{2 \times 9} = \sqrt{2} \times \sqrt{9} \) |
| Simplify the perfect square | \( \sqrt{9} = 3 \) |
| Combine the results | \( \sqrt{2} \times 3 = 3\sqrt{2} \) |
By applying these properties, you can simplify and manipulate radical expressions more effectively, making them easier to understand and work with in various mathematical problems.
Expressing √18 in Simplest Radical Form
Simplifying the square root of 18, \( \sqrt{18} \), into its simplest radical form involves a few methodical steps. This process transforms the expression into a form that is more straightforward to work with in various mathematical contexts. Here’s a detailed guide on how to express \( \sqrt{18} \) in its simplest radical form.
- Start with the Original Expression:
The original expression is \( \sqrt{18} \). Our goal is to simplify this expression.
- Perform Prime Factorization:
First, we break down 18 into its prime factors. Prime factorization of 18 gives us:
\( 18 = 2 \times 9 \) and since \( 9 = 3 \times 3 \), we can write:
\( 18 = 2 \times 3^2 \).
- Rewrite Using Prime Factors:
Substitute the prime factorization into the square root:
\( \sqrt{18} = \sqrt{2 \times 3^2} \).
- Apply the Product Property of Square Roots:
Utilize the property \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \) to separate the factors under the square root:
\( \sqrt{2 \times 3^2} = \sqrt{2} \times \sqrt{3^2} \).
- Simplify the Perfect Square:
Since \( 3^2 = 9 \) and \( \sqrt{9} = 3 \), we simplify \( \sqrt{3^2} \) to 3:
\( \sqrt{2} \times \sqrt{9} = \sqrt{2} \times 3 = 3\sqrt{2} \).
- Combine the Results:
Multiply the numbers outside the radical sign to get the simplest form:
\( \sqrt{18} = 3\sqrt{2} \).
Thus, the simplest radical form of \( \sqrt{18} \) is \( 3\sqrt{2} \). This form is more elegant and often easier to use in further calculations.
Here’s a quick reference table summarizing the steps:
| Step | Details |
|---|---|
| Start with the Expression | \( \sqrt{18} \) |
| Prime Factorization | \( 18 = 2 \times 3^2 \) |
| Rewrite under Square Root | \( \sqrt{18} = \sqrt{2 \times 3^2} \) |
| Apply Product Property | \( \sqrt{2 \times 3^2} = \sqrt{2} \times \sqrt{9} \) |
| Simplify the Perfect Square | \( \sqrt{9} = 3 \) |
| Combine the Results | \( \sqrt{2} \times 3 = 3\sqrt{2} \) |
By understanding these steps and properties, you can simplify any square root expression efficiently, leading to a deeper comprehension of radical forms and their applications.
Visualizing the Simplification Process
To simplify the square root of 18, we can break down the process into clear steps. This visualization will help in understanding each part of the simplification:
- Identify the prime factors of 18:
First, we perform the prime factorization of 18.
18 = 2 × 3 × 3
- Group the prime factors in pairs:
We look for pairs of identical factors to simplify the radical.
18 = 2 × (3 × 3)
- Extract the square root of the paired factors:
The square root of 3 × 3 is 3.
Therefore, √(3 × 3) = 3
- Write the simplified form:
Since 18 = 2 × (3 × 3), we can simplify √18 as follows:
√18 = √(2 × 3 × 3) = 3√2
Here's a detailed visual breakdown:
| Step | Action | Result |
|---|---|---|
| Prime Factorization | 18 = 2 × 3 × 3 | 18 = 2 × 32 |
| Group Pairs | 18 = 2 × (3 × 3) | 32 = 9 |
| Extract Square Root | √(3 × 3) = 3 | 3√2 |
| Final Simplification | √18 = √(2 × 32) | √18 = 3√2 |
Thus, the simplified radical form of √18 is 3√2.
Using MathJax, we can express this process mathematically as follows:
Mathematical Explanation and Proof
In this section, we will provide a detailed mathematical explanation and proof for finding the square root of 18 in its simplest radical form.
The square root of a number is a value that, when multiplied by itself, gives the original number. For 18, we want to find the value of \( \sqrt{18} \) such that:
\( \sqrt{18} = x \)
\( x^2 = 18 \)
Prime Factorization Method
We can simplify \( \sqrt{18} \) by using prime factorization. Let's start by finding the prime factors of 18.
- 18 can be factored into prime numbers as: \( 18 = 2 \times 3^2 \).
We can rewrite the square root of 18 using its prime factors:
\( \sqrt{18} = \sqrt{2 \times 3^2} \)
Using the property of square roots that \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \), we can simplify:
\( \sqrt{18} = \sqrt{2} \times \sqrt{3^2} \)
Since \( \sqrt{3^2} = 3 \), we get:
\( \sqrt{18} = 3 \sqrt{2} \)
Verification
To verify, let's square \( 3 \sqrt{2} \) and check if we get 18:
\( (3 \sqrt{2})^2 = 3^2 \times (\sqrt{2})^2 = 9 \times 2 = 18 \)
This confirms that \( \sqrt{18} = 3 \sqrt{2} \).
Properties of Square Roots
Let's consider some properties of square roots to further understand the simplification:
- The square root of a product is the product of the square roots: \( \sqrt{ab} = \sqrt{a} \times \sqrt{b} \).
- The square root of a square number \( a^2 \) is \( a \): \( \sqrt{a^2} = a \).
Conclusion
By using prime factorization and properties of square roots, we have shown that the square root of 18 in its simplest radical form is:
\( \sqrt{18} = 3 \sqrt{2} \)

Real-Life Applications of √18
The square root of 18, expressed as \(3\sqrt{2}\), finds its utility in various real-life applications across different fields. Below are some detailed examples:
-
Engineering and Construction:
Square roots are crucial in engineering and construction, especially when applying the Pythagorean theorem. For instance, when determining the length of the hypotenuse of a right-angled triangle with legs of known lengths, the square root function helps in accurate calculations.
-
Architecture and Design:
Architects often use square roots when working with right triangles and other geometrical shapes in their designs. Understanding square roots helps in the calculation of diagonal distances and structural integrity.
-
Physics and Natural Sciences:
Square roots are used in physics for calculating distances and speeds, particularly in equations derived from the Pythagorean theorem. For example, finding the resultant vector when two perpendicular components are known involves taking the square root of the sum of the squares of these components.
-
Finance:
In finance, square roots are used in various calculations, including the determination of interest rates and the analysis of financial risks. The standard deviation, a key concept in finance, involves square root calculations to understand the volatility of investments.
-
Probability and Statistics:
Square roots are essential in statistics, particularly in the computation of standard deviations and variances, which are used to measure the spread or dispersion of a set of data points. This is crucial in fields like quality control and market analysis.
-
Distance Calculation:
Square roots are used to calculate distances in both two-dimensional and three-dimensional spaces. For example, the distance formula \(D = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2}\) is derived from the Pythagorean theorem and is widely used in navigation and mapping technologies.
Understanding the applications of square roots, including the square root of 18, highlights their importance in both practical and theoretical scenarios, facilitating accurate measurements and calculations in various fields.
Common Mistakes to Avoid
When working with the square root of 18 in radical form, several common mistakes can occur. Here, we highlight these errors and explain how to avoid them:
-
Incorrectly Simplifying the Radical:
One common mistake is attempting to simplify \(\sqrt{18}\) incorrectly. The correct simplification is:
- Perform prime factorization of 18: \(18 = 2 \times 3 \times 3\).
- Pair the prime factors: \(18 = 2 \times 3^2\).
- Simplify by taking the square root of the paired factor: \(\sqrt{18} = \sqrt{2 \times 3^2} = 3\sqrt{2}\).
A common error is to try to simplify each factor under the radical separately, which is incorrect.
-
Misunderstanding Properties of Radicals:
Another mistake is misunderstanding how to apply properties of radicals, such as:
\(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\), which holds true only if \(a\) and \(b\) are non-negative. For example:
- \(\sqrt{18} = \sqrt{2 \times 9} = \sqrt{2} \times \sqrt{9} = \sqrt{2} \times 3 = 3\sqrt{2}\).
-
Leaving Radicals in the Denominator:
It is often required to rationalize the denominator. For example, instead of writing \(\frac{1}{\sqrt{18}}\), rationalize the denominator:
- Multiply numerator and denominator by \(\sqrt{18}\): \(\frac{1}{\sqrt{18}} \times \frac{\sqrt{18}}{\sqrt{18}} = \frac{\sqrt{18}}{18}\).
- Simplify \(\frac{\sqrt{18}}{18} = \frac{3\sqrt{2}}{18} = \frac{\sqrt{2}}{6}\).
-
Incorrect Decimal Approximations:
While \(\sqrt{18}\) approximately equals 4.2426, ensure that when performing calculations, you maintain appropriate precision and avoid truncating too early. Always carry through more decimal places and round only at the end of your calculations.
-
Assuming the Square Root of a Non-Perfect Square is Rational:
The square root of 18 is irrational, which means it cannot be expressed as a simple fraction. Misunderstanding this can lead to incorrect assumptions and results in further calculations.
By avoiding these common mistakes, you can ensure accurate and correct simplification and use of \(\sqrt{18}\) in various mathematical contexts.
FAQs about Square Roots and Radicals
-
What is the square root of 18?
The square root of 18 is expressed as \( \sqrt{18} \) which simplifies to \( 3\sqrt{2} \). In decimal form, it is approximately 4.2426.
-
Is 18 a perfect square?
No, 18 is not a perfect square. A perfect square is a number that can be expressed as the square of an integer. Since the square root of 18 is not an integer, 18 is not a perfect square.
-
Is the square root of 18 a rational or irrational number?
The square root of 18 is an irrational number because it cannot be expressed as a fraction \( \frac{p}{q} \) where \( p \) and \( q \) are integers and \( q \) is not zero. Its decimal representation is non-terminating and non-repeating.
-
How do you simplify the square root of 18?
The square root of 18 can be simplified by factoring 18 into its prime factors: \( 18 = 2 \times 3^2 \). Taking the square root of both sides, we get \( \sqrt{18} = \sqrt{2 \times 3^2} = 3\sqrt{2} \).
-
What is the square of 18?
The square of 18 is calculated by multiplying 18 by itself: \( 18^2 = 18 \times 18 = 324 \).
-
Is 18 a perfect cube?
No, 18 is not a perfect cube. A perfect cube is a number that can be expressed as the cube of an integer. The cube root of 18 is not an integer.
Advanced Concepts Related to Radicals
Radicals are fundamental in various branches of mathematics and have intriguing properties and applications. Here, we delve into advanced concepts related to radicals to enhance your understanding.
1. Rational Exponents and Radicals
Radicals can be expressed using rational exponents. For example, the nth root of a number \(a\) can be written as \(a^{1/n}\). This notation helps in simplifying and manipulating expressions involving radicals.
For instance:
- \(\sqrt[n]{a} = a^{1/n}\)
- \(\sqrt[3]{8} = 8^{1/3} = 2\)
2. Properties of Radicals
Understanding the properties of radicals is crucial for simplifying complex expressions. Key properties include:
- \(\sqrt{ab} = \sqrt{a} \cdot \sqrt{b}\)
- \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\)
- \((\sqrt{a})^n = a^{n/2}\)
These properties allow for the transformation and simplification of radical expressions.
3. Conjugate Pairs
Radicals often appear in denominators, and it's a standard practice to rationalize them. This is done using conjugate pairs:
For example, to rationalize \(\frac{1}{\sqrt{2} + 1}\), multiply by the conjugate \(\sqrt{2} - 1\):
\[
\frac{1}{\sqrt{2} + 1} \cdot \frac{\sqrt{2} - 1}{\sqrt{2} - 1} = \frac{\sqrt{2} - 1}{2 - 1} = \sqrt{2} - 1
\]
4. Complex Numbers and Radicals
Radicals extend into the realm of complex numbers. The square root of a negative number involves imaginary units:
\[
\sqrt{-a} = \sqrt{a} \cdot i \quad \text{where} \quad i^2 = -1
\]
This forms the basis for complex numbers, essential in advanced mathematics, engineering, and physics.
5. Higher-Order Radicals
Radicals are not limited to square roots. Higher-order radicals, such as cube roots and fourth roots, follow similar properties:
- \(\sqrt[3]{a} = a^{1/3}\)
- \(\sqrt[4]{a} = a^{1/4}\)
These higher-order roots are crucial in solving polynomial equations and analyzing functions.
6. Applications in Calculus
Radicals play a significant role in calculus, particularly in integration and differentiation. Functions involving radicals often require substitution methods or special techniques for integration:
For example, to integrate \(\int \sqrt{x} \, dx\), use the substitution \(u = \sqrt{x}\), leading to:
\[
\int \sqrt{x} \, dx = \int u^2 \cdot 2u \, du = \frac{2}{3} u^3 = \frac{2}{3} x^{3/2}
\]
7. Practical Applications
Radicals are used in various fields including physics (e.g., calculating distances and velocities), engineering (e.g., stress analysis), and statistics (e.g., standard deviation calculation).
For instance, the Pythagorean theorem in geometry, \(c = \sqrt{a^2 + b^2}\), is a direct application of square roots to determine the length of the hypotenuse in a right triangle.
Understanding these advanced concepts helps in mastering the use of radicals in different mathematical contexts and real-world applications.
Conclusion and Summary
Understanding the square root of 18 in its simplest radical form involves breaking down the number into its prime factors and applying the properties of square roots. This process not only simplifies the expression but also provides a clear and concise way to represent irrational numbers.
Here are the key points covered in this guide:
- The square root of 18 can be simplified by factorizing 18 into its prime factors.
- 18 can be expressed as \( 2 \times 3^2 \).
- Using the property of square roots, \( \sqrt{ab} = \sqrt{a} \times \sqrt{b} \), we can simplify \( \sqrt{18} \) as \( \sqrt{2 \times 3^2} \).
- This simplifies further to \( \sqrt{2} \times \sqrt{3^2} \), which results in \( 3\sqrt{2} \).
Thus, the square root of 18 in its simplest radical form is \( 3\sqrt{2} \).
The step-by-step process and visualizations provided throughout this guide should help in understanding the simplification process. This foundation is essential for further mathematical studies and real-life applications where precise calculations involving radicals are necessary.
By avoiding common mistakes and applying the correct mathematical principles, anyone can master the simplification of square roots. We hope this comprehensive guide has been helpful in demystifying the concept of radicals and providing clear, practical steps for simplification.
Thank you for reading our guide on simplifying the square root of 18!
Hướng dẫn từng bước để đơn giản hóa căn bậc hai của 18, giúp bạn hiểu rõ hơn về cách làm việc với các căn bậc hai và dạng đơn giản nhất của chúng.
Cách Đơn Giản Hóa Căn Bậc Hai của 18: √18
READ MORE:
Hướng dẫn chi tiết về cách tính và đơn giản hóa căn bậc hai của 18, giúp bạn hiểu rõ hơn về dạng đơn giản nhất của căn bậc hai.
Căn Bậc Hai của 18