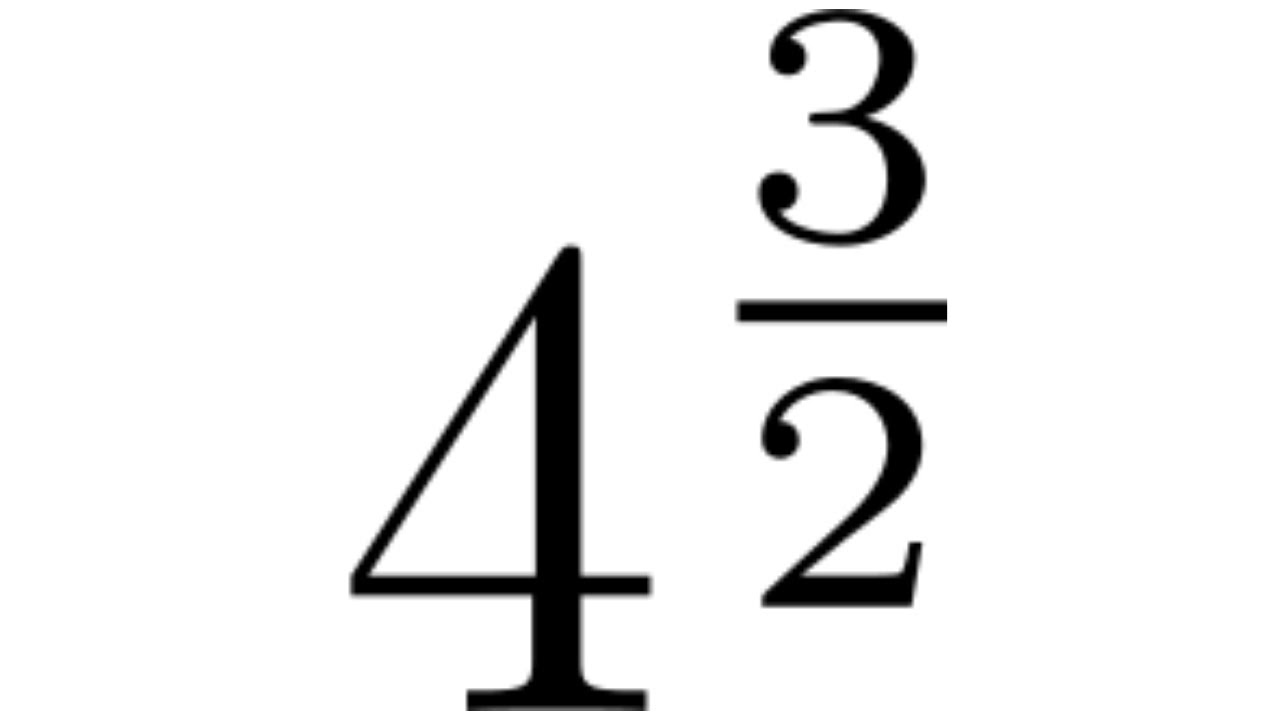Topic what are the square root numbers: Understanding square root numbers is fundamental in mathematics. This concept involves finding a number that, when multiplied by itself, gives the original number. In this article, we explore various methods to calculate square roots, the properties of square root numbers, and their applications in solving equations. Dive into this guide to master the essentials of square root numbers.
Table of Content
- Understanding Square Root Numbers
- Introduction to Square Root Numbers
- Definition of Square Roots
- Understanding Squares and Square Roots
- Methods to Calculate Square Roots
- Square Roots of Perfect Squares
- Square Roots of Non-Perfect Squares
- Square Root Function and Its Graph
- Common Square Root Values
- Solving Equations Using Square Roots
- Square Roots in Real Life
- FAQs about Square Roots
- YOUTUBE: Video này giới thiệu về khái niệm căn bậc hai, cách tính toán và ứng dụng trong thực tế.
Understanding Square Root Numbers
The square root of a number is a value that, when multiplied by itself, gives the original number. The square root is denoted by the radical symbol "√". For instance, the square root of 9 is 3, since 3 × 3 = 9.
Properties of Square Roots
- The square root of a positive number has two values: one positive and one negative. For example, √16 = 4 and -4, because both 4 × 4 and -4 × -4 equal 16.
- The square root of 0 is 0.
- Square roots of perfect squares are integers (e.g., √25 = 5), while square roots of non-perfect squares are irrational numbers (e.g., √2 ≈ 1.414).
Calculating Square Roots
Square roots can be calculated using various methods:
- Prime Factorization: For perfect squares, break down the number into its prime factors. Pair the factors and take one from each pair. For example, for 36, the prime factors are 2 × 2 × 3 × 3, so √36 = 2 × 3 = 6.
- Long Division Method: This method is useful for non-perfect squares. It involves a process similar to long division.
- Using a Calculator: For quick and accurate results, a calculator can be used to find square roots, especially for non-perfect squares.
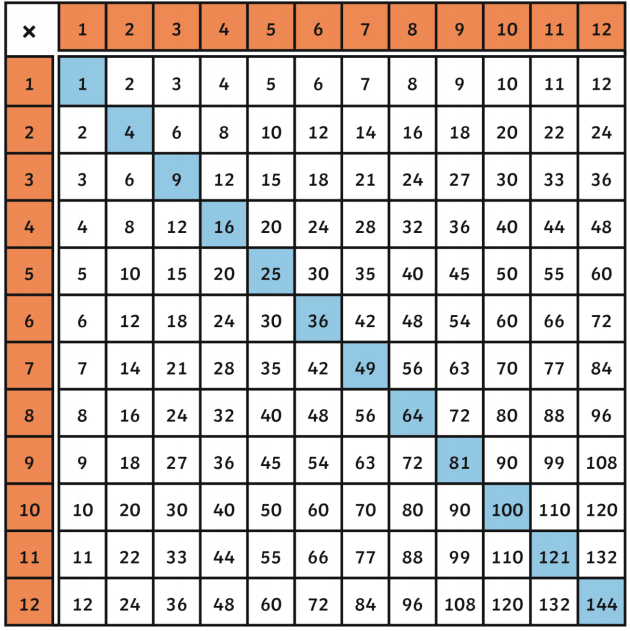
Table of Square Roots from 1 to 20
| Number | Square | Square Root |
|---|---|---|
| 1 | 1 | 1.000 |
| 2 | 4 | 1.414 |
| 3 | 9 | 1.732 |
| 4 | 16 | 2.000 |
| 5 | 25 | 2.236 |
| 6 | 36 | 2.449 |
| 7 | 49 | 2.646 |
| 8 | 64 | 2.828 |
| 9 | 81 | 3.000 |
| 10 | 100 | 3.162 |
| 11 | 121 | 3.317 |
| 12 | 144 | 3.464 |
| 13 | 169 | 3.606 |
| 14 | 196 | 3.742 |
| 15 | 225 | 3.873 |
| 16 | 256 | 4.000 |
| 17 | 289 | 4.123 |
| 18 | 324 | 4.243 |
| 19 | 361 | 4.359 |
| 20 | 400 | 4.472 |
Applications of Square Roots
- Geometry: Calculating the side length of a square when its area is known.
- Physics: Determining quantities like RMS (Root Mean Square) in electrical engineering.
- Statistics: Standard deviation calculations in data analysis.
Understanding square roots is fundamental in various fields of science, engineering, and mathematics. By mastering the concept and methods of calculating square roots, one can solve a wide range of practical and theoretical problems.

READ MORE:
Introduction to Square Root Numbers
The square root of a number is a value that, when multiplied by itself, gives the original number. This concept is fundamental in mathematics and is represented using the radical symbol '√'. For instance, the square root of 9 is 3 because 3 × 3 = 9. Similarly, the square root of 16 is 4 since 4 × 4 = 16.
Square roots can be classified into perfect squares and non-perfect squares. Perfect squares have whole number square roots, such as 1, 4, 9, and 16, while non-perfect squares like 2, 3, and 5 have irrational square roots that are non-repeating and non-terminating decimals.
There are several methods to find square roots, including:
- Prime Factorization: Express the number as a product of its prime factors and pair them to find the square root.
- Repeated Subtraction: Subtract consecutive odd numbers from the original number until you reach zero, counting the steps taken.
- Long Division Method: A manual method to find more precise square roots, often used for larger numbers.
- Estimation: Useful for quick approximations, involving finding the nearest perfect squares between which the number lies.
Understanding square roots is essential for solving various algebraic equations, especially quadratic equations. The square root function, denoted as f(x) = √x, is widely used in mathematical problems and real-life applications.
In summary, square roots are integral to mathematics, providing a basis for more advanced concepts and practical problem-solving techniques.
Definition of Square Roots
A square root of a number is a value that, when multiplied by itself, gives the original number. The square root operation is the inverse of squaring a number. Mathematically, if \( x^2 = y \), then \( x \) is the square root of \( y \). The symbol for square root is \( \sqrt{} \). For example, \( \sqrt{16} = 4 \) because \( 4 \times 4 = 16 \).
Square roots can be both positive and negative. For instance, \( \sqrt{16} = 4 \) and \( -4 \) because \( (-4) \times (-4) = 16 \). However, by convention, the square root symbol \( \sqrt{} \) typically refers to the positive square root.
To illustrate:
- \( \sqrt{25} = 5 \) because \( 5 \times 5 = 25 \)
- \( \sqrt{36} = 6 \) because \( 6 \times 6 = 36 \)
- \( \sqrt{49} = 7 \) because \( 7 \times 7 = 49 \)
Square roots are crucial in various mathematical contexts, including solving quadratic equations, simplifying radical expressions, and in real-world applications such as calculating areas.
Understanding Squares and Square Roots
Squares and square roots are fundamental concepts in mathematics, with a deep interrelation where one operation is the inverse of the other. Understanding these concepts is crucial for solving various algebraic equations and real-world problems.
Squares
The square of a number is obtained by multiplying the number by itself. It is represented mathematically as \( x^2 \). For example:
- \( 4^2 = 4 \times 4 = 16 \)
- \( (-5)^2 = -5 \times -5 = 25 \)
- \( \left(\frac{3}{2}\right)^2 = \frac{3}{2} \times \frac{3}{2} = \frac{9}{4} \)
In general, for any real number \( a \), its square is denoted as \( a^2 \).
Square Roots
The square root of a number is a value that, when multiplied by itself, gives the original number. It is denoted by the radical symbol \( \sqrt{} \). For example:
- \( \sqrt{16} = 4 \)
- \( \sqrt{25} = 5 \)
- \( \sqrt{\frac{9}{4}} = \frac{3}{2} \)
In general, if \( x^2 = y \), then \( x = \sqrt{y} \).
Properties and Relations
There are several important properties and relations between squares and square roots:
- For any positive number \( x \), \( (\sqrt{x})^2 = x \).
- The square root of a product can be split into the product of square roots: \( \sqrt{ab} = \sqrt{a} \cdot \sqrt{b} \) (for \( a \geq 0 \) and \( b \geq 0 \)).
- Square roots of negative numbers involve complex numbers, as \( \sqrt{-1} = i \), where \( i \) is the imaginary unit.
Examples and Applications
Squares and square roots are used in various applications, including geometry, physics, and finance. For instance, calculating the area of a square involves squaring the length of its side, while determining the standard deviation in statistics often involves taking the square root of the variance.
Table of Square Roots
| Number | Square | Square Root |
|---|---|---|
| 1 | 1 | 1 |
| 4 | 16 | 2 |
| 9 | 81 | 3 |
| 16 | 256 | 4 |
Understanding these concepts and their properties allows us to tackle a variety of mathematical problems with confidence and precision.
Methods to Calculate Square Roots
Calculating the square root of a number can be done using various methods. Below are some of the most common techniques used:
Repeated Subtraction Method
This method is straightforward but only applicable for perfect squares. It involves subtracting consecutive odd numbers from the original number until reaching zero. The number of subtractions performed gives the square root.
- Start with the number and subtract the smallest odd number, which is 1.
- Continue subtracting the next odd number (3, 5, 7, etc.) from the result.
- Repeat this process until the result is zero.
- The number of subtractions gives the square root.
Example: To find the square root of 81:
- 81 - 1 = 80
- 80 - 3 = 77
- 77 - 5 = 72
- 72 - 7 = 65
- 65 - 9 = 56
- 56 - 11 = 45
- 45 - 13 = 32
- 32 - 15 = 17
- 17 - 17 = 0
Since there were 9 subtractions, the square root of 81 is 9.
Prime Factorization Method
This method works well for perfect squares. It involves expressing the number as a product of prime factors and then taking the square root of these factors.
- Express the number as a product of its prime factors.
- Pair the prime factors.
- Take one factor from each pair and multiply them.
Example: To find the square root of 225:
- 225 = 3 × 3 × 5 × 5
- √225 = √(32 × 52) = 3 × 5 = 15
Long Division Method
This method is useful for both perfect and imperfect squares, especially for larger numbers. It involves a step-by-step division process.
- Group the digits of the number in pairs from right to left.
- Find the largest number whose square is less than or equal to the first group.
- Subtract the square of this number from the first group and bring down the next pair of digits.
- Double the divisor and find a digit to append to the quotient such that the new divisor, when multiplied by this digit, is less than or equal to the current dividend.
- Repeat the process until all pairs are used.
Example: To find the square root of 10:
- 10 is grouped as 10.000000.
- The first group is 10, and the largest number whose square is less than 10 is 3 (since 32 = 9).
- Subtract 9 from 10, get 1, and bring down the next pair to make 100.
- Double the divisor (3 becomes 6) and find the digit (1) to make 61 × 1 = 61.
- Continue the process to get more decimal places.
The square root of 10 up to three decimal places is approximately 3.162.

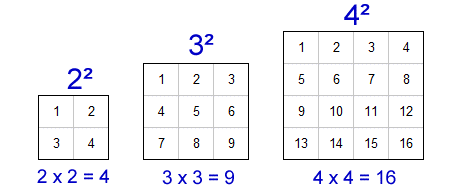
Square Roots of Perfect Squares
Square roots of perfect squares are integers. A perfect square is a number that can be expressed as the product of an integer with itself. For example, the perfect squares between 1 and 100 are:
- 1 (since \(1 \times 1 = 1\))
- 4 (since \(2 \times 2 = 4\))
- 9 (since \(3 \times 3 = 9\))
- 16 (since \(4 \times 4 = 16\))
- 25 (since \(5 \times 5 = 25\))
- 36 (since \(6 \times 6 = 36\))
- 49 (since \(7 \times 7 = 49\))
- 64 (since \(8 \times 8 = 64\))
- 81 (since \(9 \times 9 = 81\))
- 100 (since \(10 \times 10 = 100\))
Square roots of these numbers are the positive integers 1, 2, 3, 4, 5, 6, 7, 8, 9, and 10 respectively. These square roots are also called principal square roots.
To calculate the square root of a perfect square manually, we can use various methods such as prime factorization, long division, or using properties of exponents. For instance, to find the square root of 49, you can determine that it is \(7^2\), thus the square root of 49 is 7.
In mathematical notation, the square root of a perfect square \(n\) can be represented as \( \sqrt{n} \). If \(n = k^2\), where \(k\) is an integer, then \( \sqrt{n} = k \).
The concept of perfect squares and their roots is fundamental in various mathematical computations and problem-solving scenarios. Understanding this concept is crucial for higher-level mathematics including algebra, calculus, and number theory.
Square Roots of Non-Perfect Squares
Square roots of non-perfect squares are irrational numbers, meaning they cannot be expressed as a simple fraction. These square roots do not yield whole numbers and often require approximation methods for their determination. Here's a step-by-step guide on how to find the square roots of non-perfect squares:
1. Prime Factorization Method
This method involves expressing the number as a product of its prime factors and then simplifying the square root. However, for non-perfect squares, the prime factorization often results in irrational numbers, making it less practical for exact solutions.
2. Long Division Method
The long division method is a more precise approach to finding square roots of non-perfect squares. Here are the steps:
- Group the digits of the number in pairs, starting from the decimal point. If there are odd digits, the first group will be a single digit.
- Find the largest number whose square is less than or equal to the first group. This becomes the first digit of the root.
- Subtract the square of the first digit from the first group and bring down the next pair of digits.
- Double the first digit of the root and write it as the divisor. Find a digit to append to this divisor such that the product is less than or equal to the current dividend. This digit becomes the next digit of the root.
- Repeat the process until you reach the desired level of precision.
3. Estimation and Approximation Method
This method involves making an educated guess and refining it. Here's how:
- Estimate a rough value for the square root based on nearby perfect squares. For instance, to find the square root of 50, note that 49 (7²) and 64 (8²) are close, so the square root of 50 is between 7 and 8.
- Use a calculator or iterative methods to refine the guess. For example, take the average of 7 and 8, which is 7.5, and square it to get 56.25, which is too high. Then try 7.1 and so on, narrowing the range until you reach a satisfactory precision.
4. Using a Calculator
The simplest method for finding the square roots of non-perfect squares is using a calculator, which provides quick and accurate results. Most calculators have a square root function (√) that you can use directly.
Example Calculations
Here are some example calculations of square roots of non-perfect squares:
| Number | Square Root (Approx.) |
|---|---|
| 2 | 1.414 |
| 3 | 1.732 |
| 5 | 2.236 |
| 10 | 3.162 |
| 20 | 4.472 |
| 50 | 7.071 |
Understanding and calculating the square roots of non-perfect squares is crucial for various applications in mathematics, science, and engineering. These methods provide a foundation for approximating and working with irrational numbers effectively.
Square Root Function and Its Graph
The square root function is a fundamental mathematical function that is defined as:
\[ f(x) = \sqrt{x} \]
where \( f(x) \) represents the square root of \( x \). This function is only defined for non-negative values of \( x \) because the square root of a negative number involves imaginary numbers.
Graph of the Square Root Function
The graph of the square root function, \( f(x) = \sqrt{x} \), is a curve that starts at the origin (0,0) and increases slowly at first before rising more steeply as \( x \) increases. This shape occurs because the square root of larger numbers increases at a decreasing rate.
Below is a table of values for the square root function, which can be plotted to visualize the graph:
| x | f(x) = √x |
|---|---|
| 0 | 0 |
| 1 | 1 |
| 4 | 2 |
| 9 | 3 |
| 16 | 4 |
The graph can be visualized as follows:
As seen in the graph, the points with integer coordinates (such as (1,1), (4,2), (9,3)) are where \( x \) is a perfect square. These points become more spaced out as \( x \) increases because the square root function grows more slowly.
Properties of the Square Root Function
- Domain: The domain of the square root function is all non-negative real numbers \([0, \infty)\).
- Range: The range is also all non-negative real numbers \([0, \infty)\).
- Continuity: The square root function is continuous for all non-negative \( x \).
- Increasing Function: The function is monotonically increasing, meaning it never decreases as \( x \) increases.
The square root function is an important concept in mathematics, with applications in solving equations, geometry, and real-life scenarios where relationships between quantities are not linear.
Common Square Root Values
Square roots are values that, when multiplied by themselves, give the original number. Here are some common square root values:
| Number | Square Root |
|---|---|
| 1 | \(\sqrt{1} = 1\) |
| 4 | \(\sqrt{4} = 2\) |
| 9 | \(\sqrt{9} = 3\) |
| 16 | \(\sqrt{16} = 4\) |
| 25 | \(\sqrt{25} = 5\) |
| 36 | \(\sqrt{36} = 6\) |
| 49 | \(\sqrt{49} = 7\) |
| 64 | \(\sqrt{64} = 8\) |
| 81 | \(\sqrt{81} = 9\) |
| 100 | \(\sqrt{100} = 10\) |
For non-perfect squares, the square roots are irrational numbers. Here are some approximations:
- \(\sqrt{2} \approx 1.414\)
- \(\sqrt{3} \approx 1.732\)
- \(\sqrt{5} \approx 2.236\)
- \(\sqrt{6} \approx 2.449\)
- \(\sqrt{7} \approx 2.646\)
- \(\sqrt{8} \approx 2.828\)
- \(\sqrt{10} \approx 3.162\)
Square roots are essential in various mathematical contexts, including solving equations, geometry, and real-world applications. Understanding these common values helps in estimating and calculating more complex expressions.

Solving Equations Using Square Roots
Solving equations using square roots involves isolating the squared term and then applying the square root to both sides of the equation. This method is especially useful for solving quadratic equations that are not easily factorable. Below are the detailed steps for solving such equations.
Steps to Solve Quadratic Equations Using Square Roots
-
Isolate the quadratic term: Ensure that the term with the variable squared is by itself on one side of the equation. For example, in the equation \(x^2 = 25\), the term \(x^2\) is already isolated.
-
Apply the Square Root Property: Take the square root of both sides of the equation. Remember to include both the positive and negative roots. For \(x^2 = 25\), this step gives us:
\[
x = \pm \sqrt{25} \implies x = \pm 5
\] -
Simplify the radical: If the number under the square root is not a perfect square, simplify the radical as much as possible. For example, for \(x^2 = 7\), we get:
\[
x = \pm \sqrt{7}
\] -
Check the solutions: Substitute the solutions back into the original equation to verify their correctness. For \(x = \pm 5\), check:
\[
5^2 = 25 \quad \text{and} \quad (-5)^2 = 25
\]
Example Problems
Let's work through a couple of example problems to illustrate these steps:
Example 1: Solving a Simple Quadratic Equation
Solve \(x^2 = 49\).
- Isolate the quadratic term: \(x^2\) is already isolated.
- Apply the Square Root Property:
\[
x = \pm \sqrt{49} \implies x = \pm 7
\] - Simplify the radical: The square root of 49 is 7.
- Check the solutions:
\[
7^2 = 49 \quad \text{and} \quad (-7)^2 = 49
\]
Example 2: Solving a More Complex Equation
Solve \(3(x - 2)^2 = 27\).
- Isolate the quadratic term:
\[
(x - 2)^2 = 9
\] - Apply the Square Root Property:
\[
x - 2 = \pm \sqrt{9} \implies x - 2 = \pm 3
\] - Simplify the radical: The square root of 9 is 3.
- Solve for \(x\):
\[
x - 2 = 3 \implies x = 5 \quad \text{or} \quad x - 2 = -3 \implies x = -1
\] - Check the solutions:
\[
(5 - 2)^2 = 9 \implies 3(9) = 27 \quad \text{and} \quad (-1 - 2)^2 = 9 \implies 3(9) = 27
\]
Using these steps, you can solve a wide variety of quadratic equations efficiently. Always remember to include both the positive and negative roots when taking the square root of both sides of an equation.
Square Roots in Real Life
Square roots are fundamental in various real-life applications, making them an essential mathematical concept. Here are some common uses of square roots:
- Construction and Architecture: Square roots are used to calculate areas and dimensions in construction projects. For example, to find the length of a side of a square with a given area, you use the square root. This is critical for designing floor plans and ensuring accurate measurements.
- Physics: In physics, square roots are used to solve equations involving gravity, velocity, and energy. For instance, the time it takes for an object to fall to the ground can be determined using the square root of the height from which it is dropped, divided by a constant factor.
- Astronomy: Square roots help in calculating distances in space. When determining the luminosity of stars or the orbits of planets, astronomers use square roots to process large data sets accurately.
- Engineering: Engineers use square roots to determine stress and strain on materials, calculate electrical circuits, and analyze signal processing. Accurate calculations are crucial for designing safe and efficient structures and systems.
- Medicine: In medical research, square roots are used in statistical analysis to interpret data and determine the effectiveness of treatments. They are also used in calculating dosages for medications.
- Finance: Square roots are used in financial models to assess risks, calculate interest rates, and analyze market trends. For example, the standard deviation, a measure of market volatility, involves the square root of variance.
- Computer Graphics: In computer graphics, square roots are used to calculate distances between points in rendering images, animations, and video games. This is important for realistic rendering and smooth animations.
- Sports: Square roots are used in sports to analyze performance metrics and improve strategies. For example, calculating the average speed of an athlete over a distance involves square roots.
Overall, understanding and applying square roots is vital in many fields, enhancing problem-solving skills and enabling precise calculations in various professional and everyday scenarios.
FAQs about Square Roots
Here are some frequently asked questions about square roots:
-
What is the difference between square and square root?
Square: Multiplying a number by itself, expressed as \( n^2 \). For example, \( 3^2 = 9 \).
Square Root: Finding a number that, when multiplied by itself, gives the original number, expressed as \( \sqrt{n} \). For example, \( \sqrt{9} = 3 \). -
What is the vocabulary of square root?
- Square Root: The value that, when multiplied by itself, equals the original number. For example, the square root of 9 is 3.
- Radicand: The number under the square root symbol. For example, in \( \sqrt{9} \), 9 is the radicand.
- Radical Sign: The symbol \( \sqrt{} \) used to denote the square root.
- Principal Square Root: The non-negative square root of a number. For instance, the principal square root of 16 is 4.
- Perfect Square: A number that is the square of an integer. For example, 16 is a perfect square because it’s \( 4^2 \).
-
How to simplify square roots when the radicals are alike?We can add or subtract the numbers in square roots only when the values under the radical sign are equal. For example, to simplify \( \sqrt{192} \):
- Break down \( \sqrt{192} \) into \( \sqrt{2} \times \sqrt{96} \).
- Further breakdown \( \sqrt{96} \) into \( \sqrt{2} \times \sqrt{48} \).
- Continue breaking down until you get \( \sqrt{192} = \sqrt{(2 \times 2 \times 4 \times 4)} \times \sqrt{3} \).
- Finally, you get \( \sqrt{192} = 8\sqrt{3} \).
-
How is the square root of negative numbers calculated?To find the square root of a negative number, use imaginary numbers. For example, the square root of -16:
- Express -16 as \( 16 \times -1 \).
- Apply the square root to each factor separately: \( \sqrt{-16} = \sqrt{16} \times \sqrt{-1} \).
- Since \( \sqrt{16} = 4 \) and \( \sqrt{-1} = i \), the result is \( 4i \).
Video này giới thiệu về khái niệm căn bậc hai, cách tính toán và ứng dụng trong thực tế.
Căn Bậc Hai Là Gì? | Toán Học với Thầy J
READ MORE:
Video này giải thích khái niệm căn bậc hai, phương pháp tính và ứng dụng trong toán học cho trẻ em.
CĂN BẬC HAI 🚀 Căn Bậc Hai Là Gì? 👨🏻🚀 Toán Học Cho Trẻ Em