Topic square root of 48 in radical form: The square root of 48 in radical form is a fundamental concept in mathematics. This article provides a comprehensive guide to understanding and simplifying the square root of 48, offering step-by-step instructions, visual aids, and practical applications to make the process clear and accessible. Discover the elegance of radicals and enhance your math skills.
Table of Content
- Square Root of 48 in Radical Form
- Introduction
- Understanding Square Roots
- What is a Radical?
- Definition and Basics of Radical Expressions
- Prime Factorization Method
- Step-by-Step Simplification of Square Root of 48
- Detailed Prime Factorization of 48
- Simplifying Radicals with Perfect Squares
- Expressing the Square Root of 48 in Simplest Radical Form
- Mathematical Proof of the Simplification
- Visual Representation of the Simplification Process
- Applications of Simplified Radicals in Algebra
- Common Mistakes to Avoid
- Practice Problems and Solutions
- Further Reading and Resources
- YOUTUBE:
Square Root of 48 in Radical Form
The square root of 48 in radical form can be simplified by expressing 48 as a product of its prime factors.
Prime Factorization
First, we perform the prime factorization of 48:
- 48 = 2 × 24
- 24 = 2 × 12
- 12 = 2 × 6
- 6 = 2 × 3
Thus, 48 can be written as:
\[48 = 2^4 \times 3\]
Simplifying the Radical
To simplify the square root of 48, we take the square root of each prime factor:
\[\sqrt{48} = \sqrt{2^4 \times 3}\]
Since \(\sqrt{2^4} = 2^2 = 4\), we can simplify further:
\[\sqrt{48} = 4 \sqrt{3}\]
Conclusion
The simplified form of the square root of 48 in radical form is:
\[\sqrt{48} = 4\sqrt{3}\]

READ MORE:
Introduction
The square root of 48 in radical form is an important concept in mathematics, particularly in algebra and number theory. Simplifying square roots helps in solving equations and understanding mathematical relationships. This section will provide a detailed explanation of how to simplify the square root of 48 step-by-step.
To begin, let's understand the basics:
- The square root of a number \( x \) is a value \( y \) such that \( y^2 = x \).
- A radical expression involves roots, such as square roots, cube roots, etc.
- Simplifying a radical means expressing it in its simplest form.
Now, let's move to the prime factorization method to simplify \( \sqrt{48} \).
- First, perform the prime factorization of 48:
- 48 = 2 × 24
- 24 = 2 × 12
- 12 = 2 × 6
- 6 = 2 × 3
- Write 48 as a product of its prime factors:
\[48 = 2^4 \times 3\]
- Apply the square root to the prime factorized form:
\[\sqrt{48} = \sqrt{2^4 \times 3}\]
- Simplify by separating the perfect squares:
\[\sqrt{48} = \sqrt{2^4} \times \sqrt{3} = 4\sqrt{3}\]
Thus, the square root of 48 in radical form is simplified to \( 4\sqrt{3} \). This form is easier to work with and helps in various mathematical applications.
Understanding Square Roots
Square roots are fundamental in mathematics, representing a value that, when multiplied by itself, gives the original number. The square root of a number \( x \) is denoted as \( \sqrt{x} \). For instance, the square root of 9 is 3 because \( 3 \times 3 = 9 \).
Key concepts to understand square roots:
- Definition: If \( y \times y = x \), then \( y \) is the square root of \( x \), written as \( \sqrt{x} = y \).
- Properties of Square Roots:
- Square roots of perfect squares (like 1, 4, 9, 16) are integers.
- Square roots of non-perfect squares are irrational numbers, often left in radical form for simplicity.
- The square root function is the inverse of squaring a number.
- Positive and Negative Roots: Each positive number has two square roots: one positive and one negative. For example, both 3 and -3 are square roots of 9.
- Simplifying Square Roots: To simplify a square root, factor the number into its prime factors and pair the factors.
- Identify the prime factors: For example, \( 48 = 2^4 \times 3 \).
- Group the factors into pairs: \( 2^4 \) has two pairs of 2s.
- Take one factor from each pair out of the radical: \( \sqrt{2^4} = 2^2 = 4 \).
- Combine the simplified factors: \( \sqrt{48} = 4\sqrt{3} \).
Understanding these concepts will help you simplify square roots and apply them effectively in various mathematical problems.
What is a Radical?
A radical in mathematics is an expression that includes a root symbol, commonly known as the radical symbol (√). Radicals are used to denote roots of numbers and variables, with the most common radical being the square root.
Key points to understand radicals:
- Radical Symbol (√): This symbol is used to denote the root of a number. The expression under the root is called the radicand. For example, in \( \sqrt{48} \), 48 is the radicand.
- Types of Radicals: Radicals are categorized based on the type of root:
- Square Roots: The most common type, denoted as \( \sqrt{x} \) or \( x^{1/2} \).
- Cube Roots: Denoted as \( \sqrt[3]{x} \) or \( x^{1/3} \), representing a number that, when cubed, gives the original number.
- Higher-order Roots: Includes fourth roots, fifth roots, etc., denoted as \( \sqrt[n]{x} \) or \( x^{1/n} \).
- Simplifying Radicals: The process of breaking down a radical into its simplest form by factoring the radicand and extracting squares, cubes, etc.
- Factor the radicand into its prime factors: For example, \( 48 = 2^4 \times 3 \).
- Group the factors into pairs (or triplets for cube roots): For \( \sqrt{48} \), group \( 2^4 \) into pairs of 2s.
- Move each pair out of the radical as a single factor: \( \sqrt{2^4} = 2^2 = 4 \).
- Combine the simplified factors: \( \sqrt{48} = 4\sqrt{3} \).
- Radicals and Exponents: Radicals can be expressed using fractional exponents. For instance, \( \sqrt{x} = x^{1/2} \) and \( \sqrt[3]{x} = x^{1/3} \).
Understanding radicals and their properties is essential for solving equations and simplifying expressions in algebra and beyond.
Definition and Basics of Radical Expressions
Radical expressions are mathematical expressions that include a radical symbol (√) which represents the root of a number. These expressions are fundamental in algebra and are used to solve various equations and simplify complex expressions.
Key concepts of radical expressions include:
- Radicand: The number or expression inside the radical symbol. For example, in \( \sqrt{48} \), 48 is the radicand.
- Index: The small number written just outside and above the radical symbol indicating the degree of the root. For square roots, the index is 2 (usually not written), and for cube roots, it is 3. For example, \( \sqrt[3]{x} \) indicates a cube root.
Steps to simplify radical expressions:
- Identify the radicand and factor it into its prime factors. For example, for \( \sqrt{48} \):
- 48 can be factored as \( 2 \times 24 \)
- 24 can be factored as \( 2 \times 12 \)
- 12 can be factored as \( 2 \times 6 \)
- 6 can be factored as \( 2 \times 3 \)
- Write the radicand as a product of its prime factors:
\[48 = 2^4 \times 3\]
- Simplify the expression by grouping the factors based on the index of the radical. For square roots, group the factors into pairs:
\[\sqrt{48} = \sqrt{2^4 \times 3} = \sqrt{(2^2)^2 \times 3}\]
- Take out one factor from each pair:
\[\sqrt{(2^2)^2 \times 3} = 2^2 \sqrt{3} = 4\sqrt{3}\]
Thus, the simplified form of \( \sqrt{48} \) is \( 4\sqrt{3} \).
Properties of radical expressions:
- Product Property: The square root of a product is the product of the square roots:
\[\sqrt{ab} = \sqrt{a} \times \sqrt{b}\]
- Quotient Property: The square root of a quotient is the quotient of the square roots:
\[\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\]
- Simplifying Higher-order Roots: The same principles apply to cube roots and other higher-order roots, with appropriate grouping based on the index.
Understanding and simplifying radical expressions are crucial for solving algebraic equations and performing mathematical operations efficiently.
Prime Factorization Method
The prime factorization method is a systematic approach to simplify the square root of a number by breaking it down into its prime factors. This method is particularly useful for simplifying radicals and understanding the composition of numbers.
Here’s a step-by-step guide to using the prime factorization method to simplify the square root of 48:
- Find the prime factors of 48:
Start by dividing 48 by the smallest prime number, which is 2, and continue dividing the quotient by 2 until it is no longer divisible by 2. Then, proceed with the next smallest prime number, 3.
- 48 ÷ 2 = 24
- 24 ÷ 2 = 12
- 12 ÷ 2 = 6
- 6 ÷ 2 = 3
- 3 ÷ 3 = 1
So, the prime factorization of 48 is:
\[48 = 2^4 \times 3\]
- Rewrite the square root of 48 using its prime factors:
\[\sqrt{48} = \sqrt{2^4 \times 3}\]
- Separate the factors into perfect squares and non-perfect squares:
\[\sqrt{48} = \sqrt{2^4} \times \sqrt{3}\]
- Simplify the square roots of the perfect squares:
\[\sqrt{2^4} = 2^2 = 4\]
- Combine the simplified factors:
\[\sqrt{48} = 4\sqrt{3}\]
Using the prime factorization method, we have simplified the square root of 48 to \(4\sqrt{3}\). This method is effective because it leverages the properties of prime numbers and perfect squares to break down complex radicals into simpler forms.
The prime factorization method can be applied to any number to simplify its square root, making it a versatile tool in algebra and number theory.
Step-by-Step Simplification of Square Root of 48
Simplifying the square root of 48 involves breaking down the number into its prime factors and simplifying the radical expression step-by-step. Here's a detailed guide:
- Identify the Prime Factors of 48:
Start by factoring 48 into its prime factors.
- 48 ÷ 2 = 24
- 24 ÷ 2 = 12
- 12 ÷ 2 = 6
- 6 ÷ 2 = 3
- 3 ÷ 3 = 1
Thus, the prime factorization of 48 is \( 2^4 \times 3 \).
- Rewrite the Square Root Using Prime Factors:
Express the square root of 48 in terms of its prime factors:
\[\sqrt{48} = \sqrt{2^4 \times 3}\]
- Separate Perfect Squares and Non-Perfect Squares:
Identify the perfect squares within the prime factors:
\[\sqrt{2^4 \times 3} = \sqrt{(2^2)^2 \times 3} = \sqrt{2^4} \times \sqrt{3}\]
- Simplify the Perfect Squares:
Take the square root of the perfect squares:
\[\sqrt{2^4} = 2^2 = 4\]
- Combine the Simplified Factors:
Multiply the simplified square root of the perfect squares by the remaining radical:
\[\sqrt{48} = 4\sqrt{3}\]
Thus, the simplified form of the square root of 48 is \( 4\sqrt{3} \). This step-by-step process helps break down the radical into manageable parts, making it easier to understand and simplify.
Let's summarize the simplification process:
- Prime factorization of 48: \( 2^4 \times 3 \)
- Rewrite under the square root: \( \sqrt{2^4 \times 3} \)
- Identify and separate perfect squares: \( \sqrt{(2^2)^2 \times 3} \)
- Simplify perfect squares: \( 2^2 = 4 \)
- Combine the factors: \( 4\sqrt{3} \)
By following these steps, you can simplify the square root of any number efficiently.
Detailed Prime Factorization of 48
The prime factorization of a number involves breaking it down into the product of its prime numbers. Here is a detailed step-by-step process for the prime factorization of 48:
- Start with the smallest prime number, which is 2:
Divide 48 by 2:
\[ 48 \div 2 = 24 \]
- Continue dividing by 2:
Divide 24 by 2:
\[ 24 \div 2 = 12 \]
Divide 12 by 2:
\[ 12 \div 2 = 6 \]
Divide 6 by 2:
\[ 6 \div 2 = 3 \]
- Move to the next smallest prime number, which is 3:
Divide 3 by 3:
\[ 3 \div 3 = 1 \]
At this point, we have completely factored 48 into its prime factors. The prime factorization of 48 is:
\[ 48 = 2 \times 2 \times 2 \times 2 \times 3 = 2^4 \times 3 \]
We can represent this as a table for clarity:
| Step | Result |
|---|---|
| 48 ÷ 2 | 24 |
| 24 ÷ 2 | 12 |
| 12 ÷ 2 | 6 |
| 6 ÷ 2 | 3 |
| 3 ÷ 3 | 1 |
Thus, the prime factors of 48 are \(2^4\) and \(3\).
These prime factors are useful for simplifying the square root of 48 and other mathematical operations involving the number 48.
Simplifying Radicals with Perfect Squares
Simplifying radicals involves expressing the square root of a number in its simplest form. To simplify the square root of 48 using perfect squares, follow these steps:
-
First, identify the factors of 48. The factors are:
- 1, 2, 3, 4, 6, 8, 12, 16, 24, 48
-
Next, find the perfect squares among these factors. Perfect squares are numbers that can be expressed as the square of an integer. The perfect squares from the list of factors are:
- 1, 4, 16
-
Divide 48 by the largest perfect square found in the previous step. In this case:
\( \frac{48}{16} = 3 \)
-
Calculate the square root of the largest perfect square:
\( \sqrt{16} = 4 \)
-
Combine the results of steps 3 and 4 to express the square root of 48 in its simplest form:
\( \sqrt{48} = \sqrt{16 \times 3} = \sqrt{16} \times \sqrt{3} = 4\sqrt{3} \)
Thus, the square root of 48 simplified is \( 4\sqrt{3} \). This method leverages the property that the square root of a product is the product of the square roots, allowing us to simplify the expression by extracting the square root of the largest perfect square factor.

Expressing the Square Root of 48 in Simplest Radical Form
To express the square root of 48 in its simplest radical form, we need to simplify the expression by identifying and extracting perfect square factors. Here is a detailed step-by-step guide:
-
Start with the given expression:
\[\sqrt{48}\]
-
Factorize 48 into its prime factors:
\[48 = 2 \times 2 \times 2 \times 2 \times 3 = 2^4 \times 3\]
-
Group the prime factors into pairs of perfect squares:
\[48 = (2^2 \times 2^2) \times 3 = 4 \times 4 \times 3\]
-
Rewrite the expression under the square root using these pairs:
\[\sqrt{48} = \sqrt{(2^2) \times (2^2) \times 3} = \sqrt{4 \times 4 \times 3}\]
-
Extract the perfect square factors from the square root:
\[\sqrt{4 \times 4 \times 3} = 4\sqrt{3}\]
Thus, the square root of 48 in its simplest radical form is:
\[4\sqrt{3}\]
To further illustrate this, consider the following table showing the simplification process:
| Step | Expression |
|---|---|
| 1 | \(\sqrt{48}\) |
| 2 | \(48 = 2 \times 2 \times 2 \times 2 \times 3 = 2^4 \times 3\) |
| 3 | \(48 = (2^2 \times 2^2) \times 3\) |
| 4 | \(\sqrt{48} = \sqrt{(2^2) \times (2^2) \times 3}\) |
| 5 | \(\sqrt{48} = 4\sqrt{3}\) |
This simplification method ensures that the expression is in its simplest radical form, making it easier to work with in algebraic equations and other mathematical applications.
Mathematical Proof of the Simplification
To mathematically prove the simplification of the square root of 48, we will use the prime factorization method and the properties of square roots. Let's proceed step-by-step:
-
Prime Factorization of 48:
First, we find the prime factors of 48.
- 48 ÷ 2 = 24
- 24 ÷ 2 = 12
- 12 ÷ 2 = 6
- 6 ÷ 2 = 3
- 3 ÷ 3 = 1
Thus, the prime factorization of 48 is: \(48 = 2^4 \times 3^1\)
-
Expressing the Square Root:
Using the prime factorization, we express the square root of 48:
\[\sqrt{48} = \sqrt{2^4 \times 3}\]
-
Applying the Properties of Square Roots:
We apply the property \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\) to separate the factors:
\[\sqrt{48} = \sqrt{2^4} \times \sqrt{3}\]
We know that \(\sqrt{2^4} = 2^2 = 4\), so we simplify further:
\[\sqrt{48} = 4 \times \sqrt{3}\]
-
Conclusion:
Therefore, the simplified radical form of the square root of 48 is:
\[\sqrt{48} = 4\sqrt{3}\]
This proof shows that by breaking down 48 into its prime factors and applying the properties of square roots, we can simplify \(\sqrt{48}\) to \(4\sqrt{3}\).
Visual Representation of the Simplification Process
To visually represent the simplification of the square root of 48, we will use the process of prime factorization and illustrate each step clearly.
- First, we perform the prime factorization of 48:
- 48 can be divided by 2: \(48 \div 2 = 24\)
- 24 can be divided by 2: \(24 \div 2 = 12\)
- 12 can be divided by 2: \(12 \div 2 = 6\)
- 6 can be divided by 2: \(6 \div 2 = 3\)
- 3 is a prime number.
Thus, the prime factors of 48 are \(2 \times 2 \times 2 \times 2 \times 3\), or \(2^4 \times 3\).
- Next, we express the square root of 48 using these prime factors:
\(\sqrt{48} = \sqrt{2^4 \times 3}\)
- We can simplify this by taking the square root of each factor:
\(\sqrt{2^4 \times 3} = \sqrt{2^4} \times \sqrt{3}\)
\(\sqrt{2^4} = \sqrt{(2^2)^2} = 2^2 = 4\)
Therefore, \(\sqrt{2^4 \times 3} = 4 \times \sqrt{3}\)
- Thus, the simplified form of \(\sqrt{48}\) is \(4\sqrt{3}\).
Here is a visual representation of this process:
| Step | Operation | Result |
| Prime Factorization | 48 = \(2 \times 2 \times 2 \times 2 \times 3\) | \(2^4 \times 3\) |
| Square Root | \(\sqrt{48} = \sqrt{2^4 \times 3}\) | \(\sqrt{2^4} \times \sqrt{3}\) |
| Simplify | \(\sqrt{2^4} = 4\) | \(4 \times \sqrt{3}\) |
| Result | \(4\sqrt{3}\) |
This detailed breakdown helps in understanding how to simplify radicals step by step using prime factorization.
Applications of Simplified Radicals in Algebra
In algebra, simplified radicals play a crucial role in various applications. Understanding how to work with radicals can make solving equations and simplifying expressions much more manageable. Here are some key applications of simplified radicals:
- Solving Quadratic Equations: Simplified radicals often appear when solving quadratic equations using the quadratic formula. For example, consider the equation \(ax^2 + bx + c = 0\). The solutions are given by: \[ x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a} \] Simplifying the radical expression \(\sqrt{b^2 - 4ac}\) is essential for finding the roots of the equation.
- Geometry and Trigonometry: Radicals are frequently used in geometry to express the lengths of sides of right triangles (e.g., the Pythagorean theorem) and in trigonometry for finding exact values of trigonometric functions. For instance, in a 45°-45°-90° triangle, the hypotenuse is \( \sqrt{2} \) times the length of each leg.
- Simplifying Algebraic Expressions: Simplified radicals help in reducing the complexity of algebraic expressions, making them easier to manipulate. For example, simplifying \(\sqrt{48}\) to \(4\sqrt{3}\) can be useful in various algebraic operations, such as adding or subtracting radical expressions.
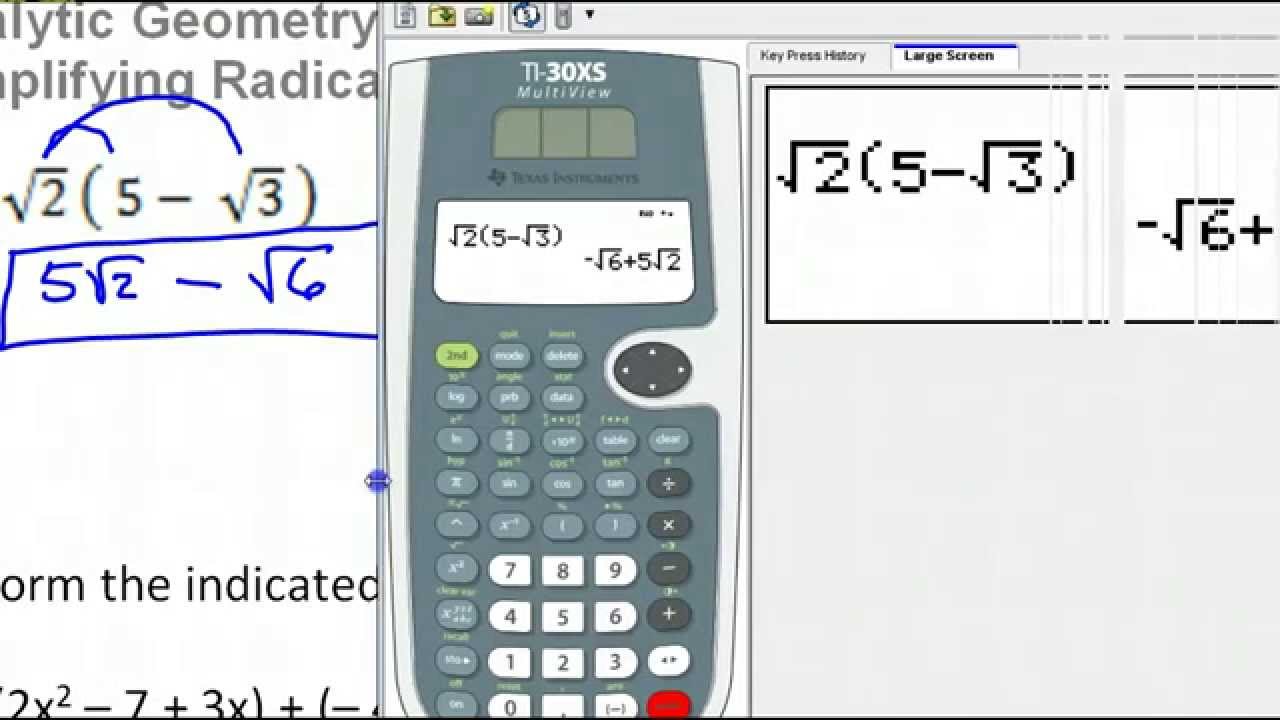
- Rationalizing Denominators: Simplifying radicals is crucial for rationalizing the denominators of fractions. For instance, to rationalize \(\frac{1}{\sqrt{3}}\), you multiply the numerator and the denominator by \(\sqrt{3}\), resulting in: \[ \frac{1}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{\sqrt{3}}{3} \]
- Exponential Functions: Radicals appear in exponential functions, and simplifying these expressions is key to solving exponential equations. For example, simplifying \( \sqrt{x^2} \) to \( |x| \) is necessary for correctly evaluating the function.
Let's look at a few specific examples to illustrate the applications of simplified radicals in algebra:
- Example 1: Solving a Quadratic Equation
Consider the quadratic equation \(2x^2 - 8x + 6 = 0\). Using the quadratic formula, we have:
\[ x = \frac{-(-8) \pm \sqrt{(-8)^2 - 4 \cdot 2 \cdot 6}}{2 \cdot 2} = \frac{8 \pm \sqrt{64 - 48}}{4} = \frac{8 \pm \sqrt{16}}{4} = \frac{8 \pm 4}{4} \]This gives the solutions \(x = 3\) and \(x = 1\).
- Example 2: Simplifying an Algebraic Expression
Simplify the expression \(\sqrt{75} + \sqrt{27}\). First, simplify each radical:
\[ \sqrt{75} = \sqrt{25 \times 3} = 5\sqrt{3}, \quad \sqrt{27} = \sqrt{9 \times 3} = 3\sqrt{3} \]Therefore, \(\sqrt{75} + \sqrt{27} = 5\sqrt{3} + 3\sqrt{3} = 8\sqrt{3}\).
- Example 3: Rationalizing the Denominator
Rationalize the denominator of \(\frac{5}{\sqrt{2}}\). Multiply the numerator and the denominator by \(\sqrt{2}\):
\[ \frac{5}{\sqrt{2}} \times \frac{\sqrt{2}}{\sqrt{2}} = \frac{5\sqrt{2}}{2} \]
These examples highlight the importance of understanding and applying simplified radicals in algebra to streamline complex expressions and solve equations effectively.

Common Mistakes to Avoid
When simplifying radicals, particularly the square root of 48, there are several common mistakes that students often make. Understanding and avoiding these errors can help ensure accuracy and efficiency in calculations.
- Incorrect Factorization: One of the most common mistakes is not correctly factorizing the number under the radical. For instance, 48 should be factorized as \(2^4 \times 3\), not \(24 \times 2\) or any other incorrect combination.
- Ignoring Perfect Squares: Failing to recognize and simplify perfect squares under the radical can lead to incorrect results. For example, in \(\sqrt{48} = \sqrt{16 \times 3}\), recognizing that 16 is a perfect square is crucial.
- Incorrect Simplification Steps: When simplifying, each step must be performed accurately. For example, \(\sqrt{48} = \sqrt{16 \times 3} = \sqrt{16} \times \sqrt{3} = 4\sqrt{3}\). Skipping steps or performing them out of order can lead to errors.
- Improper Handling of Irrational Numbers: Remember that the square root of a non-perfect square (like 3 in \(\sqrt{3}\)) remains an irrational number. Attempting to further simplify \(\sqrt{3}\) in \(\sqrt{48} = 4\sqrt{3}\) is incorrect.
- Sign Errors: Ensure that all steps maintain the correct signs. While dealing with square roots, always consider both the positive and negative roots unless the context specifies otherwise.
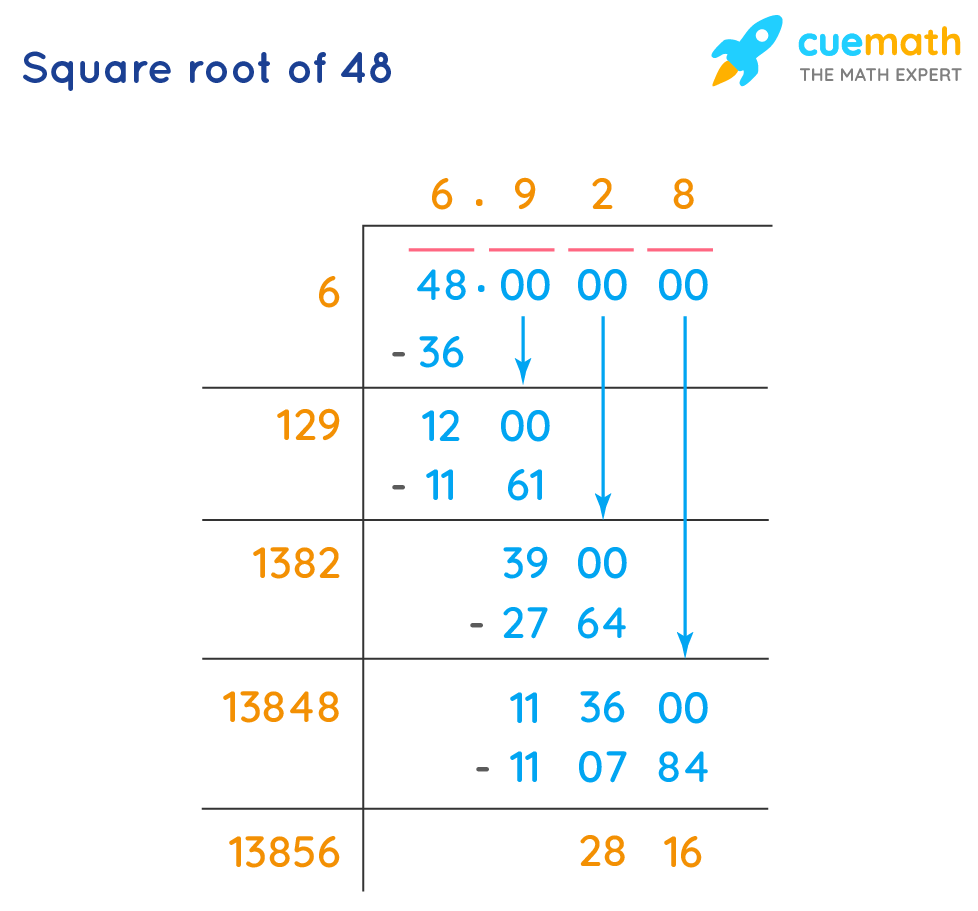
- Mistaking Approximation for Exact Form: Do not confuse the decimal approximation of the square root with its exact radical form. For instance, \(\sqrt{48} \approx 6.928\) is an approximation, while \(4\sqrt{3}\) is the exact form.
By being aware of these common pitfalls, students can avoid errors and improve their skills in simplifying radicals.
Practice Problems and Solutions
Practicing with various problems helps in understanding the concept of simplifying radicals better. Here are a few practice problems along with their step-by-step solutions:
-
Simplify the square root of 72.
- Prime factorize 72: \( 72 = 2^3 \times 3^2 \).
- Group the prime factors: \( \sqrt{72} = \sqrt{2^3 \times 3^2} \).
- Rewrite in terms of pairs: \( \sqrt{72} = \sqrt{(2^2 \times 3^2) \times 2} \).
- Simplify: \( \sqrt{72} = 2 \times 3 \times \sqrt{2} = 6\sqrt{2} \).
-
Simplify the square root of 98.
- Prime factorize 98: \( 98 = 2 \times 7^2 \).
- Group the prime factors: \( \sqrt{98} = \sqrt{2 \times 7^2} \).
- Rewrite in terms of pairs: \( \sqrt{98} = \sqrt{(7^2) \times 2} \).
- Simplify: \( \sqrt{98} = 7\sqrt{2} \).
-
Simplify the square root of 200.
- Prime factorize 200: \( 200 = 2^3 \times 5^2 \).
- Group the prime factors: \( \sqrt{200} = \sqrt{2^3 \times 5^2} \).
- Rewrite in terms of pairs: \( \sqrt{200} = \sqrt{(2^2 \times 5^2) \times 2} \).
- Simplify: \( \sqrt{200} = 2 \times 5 \times \sqrt{2} = 10\sqrt{2} \).
-
Simplify the square root of 75.
- Prime factorize 75: \( 75 = 3 \times 5^2 \).
- Group the prime factors: \( \sqrt{75} = \sqrt{3 \times 5^2} \).
- Rewrite in terms of pairs: \( \sqrt{75} = \sqrt{(5^2) \times 3} \).
- Simplify: \( \sqrt{75} = 5\sqrt{3} \).
By practicing these types of problems, one can become more proficient in simplifying radicals and understanding their properties.
Further Reading and Resources
For those looking to deepen their understanding of square roots and radical expressions, here are some valuable resources and further reading materials:
-
Khan Academy - Intro to Square Roots:
An excellent starting point for beginners, this resource provides video tutorials and practice problems to help you grasp the basics of square roots and their applications.
-
Mathematics LibreTexts - Roots and Radicals:
This comprehensive guide covers various types of roots, including square and cube roots, and provides detailed explanations on simplifying radicals. It's a great resource for both high school and college students.
-
Math is Fun - Simplifying Square Roots:
This website offers a user-friendly approach to understanding and simplifying square roots, with step-by-step guides and interactive tools to help you practice.
-
Paul's Online Math Notes - Radicals and Rational Exponents:
Paul's notes are well-known for their clarity and thoroughness. This section on radicals and rational exponents is particularly useful for those needing a deeper dive into the subject matter.
These resources should provide a solid foundation and further enhance your understanding of square roots and radical expressions.
Cách Đơn Giản Hóa Căn Bậc Hai Của 48: Căn Bậc Hai (48)
READ MORE:
Học Cách Đơn Giản Hóa Căn Bậc Hai Của 48