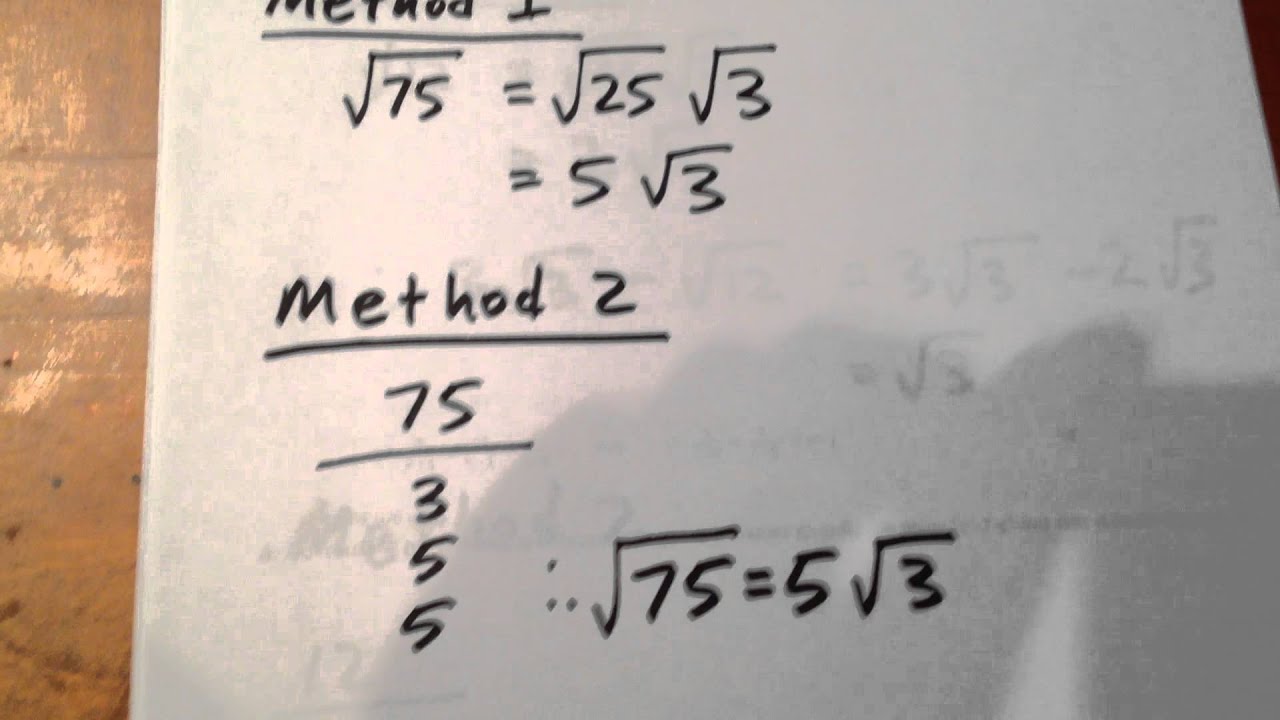Simplify Square Root 175: Easy Step-by-Step Guide

To simplify the square root of 175, we need to express it in its simplest radical form.

Step-by-Step Process

1. Identify the prime factors of 175.
2. Express 175 as a product of its prime factors.
3. Pair the prime factors to simplify the radical.

Prime Factorization

The prime factorization of 175 is:

\( 175 = 5 \times 5 \times 7 \)

Simplifying the Radical

To simplify \( \sqrt{175} \), we look for pairs of prime factors:

\( \sqrt{175} = \sqrt{5 \times 5 \times 7} \)

Since \( 5 \times 5 \) is a perfect square, we can simplify this to:

\( \sqrt{175} = \sqrt{5^2 \times 7} = 5\sqrt{7} \)

Conclusion

The simplified form of \( \sqrt{175} \) is:

\( \sqrt{175} = 5\sqrt{7} \)

This is the most simplified radical form of the square root of 175.

The square root of a number is a value that, when multiplied by itself, gives the original number. Simplifying square roots involves expressing the square root in its simplest radical form. This process is particularly useful in mathematics to make calculations easier and results more understandable.

In this guide, we will focus on simplifying the square root of 175. By breaking it down into its prime factors, we can simplify it step by step.

Before we dive into the simplification process, let's briefly review the concept of square roots and why simplification is important:

• Understanding Square Roots: The square root symbol is √, and it denotes the principal square root, which is always positive. For example, √4 = 2.
• Importance of Simplification: Simplifying square roots helps in solving equations, performing calculations, and understanding the underlying structure of numbers.

With this foundation, let's move on to the prime factorization method, which is crucial for simplifying square roots effectively.

Square roots are mathematical operations that determine which number, when multiplied by itself, yields the original number. The square root of a number \(x\) is denoted as \(\sqrt{x}\).

For example, \(\sqrt{25} = 5\) because \(5 \times 5 = 25\). This shows that 25 is a perfect square because its square root is an integer.

However, not all numbers are perfect squares. For instance, 175 is not a perfect square, meaning its square root is not an integer. Instead, we simplify it using prime factorization.

Steps to Simplify the Square Root of 175

1. Factorize 175 into its prime factors: \(175 = 5^2 \times 7\).
2. Rewrite the square root of the product as the product of square roots: \(\sqrt{175} = \sqrt{5^2 \times 7}\).
3. Take the square root of the perfect square (5²): \(\sqrt{5^2} = 5\).
4. Combine the results: \(5\sqrt{7}\).

Thus, the simplified form of \(\sqrt{175}\) is \(5\sqrt{7}\).

In decimal form, this is approximately \(13.228\), which can be useful for practical applications where an exact square root is not required.

Understanding square roots and their simplification helps in various fields such as geometry, algebra, and real-world applications where measurement and scaling are involved.

The prime factorization method is a useful technique for simplifying square roots. It involves breaking down the number under the square root into its prime factors. Here's a step-by-step guide to using this method for the square root of 175:

1. List the prime factors of 175:

   
   The prime factors of 175 are 5 and 7, because 175 can be written as \(5^2 \times 7\).

2. Group the factors in pairs:

   
   From the prime factorization \(5^2 \times 7\), we can see that 5 is a perfect square.

3. Rewrite the square root using these groups:

   
   The square root of 175 can be rewritten as:
   \[
   \sqrt{175} = \sqrt{5^2 \times 7} = \sqrt{5^2} \times \sqrt{7}
   \]

4. Simplify the square roots:

   
   The square root of \(5^2\) is 5, and the square root of 7 remains under the radical because it is not a perfect square. Therefore:
   \[
   \sqrt{175} = 5 \sqrt{7}
   \]

This simplified form, \(5 \sqrt{7}\), is the simplest radical form of the square root of 175. Using the prime factorization method helps in breaking down the number systematically, ensuring accuracy and clarity in simplification.

The process of simplifying the square root of 175 involves a few straightforward steps. Here, we break it down step by step:

1. Factor 175 into its prime factors:

   We start by expressing 175 as a product of its prime factors:

   \[ 175 = 5^2 \times 7 \]

2. Rewrite the square root:

   Next, we rewrite the square root of 175 using the prime factors:

   \[ \sqrt{175} = \sqrt{5^2 \times 7} \]

3. Simplify the expression:

   Since the square root of a product is the product of the square roots, we can separate the terms under the radical sign:

   \[ \sqrt{5^2 \times 7} = \sqrt{5^2} \times \sqrt{7} \]

   Then, simplify the square root of 25:

   \[ \sqrt{5^2} = 5 \]

4. Combine the simplified terms:

   We multiply the simplified square root of 25 by the remaining square root:

   \[ 5 \times \sqrt{7} \]

Therefore, the simplified form of the square root of 175 is:

\[ \sqrt{175} = 5 \sqrt{7} \]

This step-by-step method ensures that we break down the process logically and understand each part of the simplification.

Here are a few examples to illustrate the process of simplifying square roots. Each example demonstrates the method step-by-step to ensure clarity and understanding.

Example 1: Simplifying √175

1. Identify the prime factors of 175:

   \(175 = 5^2 \times 7\)

2. Rewrite the square root using these factors:

   \(\sqrt{175} = \sqrt{5^2 \times 7}\)

3. Simplify by taking the square root of the perfect square factor:

   \(\sqrt{5^2} = 5\)

   \(\sqrt{175} = 5\sqrt{7}\)

Example 2: Simplifying √50

1. Identify the prime factors of 50:

   \(50 = 2 \times 5^2\)

2. Rewrite the square root using these factors:

   \(\sqrt{50} = \sqrt{2 \times 5^2}\)

3. Simplify by taking the square root of the perfect square factor:

   \(\sqrt{5^2} = 5\)

   \(\sqrt{50} = 5\sqrt{2}\)

Example 3: Simplifying √72

1. Identify the prime factors of 72:

   \(72 = 2^3 \times 3^2\)

2. Rewrite the square root using these factors:

   \(\sqrt{72} = \sqrt{2^3 \times 3^2}\)

3. Simplify by taking the square root of the perfect square factor:

   \(\sqrt{3^2} = 3\)

   \(\sqrt{72} = 3\sqrt{2^3} = 3\sqrt{8}\)

4. Further simplify if possible:

   \(\sqrt{8} = \sqrt{4 \times 2} = 2\sqrt{2}\)

   \(\sqrt{72} = 3 \times 2\sqrt{2} = 6\sqrt{2}\)

Example 4: Simplifying √32

1. Identify the prime factors of 32:

   \(32 = 2^5\)

2. Rewrite the square root using these factors:

   \(\sqrt{32} = \sqrt{2^5}\)

3. Simplify by taking the square root of the perfect square factor:

   \(\sqrt{2^4} = 4\)

   \(\sqrt{32} = 4\sqrt{2}\)

Example 5: Simplifying √98

1. Identify the prime factors of 98:

   \(98 = 2 \times 7^2\)

2. Rewrite the square root using these factors:

   \(\sqrt{98} = \sqrt{2 \times 7^2}\)

3. Simplify by taking the square root of the perfect square factor:

   \(\sqrt{7^2} = 7\)

   \(\sqrt{98} = 7\sqrt{2}\)

When simplifying square roots, it's easy to make mistakes that can lead to incorrect results. Here are some common errors to watch out for and how to avoid them:

• Forgetting to Check for Perfect Squares:

  One of the most common mistakes is not checking if the number under the square root is a perfect square. Always identify and extract perfect squares from the radicand to simplify the expression correctly.

  Example: \(\sqrt{175}\) can be broken down into \(\sqrt{25 \times 7} = \sqrt{25} \times \sqrt{7} = 5\sqrt{7}\).

• Incorrect Prime Factorization:

  Accurate prime factorization is crucial. Misidentifying prime factors can lead to incorrect simplification. Ensure to break down the number completely into its prime factors.

  Example: Prime factors of 175 are 5, 5, and 7. So, \(\sqrt{175} = \sqrt{5 \times 5 \times 7} = 5\sqrt{7}\).

• Rushing Through the Process:

  Take your time with each step of the simplification. Rushing can lead to missed steps or errors in calculation. Approach each part methodically to ensure accuracy.

  Strategy: Write down each step clearly and verify before moving on to the next.

• Neglecting to Simplify Completely:

  Sometimes, simplification can be left incomplete. Always double-check that the expression is in its simplest form before concluding.

  Example: Simplify \(\sqrt{75}\) correctly as \(\sqrt{25 \times 3} = 5\sqrt{3}\), not stopping at \(\sqrt{75}\).

• Misunderstanding Radicals and Exponents:

  Ensure a solid understanding of the properties of radicals and exponents. Misapplying these properties can lead to errors.

  Tip: Remember that \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\) and use this property correctly.

By being aware of these common mistakes and taking a careful, step-by-step approach, you can simplify square roots accurately and efficiently.

The simplification of square roots, such as \( \sqrt{175} \), has practical applications across various fields. Understanding these applications can help in solving real-world problems effectively. Here are some common applications:

• Geometry and Trigonometry:

  Square roots are often used in geometric calculations. For instance, finding the side length of a square when the area is known involves taking the square root of the area. Similarly, in trigonometry, the Pythagorean theorem uses square roots to determine the lengths of sides in right-angled triangles.

• Engineering and Physics:

  In engineering, square roots are used in formulas to calculate stress, strain, and other physical properties. For example, when dealing with wave equations, square roots can be used to determine the amplitude and energy of waves.

• Statistics:

  Square roots play a crucial role in statistics, particularly in the calculation of standard deviation and variance. These measures are essential for understanding the spread and distribution of data in a dataset.

• Finance:

  In finance, square roots are used to calculate the volatility of stock prices and the standard deviation of returns on investments. These calculations help in assessing risk and making informed investment decisions.

• Computer Science:

  Square root algorithms are fundamental in computer science for various computational tasks. For instance, the square root function is used in graphics to calculate distances between points in space, essential for rendering and simulation.

• Astronomy:

  In astronomy, square roots are used to compute the luminosity of stars and the distances between celestial objects. The inverse-square law, which describes how light intensity diminishes with distance, involves square root calculations.

These applications illustrate the importance of understanding and simplifying square roots like \( \sqrt{175} = 5\sqrt{7} \) in various disciplines, making complex calculations more manageable and accurate.

For further understanding and practice on simplifying square roots, including the square root of 175, the following resources are highly recommended:

• This site provides step-by-step instructions on how to simplify the square root of 175, along with other algebraic problems.

• Utilize this tool to input various math problems and get detailed solutions, including the simplification of square roots.

• This educational resource explains the process of simplifying square roots using clear examples and rules.

• Learn about the decimal and simplified radical forms of the square root of 175, and use the online calculator for additional practice.

• This site offers a detailed explanation of simplifying the square root of 175 using both prime factorization and the long division method.