Topic simplify square root 200: Unlock the secrets to simplifying the square root of 200 with our easy, step-by-step guide. Discover the methods to break down and simplify complex square roots, making math simpler and more understandable. Perfect for students, educators, and math enthusiasts looking to enhance their skills and confidence in mathematics.
Table of Content
- Simplifying the Square Root of 200
- Introduction to Square Roots
- Understanding Square Roots
- Basic Properties of Square Roots
- Prime Factorization
- Steps to Simplify the Square Root of 200
- Finding Prime Factors of 200
- Grouping Prime Factors
- Applying Square Root Properties
- Simplified Form of Square Root of 200
- Example Problems
- Practice Problems
- Common Mistakes to Avoid
- Conclusion
- Further Reading and Resources
- YOUTUBE:
Simplifying the Square Root of 200
To simplify the square root of 200, follow these steps:
Step-by-Step Solution:
- Factorize the number 200.
- Find pairs of factors.
- Simplify the expression using the properties of square roots.
Factorization:
The prime factorization of 200 is:
\[ 200 = 2^3 \times 5^2 \]
Pairing Factors:
Group the prime factors into pairs:
\[ 200 = (2^2) \times (2 \times 5^2) = 4 \times 25 \times 2 \]
Simplify Using Square Roots:
Take the square root of each pair:
\[ \sqrt{200} = \sqrt{4 \times 25 \times 2} \]
Since \(\sqrt{4} = 2\) and \(\sqrt{25} = 5\), we can write:
\[ \sqrt{200} = \sqrt{4} \times \sqrt{25} \times \sqrt{2} = 2 \times 5 \times \sqrt{2} = 10\sqrt{2} \]
Conclusion:
The simplified form of \(\sqrt{200}\) is:
\[ \sqrt{200} = 10\sqrt{2} \]

READ MORE:
Introduction to Square Roots
Square roots are a fundamental concept in mathematics, representing a number that, when multiplied by itself, gives the original number. For example, the square root of 9 is 3 because \(3 \times 3 = 9\). Understanding square roots is essential for simplifying expressions, solving equations, and working with various mathematical concepts.
Here is a step-by-step guide to understanding square roots:
-
Definition:
The square root of a number \(x\) is denoted as \(\sqrt{x}\) and represents a value that, when squared, equals \(x\).
-
Properties of Square Roots:
- \(\sqrt{x \times y} = \sqrt{x} \times \sqrt{y}\)
- \(\sqrt{\frac{x}{y}} = \frac{\sqrt{x}}{\sqrt{y}}\) (for \(y \neq 0\))
- \((\sqrt{x})^2 = x\)
-
Positive and Negative Roots:
While the principal square root of a positive number is positive, every positive number actually has two square roots: one positive and one negative. For example, the square roots of 16 are 4 and -4 because \(4^2 = 16\) and \((-4)^2 = 16\).
-
Perfect Squares:
A perfect square is an integer that is the square of another integer. Examples include 1, 4, 9, 16, and 25. The square roots of perfect squares are always integers.
-
Non-Perfect Squares:
Numbers that are not perfect squares have irrational square roots, meaning their decimal representation is non-repeating and non-terminating. For instance, \(\sqrt{2}\) is approximately 1.414, but its exact value cannot be precisely written as a decimal.
Understanding Square Roots
Square roots are an essential concept in mathematics, helping us understand the relationship between numbers and their factors. A square root of a number \( x \) is a value that, when multiplied by itself, yields \( x \). For example, \( \sqrt{16} = 4 \) because \( 4 \times 4 = 16 \).
To deepen your understanding of square roots, consider the following key points:
-
Symbol and Notation:
The square root of a number is represented by the radical symbol \( \sqrt{} \). The number under the radical sign is called the radicand.
-
Basic Properties:
- \(\sqrt{x \times y} = \sqrt{x} \times \sqrt{y}\)
- \(\sqrt{\frac{x}{y}} = \frac{\sqrt{x}}{\sqrt{y}}\) (for \( y \neq 0 \))
- \((\sqrt{x})^2 = x\)
-
Principal Square Root:
The principal square root of a positive number is its non-negative square root. For instance, the principal square root of 25 is 5.
-
Positive and Negative Roots:
While the principal square root is positive, every positive number has two square roots: one positive and one negative. For example, \( \sqrt{25} = 5 \) and \( \sqrt{25} = -5 \), since \( 5 \times 5 = 25 \) and \( -5 \times -5 = 25 \).
-
Perfect Squares:
Numbers like 1, 4, 9, 16, and 25 are perfect squares because they are the squares of whole numbers. For example, 16 is a perfect square because it is \( 4^2 \).
-
Non-Perfect Squares:
Numbers that are not perfect squares have irrational square roots, meaning their decimal expansions are non-terminating and non-repeating. For example, \( \sqrt{2} \) is approximately 1.414, but it cannot be expressed as a precise decimal or fraction.
-
Applications:
Square roots are used in various mathematical applications, including solving quadratic equations, analyzing geometric properties, and in real-world contexts such as physics and engineering.
Basic Properties of Square Roots
The properties of square roots are fundamental in simplifying radical expressions. Here are the key properties:
- Product Property: For any non-negative real numbers \(a\) and \(b\), the square root of their product is the product of their square roots.
\[ \sqrt{ab} = \sqrt{a} \cdot \sqrt{b} \] - Quotient Property: For any non-negative real numbers \(a\) and \(b\), where \(b \neq 0\), the square root of their quotient is the quotient of their square roots.
\[ \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \]
These properties help in simplifying expressions involving square roots. Let's look at some examples to understand these properties better.
Example 1: Using the Product Property
Suppose we need to simplify \(\sqrt{50}\). We can break it down using its factors:
- First, we find that \(50 = 25 \cdot 2\).
- Using the product property, we get:
\[ \sqrt{50} = \sqrt{25 \cdot 2} = \sqrt{25} \cdot \sqrt{2} = 5\sqrt{2} \]
Example 2: Using the Quotient Property
Consider the expression \(\sqrt{\frac{49}{16}}\). We can simplify it using the quotient property:
- We separate the numerator and the denominator under the radical:
\[ \sqrt{\frac{49}{16}} = \frac{\sqrt{49}}{\sqrt{16}} = \frac{7}{4} \]
Example 3: Simplifying Radicals with Variables
To simplify an expression like \(\sqrt{32x^4}\), follow these steps:
- Factor the radicand into perfect squares and other factors:
\[ 32x^4 = 16 \cdot 2 \cdot x^4 \] - Apply the product property:
\[ \sqrt{32x^4} = \sqrt{16 \cdot 2 \cdot x^4} = \sqrt{16} \cdot \sqrt{2} \cdot \sqrt{x^4} \] - Simplify each square root:
\[ \sqrt{16} = 4, \quad \sqrt{2} \text{ remains as } \sqrt{2}, \quad \sqrt{x^4} = x^2 \] - Combine the results:
\[ \sqrt{32x^4} = 4x^2\sqrt{2} \]
These properties and examples illustrate the fundamental techniques used to simplify square roots, making it easier to handle more complex mathematical expressions.
Prime Factorization
Prime factorization is a method of breaking down a composite number into the product of its prime factors. To simplify the square root of 200, we first need to find its prime factors.
- Factorize the Number:
We start by dividing 200 by the smallest prime number, which is 2, and continue the process with the quotient until we reach a prime number:
- 200 ÷ 2 = 100
- 100 ÷ 2 = 50
- 50 ÷ 2 = 25
- 25 ÷ 5 = 5
- 5 is a prime number.
Thus, the prime factors of 200 are: 2 × 2 × 2 × 5 × 5.
- Organize the Prime Factors:
Arrange the prime factors in pairs:
- (2, 2)
- (5, 5)
- 2 (unpaired)
- Multiply One Element from Each Pair:
From each pair of identical primes, select one prime:
- 2 from (2, 2)
- 5 from (5, 5)
Multiply the selected primes: 2 × 5 = 10.
- Calculate the Square Root:
The unpaired prime (2) remains under the square root symbol:
- √200 = 10√2
So, the simplified form of the square root of 200 is 10√2.
The steps above detail the process of simplifying the square root of 200 through prime factorization. This method is effective for understanding the structure of the number and extracting the square root in its simplest form.

Steps to Simplify the Square Root of 200
To simplify the square root of 200, follow these detailed steps:
-
Find the Prime Factors of 200:
First, determine the prime factors of 200. Prime factorization involves breaking down the number into its prime factors.
200 can be written as:
\[
200 = 2 \times 2 \times 2 \times 5 \times 5 = 2^3 \times 5^2
\] -
Group the Prime Factors:
Next, group the prime factors into pairs of identical factors.
\[
200 = (2^2 \times 5^2) \times 2
\] -
Apply the Square Root to the Groups:
Take the square root of each pair of prime factors. Remember that the square root of a squared number is the number itself.
\[
\sqrt{200} = \sqrt{(2^2 \times 5^2) \times 2} = \sqrt{2^2} \times \sqrt{5^2} \times \sqrt{2}
\] -
Simplify:
Simplify by removing the squares from under the radical sign.
\[
\sqrt{200} = 2 \times 5 \times \sqrt{2} = 10 \sqrt{2}
\]
Therefore, the simplified form of the square root of 200 is:
\[
\sqrt{200} = 10 \sqrt{2}
\]
This method shows that \(\sqrt{200}\) in its simplest radical form is \(10 \sqrt{2}\). The decimal form of this is approximately 14.142.
Finding Prime Factors of 200
To simplify the square root of 200, we first need to find its prime factors. The prime factorization process involves expressing 200 as a product of prime numbers.
Here are the steps to find the prime factors of 200:
- Start by dividing 200 by the smallest prime number, which is 2:
- 200 ÷ 2 = 100
- Divide 100 by 2 again:
- 100 ÷ 2 = 50
- Continue dividing by 2:
- 50 ÷ 2 = 25
- Since 25 is not divisible by 2, move to the next prime number, which is 5:
- 25 ÷ 5 = 5
- 5 ÷ 5 = 1
Now, we can express 200 as a product of its prime factors:
\(200 = 2 \times 2 \times 2 \times 5 \times 5\)
Or using exponents to group the prime factors:
\(200 = 2^3 \times 5^2\)
We have found that the prime factors of 200 are 2 and 5, with the factorization being \(2^3 \times 5^2\).
Grouping Prime Factors
To simplify the square root of 200, we first need to perform prime factorization and then group the prime factors.
- Prime factorize 200:
We start by dividing 200 by the smallest prime number, which is 2.
\[200 \div 2 = 100\]
Next, we continue factoring 100.
\[100 \div 2 = 50\]
We continue factoring 50.
\[50 \div 2 = 25\]
Finally, we factor 25 using the next smallest prime number, which is 5.
\[25 \div 5 = 5\]
And 5 is already a prime number.
- List all the prime factors:
\[200 = 2 \times 2 \times 2 \times 5 \times 5\]
- Group the prime factors into pairs:
Since we are dealing with square roots, we group the factors in pairs of two identical numbers.
\[200 = (2 \times 2) \times (5 \times 5) \times 2\]
- Group 1: \(2 \times 2\)
- Group 2: \(5 \times 5\)
- Remaining factor: 2
By grouping the prime factors, we have two pairs of identical numbers and one remaining factor. This sets us up to simplify the square root by applying square root properties in the next section.
Applying Square Root Properties
To simplify the square root of 200 using square root properties, we can follow these steps:
- Prime Factorization:
First, we need to find the prime factors of 200. The prime factorization of 200 is:
\[ 200 = 2 \times 2 \times 2 \times 5 \times 5 \]
- Group the Prime Factors:
Next, we group the prime factors in pairs of two:
\[ 200 = (2 \times 2) \times (5 \times 5) \times 2 \]
- Apply the Square Root to Each Group:
We can apply the square root to each group of prime factors:
\[ \sqrt{200} = \sqrt{(2^2 \times 5^2 \times 2)} \]
Using the property \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\), we get:
\[ \sqrt{200} = \sqrt{2^2} \times \sqrt{5^2} \times \sqrt{2} \]
- Simplify the Expression:
We know that \(\sqrt{2^2} = 2\) and \(\sqrt{5^2} = 5\), so the expression simplifies to:
\[ \sqrt{200} = 2 \times 5 \times \sqrt{2} \]
\[ \sqrt{200} = 10\sqrt{2} \]
Therefore, the simplified form of the square root of 200 is \(10\sqrt{2}\).
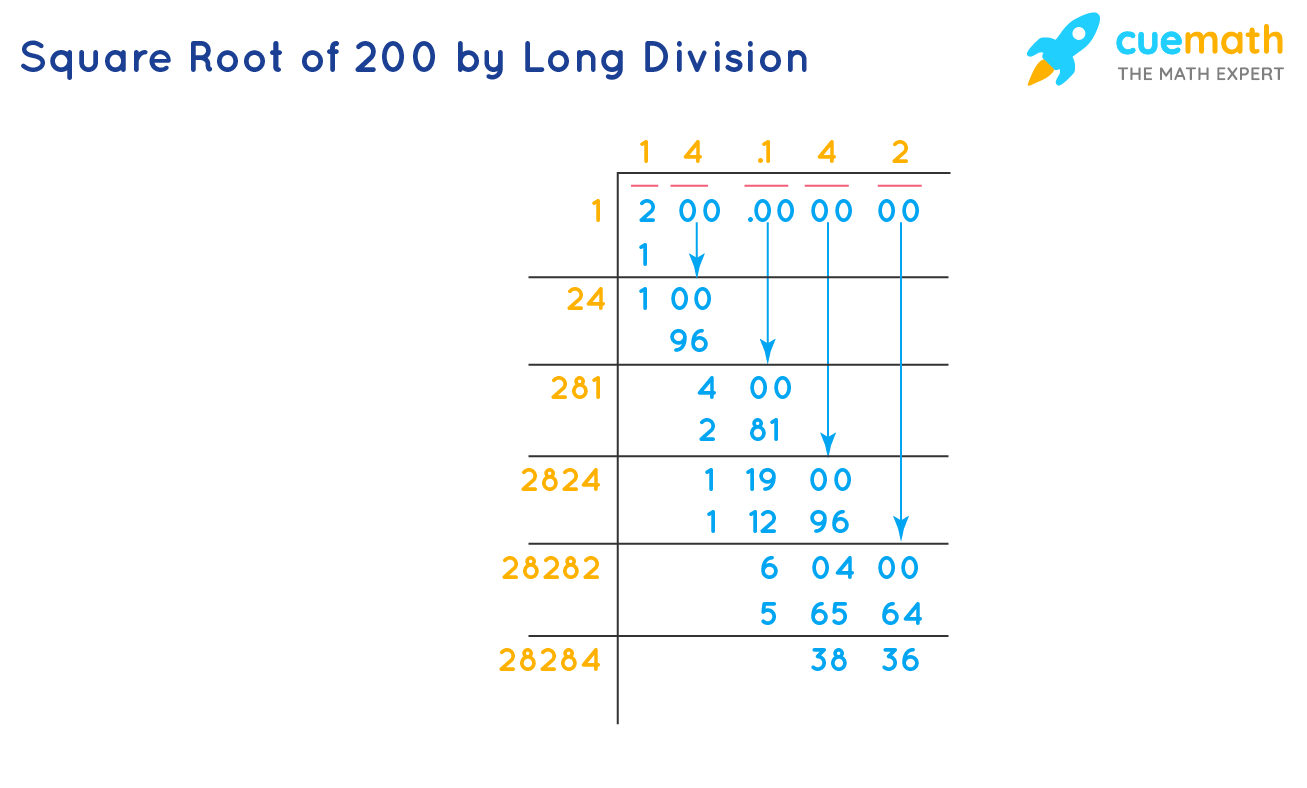
Simplified Form of Square Root of 200
The square root of 200 can be simplified using the method of prime factorization. Let's break down the steps to simplify √200.
- Prime Factorization: Start by finding the prime factors of 200. The prime factorization of 200 is:
- 200 = 2 × 2 × 2 × 5 × 5
- In exponent form: 200 = 23 × 52
- Grouping Prime Factors: Group the factors in pairs of two:
- (2 × 2) × (5 × 5) × 2
- This can be written as: (22) × (52) × 2
- Applying Square Root Properties: Use the property of square roots that states √(a × b) = √a × √b:
- √(22 × 52 × 2) = √(22) × √(52) × √2
- This simplifies to: 2 × 5 × √2
- Simplified Form: Multiply the constants outside the radical:
- 2 × 5 = 10
- So, the simplified form of √200 is: 10√2
Thus, the square root of 200 simplified is 10√2.
Example Problems
Here are some example problems to help you understand how to simplify the square root of 200.
-
Simplify \(\sqrt{200}\)
- Find the largest perfect square factor of 200. The largest perfect square factor of 200 is 100.
- Rewrite \(\sqrt{200}\) as \(\sqrt{100 \times 2}\).
- Separate the square root into two factors: \(\sqrt{100} \times \sqrt{2}\).
- Simplify \(\sqrt{100}\) to get 10.
- The simplified form is \(10\sqrt{2}\).
Thus, \(\sqrt{200} = 10\sqrt{2}\).
-
Simplify \(\sqrt{72}\)
- Find the largest perfect square factor of 72. The largest perfect square factor of 72 is 36.
- Rewrite \(\sqrt{72}\) as \(\sqrt{36 \times 2}\).
- Separate the square root into two factors: \(\sqrt{36} \times \sqrt{2}\).
- Simplify \(\sqrt{36}\) to get 6.
- The simplified form is \(6\sqrt{2}\).
Thus, \(\sqrt{72} = 6\sqrt{2}\).
-
Simplify \(\sqrt{450}\)
- Find the largest perfect square factor of 450. The largest perfect square factor of 450 is 225.
- Rewrite \(\sqrt{450}\) as \(\sqrt{225 \times 2}\).
- Separate the square root into two factors: \(\sqrt{225} \times \sqrt{2}\).
- Simplify \(\sqrt{225}\) to get 15.
- The simplified form is \(15\sqrt{2}\).
Thus, \(\sqrt{450} = 15\sqrt{2}\).
Practice Problems
Try solving these practice problems to reinforce your understanding of simplifying square roots:
-
Simplify \(\sqrt{50}\)
- Find the largest perfect square factor of 50. The largest perfect square factor of 50 is 25.
- Rewrite \(\sqrt{50}\) as \(\sqrt{25 \times 2}\).
- Separate the square root into two factors: \(\sqrt{25} \times \sqrt{2}\).
- Simplify \(\sqrt{25}\) to get 5.
- The simplified form is \(5\sqrt{2}\).
-
Simplify \(\sqrt{98}\)
- Find the largest perfect square factor of 98. The largest perfect square factor of 98 is 49.
- Rewrite \(\sqrt{98}\) as \(\sqrt{49 \times 2}\).
- Separate the square root into two factors: \(\sqrt{49} \times \sqrt{2}\).
- Simplify \(\sqrt{49}\) to get 7.
- The simplified form is \(7\sqrt{2}\).
-
Simplify \(\sqrt{128}\)
- Find the largest perfect square factor of 128. The largest perfect square factor of 128 is 64.
- Rewrite \(\sqrt{128}\) as \(\sqrt{64 \times 2}\).
- Separate the square root into two factors: \(\sqrt{64} \times \sqrt{2}\).
- Simplify \(\sqrt{64}\) to get 8.
- The simplified form is \(8\sqrt{2}\).
-
Simplify \(\sqrt{18}\)
- Find the largest perfect square factor of 18. The largest perfect square factor of 18 is 9.
- Rewrite \(\sqrt{18}\) as \(\sqrt{9 \times 2}\).
- Separate the square root into two factors: \(\sqrt{9} \times \sqrt{2}\).
- Simplify \(\sqrt{9}\) to get 3.
- The simplified form is \(3\sqrt{2}\).
-
Simplify \(\sqrt{72}\)
- Find the largest perfect square factor of 72. The largest perfect square factor of 72 is 36.
- Rewrite \(\sqrt{72}\) as \(\sqrt{36 \times 2}\).
- Separate the square root into two factors: \(\sqrt{36} \times \sqrt{2}\).
- Simplify \(\sqrt{36}\) to get 6.
- The simplified form is \(6\sqrt{2}\).
Common Mistakes to Avoid
When simplifying square roots, there are several common mistakes that can lead to incorrect results. By being aware of these mistakes, you can avoid them and simplify square roots correctly and efficiently.
-
Forgetting to Check for Perfect Squares:
Before simplifying a square root, always check if the number is a perfect square. For instance, \( \sqrt{16} \) simplifies directly to 4. Skipping this step can lead to unnecessary complications.
-
Incorrect Prime Factorization:
Ensure that you perform prime factorization correctly. For example, the prime factors of 200 are 2, 2, 2, and 5. Incorrect factorization can lead to errors in simplification.
-
Not Grouping Factors Properly:
When grouping prime factors, make sure to pair them correctly to simplify outside the square root. For \( \sqrt{200} \), grouping the factors as \( 2 \times 2 \times 5 \times 5 \) helps to correctly simplify to \( 10\sqrt{2} \).
-
Rushing Through the Simplification Process:
Simplifying square roots requires careful and deliberate steps. Take your time to factorize, group, and simplify each component.
-
Combining Unlike Radicals:
Remember that you can only combine like radicals. For example, \( 2\sqrt{3} \) and \( 5\sqrt{3} \) can be combined to \( 7\sqrt{3} \), but \( 2\sqrt{3} \) and \( 5\sqrt{5} \) cannot be combined.
By keeping these common mistakes in mind and practicing regularly, you can master the process of simplifying square roots efficiently and accurately.

Conclusion
Simplifying square roots is a fundamental skill in algebra that helps in making complex expressions more manageable. By breaking down the number inside the radical into its prime factors, we can identify and extract perfect squares, thus simplifying the square root.
In the case of √200, we determined that 200 can be factored into 2 × 100, where 100 is a perfect square. This allowed us to simplify √200 to 10√2.
Understanding the steps and properties of square roots not only aids in simplifying radicals but also enhances overall problem-solving skills in mathematics. Practice with various examples and problem sets to strengthen your ability to simplify square roots efficiently.
Remember, the key steps are:
- Identify the prime factors of the number inside the radical.
- Group the factors to find perfect squares.
- Extract the square root of the perfect squares and simplify the expression.
With consistent practice and attention to detail, you will avoid common mistakes and become proficient in simplifying square roots. Keep exploring and practicing to master this essential mathematical concept.
Further Reading and Resources
For more information and deeper understanding of simplifying square roots, consider exploring the following resources:
-
This resource provides comprehensive lessons on the properties and simplification of square roots and radicals, with videos and practice problems to reinforce learning.
-
Mathway offers step-by-step solutions to various algebra problems, including the simplification of square roots, helping to visualize each step of the process.
-
This guide breaks down the process of simplifying the square root of 200 into clear, easy-to-follow steps, making it a useful reference for students.
-
Symbolab provides an online calculator and detailed explanations for simplifying square roots and other algebraic expressions.
sqrt(200), căn bậc hai của 200 đơn giản hóa
READ MORE:
Giản thể căn bậc hai của 200 và các phép tính liên quan.
Giản thể căn bậc hai của 200 - Video