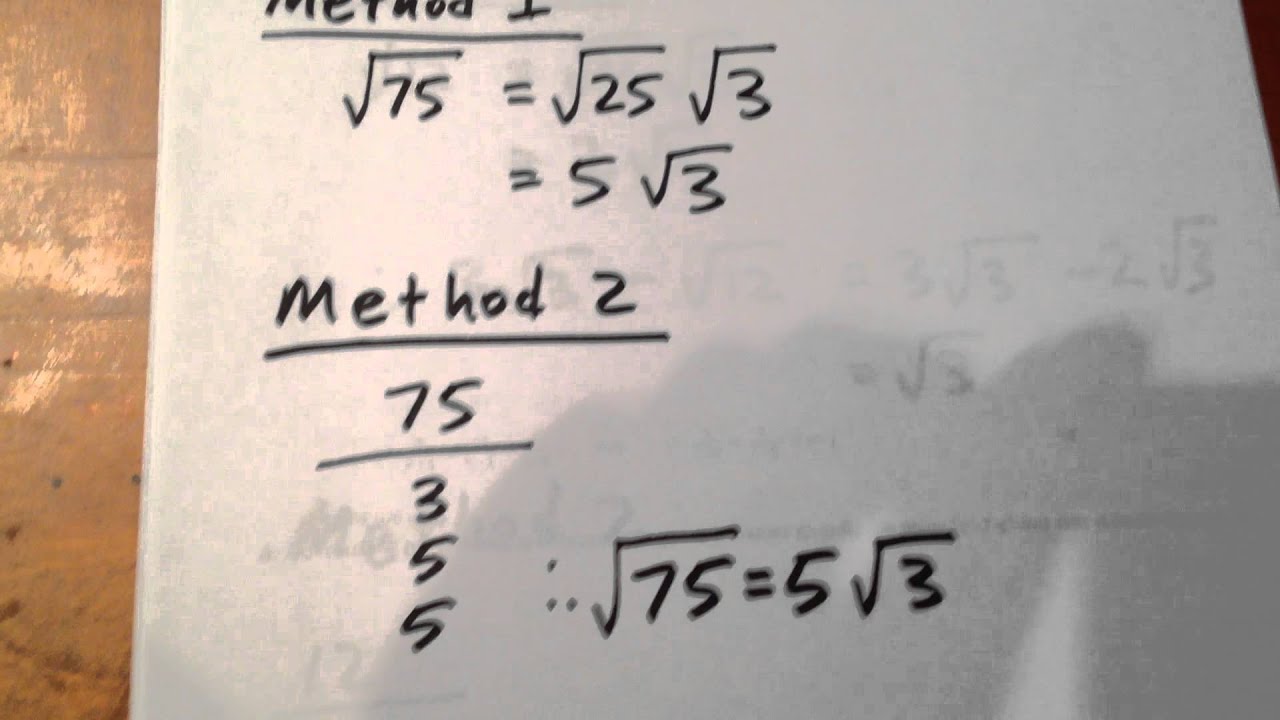Calculator for Simplifying Square Roots: Your Ultimate Guide

Use this calculator to find the principal square root and roots of real numbers. You can enter positive or negative real numbers to get both exact and approximate results.

Calculator Use

Simply input the radicand and the calculator will provide the square root in both simplest radical form and as a decimal approximation. It also handles complex numbers for negative radicands.

Example Calculations

• \(\sqrt{81} = \pm 9\)
• \(\sqrt{25} = \pm 5\)
• \(\sqrt{100} = \pm 10\)
• \(\sqrt{10} \approx \pm 3.162\)

Square Root Simplification Rules

• \(\sqrt{x \cdot y} = \sqrt{x} \cdot \sqrt{y}\)
• \(\frac{\sqrt{x}}{\sqrt{y}} = \sqrt{\frac{x}{y}}\)
• \(\sqrt{x^2 \cdot y} = x \cdot \sqrt{y}\)

Steps for Simplifying Square Roots

1. Identify the radical expression.
2. Group multiplying radicals under one radical using Rule 1.
3. If dividing radicals, use Rule 2 to combine them under one radical.
4. Simplify the expression inside the radical as much as possible.

Special Cases

For fractions, multiply the numerator and the denominator by the radical to rationalize the denominator.

Example: \(\frac{a}{\sqrt{b}} = \frac{a\sqrt{b}}{b}\)

Why Simplify Square Roots?

Simplifying square roots is essential for solving equations in algebra, calculating lengths in geometry, and more. Simplified forms are easier to work with and understand in various mathematical contexts.

Common Uses of Square Roots

• Solving polynomial equations
• Calculating vector norms in geometry
• Applying the Pythagorean theorem

What is a Square Number?

A square number is the square of an integer. For example, 9 is a square number because \(3^2 = 9\).

Simplifying square roots is an essential skill in algebra that helps in solving equations and simplifying expressions. The process involves expressing the square root in its simplest form by factoring out perfect squares. This not only makes calculations easier but also provides a clearer understanding of the mathematical relationships involved.

When simplifying square roots, you follow specific rules and steps to break down the radical into its simplest components. Here's a detailed step-by-step guide to simplify square roots:

1. Identify the radical expression that needs simplification.
2. Factor the number under the square root into its prime factors.
3. Group the prime factors into pairs.
4. Move each pair of prime factors outside the square root as a single factor.
5. Multiply the factors outside the square root together.
6. If there are any remaining factors inside the square root, multiply them together.
7. Combine the simplified factors outside and inside the square root to get the final simplified form.

For example, to simplify \(\sqrt{72}\):

• Factor 72 into prime factors: \(72 = 2 \times 2 \times 2 \times 3 \times 3\).
• Group the factors into pairs: \((2 \times 2) \) and \((3 \times 3)\).
• Move each pair outside the square root: \(2 \times 3\).
• Multiply the factors outside the square root: \(2 \times 3 = 6\).
• No remaining factors inside the square root.
• The simplified form is \(6\sqrt{2}\).

Understanding and applying these steps will make working with square roots more manageable and intuitive. Practice with different numbers will help reinforce these concepts and improve your algebra skills.

Understanding how to simplify square roots involves recognizing a few basic rules and concepts. Simplifying square roots can make calculations easier and expressions more manageable.

• Square Root Definition: The square root of a number \( x \) is a number \( y \) such that \( y^2 = x \). For example, \( \sqrt{25} = 5 \) because \( 5^2 = 25 \).
• Product Rule: The square root of a product is the product of the square roots: \( \sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b} \).
• Quotient Rule: The square root of a quotient is the quotient of the square roots: \( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \).

When simplifying square roots, the goal is to reduce the number inside the square root to its simplest form. Here are the steps:

1. Identify the factors of the number under the square root.
2. Pair up any prime factors.
3. Move each pair of prime factors outside the square root.
4. Multiply the numbers outside the square root and leave the remaining factors inside.

Let's look at an example to illustrate these steps:

Example: Simplify \( \sqrt{72} \)

• First, factor 72 into its prime factors: \( 72 = 2 \times 2 \times 2 \times 3 \times 3 \).
• Pair the prime factors: \( (2 \times 2) \) and \( (3 \times 3) \).
• Move each pair outside the square root: \( \sqrt{72} = \sqrt{(2 \times 2) \times (3 \times 3) \times 2} = 2 \times 3 \times \sqrt{2} \).
• The simplified form is \( 6\sqrt{2} \).

These rules and steps can be applied to any square root to simplify it. Practice with different numbers will help reinforce these concepts and improve your skills in simplifying square roots.

To simplify square roots, follow these detailed steps. This guide will help you understand the process and make it easier to handle square root calculations.

1. Identify the Radicand

   The radicand is the number inside the square root symbol. For example, in √12, the radicand is 12.

2. Factorize the Radicand

   Break down the radicand into its prime factors. For instance, 12 can be factored into 2 × 2 × 3.

3. Pair the Prime Factors

   Group the prime factors into pairs. Each pair of the same number can be taken out of the square root. For √12:

   • √(2 × 2 × 3) = √(4 × 3)
   • √(4 × 3) = √4 × √3
   • Since √4 = 2, we get 2√3.
4. Simplify the Expression

   Write the simplified expression by taking the pairs out of the square root and leaving any unpaired factors inside. Thus, √12 simplifies to 2√3.

Let's look at another example:

1. Example: Simplify √45

   Step 1: Factorize 45 into prime factors: 45 = 3 × 3 × 5.

   Step 2: Group the pairs: √(3 × 3 × 5).

   Step 3: Take the pair of 3 out: 3√5.

   Therefore, √45 simplifies to 3√5.

2. Example: Simplify √72

   Step 1: Factorize 72 into prime factors: 72 = 2 × 2 × 2 × 3 × 3.

   Step 2: Group the pairs: √(2 × 2 × 2 × 3 × 3).

   Step 3: Take out the pairs of 2 and 3: 6√2.

   Therefore, √72 simplifies to 6√2.

These steps can be used to simplify any square root, making it easier to handle in various mathematical problems. Practice these steps to become proficient in simplifying square roots.

Simplifying square roots can be a straightforward process when you follow specific rules and steps. Here are some detailed examples to help you understand how to simplify square roots:

• Example 1: Simplifying √27
  1. Identify factors of 27: 1, 3, 9, 27.
  2. Find the largest perfect square factor: 9.
  3. Rewrite √27 as √(9×3).
  4. Use the property √(a×b) = √a × √b to simplify: √9 × √3 = 3√3.
• Example 2: Simplifying √18
  1. Identify factors of 18: 1, 2, 3, 6, 9, 18.
  2. Find the largest perfect square factor: 9.
  3. Rewrite √18 as √(9×2).
  4. Use the property √(a×b) = √a × √b to simplify: √9 × √2 = 3√2.
• Example 3: Simplifying √50
  1. Identify factors of 50: 1, 2, 5, 10, 25, 50.
  2. Find the largest perfect square factor: 25.
  3. Rewrite √50 as √(25×2).
  4. Use the property √(a×b) = √a × √b to simplify: √25 × √2 = 5√2.
• Example 4: Simplifying √72
  1. Identify factors of 72: 1, 2, 3, 4, 6, 8, 9, 12, 18, 24, 36, 72.
  2. Find the largest perfect square factor: 36.
  3. Rewrite √72 as √(36×2).
  4. Use the property √(a×b) = √a × √b to simplify: √36 × √2 = 6√2.

By following these steps and applying the properties of square roots, you can simplify various square root expressions efficiently.

In today's digital age, several online tools are available to help you simplify square roots quickly and accurately. These calculators not only perform the basic arithmetic but also provide step-by-step solutions, making the process easier to understand. Here are some notable calculator tools for simplifying square roots:

• Omni Simplifying Radicals Calculator:

  This tool simplifies square roots by breaking down the radical expression into its simplest form. It allows you to input multiple radicals and performs addition, subtraction, multiplication, and division of radical expressions. The calculator also provides a detailed explanation of each step involved in the simplification process.

• Mathway Square Root Calculator:

  Mathway's calculator finds the square root of any given radical expression and provides the result in both exact and decimal forms. It's user-friendly and offers a step-by-step guide to help users understand how the solution is derived.

• MathCracker Simplifying Radicals Calculator:

  This calculator focuses on simplifying radicals using fundamental rules, such as \(\sqrt{x} \cdot \sqrt{y} = \sqrt{xy}\) and \(\frac{\sqrt{x}}{\sqrt{y}} = \sqrt{\frac{x}{y}}\). It helps group and reduce radical expressions efficiently.

• MathWarehouse Square Root Calculator:

  MathWarehouse offers a free tool that reduces any square root to its simplest radical form and also provides an approximate decimal value. It supports both positive and negative numbers, and handles complex numbers as well.

• Number Maniacs Simplify Square Root Calculator:

  This calculator provides step-by-step instructions to simplify any given square root to its simplest form. It is particularly useful for educational purposes, demonstrating the simplification process in a clear and concise manner.

Using these tools can significantly enhance your understanding and efficiency in simplifying square roots, making complex calculations more manageable and less time-consuming.

When simplifying square roots, it's easy to make mistakes that can lead to incorrect results. Here are some common mistakes and tips on how to avoid them:

• Forgetting to factor completely: Always factor the number inside the square root completely to identify all possible perfect squares.
• Not applying the product rule correctly: Remember that \(\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\). Ensure you apply this rule correctly to simplify the expression.
• Ignoring negative signs: Be mindful of the properties of square roots with negative numbers. For real numbers, \(\sqrt{a}\) is only defined for \(a \geq 0\).
• Incorrectly combining radicals: Ensure that when you combine radicals, the numbers under the radicals are multiplied correctly. For example, \(\sqrt{4} \cdot \sqrt{9} = \sqrt{36} = 6\).
• Misapplying the quotient rule: When dividing under a square root, use the rule \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\) correctly.

By keeping these tips in mind and practicing regularly, you can avoid these common mistakes and become proficient in simplifying square roots.

When dealing with complex radicals, especially those involving higher-degree roots or multiple terms, it's essential to employ advanced techniques for simplification. Here are some detailed methods to handle these complex radicals:

1. Prime Factorization

   Break down the number inside the radical into its prime factors. This helps in identifying perfect squares (or cubes, etc.) that can be simplified. For example:

   \( \sqrt[3]{72} \)

   • Prime factorize 72: \( 72 = 2^3 \times 3^2 \)
   • Simplify inside the radical: \( \sqrt[3]{2^3 \times 3^2} = 2 \sqrt[3]{3^2} \)
2. Rationalizing the Denominator

   To simplify a radical expression with a radical in the denominator, multiply both the numerator and the denominator by a conjugate or an appropriate term to eliminate the radical. For instance:

   \( \frac{1}{\sqrt{2}} \times \frac{\sqrt{2}}{\sqrt{2}} = \frac{\sqrt{2}}{2} \)

3. Using Conjugates

   For expressions involving sums or differences with radicals, multiply by the conjugate to simplify. Example:

   \( \frac{1}{\sqrt{a} + \sqrt{b}} \times \frac{\sqrt{a} - \sqrt{b}}{\sqrt{a} - \sqrt{b}} = \frac{\sqrt{a} - \sqrt{b}}{a - b} \)

4. Combining Like Terms

   If the expression involves multiple radicals, combine like terms by factoring out common radicals:

   \( \sqrt{50} + 2\sqrt{2} = 5\sqrt{2} + 2\sqrt{2} = 7\sqrt{2} \)

5. Higher-Degree Radicals

   For higher-degree roots, use similar principles as square roots, but factorize to the appropriate degree. For example, to simplify \( \sqrt[4]{16x^8} \):

   \( 16x^8 = (2^4)(x^8) \rightarrow \sqrt[4]{(2^4)(x^8)} = 2x^2 \)

By mastering these advanced techniques, you'll be able to simplify even the most complex radical expressions effectively.

Simplifying fractions with square roots involves a few steps to ensure that the fraction is expressed in its simplest form. Below are the detailed steps and examples to guide you through the process:

Step-by-Step Guide

1. Identify the fraction: Start with a fraction that has a square root in the numerator, the denominator, or both. For example, consider the fraction \( \frac{a}{\sqrt{b}} \).

2. Rationalize the denominator: If the denominator contains a square root, multiply both the numerator and the denominator by the square root found in the denominator. This process is called rationalizing the denominator.

   For example, to simplify \( \frac{5}{\sqrt{3}} \):

   • Multiply both the numerator and the denominator by \( \sqrt{3} \):
   • \( \frac{5}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{5\sqrt{3}}{3} \)

3. Combine and simplify: Simplify the resulting fraction if possible. The result should have no square root in the denominator.

Examples

• Example 1: Simplify \( \frac{\sqrt{8}}{\sqrt{2}} \)

  • Combine the square roots: \( \frac{\sqrt{8}}{\sqrt{2}} = \sqrt{\frac{8}{2}} \)
  • Simplify the fraction under the square root: \( \sqrt{\frac{8}{2}} = \sqrt{4} \)
  • Take the square root of the simplified fraction: \( \sqrt{4} = 2 \)
  • So, \( \frac{\sqrt{8}}{\sqrt{2}} = 2 \)

• Example 2: Simplify \( \frac{7}{\sqrt{5}} \)

  • Rationalize the denominator: \( \frac{7}{\sqrt{5}} \times \frac{\sqrt{5}}{\sqrt{5}} = \frac{7\sqrt{5}}{5} \)
  • The simplified fraction is \( \frac{7\sqrt{5}}{5} \)

Complex Fractions

For more complex fractions, such as those involving sums or differences of square roots in the denominator, apply the conjugate method:

• To simplify \( \frac{a}{\sqrt{b} + \sqrt{c}} \), multiply both the numerator and the denominator by the conjugate \( \sqrt{b} - \sqrt{c} \).
• This results in \( \frac{a (\sqrt{b} - \sqrt{c})}{(\sqrt{b} + \sqrt{c})(\sqrt{b} - \sqrt{c})} = \frac{a (\sqrt{b} - \sqrt{c})}{b - c} \).

Calculator Tools

Using online calculator tools can greatly simplify the process of handling square roots in fractions. These tools can provide step-by-step solutions and ensure accuracy.

Some recommended online tools include:

These calculators can help you verify your steps and understand the simplification process better.

By following these methods, you can simplify any fraction with square roots and ensure that your final expression is in its simplest form.

Square roots are fundamental in various areas of mathematics, providing essential tools for solving equations, calculating distances, and understanding geometric properties. Here are some key applications of square roots:

• Solving Quadratic Equations

  Square roots are used in the quadratic formula to find the solutions of quadratic equations. The quadratic formula is given by:

  $$x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}$$

  This formula provides the roots of the equation \(ax^2 + bx + c = 0\), where \(a\), \(b\), and \(c\) are constants.

• Pythagorean Theorem

  In geometry, the square root is crucial in the Pythagorean Theorem, which relates the lengths of the sides of a right triangle:

  $$a^2 + b^2 = c^2$$

  Here, \(c\) is the hypotenuse, and \(a\) and \(b\) are the other two sides. To find the length of the hypotenuse, you can use:

  $$c = \sqrt{a^2 + b^2}$$

• Distance Formula

  The distance between two points \((x_1, y_1)\) and \((x_2, y_2)\) in a plane can be found using the distance formula, which is derived from the Pythagorean Theorem:

  $$d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2}$$

• Standard Deviation in Statistics

  Square roots are used to calculate the standard deviation, a measure of the amount of variation or dispersion in a set of values. The formula for standard deviation is:

  $$\sigma = \sqrt{\frac{\sum_{i=1}^{N} (x_i - \mu)^2}{N}}$$

  where \(N\) is the number of observations, \(x_i\) are the values, and \(\mu\) is the mean of the values.

• Area and Volume Calculations

  Square roots are also used in various formulas to calculate areas and volumes. For example, the formula for the area of a circle involves the square root when derived from the equation of a circle:

  $$A = \pi r^2$$

  where \(r\) is the radius. To find the radius from the area, you would use:

  $$r = \sqrt{\frac{A}{\pi}}$$

Simplifying square roots is a fundamental skill in mathematics that has a wide range of applications, from basic arithmetic to advanced algebra and geometry. Understanding the rules and techniques for simplifying square roots can greatly enhance your problem-solving abilities and mathematical proficiency.

To conclude, let's recap some key points:

• Basic Rules: Remember the essential rules for simplifying square roots, such as \(\sqrt{a} \times \sqrt{b} = \sqrt{a \times b}\) and \(\frac{\sqrt{a}}{\sqrt{b}} = \sqrt{\frac{a}{b}}\). These rules are crucial for manipulating and simplifying radical expressions.
• Factorization: Simplifying a square root often involves factoring the number under the radical into its prime components and extracting squares from the radical. For example, \(\sqrt{50} = \sqrt{25 \times 2} = 5\sqrt{2}\).
• Calculator Tools: There are numerous online calculators available that can simplify square roots and provide step-by-step solutions, such as those from Omni Calculator and MathCracker. These tools can be very helpful for checking your work and understanding the process.
• Applications: Square roots are used in various areas of mathematics, including solving quadratic equations, calculating distances using the Pythagorean theorem, and working with complex numbers.

For further reading and practice, consider exploring the following resources:

• : A comprehensive tool for simplifying radical expressions with detailed explanations.
• : Another excellent calculator that provides step-by-step solutions for square root problems.
• : Offers various methods for calculating and simplifying square roots, including by hand and using a calculator.

By practicing regularly and utilizing these resources, you can master the art of simplifying square roots and apply this knowledge effectively in your mathematical endeavors.