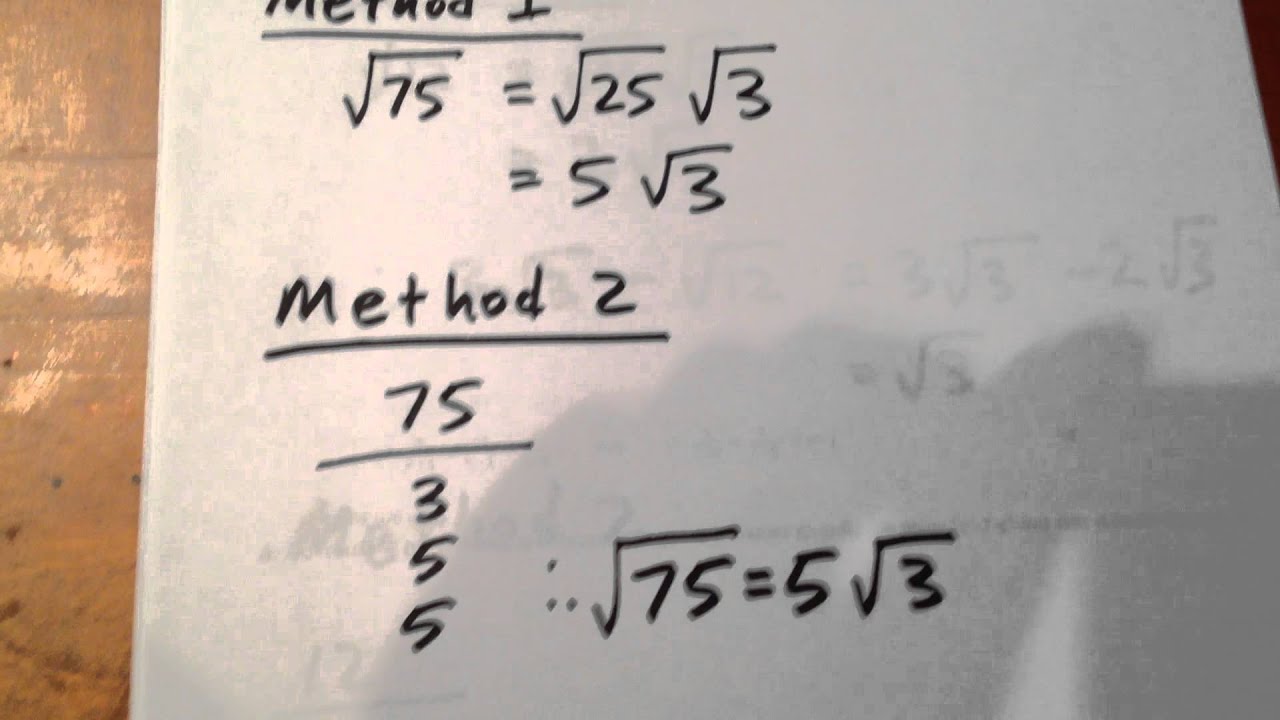Simplifying Square Root Fractions: A Comprehensive Guide

Simplifying square root fractions can often be made easier by breaking down the steps and using properties of square roots. Below, we'll guide you through the process with clear examples and rules.

Basic Principles

• The square root of a fraction can be simplified by taking the square root of the numerator and the denominator separately:

$$ \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} $$

• Rationalize the denominator by eliminating the square root in the denominator:

$$ \frac{a}{\sqrt{b}} = \frac{a \sqrt{b}}{b} $$

Step-by-Step Example

Let's simplify the fraction \( \sqrt{\frac{50}{18}} \):

1. Simplify the fraction under the square root:

$$ \frac{50}{18} = \frac{25}{9} $$

2. Take the square root of the numerator and the denominator:

$$ \sqrt{\frac{25}{9}} = \frac{\sqrt{25}}{\sqrt{9}} = \frac{5}{3} $$

3. The simplified form of \( \sqrt{\frac{50}{18}} \) is \( \frac{5}{3} \).

Additional Examples

Let's look at a few more examples to understand the concept better:

Example 1

$$ \sqrt{\frac{8}{2}} $$

1. Simplify the fraction under the square root:

$$ \frac{8}{2} = 4 $$

2. Take the square root:

$$ \sqrt{4} = 2 $$

Example 2

$$ \sqrt{\frac{32}{18}} $$

1. Simplify the fraction under the square root:

$$ \frac{32}{18} = \frac{16}{9} $$

2. Take the square root of the numerator and the denominator:

$$ \sqrt{\frac{16}{9}} = \frac{\sqrt{16}}{\sqrt{9}} = \frac{4}{3} $$

Example 3

$$ \sqrt{\frac{12}{75}} $$

1. Simplify the fraction under the square root:

$$ \frac{12}{75} = \frac{4}{25} $$

2. Take the square root of the numerator and the denominator:

$$ \sqrt{\frac{4}{25}} = \frac{\sqrt{4}}{\sqrt{25}} = \frac{2}{5} $$

Rationalizing the Denominator

Sometimes, the fraction might not simplify neatly. For example:

$$ \frac{3}{\sqrt{5}} $$

1. Multiply the numerator and the denominator by the square root in the denominator:

$$ \frac{3}{\sqrt{5}} \cdot \frac{\sqrt{5}}{\sqrt{5}} = \frac{3 \sqrt{5}}{5} $$

2. The fraction is now rationalized:

$$ \frac{3 \sqrt{5}}{5} $$

Simplifying square root fractions may seem challenging, but with a clear understanding of the steps involved, it becomes manageable. The key is to simplify the square roots and then rationalize the denominator if needed. This process can be broken down into several steps to make it easier.

• Step 1: Simplify the Square Root - Begin by simplifying any square roots in the fraction. For instance, the square root of 72 can be simplified to \(6\sqrt{2}\) because \(72 = 36 \times 2\).
• Step 2: Rationalize the Denominator - If the denominator contains a square root, multiply the numerator and the denominator by the conjugate of the denominator. This eliminates the square root in the denominator. For example, to rationalize \(\frac{5}{\sqrt{3}}\), multiply by \(\frac{\sqrt{3}}{\sqrt{3}}\) to get \(\frac{5\sqrt{3}}{3}\).
• Step 3: Simplify the Fraction - Finally, simplify the resulting fraction if possible. For example, \(\frac{\sqrt{18}}{3}\) simplifies to \(\sqrt{2}\) after simplifying the square root and the fraction.

Mastering these steps will help you confidently simplify any fraction involving square roots, making it easier to work with in mathematical problems.

Square roots and fractions are fundamental concepts in mathematics that often appear together in various problems. To understand how to simplify square root fractions, it's essential to grasp these concepts individually first.

Square Roots: The square root of a number \( x \) is a value that, when multiplied by itself, gives the number \( x \). It is represented as \( \sqrt{x} \). For example, \( \sqrt{25} = 5 \) because \( 5 \times 5 = 25 \). The square root function is essential when dealing with quadratic equations and other areas of algebra.

Fractions: A fraction represents a part of a whole and is written as \( \frac{a}{b} \), where \( a \) is the numerator and \( b \) is the denominator. Fractions are used to express ratios, proportions, and divisions. Simplifying fractions involves reducing the numerator and the denominator to their smallest possible values while maintaining the same value of the fraction.

When we combine these two concepts, we get square root fractions, which are fractions that contain square roots in either the numerator, the denominator, or both. Simplifying these fractions follows specific steps and principles.

Steps to Simplify Square Root Fractions

1. Identify the Square Root: Determine whether the square root is in the numerator, the denominator, or both.
2. Simplify the Square Root: Simplify the square root by finding the prime factors and reducing it to its simplest form. For example, \( \sqrt{18} = \sqrt{9 \times 2} = 3\sqrt{2} \).
3. Simplify the Fraction: If possible, reduce the fraction by finding the greatest common divisor (GCD) of the numerator and the denominator.
4. Rationalize the Denominator: If the square root is in the denominator, multiply the numerator and the denominator by a value that will eliminate the square root from the denominator. For example, to simplify \( \frac{1}{\sqrt{2}} \), multiply by \( \frac{\sqrt{2}}{\sqrt{2}} \) to get \( \frac{\sqrt{2}}{2} \).

Example

Let's simplify the fraction \( \frac{\sqrt{50}}{10} \):

1. Identify the Square Root: The square root is in the numerator.
2. Simplify the Square Root: \( \sqrt{50} = \sqrt{25 \times 2} = 5\sqrt{2} \).
3. Simplify the Fraction: \( \frac{5\sqrt{2}}{10} = \frac{\sqrt{2}}{2} \).
4. The fraction is now simplified: \( \frac{\sqrt{2}}{2} \).

By following these steps, you can simplify any fraction involving square roots, making them easier to work with in mathematical problems.

Simplifying fractions involves reducing the fraction to its simplest form so that the numerator and the denominator have no common factors other than 1. This is a crucial skill in algebra and arithmetic. Here are the basic principles to follow:

1. Identify the Greatest Common Factor (GCF):

   Find the largest number that divides both the numerator and the denominator without leaving a remainder. This is known as the Greatest Common Factor (GCF).

   For example, in the fraction \\(\frac{18}{24}\\), the GCF of 18 and 24 is 6.

2. Divide Both the Numerator and the Denominator by the GCF:

   Once the GCF is identified, divide both the numerator and the denominator by this number.

   Continuing with our example, \\(\frac{18}{24} = \frac{18 \div 6}{24 \div 6} = \frac{3}{4}\\).

3. Simplify Square Roots:

   If the fraction involves square roots, simplify the square roots before simplifying the fraction.

   For example, \\(\frac{\sqrt{72}}{8}\\) can be simplified by first simplifying the square root: \\(\sqrt{72} = \sqrt{36 \cdot 2} = 6\sqrt{2}\\). Thus, the fraction becomes \\(\frac{6\sqrt{2}}{8}\\), which simplifies to \\(\frac{3\sqrt{2}}{4}\\) when divided by 2.

4. Rationalize the Denominator:

   If the denominator contains a square root, multiply both the numerator and the denominator by the square root to eliminate it.

   For instance, \\(\frac{5}{\sqrt{3}}\\) can be rationalized by multiplying by \\(\frac{\sqrt{3}}{\sqrt{3}}\\), resulting in \\(\frac{5\sqrt{3}}{3}\\).

5. Combine Like Terms:

   After simplifying the square roots and rationalizing the denominator, combine any like terms in the numerator or the denominator if possible.

   For example, \\(\frac{3\sqrt{2} + 2\sqrt{2}}{\sqrt{3}}\\) can be combined to \\(\frac{5\sqrt{2}}{\sqrt{3}}\\), which can then be rationalized to \\(\frac{5\sqrt{6}}{3}\\).

By following these steps, you can simplify any fraction, whether it involves square roots or not. Simplifying fractions makes them easier to work with in more complex mathematical operations.

Simplifying square root fractions involves a few key methods that can help transform complex expressions into more manageable forms. Below are detailed steps to achieve this:

Simplify the Square Root

The first step is to simplify any square roots in the fraction. This involves finding the largest perfect square that can be factored out of the radicand (the number under the square root).

• For example, to simplify $\sqrt{72}$, we recognize that 72 = 36 × 2, so we have:

  $\sqrt{72}=\sqrt{36}\sqrt{2}=\; 6\sqrt{2}$

Rationalize the Denominator

When a fraction has a square root in the denominator, we rationalize it by multiplying both the numerator and denominator by a value that will eliminate the square root from the denominator.

• For instance, to rationalize $\frac{5}{\sqrt{3}}$, we multiply by $\frac{\sqrt{3}}{\sqrt{3}}$:

  $\frac{5}{\sqrt{3}}\times \frac{\sqrt{3}}{\sqrt{3}}=\frac{5}{3}\sqrt{3}$

Simplify the Fraction

Next, simplify the fraction by combining like terms and reducing it to its simplest form. This includes combining square roots where possible and reducing the numerical fractions.

• For example, to simplify $\frac{\sqrt{18}}{3}$:

  $\sqrt{18}=\sqrt{9}\sqrt{2}=\; 3\sqrt{2}$

  Then, $\frac{3}{3}\sqrt{2}=\sqrt{2}$

Examples

• To simplify $\frac{2}{\sqrt{12}}$:

  $\sqrt{12}=\sqrt{4}\sqrt{3}=\; 2\sqrt{3}$

  Then, $\frac{2}{2}\sqrt{3}=\frac{1}{3}$

• To simplify $\frac{\sqrt{6}}{\sqrt{2}}$:

  $\sqrt{6}=\sqrt{2}\sqrt{3}$

  Then, $\frac{\sqrt{2\sqrt{3}}}{\sqrt{2}}=\sqrt{3}$

Practice Problems

To master these methods, practice with additional problems:

• Simplify $\frac{\sqrt{50}}{5}$
• Simplify $\frac{4}{\sqrt{18}}$

When simplifying fractions with square roots in the numerator, the goal is to simplify the square root and reduce the fraction to its simplest form. Here are the steps to follow:

1. Simplify the Square Root:

   Factor the number inside the square root to find any perfect squares. Extract the square root of these perfect squares.

   For example, simplify \( \frac{\sqrt{18}}{5} \):

   \( \sqrt{18} = \sqrt{9 \times 2} = \sqrt{9} \times \sqrt{2} = 3\sqrt{2} \)

   So, \( \frac{\sqrt{18}}{5} = \frac{3\sqrt{2}}{5} \).

2. Rationalize the Denominator (if needed):

   If there is a square root in the denominator, rationalize it by multiplying the numerator and denominator by the conjugate of the denominator.

   For example, simplify \( \frac{2}{\sqrt{12}} \):

   \( \sqrt{12} = \sqrt{4 \times 3} = 2\sqrt{3} \)

   Thus, \( \frac{2}{\sqrt{12}} = \frac{2}{2\sqrt{3}} = \frac{1}{\sqrt{3}} \). To rationalize:

   \( \frac{1}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{\sqrt{3}}{3} \).

3. Simplify the Fraction:

   If possible, further simplify the fraction by reducing it to its lowest terms.

   For example, simplify \( \frac{4\sqrt{2}}{2} \):

   \( \frac{4\sqrt{2}}{2} = 2\sqrt{2} \).

Examples:

• Example 1: Simplify \( \frac{\sqrt{50}}{2} \)

\( \sqrt{50} = \sqrt{25 \times 2} = 5\sqrt{2} \)

So, \( \frac{\sqrt{50}}{2} = \frac{5\sqrt{2}}{2} \).

• Example 2: Simplify \( \frac{3\sqrt{8}}{4} \)

\( \sqrt{8} = \sqrt{4 \times 2} = 2\sqrt{2} \)

Thus, \( \frac{3\sqrt{8}}{4} = \frac{3 \times 2\sqrt{2}}{4} = \frac{6\sqrt{2}}{4} = \frac{3\sqrt{2}}{2} \).

Practice these steps with different fractions to become proficient in simplifying fractions with square roots in the numerator.

Complex fractions are fractions where the numerator, the denominator, or both contain a fraction themselves. When these fractions involve square roots, the simplification process can be more intricate. Here are steps and methods to simplify complex fractions involving square roots:

1. Simplify the Numerator and Denominator:

   Start by simplifying the fractions within the numerator and the denominator separately. If there are any common factors, cancel them out.

   Example:

   \[
   \frac{\frac{\sqrt{2}}{3} + \frac{1}{\sqrt{5}}}{\frac{2}{\sqrt{7}} - \frac{\sqrt{3}}{4}}
   \]

   Simplify each part:

   • Numerator: \(\frac{\sqrt{2}}{3} + \frac{1}{\sqrt{5}}\)
   • Denominator: \(\frac{2}{\sqrt{7}} - \frac{\sqrt{3}}{4}\)

2. Find a Common Denominator:

   Find a common denominator for the fractions within the numerator and the denominator to combine them into single fractions.

   Example:

   Numerator: \(\frac{\sqrt{2}\sqrt{5} + 3}{3\sqrt{5}}\)

   Denominator: \(\frac{8 - \sqrt{21}}{4\sqrt{7}}\)

3. Combine and Simplify:

   Combine the fractions in the numerator and the denominator:

   Example:

   \[
   \frac{\frac{\sqrt{2}\sqrt{5} + 3}{3\sqrt{5}}}{\frac{8 - \sqrt{21}}{4\sqrt{7}}}
   \]

   Simplify by multiplying the numerator by the reciprocal of the denominator:

   \[
   \left(\frac{\sqrt{2}\sqrt{5} + 3}{3\sqrt{5}}\right) \times \left(\frac{4\sqrt{7}}{8 - \sqrt{21}}\right)
   \]

4. Rationalize the Denominator:

   If the final expression has a square root in the denominator, rationalize it by multiplying by the conjugate of the denominator.

   Example:

   To rationalize \(\frac{4\sqrt{7}}{8 - \sqrt{21}}\), multiply by the conjugate \(\frac{8 + \sqrt{21}}{8 + \sqrt{21}}\):

   \[
   \frac{4\sqrt{7} \cdot (8 + \sqrt{21})}{(8 - \sqrt{21})(8 + \sqrt{21})}
   \]

   Simplify the numerator and the denominator:

   Numerator: \(4\sqrt{7}(8 + \sqrt{21})\)

   Denominator: \((8)^2 - (\sqrt{21})^2 = 64 - 21 = 43\)

5. Simplify the Final Expression:

   Combine all parts and simplify to get the final expression.

   Example:

   \[
   \frac{4\sqrt{7} (8 + \sqrt{21})}{43}
   \]

   This is the simplified form of the original complex fraction.

In this section, we will go through detailed examples to demonstrate the process of simplifying fractions involving square roots.

Example 1: Simplify \( \frac{3\sqrt{7}}{\sqrt{14}} \)

1. Step 1: Simplify the expression

   First, rewrite the fraction:

   \( \frac{3\sqrt{7}}{\sqrt{14}} = \frac{3\sqrt{7}}{\sqrt{2 \cdot 7}} = \frac{3\sqrt{7}}{\sqrt{2} \cdot \sqrt{7}} \)

   The \( \sqrt{7} \) terms cancel out:

   \( \frac{3\sqrt{7}}{\sqrt{2}\sqrt{7}} = \frac{3}{\sqrt{2}} \)

2. Step 2: Rationalize the denominator

   To eliminate the square root in the denominator, multiply both the numerator and the denominator by \( \sqrt{2} \):

   \( \frac{3}{\sqrt{2}} \times \frac{\sqrt{2}}{\sqrt{2}} = \frac{3\sqrt{2}}{2} \)

   So, \( \frac{3\sqrt{7}}{\sqrt{14}} \) simplifies to \( \frac{3\sqrt{2}}{2} \).

Example 2: Simplify \( \frac{\sqrt{15} - 2}{\sqrt{3} + 1} \)

1. Step 1: Rationalize the denominator

   To eliminate the square root in the denominator, multiply both the numerator and the denominator by the conjugate of the denominator:

   \( \frac{\sqrt{15} - 2}{\sqrt{3} + 1} \times \frac{\sqrt{3} - 1}{\sqrt{3} - 1} = \frac{(\sqrt{15} - 2)(\sqrt{3} - 1)}{(\sqrt{3} + 1)(\sqrt{3} - 1)} \)

   Expand and simplify the numerator and denominator:

   Numerator: \( (\sqrt{15} - 2)(\sqrt{3} - 1) = \sqrt{45} - \sqrt{15} - 2\sqrt{3} + 2 = 3\sqrt{5} - \sqrt{15} - 2\sqrt{3} + 2 \)

   Denominator: \( (\sqrt{3})^2 - 1^2 = 3 - 1 = 2 \)

   The fraction becomes:

   \( \frac{3\sqrt{5} - \sqrt{15} - 2\sqrt{3} + 2}{2} \)

2. Step 2: Simplify the expression

   Divide each term in the numerator by the denominator:

   \( \frac{3\sqrt{5}}{2} - \frac{\sqrt{15}}{2} - \frac{2\sqrt{3}}{2} + \frac{2}{2} \)

   Which simplifies to:

   \( \frac{3\sqrt{5}}{2} - \frac{\sqrt{15}}{2} - \sqrt{3} + 1 \)

Example 3: Simplify \( \frac{\sqrt{18}}{\sqrt{2}} \)

1. Step 1: Simplify the expression

   First, combine the square roots:

   \( \frac{\sqrt{18}}{\sqrt{2}} = \sqrt{\frac{18}{2}} = \sqrt{9} = 3 \)

   So, \( \frac{\sqrt{18}}{\sqrt{2}} \) simplifies to \( 3 \).

These examples demonstrate the process of simplifying square root fractions step-by-step, including rationalizing the denominator when necessary.

When simplifying square root fractions, it's easy to make mistakes that can lead to incorrect results. Here are some common errors and tips on how to avoid them:

• Misunderstanding the square root of a fraction:

  One common mistake is thinking that the square root of a fraction is the same as the square root of the numerator divided by the square root of the denominator. While this is true, it must be handled carefully. For example, \(\sqrt{\frac{4}{9}} = \frac{\sqrt{4}}{\sqrt{9}} = \frac{2}{3}\) .

• Not simplifying the square root first:

  Before rationalizing the denominator, simplify the square root if possible. For instance, \(\sqrt{50}\) should be simplified to \(\sqrt{25 \times 2} = 5\sqrt{2}\) first.

• Incorrectly rationalizing the denominator:

  Rationalizing the denominator involves removing the square root from the denominator by multiplying by the conjugate. For example, to simplify \(\frac{5}{\sqrt{3}}\) , multiply by \(\frac{\sqrt{3}}{\sqrt{3}}\) to get \(\frac{5\sqrt{3}}{3}\) .

• Ignoring perfect square factors:

  Always look for perfect square factors in the numerator and denominator. For instance, in the fraction \(\frac{\sqrt{18}}{3}\) , simplify \(\sqrt{18} = \sqrt{9 \times 2} = 3\sqrt{2}\) to get \(\frac{3\sqrt{2}}{3} = \sqrt{2}\) .

• Forgetting to simplify the fraction:

  After rationalizing and simplifying the square roots, always simplify the resulting fraction. For example, \(\frac{4\sqrt{2}}{2}\) should be simplified to \(\frac{4}{2} \sqrt{2} = 2\sqrt{2}\) .

By keeping these common mistakes in mind and following these tips, you can simplify square root fractions accurately and efficiently.

Practice is essential for mastering the simplification of square root fractions. Here are some problems to help you practice:

1. Simplify the following square root fractions:

   • \(\sqrt{\frac{25}{49}}\)
   • \(\frac{3}{\sqrt{12}}\)
   • \(\frac{\sqrt{18}}{6}\)
   • \(\frac{\sqrt{50}}{\sqrt{2}}\)
   • \(\frac{2\sqrt{6}}{\sqrt{3}}\)

2. Simplify the following mixed fractions with square roots:

   • \(\sqrt{1 \frac{13}{36}}\)
   • \(\sqrt{3 \frac{4}{25}}\)

3. Simplify the following fractions by rationalizing the denominator:

   • \(\frac{5}{\sqrt{3}}\)
   • \(\frac{4}{\sqrt{8}}\)
   • \(\frac{2}{\sqrt{5} + 1}\)

Solutions:

1. • \(\sqrt{\frac{25}{49}} = \frac{5}{7}\)
   • \(\frac{3}{\sqrt{12}} = \frac{3\sqrt{3}}{6} = \frac{\sqrt{3}}{2}\)
   • \(\frac{\sqrt{18}}{6} = \frac{3\sqrt{2}}{6} = \frac{\sqrt{2}}{2}\)
   • \(\frac{\sqrt{50}}{\sqrt{2}} = \sqrt{\frac{50}{2}} = \sqrt{25} = 5\)
   • \(\frac{2\sqrt{6}}{\sqrt{3}} = 2\sqrt{2}\)
2. • \(\sqrt{1 \frac{13}{36}} = \sqrt{\frac{49}{36}} = \frac{7}{6}\)
   • \(\sqrt{3 \frac{4}{25}} = \sqrt{\frac{79}{25}} = \frac{\sqrt{79}}{5}\)
3. • \(\frac{5}{\sqrt{3}} = \frac{5\sqrt{3}}{3}\)
   • \(\frac{4}{\sqrt{8}} = \frac{4\sqrt{2}}{4} = \sqrt{2}\)
   • \(\frac{2}{\sqrt{5} + 1} = \frac{2(\sqrt{5} - 1)}{(\sqrt{5} + 1)(\sqrt{5} - 1)} = \frac{2(\sqrt{5} - 1)}{5 - 1} = \frac{2(\sqrt{5} - 1)}{4} = \frac{\sqrt{5} - 1}{2}\)

Continue practicing with more problems to build confidence and proficiency in simplifying square root fractions. The more you practice, the more intuitive these steps will become!

When dealing with complex fractions involving square roots, advanced techniques can simplify the process. Here are some methods:

• Distributive Property: Apply the distributive property to multiply numerators and denominators in complex fractions, simplifying the expression before addressing the square roots.
• Leveraging Conjugates: For fractions with square roots in the denominator, use the conjugate of the denominator to rationalize it. Multiply both the numerator and the denominator by the conjugate to eliminate the square root.
• Least Common Denominator (LCD): When combining fractions with different denominators, find the LCD to merge them into a single fraction, which can simplify the process of dealing with square roots.
• Factorization: Deeply factorize the square roots into their prime factors to identify and simplify perfect square factors more easily, reducing the complexity of the fraction.
• Division by a Common Factor: After simplifying the square roots and rationalizing the denominator, always look for a common factor that can be divided out of both the numerator and the denominator to further simplify the fraction.

Example

Consider the fraction:

\[\frac{\sqrt{50}}{\sqrt{2}}\]

1. Factorize the numerator: \(\sqrt{50} = \sqrt{25 \times 2} = 5\sqrt{2}\).
2. Simplify the fraction: \(\frac{5\sqrt{2}}{\sqrt{2}} = 5\).

These advanced techniques enhance problem-solving skills and prepare you for more complex mathematical challenges.

Simplified square root fractions are widely used in various fields. Here are some applications:

• Mathematics and Algebra:

  Simplifying square root fractions is essential for solving algebraic equations, particularly those involving quadratic formulas and other polynomial expressions. For example, when dealing with quadratic equations, the solutions often involve square roots that need to be simplified to find the most concise form of the answer.

• Physics:

  In physics, many formulas involve square roots. For instance, calculating the root mean square (RMS) value of alternating current (AC) voltages and currents requires the use of square root fractions. Simplifying these fractions helps in obtaining precise and manageable results.

• Engineering:

  Engineers often encounter square root fractions when analyzing systems and materials. For example, the natural frequency of mechanical systems and the stress analysis in materials involve square roots that need to be simplified for accurate computations.

• Computer Science:

  Algorithms involving numerical methods, such as those used in graphics rendering and scientific computations, frequently require the simplification of square root fractions to optimize performance and accuracy.

• Finance:

  In finance, the calculation of standard deviation and other statistical measures involves square root operations. Simplifying these fractions is crucial for accurate risk assessment and investment analysis.

• Statistics:

  Simplified square root fractions are used in statistical formulas, such as those calculating variance and standard deviation, to make the results more interpretable and easier to work with.

Overall, the ability to simplify square root fractions is a fundamental skill that aids in clearer, more concise mathematical expressions across various scientific and practical applications.

Simplifying square root fractions can initially seem challenging, but with a systematic approach and practice, it becomes manageable and even intuitive. Throughout this guide, we have covered various methods and techniques to simplify square root fractions, including:

• Understanding the Basics: Grasping the fundamental concepts of square roots and fractions.
• Simplifying Square Roots: Breaking down the square root into its simplest form by factoring out perfect squares.
• Rationalizing the Denominator: Removing square roots from the denominator by multiplying by the conjugate or an appropriate value.
• Combining Like Terms: Simplifying the expression by combining terms involving the same square root.
• Advanced Techniques: Applying more complex methods for special cases, such as nested radicals or higher-order roots.

Each of these steps builds upon the previous ones, creating a comprehensive approach to tackling square root fractions. By consistently applying these methods, students can simplify fractions with square roots efficiently and accurately.

It's important to practice these techniques regularly to become proficient. Working through various examples and exercises helps solidify the understanding and application of these methods.

In summary, simplifying square root fractions is a valuable skill in mathematics, useful in various applications such as solving equations, evaluating expressions, and working with geometric problems. With dedication and practice, anyone can master the process and confidently simplify square root fractions.

We hope this guide has been helpful in your journey to understanding and simplifying square root fractions. Keep practicing, and you'll find that these problems become easier and more intuitive over time.