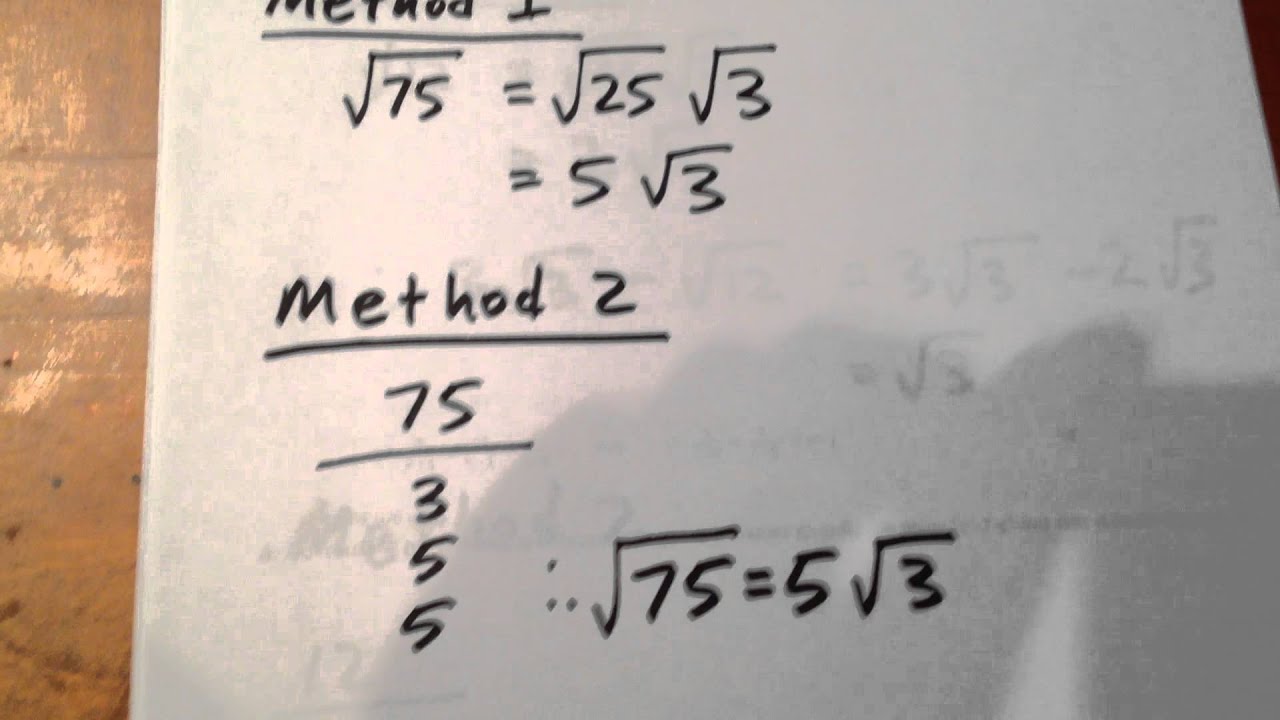Topic simplifying square root of fractions: Understanding how to simplify square roots of fractions is an essential skill in mathematics. This guide will walk you through the basic concepts, steps, and common mistakes to avoid, ensuring you can confidently tackle any square root fraction problem. With examples, practice problems, and helpful tips, you'll master this topic in no time.
Table of Content
- Simplifying the Square Root of Fractions
- Introduction to Simplifying Square Root of Fractions
- Basic Concepts and Terminology
- Steps to Simplify Square Roots of Fractions
- Examples of Simplifying Square Roots of Fractions
- Rationalizing the Denominator
- Special Cases and Complex Fractions
- Practice Problems and Solutions
- Additional Tips and Tricks
- Common Mistakes to Avoid
- Tools and Calculators for Simplifying Fractions
- Further Reading and Resources
- YOUTUBE: Video này hướng dẫn cách đơn giản hóa một phân số dưới căn bậc hai với những mẹo và thủ thuật hợp pháp giúp bạn học toán dễ dàng hơn.
Simplifying the Square Root of Fractions
Understanding how to simplify the square root of fractions can make complex expressions much easier to handle. This process involves a few clear steps to ensure that the fraction is reduced to its simplest form.
Step-by-Step Simplification Process
-
Simplify the Square Root
First, simplify the square roots in both the numerator and the denominator. Look for the largest perfect square factor of the radicand (the number inside the square root). For example:
\[\sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2}\]
-
Convert to Fraction Form
Rewrite the expression so that it is in the form of a fraction where both the numerator and the denominator are simplified square roots:
\[\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\]
-
Rationalize the Denominator
Eliminate any square roots in the denominator by multiplying both the numerator and the denominator by the conjugate of the denominator. This step ensures that the denominator is a rational number:
Example: \(\frac{5}{\sqrt{3}} \rightarrow \frac{5 \times \sqrt{3}}{\sqrt{3} \times \sqrt{3}} = \frac{5\sqrt{3}}{3}\)
-
Simplify the Result
Finally, simplify the resulting fraction if possible. This may involve further reducing the fraction to its simplest terms:
Example: \(\frac{\sqrt{18}}{3} \rightarrow \frac{3\sqrt{2}}{3} = \sqrt{2}\)
Examples
-
Simplify: \(\sqrt{\frac{18}{50}}\)
Solution: \(\sqrt{\frac{9 \times 2}{25}} = \frac{\sqrt{9} \times \sqrt{2}}{\sqrt{25}} = \frac{3\sqrt{2}}{5}\)
-
Simplify: \(\frac{2}{\sqrt{12}}\)
Solution: \(\frac{2}{\sqrt{4 \times 3}} = \frac{2}{2\sqrt{3}} = \frac{1}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{\sqrt{3}}{3}\)
-
Simplify: \(\frac{\sqrt{6}}{\sqrt{2}}\)
Solution: \(\frac{\sqrt{6}}{\sqrt{2}} = \sqrt{\frac{6}{2}} = \sqrt{3}\)
Practice Problems
- Simplify: \(\frac{\sqrt{27}}{\sqrt{3}}\)
- Simplify: \(\frac{4}{\sqrt{20}}\)
- Simplify: \(\sqrt{\frac{50}{2}}\)
By mastering these steps, you will be able to simplify any fraction with a square root, making your calculations more manageable and accurate.

READ MORE:
Introduction to Simplifying Square Root of Fractions
Simplifying the square root of fractions can seem challenging at first, but with a systematic approach, it becomes manageable. The process involves two primary steps: simplifying the square root itself and rationalizing the denominator. This introduction will guide you through the fundamental concepts and techniques needed to master this skill.
When you encounter a fraction under a square root, the goal is to simplify it to its lowest terms. This often involves breaking down the numerator and denominator into their prime factors and simplifying any perfect squares. Let's explore the steps in detail.
Step-by-Step Process
-
Identify and Simplify Square Roots:
First, look at the square roots in both the numerator and the denominator. Simplify them by factoring out any perfect squares.
For example:
- \(\sqrt{72} = \sqrt{36 \times 2} = 6\sqrt{2}\)
- \(\sqrt{50} = \sqrt{25 \times 2} = 5\sqrt{2}\)
-
Simplify the Fraction:
Once the square roots are simplified, write the fraction in its simplest form by dividing the simplified numerator by the simplified denominator.
Example:
- \(\frac{\sqrt{18}}{3} = \frac{3\sqrt{2}}{3} = \sqrt{2}\)
-
Rationalize the Denominator:
If the denominator contains a square root, rationalize it by multiplying both the numerator and the denominator by the conjugate of the denominator. This eliminates the square root from the denominator.
Example:
- \(\frac{5}{\sqrt{3}} = \frac{5 \times \sqrt{3}}{\sqrt{3} \times \sqrt{3}} = \frac{5\sqrt{3}}{3}\)
Example
Consider the fraction \(\frac{\sqrt{48}}{\sqrt{3}}\):
- Simplify the numerator: \(\sqrt{48} = \sqrt{16 \times 3} = 4\sqrt{3}\)
- Simplify the fraction: \(\frac{4\sqrt{3}}{\sqrt{3}} = 4\)
By practicing these steps, simplifying square roots of fractions will become more intuitive. Continue to practice with different examples to solidify your understanding.
Basic Concepts and Terminology
Simplifying the square root of fractions involves understanding key concepts and terminology. Below are some fundamental principles to grasp:
- Radicand: The number or expression inside the square root symbol.
- Square Root: A value that, when multiplied by itself, gives the radicand. Represented as \( \sqrt{x} \).
- Fraction: A mathematical expression representing the division of one quantity by another, expressed as \( \frac{a}{b} \).
When simplifying square roots of fractions, there are essential properties to use:
- Product Property: \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \).
- Quotient Property: \( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \) (for \( b \neq 0 \)).
To simplify a square root involving fractions, follow these steps:
- Identify Perfect Squares: Look for perfect squares in the numerator and the denominator.
- Apply the Quotient Property: Use \( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \).
- Simplify: Reduce the fraction by taking the square root of the numerator and the denominator.
For example:
\[
\sqrt{\frac{49}{36}} = \frac{\sqrt{49}}{\sqrt{36}} = \frac{7}{6}
\]
Special cases may involve mixed numbers or require rationalizing the denominator. Understanding these basic concepts and terminology is crucial for mastering the simplification of square roots of fractions.
Steps to Simplify Square Roots of Fractions
To simplify the square root of a fraction, follow these detailed steps:
-
Convert the Expression into Fraction Form
The square root of a fraction is equal to the square root of the numerator divided by the square root of the denominator:
\(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\)
-
Find the Simplified Forms of the Numerator and Denominator
Simplify the numerator and the denominator separately. For instance, if \(a = 50\) and \(b = 18\), find the square root of each:
\(\sqrt{50} = \sqrt{25 \cdot 2} = 5\sqrt{2}\)
\(\sqrt{18} = \sqrt{9 \cdot 2} = 3\sqrt{2}\)
-
Simplify the Radicands
Combine the simplified numerator and denominator:
\(\frac{5\sqrt{2}}{3\sqrt{2}}\)
Since \(\sqrt{2}\) in the numerator and denominator cancel each other out, the expression simplifies to:
\(\frac{5}{3}\)
-
Rationalize the Denominator (if necessary)
If the denominator is not a rational number, multiply the numerator and the denominator by the conjugate of the denominator to rationalize it.
For example, if the simplified expression was \(\frac{\sqrt{2}}{3}\), you would multiply by \(\sqrt{3}\) to get:
\(\frac{\sqrt{2} \cdot \sqrt{3}}{3 \cdot \sqrt{3}} = \frac{\sqrt{6}}{3}\)
-
Simplify the Resulting Fraction
Ensure the fraction is in its simplest form by dividing the numerator and the denominator by their greatest common divisor (GCD).
By following these steps, you can simplify any square root of a fraction effectively.
Examples of Simplifying Square Roots of Fractions
Simplifying square roots of fractions involves breaking down the fraction into simpler forms. Here are some detailed examples to guide you through the process:
-
Example 1: Simplify \( \frac{\sqrt{18}}{3} \)
- Simplify the square root: \( \sqrt{18} = \sqrt{9 \times 2} = 3\sqrt{2} \)
- Simplify the fraction: \( \frac{3\sqrt{2}}{3} = \sqrt{2} \)
- So, \( \frac{\sqrt{18}}{3} \) simplifies to \( \sqrt{2} \).
-
Example 2: Simplify \( \frac{2}{\sqrt{12}} \)
- Simplify the square root: \( \sqrt{12} = \sqrt{4 \times 3} = 2\sqrt{3} \)
- Rationalize the denominator: \( \frac{2}{2\sqrt{3}} = \frac{2\sqrt{3}}{6} = \frac{\sqrt{3}}{3} \)
- So, \( \frac{2}{\sqrt{12}} \) simplifies to \( \frac{\sqrt{3}}{3} \).
-
Example 3: Simplify \( \frac{\sqrt{6}}{\sqrt{2}} \)
- Combine the square roots: \( \frac{\sqrt{6}}{\sqrt{2}} = \sqrt{\frac{6}{2}} = \sqrt{3} \)
- So, \( \frac{\sqrt{6}}{\sqrt{2}} \) simplifies to \( \sqrt{3} \).
-
Example 4: Simplify \( \frac{3\sqrt{5}}{5} \)
- Simplify the fraction: \( \frac{3\sqrt{5}}{5} = \frac{3}{5} \sqrt{5} \)
- So, \( \frac{3\sqrt{5}}{5} \) simplifies to \( \frac{3}{5} \sqrt{5} \).
-
Example 5: Simplify \( \frac{4}{\sqrt{18}} \)
- Simplify the square root: \( \sqrt{18} = \sqrt{9 \times 2} = 3\sqrt{2} \)
- Rationalize the denominator: \( \frac{4}{3\sqrt{2}} = \frac{4\sqrt{2}}{6} = \frac{2\sqrt{2}}{3} \)
- So, \( \frac{4}{\sqrt{18}} \) simplifies to \( \frac{2\sqrt{2}}{3} \).
With practice, simplifying square roots of fractions becomes easier. Use these examples as a guide and try more on your own!

Rationalizing the Denominator
Rationalizing the denominator is an essential step in simplifying square roots of fractions. This process involves eliminating any radical expressions in the denominator by multiplying both the numerator and the denominator by a suitable value. Here's a detailed guide on how to rationalize the denominator:
-
Identify the radical in the denominator. For instance, consider the fraction:
\[\frac{\sqrt{a}}{b}\]
where \(a\) and \(b\) are any integers.
-
Multiply both the numerator and the denominator by the radical present in the denominator. For the example above, multiply by \(\sqrt{b}\):
\[\frac{\sqrt{a}}{b} \times \frac{\sqrt{b}}{\sqrt{b}} = \frac{\sqrt{a} \cdot \sqrt{b}}{b \cdot \sqrt{b}} = \frac{\sqrt{ab}}{b\sqrt{b}}\]
-
Simplify the fraction. The denominator now becomes \(b\sqrt{b}\), which can be further simplified to:
\[\frac{\sqrt{ab}}{b}\]
-
If the denominator is a binomial, such as \(\frac{1}{a + \sqrt{b}}\), multiply by the conjugate of the denominator. The conjugate of \(a + \sqrt{b}\) is \(a - \sqrt{b}\):
\[\frac{1}{a + \sqrt{b}} \times \frac{a - \sqrt{b}}{a - \sqrt{b}} = \frac{a - \sqrt{b}}{a^2 - b}\]
This eliminates the radical in the denominator.
-
Combine like terms and simplify the numerator and denominator if possible.
Let's see a couple of examples to illustrate these steps:
-
Example 1: Rationalize \(\frac{2}{\sqrt{3}}\)
Multiply by \(\sqrt{3}/\sqrt{3}\):
\[\frac{2}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{2\sqrt{3}}{3}\]
-
Example 2: Rationalize \(\frac{3}{2 + \sqrt{5}}\)
Multiply by the conjugate \(2 - \sqrt{5}\):
\[\frac{3}{2 + \sqrt{5}} \times \frac{2 - \sqrt{5}}{2 - \sqrt{5}} = \frac{3(2 - \sqrt{5})}{(2)^2 - (\sqrt{5})^2} = \frac{6 - 3\sqrt{5}}{4 - 5} = \frac{6 - 3\sqrt{5}}{-1} = -6 + 3\sqrt{5}\]
By rationalizing the denominator, we ensure that our fractions are in their simplest and most understandable form, free from radicals in the denominator.
Special Cases and Complex Fractions
When simplifying square roots of fractions, certain special cases and complex fractions require unique approaches. Here, we explore these scenarios in detail:
Special Cases
- Perfect Square Numerators and Denominators: When both the numerator and the denominator are perfect squares, simplifying is straightforward: \[ \sqrt{\frac{a^2}{b^2}} = \frac{\sqrt{a^2}}{\sqrt{b^2}} = \frac{a}{b} \]
- Square Root of One: Since the square root of one is one, any fraction where the numerator or denominator is one can be simplified accordingly: \[ \sqrt{\frac{1}{b}} = \frac{1}{\sqrt{b}} \]
- Numerator is Zero: If the numerator is zero, the entire expression is zero regardless of the denominator: \[ \sqrt{\frac{0}{b}} = 0 \]
Complex Fractions
Complex fractions are fractions where the numerator, denominator, or both are also fractions. Simplifying these involves a few more steps:
- Identify the Least Common Denominator (LCD): Find the LCD of all the fractions involved.
- Rewrite Each Fraction: Rewrite each fraction so that they share the common denominator.
-
Simplify the Fraction: Simplify by combining the fractions:
\[ \frac{\frac{a}{b}}{\frac{c}{d}} = \frac{a \cdot d}{b \cdot c} \] For example: \[ \frac{\frac{1}{2}}{\frac{3}{4}} = \frac{1 \cdot 4}{2 \cdot 3} = \frac{4}{6} = \frac{2}{3} \] - Simplify the Resulting Fraction: Once simplified, take the square root of the fraction as needed.
By understanding these special cases and the process for simplifying complex fractions, you can handle a variety of problems involving square roots of fractions with confidence.
Practice Problems and Solutions
Sharpen your skills in simplifying square roots with fractions through these practice problems. Solutions are provided to help you verify your answers and understand the process of simplification in detail.
-
Problem 1: Simplify \( \frac{\sqrt{50}}{\sqrt{2}} \)
Solution: Factor \( \sqrt{50} \) into \( \sqrt{25 \cdot 2} = 5\sqrt{2} \). Then, \( \frac{5\sqrt{2}}{\sqrt{2}} = 5 \).
-
Problem 2: Simplify \( \frac{1}{\sqrt{3}} \)
Solution: Rationalize the denominator by multiplying the numerator and denominator by \( \sqrt{3} \), resulting in \( \frac{\sqrt{3}}{3} \).
-
Problem 3: Simplify \( \frac{\sqrt{18} + \sqrt{2}}{\sqrt{9}} \)
Solution: Simplify \( \sqrt{18} \) to \( 3\sqrt{2} \) and \( \sqrt{9} \) to \( 3 \). The expression becomes \( \frac{3\sqrt{2} + \sqrt{2}}{3} = \frac{4\sqrt{2}}{3} \).
-
Problem 4: Simplify \( \frac{2\sqrt{5}}{\sqrt{20}} \)
Solution: Simplify \( \sqrt{20} \) to \( 2\sqrt{5} \). The expression becomes \( \frac{2\sqrt{5}}{2\sqrt{5}} = 1 \).
-
Problem 5: Simplify \( \frac{\sqrt{72}}{\sqrt{8}} \)
Solution: Simplify \( \sqrt{72} \) to \( 6\sqrt{2} \) and \( \sqrt{8} \) to \( 2\sqrt{2} \). The expression becomes \( \frac{6\sqrt{2}}{2\sqrt{2}} = 3 \).
Additional Tips and Tricks
Simplifying square roots of fractions can be made easier with some helpful tips and tricks:
- Factor Perfect Squares: When possible, factor the numerator and denominator into perfect squares to simplify the square root more easily. For example,
\(\sqrt{\frac{50}{18}}\) can be simplified by first converting it to \(\sqrt{\frac{25 \cdot 2}{9 \cdot 2}} = \frac{\sqrt{25}}{\sqrt{9}} = \frac{5}{3}\). - Quotient Property: Utilize the quotient property of square roots, which states that
\(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\).This is particularly useful for simplifying complex fractions. - Rationalize the Denominator: If the denominator is irrational, multiply both the numerator and denominator by the same square root to make the denominator rational. For example,
\(\frac{1}{\sqrt{2}}\) can be rationalized to \(\frac{\sqrt{2}}{\sqrt{2} \cdot \sqrt{2}} = \frac{\sqrt{2}}{2}\). - Estimate Non-Perfect Squares: When dealing with non-perfect squares, estimate their square roots by finding the closest perfect squares. For example, the square root of 5 is between 2 and 3, closer to 2.2.
- Mixed Numbers: Convert mixed numbers to improper fractions before simplifying. For instance,
\(\sqrt{1 \frac{13}{36}}\) should be converted to \(\sqrt{\frac{49}{36}} = \frac{\sqrt{49}}{\sqrt{36}} = \frac{7}{6}\). - Use Technology: Tools like online calculators and apps can simplify the process and verify your results. Utilize these resources for complex calculations.
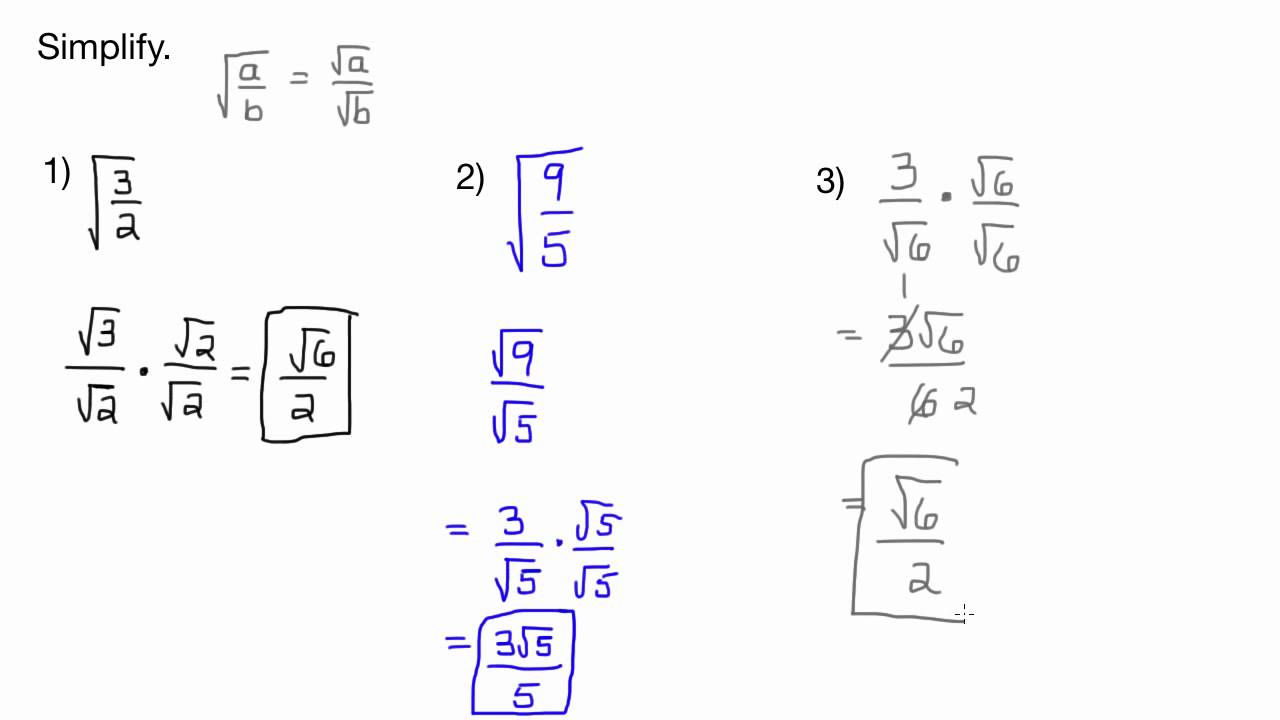
Common Mistakes to Avoid
When simplifying square roots of fractions, several common mistakes can lead to incorrect results. Here are some of the most frequent errors and how to avoid them:
- Not Simplifying the Square Root Completely: Ensure that you simplify the square root as much as possible. Use prime factorization to find the largest perfect square factor. For example, √72 can be simplified to 6√2 because 72 = 36 × 2 and √36 = 6.
- Incorrect Rationalization of the Denominator: When rationalizing the denominator, remember to multiply both the numerator and the denominator by the conjugate or the necessary square root to eliminate the square root from the denominator completely. For instance, to rationalize 5/√3, multiply by √3/√3 to get 5√3/3.
- Forgetting to Simplify the Fraction: After simplifying the square root and rationalizing the denominator, always check if the fraction itself can be simplified further by reducing it to its lowest terms. For example, (6√2)/12 can be simplified to √2/2.
- Mixing Up Operations: Keep the order of operations in mind. Simplify square roots before performing operations with fractions, and be careful with multiplication and division steps to avoid mistakes.
- Overlooking Negative Signs: Pay attention to negative signs, especially when dealing with square roots. A negative square root can significantly change the problem’s outcome. For example, -√4 is -2, not 2.
By being mindful of these common mistakes and applying the correct simplification techniques, you can confidently simplify square roots in fractions and tackle more complex mathematical problems.
Tools and Calculators for Simplifying Fractions
When simplifying square roots of fractions, several online tools and calculators can assist you in performing these calculations efficiently and accurately. Below is a list of some popular tools and how to use them:
-
Symbolab Square Root Calculator:
This tool allows you to simplify square root expressions step-by-step. You can enter the fraction under the square root, and it will guide you through the simplification process.
- Visit the .
- Enter your square root expression in the input box.
- Click on the "Simplify" button to see the step-by-step solution.
-
MathCracker Square Root Calculator:
This calculator simplifies square roots of both integers and fractions, providing detailed steps and explanations.
- Go to the .
- Input the fraction under the square root you want to simplify.
- Press "Calculate" to get the simplified form along with the steps.
-
Calculator.io Simplify Fractions Tool:
This tool can handle a variety of fraction-related calculations, including simplifying square roots of fractions.
- Access the .
- Enter the fraction in the provided fields.
- Click "Simplify" to see the result and the steps taken to achieve it.
These tools not only simplify the square roots of fractions but also help in understanding the process by providing detailed steps. They are particularly useful for students and educators to verify their work and gain deeper insights into the simplification process.
Further Reading and Resources
For those looking to deepen their understanding of simplifying square roots of fractions, here are some valuable resources:
-
Khan Academy - Simplifying Square Roots:
This comprehensive resource includes detailed video tutorials and practice exercises that cover the basics and more advanced concepts of simplifying square roots, including those involving fractions.
-
Mathematics LibreTexts - Simplifying Square Root Expressions:
Explore in-depth explanations and examples of simplifying square root expressions, focusing on both general and specific cases such as fractions.
-
Interactive Math Websites:
Many educational websites offer interactive tools and calculators that help visualize and simplify square root expressions. These can be particularly useful for practicing different types of problems and checking your work.
-
Algebra Textbooks:
Traditional algebra textbooks often contain thorough sections on square roots and fractions, complete with examples, exercises, and additional practice problems.
-
Online Math Forums:
Engage with communities on platforms like Reddit, Stack Exchange, or specific math forums where you can ask questions, share problems, and get explanations from experienced mathematicians and educators.
-
Educational YouTube Channels:
Channels such as Math Antics and PatrickJMT provide video tutorials that break down complex mathematical concepts into easy-to-understand segments, including square roots and fractions.
By leveraging these resources, you can build a stronger foundation in simplifying square roots of fractions and improve your overall mathematical proficiency.
Video này hướng dẫn cách đơn giản hóa một phân số dưới căn bậc hai với những mẹo và thủ thuật hợp pháp giúp bạn học toán dễ dàng hơn.
Đơn Giản Hóa Một Phân Số Dưới Căn Bậc Hai - Mẹo Học Toán Hợp Pháp
READ MORE:
Đơn Giản Hóa Căn Bậc Hai Của Phân Số: sqrt(a/b), a/sqrt(b)