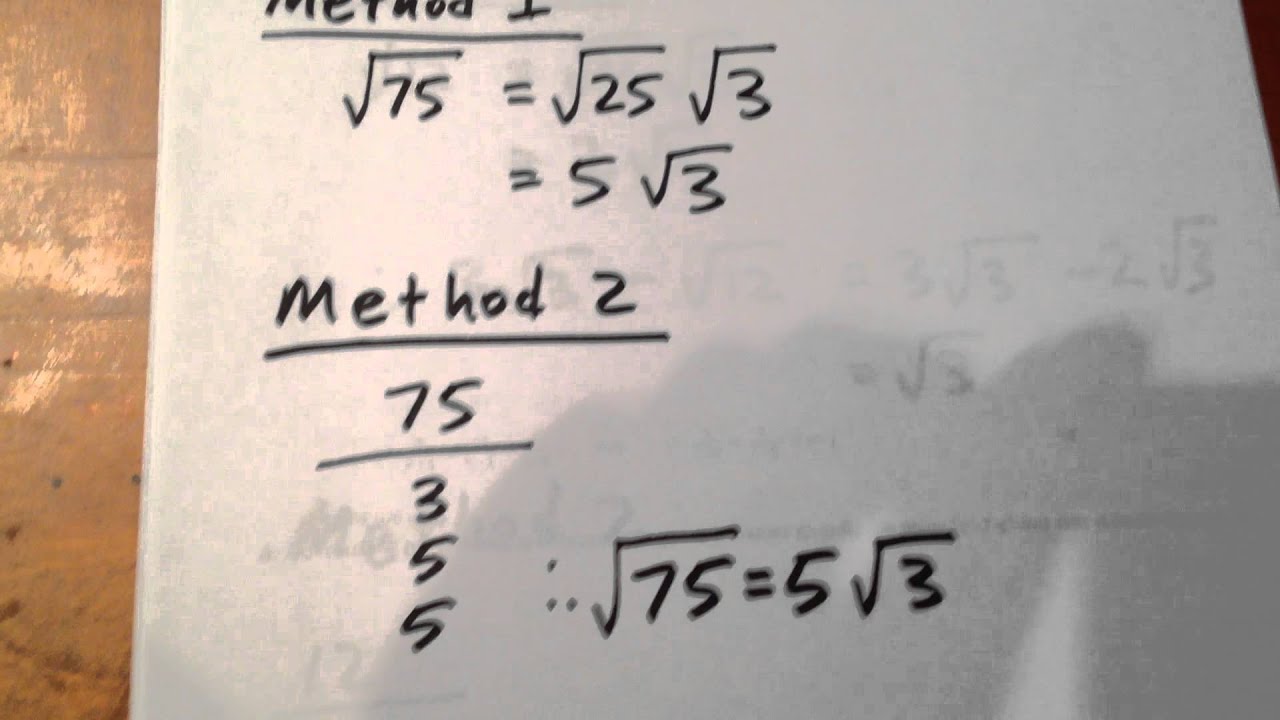Simplify Square Root 36: A Comprehensive Guide

1. Identify Perfect Squares

   First, recognize that 36 is a perfect square. The perfect squares less than or equal to 36 are 1, 4, 9, 16, 25, and 36 itself.

2. Rewrite the Number

   The square root of a perfect square can be rewritten using its square root:
   $$
   
   36
   
   = 6
   

3. Verification

   Verify by squaring the result to see if you get the original number:
   $$
   
   6
   2
   
   = 36
   .

Thus, the square root of 36 is $6$.

• Problem 1

  Jack needs to find the square root of 36 using exponents. The square root can be written as an exponent:
  $$
  
  36
  
  1
  2
  
  
  = 6
  .

• Problem 2

  If a minivan has 36 seats arranged in a square, how many rows are there? Let y be the number of rows:
  $$
  
  y
  2
  
  = 36,
  
  
  y
  2
  
  = 6.
  

By understanding and applying these steps, simplifying the square root of any perfect square, such as 36, becomes an easy task.

• Problem 1

  Jack needs to find the square root of 36 using exponents. The square root can be written as an exponent:
  $$
  
  36
  
  1
  2
  
  
  = 6
  .

• Problem 2

  If a minivan has 36 seats arranged in a square, how many rows are there? Let y be the number of rows:
  $$
  
  y
  2
  
  = 36,
  
  
  y
  2
  
  = 6.
  

By understanding and applying these steps, simplifying the square root of any perfect square, such as 36, becomes an easy task.

A square root of a number is a value that, when multiplied by itself, gives the original number. It is a fundamental concept in mathematics with applications in various fields. Understanding how to simplify square roots can make complex calculations easier and more intuitive.

For instance, the square root of 36 is 6 because 6 × 6 = 36. This process of finding a square root involves identifying the principal (positive) square root for simplicity and consistency.

When simplifying square roots, it is essential to break down the number into its prime factors. For example, to simplify √36, you can express 36 as the product of prime factors:

• 36 = 2 × 2 × 3 × 3

By grouping the prime factors into pairs, you can pull out the square root of each pair:

• √36 = √(2 × 2 × 3 × 3) = √(2^2 × 3^2) = 2 × 3 = 6

This method helps in understanding how to simplify non-perfect square roots as well, by breaking them into the product of smaller numbers and simplifying each part separately. This approach is beneficial in algebra and higher mathematics, where simplifying radicals is a common requirement.

A perfect square is a number that can be expressed as the product of an integer with itself. For example, 36 is a perfect square because it equals \(6 \times 6\). Understanding perfect squares is essential when simplifying square roots, as it allows us to identify when a square root can be simplified to a whole number.

Here are the steps to understand and identify perfect squares:

1. List the factors of the number.
2. Identify which of these factors are perfect squares.
3. Determine if the number itself is a perfect square by checking if it equals the product of an integer with itself.

For example, to determine if 36 is a perfect square:

• List the factors of 36: 1, 2, 3, 4, 6, 9, 12, 18, 36.
• Identify the perfect squares from the list of factors: 1, 4, 9, 36.
• Since 36 is in the list and it equals \(6 \times 6\), we conclude that 36 is a perfect square.

Recognizing perfect squares helps simplify square roots effectively. For instance, the square root of 36 simplifies directly to 6 because 36 is a perfect square.

The process of simplifying the square root of a number involves breaking down the number into its prime factors and identifying any perfect squares. Here are the detailed steps to simplify the square root of 36:

1. Identify the number under the square root symbol: \( \sqrt{36} \).

2. Find the factors of 36: 1, 2, 3, 4, 6, 9, 12, 18, 36.

3. Identify the perfect squares among the factors: 1, 4, 9, 36. Since 36 is a perfect square, it simplifies easily.

4. Rewrite 36 as a product of its prime factors: \( 36 = 6 \times 6 \).

5. Apply the square root to the product: \( \sqrt{36} = \sqrt{6 \times 6} \).

6. Simplify the square root by taking the square root of the perfect square: \( \sqrt{6 \times 6} = 6 \).

Therefore, the simplified form of \( \sqrt{36} \) is \( 6 \).

Prime factorization is a method used to simplify the square root of a number by breaking it down into its prime factors. This method is especially useful for simplifying square roots of perfect squares. Follow these steps to simplify the square root of 36 using prime factorization:

1. Break the number under the square root into its prime factors.
• 36 = 2 × 2 × 3 × 3
2. Group the prime factors into pairs.
• (2 × 2) and (3 × 3)
3. For each pair of prime factors, take one factor out of the square root.
• √(2 × 2) × √(3 × 3) = 2 × 3
4. Multiply the factors outside the square root.
• 2 × 3 = 6
5. The simplified form of the square root of 36 is:
• √36 = 6

Using the prime factorization method helps to simplify square roots efficiently by reducing the number under the square root to its smallest possible factors. This method ensures that the square root is expressed in its simplest form.

The exponent method is an efficient way to simplify square roots by using the property of exponents. Here’s a step-by-step guide to simplify the square root of 36 using this method:

1. Express the Square Root as an Exponent:

   The square root of a number can be written as an exponent of 1/2. Therefore, the square root of 36 is written as:

   \(\sqrt{36} = 36^{1/2}\)

2. Factor the Number:

   Next, factor the number 36 into its prime factors:

   \(36 = 6^2\)

3. Apply the Exponent Rule:

   Apply the rule \((a^m)^n = a^{m \cdot n}\) to simplify the expression:

   \((6^2)^{1/2} = 6^{2 \cdot 1/2} = 6^1\)

4. Simplify:

   Simplify the exponent to get the final answer:

   \(6^1 = 6\)

Thus, the simplified form of \(\sqrt{36}\) using the exponent method is 6.

To verify the simplified square root of 36, we follow a few steps to ensure accuracy:

1. Calculate the Square Root

   First, calculate the square root of 36:

   \(\sqrt{36} = 6\)

   This calculation is based on the fact that 6² = 36.

2. Prime Factorization Verification

   Verify the simplification using prime factorization:

   36 can be factored into prime numbers as follows:

   36 = 2² × 3²

   Taking the square root of both sides, we get:

   \(\sqrt{36} = \sqrt{2^2 \times 3^2} = 2 \times 3 = 6\)

3. Exponent Method Verification

   Using the exponent method, express 36 as an exponent:

   36 = 6²

   The square root of 36 can then be written as:

   \(\sqrt{36} = (6^2)^{1/2} = 6^{2 \times 1/2} = 6^1 = 6\)

4. Double-Check with Calculator

   To further verify, use a calculator to find the square root of 36, which should also return:

   \(\sqrt{36} = 6\)

By following these steps, we confirm that the simplified square root of 36 is indeed 6, verifying the calculation through multiple methods.

Simplifying square roots can be approached using different methods such as factoring out perfect squares or using prime factorization. Here are some examples:

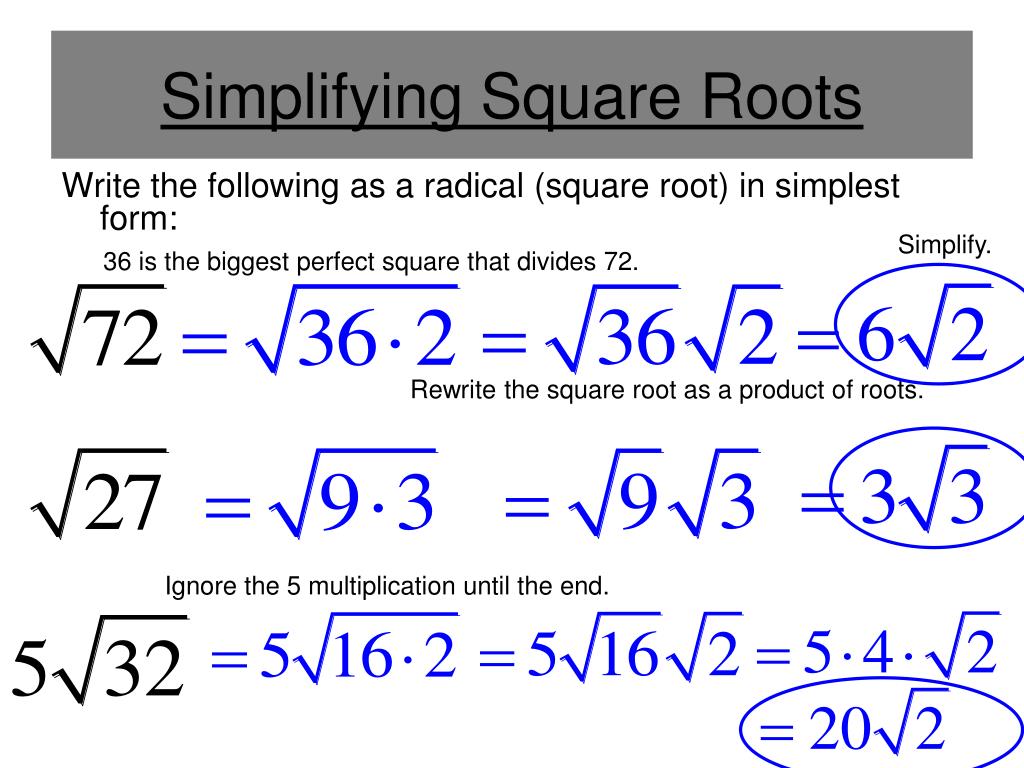
Example 1: Simplifying \(\sqrt{72}\)

1. Factor the number inside the square root into its prime factors:

   \(72 = 2 \times 36\)

2. Recognize that 36 is a perfect square:

   \(\sqrt{72} = \sqrt{2 \times 36} = \sqrt{2} \times \sqrt{36}\)

3. Simplify the square root of the perfect square:

   \(\sqrt{36} = 6\)

4. Combine the results:

   \(\sqrt{72} = 6\sqrt{2}\)

Example 2: Simplifying \(\sqrt{50}\)

1. Factor the number inside the square root into its prime factors:

   \(50 = 2 \times 25\)

2. Recognize that 25 is a perfect square:

   \(\sqrt{50} = \sqrt{2 \times 25} = \sqrt{2} \times \sqrt{25}\)

3. Simplify the square root of the perfect square:

   \(\sqrt{25} = 5\)

4. Combine the results:

   \(\sqrt{50} = 5\sqrt{2}\)

Example 3: Simplifying \(\sqrt{18}\)

1. Factor the number inside the square root into its prime factors:

   \(18 = 2 \times 9\)

2. Recognize that 9 is a perfect square:

   \(\sqrt{18} = \sqrt{2 \times 9} = \sqrt{2} \times \sqrt{9}\)

3. Simplify the square root of the perfect square:

   \(\sqrt{9} = 3\)

4. Combine the results:

   \(\sqrt{18} = 3\sqrt{2}\)

Example 4: Simplifying \(\sqrt{200}\)

1. Factor the number inside the square root into its prime factors:

   \(200 = 2 \times 100\)

2. Recognize that 100 is a perfect square:

   \(\sqrt{200} = \sqrt{2 \times 100} = \sqrt{2} \times \sqrt{100}\)

3. Simplify the square root of the perfect square:

   \(\sqrt{100} = 10\)

4. Combine the results:

   \(\sqrt{200} = 10\sqrt{2}\)

Example 5: Simplifying \(\sqrt{45}\)

1. Factor the number inside the square root into its prime factors:

   \(45 = 3 \times 15\)

2. Recognize that 15 can be factored further:

   \(15 = 3 \times 5\)

3. Combine the factors and look for pairs:

   \(45 = 3 \times 3 \times 5\)

4. Simplify the pairs of factors:

   \(\sqrt{45} = \sqrt{3 \times 3 \times 5} = 3\sqrt{5}\)

Square roots have numerous applications in various fields, demonstrating their importance beyond academic exercises. Here are some practical uses:

• Engineering and Construction:

  Square roots are essential in engineering and construction, especially in calculating dimensions and structural integrity. For instance, the Pythagorean Theorem, which uses square roots, helps in determining the lengths of sides in right-angled triangles. This is crucial in tasks like designing ramps, roofs, and other structural components.

  Formula: \( c = \sqrt{a^2 + b^2} \)

• Finance:

  In finance, square roots help in calculating the rate of return on investments over multiple periods. The compound interest formula often involves square roots to determine the annual growth rate.

  Formula: \( R = \sqrt{\frac{V_2}{V_0}} - 1 \)

• Physics:

  Square roots are frequently used in physics to solve equations related to motion, energy, and wave functions. For example, the distance formula in physics to determine the distance between two points in a plane or space uses square roots.

  Formula in 2D: \( D = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2} \)

  Formula in 3D: \( D = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2 + (z_2 - z_1)^2} \)

• Statistics and Probability:

  Square roots are vital in statistics, particularly in the calculation of standard deviation, which measures the amount of variation or dispersion in a set of values. They also appear in the probability density functions of normal distributions.

  Formula for standard deviation: \( \sigma = \sqrt{\frac{\sum (x_i - \mu)^2}{N}} \)

• Computer Graphics:

  In computer graphics, square roots are used to calculate distances between points, essential for rendering images, animations, and simulations. This helps in creating realistic visual effects and 3D models.

• Architecture:

  Architects use square roots to design buildings and structures, ensuring that dimensions and angles are accurate. The Pythagorean Theorem again plays a crucial role here.

• What is the Value of the Square Root of 36?

  The square root of 36 is 6, because 6 × 6 = 36.

• Why is the Square Root of 36 a Rational Number?

  The square root of 36 is a rational number because it can be expressed as an integer. The prime factorization of 36 is \(2^2 \times 3^2\), both of which are even, making the square root a whole number (6).

• What is the Square Root of -36?

  The square root of -36 is an imaginary number. It is written as \( \sqrt{-36} = 6i \), where \( i \) is the imaginary unit and \( i = \sqrt{-1} \).

• How Do You Simplify Square Roots of Non-Perfect Squares?

  To simplify square roots of non-perfect squares, factor the number under the square root into its prime factors and pair them up. For example, to simplify \( \sqrt{50} \):
  

  
  
  1. Prime factorize 50: \( 50 = 2 \times 5 \times 5 \)
  
  
  2. Group the factors into pairs: \( \sqrt{50} = \sqrt{2 \times 5^2} \)
  
  
  3. Pull out the pairs: \( \sqrt{2 \times 5^2} = 5\sqrt{2} \)
  
  
  
  


• 
  Can the Square Root of 36 Be Negative?

  While the principal square root of 36 is 6, mathematically, both 6 and -6 are square roots of 36 because \( (-6) \times (-6) = 36 \). However, the principal (or default) square root is the positive value.

• How Do Square Roots Apply in Real Life?

  Square roots are used in various fields such as architecture, engineering, and physics to calculate dimensions, areas, and other measurements. For instance, they help in determining the side length of a square when given the area, calculating distances in coordinate geometry, and solving quadratic equations.

Simplifying square roots can sometimes lead to errors if certain common pitfalls are not avoided. Here are some of the most frequent mistakes and how to avoid them:

• Overlooking Perfect Squares: Always check for perfect squares within the radicand. For example, in √36, recognize that 36 is a perfect square (6 * 6), so √36 simplifies directly to 6.
• Misapplying Properties: Incorrectly applying properties such as assuming √a + √b = √(a + b) can lead to errors. Remember, properties like √ab = √a * √b apply to multiplication and division, not addition or subtraction.
• Ignoring Variables: When dealing with expressions that include variables, identify and simplify perfect square variables. For example, √(x^4) simplifies to x^2.
• Simplification Errors: Double-check your arithmetic operations and factorization. Mistakes in these steps can lead to incorrect simplifications. Ensure each step is correct before proceeding to the next.
• Forgetting to Rationalize the Denominator: Leaving a square root in the denominator is often considered unfinished. Make sure to rationalize the denominator. For example, to simplify 1/√2, multiply by √2/√2 to get √2/2.

By being aware of these common mistakes and carefully applying the correct simplification steps, you can improve your mathematical precision and efficiency.

Exploring additional resources and tools can greatly enhance your understanding of square roots and their simplification processes. Below is a list of recommended resources and tools to aid in your learning:

• Online Calculators: Use online calculators to quickly verify your square root calculations. Some popular options include:

• Tutorial Videos: Watch tutorial videos that provide step-by-step instructions on simplifying square roots. Some helpful YouTube channels include:

• Interactive Learning Platforms: Engage with interactive learning platforms to practice simplifying square roots:

• Math Software: Utilize software tools designed for mathematical computations to assist in simplifying square roots:

• Books and E-books: Refer to books and e-books for a more in-depth understanding of square roots and their properties: