Topic simplifying square roots 72: Unlock the secrets of simplifying the square root of 72 with our comprehensive guide. This article breaks down the process step-by-step, making it easy to understand and apply. Whether you're a student or a math enthusiast, you'll find practical tips and clear explanations to help you master this essential math skill.
Table of Content
- Simplifying Square Roots of 72
- Introduction to Square Roots
- Understanding the Concept of Simplification
- Prime Factorization Method
- Step-by-Step Simplification of √72
- Visualizing the Simplification Process
- Mathematical Notation and Explanations
- Common Mistakes and How to Avoid Them
- Practical Examples and Practice Problems
- Applications in Real-World Scenarios
- Additional Resources and Further Reading
- YOUTUBE:
Simplifying Square Roots of 72
The process of simplifying the square root of 72 involves expressing it in its simplest radical form. Below is a detailed explanation of the steps involved:
Steps to Simplify √72
- Prime Factorize 72:
Begin by finding the prime factors of 72. The prime factorization is:
\[72 = 2^3 \times 3^2\]
- Break Down the Square Root:
Use the prime factorization to simplify the square root:
\[\sqrt{72} = \sqrt{2^3 \times 3^2}\]
- Separate into Perfect Squares:
Identify and separate the perfect squares:
\[\sqrt{72} = \sqrt{(2^2 \times 3^2) \times 2} = \sqrt{36 \times 2}\]
- Simplify the Expression:
Simplify the square root by taking the square root of the perfect squares:
\[\sqrt{72} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2}\]
Visual Representation
The simplification process can be visualized as:
| Step | Expression | Simplified Form |
| 1 | \(\sqrt{72}\) | |
| 2 | \(\sqrt{2^3 \times 3^2}\) | |
| 3 | \(\sqrt{36 \times 2}\) | |
| 4 | \(6\sqrt{2}\) |
Conclusion
The simplest form of the square root of 72 is \(6\sqrt{2}\). By following these steps, you can simplify similar square root problems efficiently.
Practice Problems
- Simplify \(\sqrt{98}\)
- Simplify \(\sqrt{50}\)
- Simplify \(\sqrt{200}\)

READ MORE:
Introduction to Square Roots
Square roots are a fundamental concept in mathematics that represent a value which, when multiplied by itself, yields the original number. Understanding square roots is essential for solving various algebraic equations and real-world problems. Below is an introduction to the key concepts and methods for working with square roots.
The square root of a number \( x \) is denoted as \( \sqrt{x} \). It is the inverse operation of squaring, which involves multiplying a number by itself. For example:
\[ \sqrt{25} = 5 \quad \text{because} \quad 5 \times 5 = 25 \]
To gain a deeper understanding of square roots, let's explore their properties and applications:
- Non-negative Results: The principal square root of a non-negative number is always non-negative. For instance, \( \sqrt{49} = 7 \), not \(-7\).
- Perfect Squares: Numbers like 1, 4, 9, 16, and 25 are perfect squares because their square roots are integers.
- Non-perfect Squares: For numbers that are not perfect squares, their square roots are irrational. For example, \( \sqrt{2} \approx 1.414 \) and cannot be expressed exactly as a fraction.
Understanding how to simplify square roots is crucial, especially when dealing with non-perfect squares. Simplifying involves expressing the square root in its simplest form, often using prime factorization. Here's a step-by-step approach:
- Prime Factorization: Break down the number inside the square root into its prime factors. For example:
\[ 72 = 2^3 \times 3^2 \]
- Identify Perfect Squares: Group the prime factors into pairs of squares. For instance:
\[ \sqrt{72} = \sqrt{(2^2 \times 3^2) \times 2} \]
- Simplify: Take the square root of the perfect squares and multiply by the remaining factor:
\[ \sqrt{72} = 6\sqrt{2} \]
These steps form the foundation for simplifying square roots and enable solving more complex mathematical problems efficiently. Understanding square roots and their simplification is a valuable skill that applies across various areas of math and science.
Understanding the Concept of Simplification
Simplifying square roots involves reducing a square root to its simplest form, making it easier to work with in mathematical calculations. This process typically includes identifying and extracting the largest perfect square factor from the number under the square root. Here’s a detailed explanation of the simplification concept:
The goal of simplification is to express the square root in the form \( a\sqrt{b} \), where \( a \) and \( b \) are integers and \( b \) is as small as possible. Simplifying square roots can be broken down into the following steps:
- Prime Factorization:
First, find the prime factors of the number under the square root. Prime factorization involves expressing the number as a product of its prime factors.
For example, to simplify \( \sqrt{72} \):
\[ 72 = 2^3 \times 3^2 \] - Identify and Group Perfect Squares:
Next, group the prime factors into pairs to identify perfect squares. A perfect square is a number that has an integer as its square root.
In the case of \( \sqrt{72} \):
\[ \sqrt{72} = \sqrt{2^3 \times 3^2} \]
Group the factors:
\[ \sqrt{72} = \sqrt{(2^2 \times 3^2) \times 2} = \sqrt{36 \times 2} \] - Simplify the Square Root:
Extract the square roots of the perfect squares and multiply them together, leaving the non-perfect square under the radical.
For \( \sqrt{72} \):
\[ \sqrt{72} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2} \]
This method works for any positive integer under the square root, helping to simplify expressions for easier computation. Let's look at another example to solidify the concept:
Simplify \( \sqrt{50} \):
- Prime factorize 50: \[ 50 = 2 \times 5^2 \]
- Identify and group perfect squares: \[ \sqrt{50} = \sqrt{2 \times 5^2} = \sqrt{25 \times 2} \]
- Simplify the square root: \[ \sqrt{50} = \sqrt{25} \times \sqrt{2} = 5\sqrt{2} \]
Understanding and applying the concept of simplification makes solving equations involving square roots more manageable and is a valuable tool in both academic and practical mathematics.
Prime Factorization Method
The prime factorization method is a powerful technique for simplifying square roots by breaking down the number into its prime factors. This method helps to identify and extract perfect square factors, making it easier to simplify the expression. Here’s a detailed step-by-step guide to using the prime factorization method:
- Find the Prime Factors:
Begin by finding the prime factors of the number inside the square root. Prime factors are the prime numbers that multiply together to give the original number.
For example, to simplify \( \sqrt{72} \):
- Start with the smallest prime, 2. Divide 72 by 2:
\[ 72 \div 2 = 36 \]
- Continue dividing by 2 until it’s no longer possible:
\[ 36 \div 2 = 18 \]
\[ 18 \div 2 = 9 \] - Switch to the next smallest prime, 3. Divide 9 by 3:
\[ 9 \div 3 = 3 \]
\[ 3 \div 3 = 1 \]
So, the prime factorization of 72 is:
\[ 72 = 2^3 \times 3^2 \] - Start with the smallest prime, 2. Divide 72 by 2:
- Group the Prime Factors:
Identify pairs of prime factors to form perfect squares. A perfect square is a product of pairs of identical prime numbers.
For \( \sqrt{72} \):
\[ \sqrt{72} = \sqrt{2^3 \times 3^2} \]
Group the prime factors:
\[ \sqrt{72} = \sqrt{(2^2 \times 3^2) \times 2} = \sqrt{36 \times 2} \] - Simplify the Square Root:
Extract the square roots of the perfect squares from under the radical and multiply them together, leaving any remaining factors inside the square root.
For \( \sqrt{72} \):
\[ \sqrt{72} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2} \]
This method simplifies the process of dealing with large numbers under the square root by reducing them to more manageable forms. Let’s practice with another example:
Simplify \( \sqrt{180} \):
- Prime factorize 180: \[ 180 = 2^2 \times 3^2 \times 5 \]
- Group the prime factors: \[ \sqrt{180} = \sqrt{(2^2 \times 3^2) \times 5} = \sqrt{36 \times 5} \]
- Simplify the square root: \[ \sqrt{180} = 6\sqrt{5} \]
Using the prime factorization method allows you to simplify square roots accurately and efficiently, providing a strong foundation for tackling more complex mathematical problems.
Step-by-Step Simplification of √72
Simplifying the square root of 72 involves breaking down the number into its simplest form. This process makes it easier to understand and work with in mathematical problems. Follow these detailed steps to simplify \( \sqrt{72} \):
- Prime Factorize 72:
Start by finding the prime factors of 72. Prime factorization involves dividing the number by its smallest prime factors:
- Divide 72 by 2:
\[ 72 \div 2 = 36 \]
- Continue dividing by 2:
\[ 36 \div 2 = 18 \]
\[ 18 \div 2 = 9 \] - Switch to the next smallest prime, 3:
\[ 9 \div 3 = 3 \]
\[ 3 \div 3 = 1 \]
The prime factors of 72 are:
\[ 72 = 2^3 \times 3^2 \] - Divide 72 by 2:
- Rewrite the Square Root:
Express the square root of 72 using its prime factors:
\[ \sqrt{72} = \sqrt{2^3 \times 3^2} \]
- Identify Perfect Squares:
Group the prime factors into pairs to form perfect squares. A perfect square can be extracted from the square root:
\[ \sqrt{72} = \sqrt{(2^2 \times 3^2) \times 2} = \sqrt{36 \times 2} \]
- Extract the Square Root:
Take the square root of the perfect squares and simplify:
\[ \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2} \]
The square root of 36 is 6, so:
\[ \sqrt{36} = 6 \]
- Combine the Results:
Combine the extracted square root with the remaining factor under the square root:
\[ \sqrt{72} = 6\sqrt{2} \]
The simplified form of \( \sqrt{72} \) is \( 6\sqrt{2} \). This step-by-step process ensures that the square root is expressed in its simplest radical form, making it more manageable for further calculations.
Let’s summarize the simplification in a table:
| Step | Description | Result |
|---|---|---|
| 1 | Prime Factorize 72 | \( 72 = 2^3 \times 3^2 \) |
| 2 | Rewrite the Square Root | \( \sqrt{72} = \sqrt{2^3 \times 3^2} \) |
| 3 | Identify Perfect Squares | \( \sqrt{72} = \sqrt{36 \times 2} \) |
| 4 | Extract the Square Root | \( \sqrt{36} = 6 \) |
| 5 | Combine the Results | \( \sqrt{72} = 6\sqrt{2} \) |
This method is applicable to any number under the square root and provides a systematic way to simplify complex square root expressions.

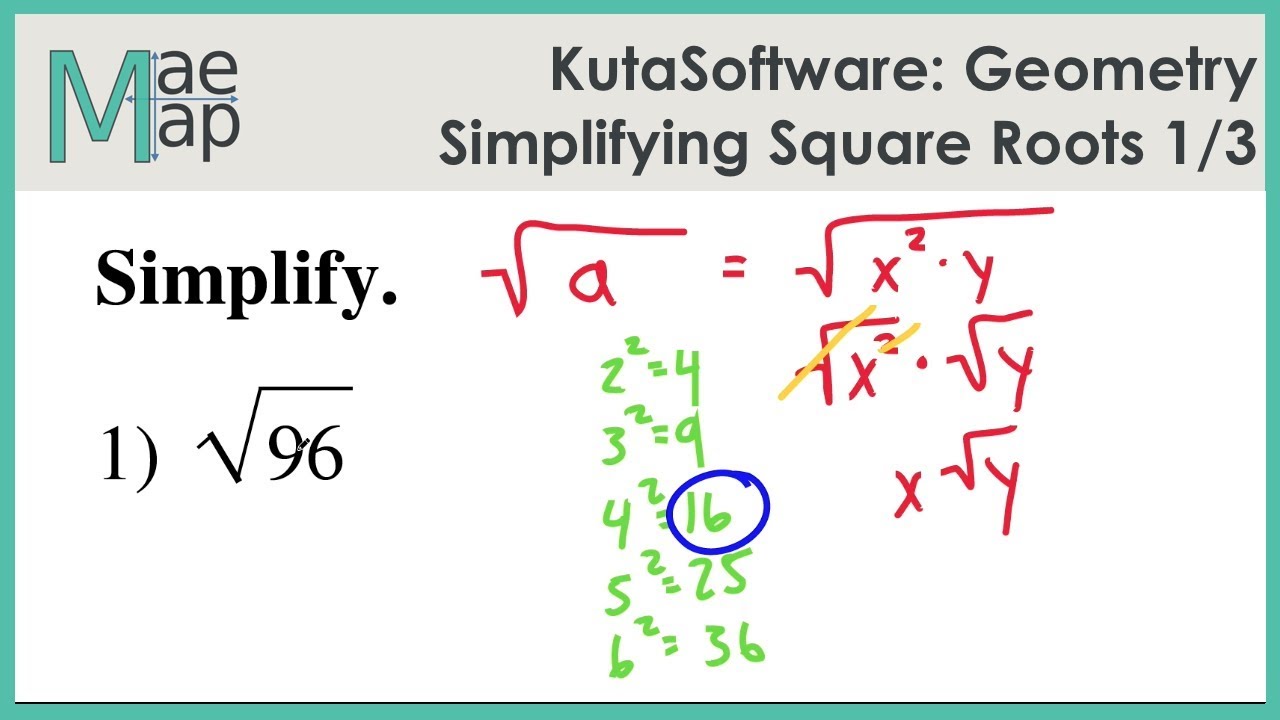
Visualizing the Simplification Process
Visualizing the simplification of \( \sqrt{72} \) helps in understanding the transformation from a complex expression to its simplest form. This section illustrates each step through diagrams and breakdowns, making the process clear and intuitive.
Here’s a visual guide to simplifying \( \sqrt{72} \):
- Prime Factorization Tree:
Start by constructing a prime factorization tree for 72.

Decompose 72 into its prime factors:
- Divide 72 by 2:
\[ 72 \div 2 = 36 \]
- Divide 36 by 2:
\[ 36 \div 2 = 18 \]
- Divide 18 by 2:
\[ 18 \div 2 = 9 \]
- Divide 9 by 3:
\[ 9 \div 3 = 3 \]
- Divide 3 by 3:
\[ 3 \div 3 = 1 \]
The prime factors of 72 are:
\[ 72 = 2^3 \times 3^2 \] - Divide 72 by 2:
- Breaking Down the Square Root:
Write the square root of 72 using its prime factors:
Expression
Visualization
\( \sqrt{72} \)

\( \sqrt{2^3 \times 3^2} \)

\( \sqrt{(2^2 \times 3^2) \times 2} \)

- Extracting Perfect Squares:
Group the factors to form perfect squares and extract their square roots:
Perfect Squares
Non-Perfect Squares
\( \sqrt{36} = 6 \)
\( \sqrt{2} \)
- Combining the Results:
Combine the simplified factors:
\[ \sqrt{72} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2} \]

This visual breakdown makes it easier to understand how to simplify square roots by following a clear and logical sequence of steps. Visual aids can reinforce the process and aid in retention, making complex mathematical operations more accessible.
Mathematical Notation and Explanations
In mathematics, simplifying square roots involves expressing the number inside the square root in its simplest form. For \( \sqrt{72} \), this process can be clearly understood using mathematical notation and step-by-step explanations. Let’s break down the simplification process with proper notation:
- Prime Factorization:
The first step is to find the prime factors of 72. Write 72 as a product of its prime factors:
\[ 72 = 2^3 \times 3^2 \] - Rewrite Using Square Roots:
Express the square root of 72 using these prime factors:
\[ \sqrt{72} = \sqrt{2^3 \times 3^2} \] - Identify and Extract Perfect Squares:
Separate the prime factors into perfect squares and non-perfect squares. A perfect square is a product of two identical prime factors:
\[ \sqrt{2^3 \times 3^2} = \sqrt{2^2 \times 2 \times 3^2} \]Rewrite this as:
\[ \sqrt{(2^2 \times 3^2) \times 2} \] - Simplify the Expression:
Take the square root of the perfect squares and multiply them outside the radical:
\[ \sqrt{2^2 \times 3^2} = \sqrt{(2 \times 3)^2} = 6 \]
\]So, we have:
\[ \sqrt{(2^2 \times 3^2) \times 2} = 6 \times \sqrt{2} \] - Combine and Simplify:
Combine the simplified parts to get the final simplified form:
\[ \sqrt{72} = 6\sqrt{2} \]
Here’s a summary of the steps using a table for clarity:
| Step | Description | Expression |
|---|---|---|
| 1 | Prime Factorize 72 | \( 72 = 2^3 \times 3^2 \) |
| 2 | Rewrite Using Square Roots | \( \sqrt{72} = \sqrt{2^3 \times 3^2} \) |
| 3 | Identify Perfect Squares | \( \sqrt{(2^2 \times 3^2) \times 2} \) |
| 4 | Extract Perfect Squares | \( \sqrt{2^2 \times 3^2} = 6 \) |
| 5 | Combine and Simplify | \( \sqrt{72} = 6\sqrt{2} \) |
This detailed explanation with mathematical notation shows how to simplify square roots systematically. Each step ensures that you can break down complex expressions into simpler forms, making calculations and further operations easier to manage.
Common Mistakes and How to Avoid Them
When simplifying square roots, especially √72, students often make several common mistakes. Understanding these mistakes and how to avoid them can help improve accuracy and confidence in solving these problems.
-
Mistake 1: Incorrect Prime Factorization
Many students incorrectly factorize the number 72. The correct prime factorization of 72 is:
\[
72 = 2^3 \times 3^2
\]How to avoid: Double-check your factorization process and ensure you have correctly identified all prime factors.
-
Mistake 2: Forgetting to Pair Factors
Another common error is not pairing the factors properly when taking the square root.
\[
\sqrt{72} = \sqrt{2^3 \times 3^2} = \sqrt{(2^2 \times 3^2) \times 2} = \sqrt{(2 \times 3)^2 \times 2} = \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2}
\]How to avoid: Always look for pairs of factors under the square root sign and simplify them first.
-
Mistake 3: Simplifying Too Early
Sometimes, students try to simplify the square root before completing the prime factorization process.
\[
\sqrt{72} = \sqrt{36 \times 2} = 6\sqrt{2}
\]How to avoid: Complete the factorization process before simplifying the square root to ensure accuracy.
-
Mistake 4: Incorrectly Simplifying the Result
After correctly factorizing and pairing, students sometimes incorrectly simplify the final result.
How to avoid: Double-check your simplification steps and ensure you multiply and simplify correctly:
\[
\sqrt{72} = 6\sqrt{2}
\] -
Mistake 5: Confusing Square Roots with Other Operations
Students sometimes confuse square roots with other mathematical operations such as squaring or multiplying.
How to avoid: Pay close attention to the operation being performed and remember that simplifying square roots involves finding pairs of factors.
By being aware of these common mistakes and taking steps to avoid them, you can simplify square roots, such as √72, more accurately and efficiently.
Practical Examples and Practice Problems
Understanding how to simplify square roots involves recognizing and breaking down the number inside the square root into its prime factors and simplifying. Here are some practical examples and practice problems to help reinforce these concepts.
Example 1: Simplifying √72
To simplify √72, follow these steps:
- Factor 72 into its prime factors: \(72 = 2 \times 2 \times 2 \times 3 \times 3\)
- Group the prime factors into pairs: \(\sqrt{72} = \sqrt{(2 \times 2) \times (3 \times 3) \times 2}\)
- Simplify by taking the square root of each pair: \(\sqrt{72} = 2 \times 3 \times \sqrt{2} = 6\sqrt{2}\)
Thus, \( \sqrt{72} = 6\sqrt{2} \).
Practice Problems
- Simplify \( \sqrt{50} \)
- Simplify \( \sqrt{128} \)
- Simplify \( \sqrt{45} \)
- Simplify \( \sqrt{200} \)
- Simplify \( \sqrt{98} \)
Solutions to Practice Problems
- \( \sqrt{50} \)
- Factor 50: \(50 = 2 \times 5 \times 5\)
- Group the factors: \( \sqrt{50} = \sqrt{(5 \times 5) \times 2} \)
- Simplify: \( \sqrt{50} = 5\sqrt{2} \)
- \( \sqrt{128} \)
- Factor 128: \(128 = 2 \times 2 \times 2 \times 2 \times 2 \times 2 \times 2\)
- Group the factors: \( \sqrt{128} = \sqrt{(2 \times 2) \times (2 \times 2) \times (2 \times 2) \times 2} \)
- Simplify: \( \sqrt{128} = 2 \times 2 \times 2\sqrt{2} = 8\sqrt{2} \)
- \( \sqrt{45} \)
- Factor 45: \(45 = 3 \times 3 \times 5\)
- Group the factors: \( \sqrt{45} = \sqrt{(3 \times 3) \times 5} \)
- Simplify: \( \sqrt{45} = 3\sqrt{5} \)
- \( \sqrt{200} \)
- Factor 200: \(200 = 2 \times 2 \times 2 \times 5 \times 5\)
- Group the factors: \( \sqrt{200} = \sqrt{(2 \times 2) \times (5 \times 5) \times 2} \)
- Simplify: \( \sqrt{200} = 2 \times 5\sqrt{2} = 10\sqrt{2} \)
- \( \sqrt{98} \)
- Factor 98: \(98 = 2 \times 7 \times 7\)
- Group the factors: \( \sqrt{98} = \sqrt{(7 \times 7) \times 2} \)
- Simplify: \( \sqrt{98} = 7\sqrt{2} \)
These examples and practice problems should help you become more comfortable with the process of simplifying square roots. Keep practicing with different numbers to reinforce your understanding.
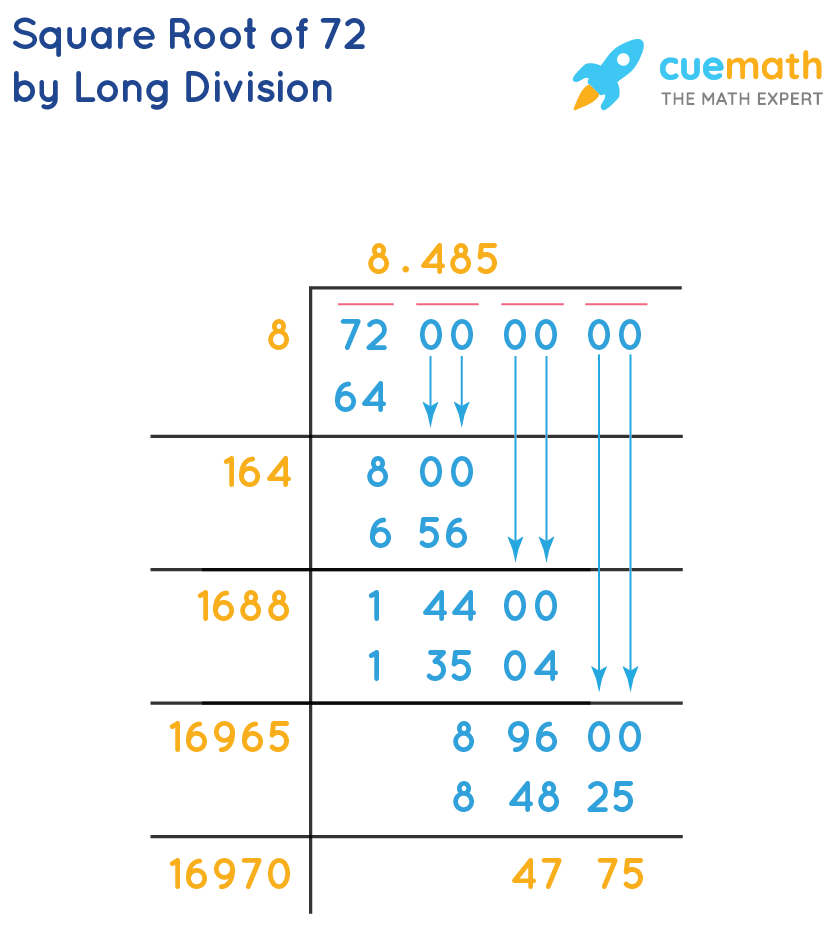
Applications in Real-World Scenarios
The simplification of square roots, such as √72, has numerous practical applications across various fields. Here are some examples:
-
Architecture and Engineering:
Square roots are used to calculate dimensions and structural elements. For example, when designing buildings and bridges, engineers use square roots to determine the natural frequency of structures to ensure stability and safety.
-
Finance:
In finance, square roots are employed to calculate stock market volatility. The standard deviation, which measures the amount of variation or dispersion of a set of values, is derived by taking the square root of the variance.
-
Science:
Square roots are crucial in various scientific calculations. For example, in physics, the time it takes for an object to fall to the ground can be calculated using the formula involving the square root of the height from which it is dropped.
-
Statistics:
In statistics, the standard deviation is the square root of the variance and is used to measure the spread of a set of data points. This helps in data analysis and making informed decisions based on statistical results.
-
Computer Science and Cryptography:
Square roots are used in algorithms for encryption and data security. For instance, many cryptographic protocols rely on the properties of square roots to ensure secure communication.
-
Navigation:
Calculating distances between points on a map or globe often involves using square roots. This is essential for navigation and geographic information systems (GIS).
-
Electrical Engineering:
In electrical engineering, square roots are used to calculate power, voltage, and current in circuits, which are fundamental for designing and analyzing electrical systems.
-
Photography:
The aperture of a camera lens is expressed as an f-number, which involves square roots to control the amount of light entering the camera. Adjusting the f-number changes the exposure and depth of field in photography.
-
Geometry:
Square roots are used to find the length of sides in right triangles, applying the Pythagorean theorem. This is crucial in fields like carpentry, land surveying, and any work involving geometric calculations.
-
Cooking:
When scaling recipes, especially for large quantities, square roots can help in adjusting the amounts of ingredients proportionally to maintain the desired flavor and consistency.
These examples illustrate the broad utility of square roots in simplifying complex problems and providing practical solutions in everyday life and professional practices.
Additional Resources and Further Reading
For those who wish to delve deeper into the topic of simplifying square roots, the following resources provide comprehensive guides, detailed examples, and interactive tools:
-
This resource provides a step-by-step approach to simplifying square roots using the product rule. It includes examples and practice problems to help reinforce the concepts.
-
Math is Fun offers an accessible explanation of simplifying square roots, with numerous examples demonstrating the process. This site also covers related topics such as simplifying fractions under square roots.
-
This resource focuses on simplifying square roots that involve fractions. It provides a detailed methodology and worked examples to illustrate the process.
-
Khan Academy offers video tutorials and practice exercises on simplifying square roots. The platform provides a structured learning path with interactive content to test your understanding.
-
Purplemath provides in-depth lessons on simplifying radicals, including square roots. The site includes step-by-step instructions, examples, and tips to avoid common mistakes.
These resources are excellent for students and educators looking to enhance their understanding of simplifying square roots and to find additional practice problems. Whether you prefer written guides, interactive tools, or video tutorials, these links offer valuable content to support your learning journey.
Cách Tốt Nhất Để Đơn Giản Hóa Căn Bậc Hai Của Một Số, √72
READ MORE:
Cách Tính Căn Bậc Hai của 72: √72