Topic simplify square root 72: Simplifying the square root of 72 can seem challenging, but with the right approach, it becomes straightforward. This guide will walk you through the steps to simplify √72, explore different methods, and explain its significance in various forms. Whether you're a student or math enthusiast, you'll find this information valuable and easy to understand.
Table of Content
Simplifying the Square Root of 72
The square root of 72 can be simplified by following these steps:
Step-by-Step Simplification
- List the factors of 72: 1, 2, 3, 4, 6, 8, 9, 12, 18, 24, 36, 72.
- Identify the perfect squares from the list: 1, 4, 9, 36.
- Divide 72 by the largest perfect square: \( 72 \div 36 = 2 \).
- Calculate the square root of the largest perfect square: \( \sqrt{36} = 6 \).
- Combine the results to get the simplest form: \( \sqrt{72} = 6\sqrt{2} \).
Thus, the simplified form of the square root of 72 is \( 6\sqrt{2} \).
Decimal Form
The decimal approximation of \( \sqrt{72} \) is:
\( \sqrt{72} \approx 8.485 \) (up to three decimal places)
Exponential Form
The square root of 72 can also be expressed using exponents:
\( \sqrt{72} = 72^{1/2} = 6 \cdot 2^{1/2} \)
Methods to Find the Square Root
- Repeated Subtraction
- Long Division
Prime Factorization Method
To simplify \( \sqrt{72} \) using prime factorization:
- Express 72 as a product of prime factors: \( 72 = 2^3 \cdot 3^2 \).
- Group the prime factors into pairs: \( 72 = (2 \cdot 3)^2 \cdot 2 \).
- Take the square root of the grouped factors: \( \sqrt{72} = \sqrt{(2 \cdot 3)^2 \cdot 2} = 6\sqrt{2} \).
Examples and Applications
For instance, the radius of a circle with an area of \( 72\pi \) square inches can be found using the square root of 72:
If the area of a circle \( \pi r^2 = 72\pi \), then \( r^2 = 72 \). Taking the square root of both sides, \( r = \sqrt{72} = 8.48 \) inches.
Conclusion
The square root of 72 simplifies to \( 6\sqrt{2} \). This value can be expressed in various forms and calculated using different methods, each providing a clear path to the simplified result.

READ MORE:
Introduction
Understanding how to simplify the square root of 72 can help in various mathematical applications. The square root of 72, represented as √72, can be simplified to 6√2. This involves breaking down the number into its prime factors and using the properties of square roots to simplify. Below are the steps to simplify √72:
- Prime Factorization: Break down 72 into its prime factors. 72 = 2 × 2 × 2 × 3 × 3 = 23 × 32.
- Grouping: Group the prime factors into pairs. Here, 72 = (2 × 3) × (2 × 3) × 2 = (6) × (6) × 2.
- Simplifying: Take the square root of each pair. √72 = √(6 × 6 × 2) = 6√2.
The simplified form of the square root of 72 is 6√2, and this can be further verified using different mathematical methods such as the repeated subtraction method, prime factorization method, and long division method.
| Exact Form | 6√2 |
| Decimal Form | Approximately 8.49 |
The square root of 72 is an irrational number, as it cannot be expressed as a simple fraction and its decimal form is non-terminating and non-repeating.
What is the Square Root of 72?
The square root of 72 is a number that, when multiplied by itself, equals 72. This can be represented mathematically as \( \sqrt{72} \), where the radical symbol \( \sqrt{} \) denotes the square root, and 72 is the radicand.
To understand the square root of 72, it helps to simplify it. The prime factorization of 72 is \( 72 = 2^3 \times 3^2 \). Using the property of square roots that \( \sqrt{a \times b} = \sqrt{a} \times \sqrt{b} \), we can simplify \( \sqrt{72} \) as follows:
- Factor 72 into its prime factors: \( 72 = 2^3 \times 3^2 \).
- Group the factors into pairs: \( 72 = (2^2 \times 3^2) \times 2 \).
- Take the square root of each pair: \( \sqrt{72} = \sqrt{(2 \times 3)^2 \times 2} = 6\sqrt{2} \).
Thus, the simplified form of \( \sqrt{72} \) is \( 6\sqrt{2} \). In decimal form, \( \sqrt{72} \approx 8.485 \). This value is non-repeating and non-terminating, confirming that \( \sqrt{72} \) is an irrational number.
Various methods can be used to calculate the square root of 72, including the prime factorization method, long division method, and repeated subtraction method. Each method provides a step-by-step approach to understanding the value and properties of the square root of 72.
In conclusion, the square root of 72 is a fundamental concept in mathematics, represented as \( 6\sqrt{2} \) in its simplest form and approximately 8.485 in decimal form. This value plays a crucial role in various mathematical applications and problem-solving scenarios.
Methods to Simplify Square Root of 72
To simplify the square root of 72, we can use several methods. Below are the detailed steps for each method:
- Prime Factorization Method
This method involves breaking down the number 72 into its prime factors:
- First, we find the prime factors of 72. The prime factorization of 72 is:
- 72 = 2 × 36
- 36 = 2 × 18
- 18 = 2 × 9
- 9 = 3 × 3
- Therefore, 72 can be expressed as 23 × 32.
- To simplify the square root, we pair the prime factors:
- √72 = √(23 × 32)
- We take one number from each pair out of the square root:
- √72 = √(2 × 2 × 2 × 3 × 3) = 6√2
- Repeated Subtraction Method
This method involves subtracting consecutive odd numbers from 72 until the result is zero or a negative number, which is more conceptual and less practical for large numbers like 72.
- Long Division Method
This method is used to find the square root of non-perfect squares more accurately. Here are the steps:
- Step 1: Pair the digits of 72 starting from the decimal point.
- Step 2: Find a number whose square is less than or equal to 72. The largest such number is 8 (8 × 8 = 64).
- Step 3: Subtract 64 from 72 to get the remainder 8.
- Step 4: Bring down pairs of zeros and continue the process to get a more accurate decimal value.
- The square root of 72 is approximately 8.485 when using the long division method.
Using these methods, we can conclude that the simplified form of the square root of 72 is 6√2.
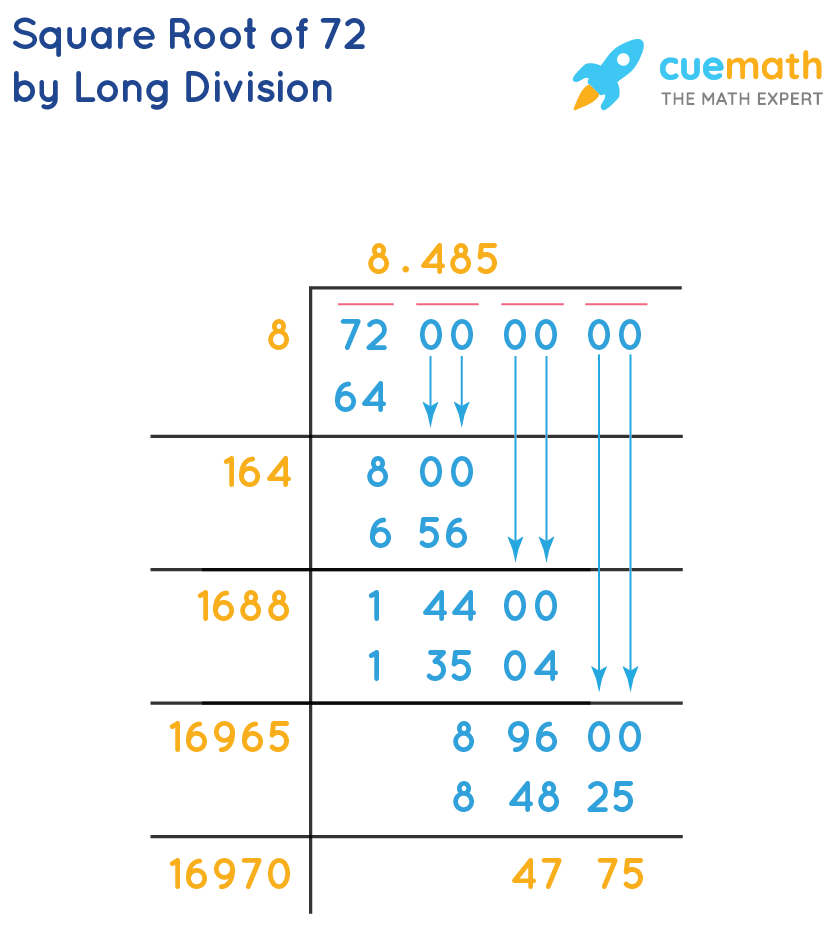
Long Division Method
The long division method is an effective way to find the square root of a number, especially when the number is not a perfect square. Below are the detailed steps to simplify the square root of 72 using the long division method:
- Write the number 72 as 72.00000000.
- Start by pairing the digits from the right. Here, we have 72.
- Find the largest number whose square is less than or equal to the first pair. In this case, 82 = 64, and 64 < 72.
- Place 8 as the first digit of the quotient. Subtract 64 from 72 to get a remainder of 8.
- Bring down the next pair of zeros to make the number 800.
- Double the quotient (8) and write it as the new divisor's first part (16_).
- Find the largest digit (x) such that 16x * x is less than or equal to 800. The digit 4 works, as 164 * 4 = 656.
- Write 4 in the quotient next to 8, giving 84. Subtract 656 from 800 to get a remainder of 144.
- Bring down the next pair of zeros to get 14400.
- Double the quotient (84) to get 168 and find the next digit (y) such that 168y * y ≤ 14400. The digit 8 works, as 1688 * 8 = 13504.
- Write 8 in the quotient next to 84, giving 848. Subtract 13504 from 14400 to get a remainder of 896.
- Repeat this process to further decimal places if more precision is needed.
After following these steps, the square root of 72 is approximately 8.485, accurate to three decimal places.

Square Root of 72 in Different Forms
The square root of 72 can be represented in various forms, each providing a different perspective on the value. These forms include the radical form, surd form, and exponential form. Understanding these representations is crucial for different mathematical applications.
- Radical Form: The simplest way to express the square root of 72 is in its radical form, which is written as \( \sqrt{72} \). This notation indicates that we are looking for a number which, when multiplied by itself, gives 72.
- Surd Form: By simplifying the radical, we can express the square root of 72 in surd form. This involves factoring 72 into its prime factors. The process is as follows:
- Prime factorization of 72: \( 72 = 2 \times 2 \times 2 \times 3 \times 3 \).
- Group the factors into pairs: \( (2 \times 2) \times 2 \times (3 \times 3) \).
- Take the square root of each pair: \( \sqrt{(2 \times 2) \times 2 \times (3 \times 3)} = 2 \times 3 \times \sqrt{2} = 6\sqrt{2} \).
- Exponential Form: The exponential form of the square root of 72 is expressed as \( 72^{1/2} \). This notation is particularly useful in higher mathematics and calculus.
- Decimal Form: The decimal approximation of the square root of 72 is approximately \( 8.485 \). This is useful for practical calculations where an exact value is not required.
These different forms provide various ways to work with the square root of 72, depending on the mathematical context and the level of precision needed.
Why is the Square Root of 72 an Irrational Number?
The square root of 72 is an irrational number, which means it cannot be expressed as a simple fraction of two integers. Here are the detailed reasons why:
-
Definition of Irrational Numbers: An irrational number cannot be written as a fraction \(\frac{p}{q}\), where \(p\) and \(q\) are integers and \(q \neq 0\). Irrational numbers have non-terminating and non-repeating decimal expansions.
-
Prime Factorization of 72: To understand why the square root of 72 is irrational, let's look at its prime factorization:
\[
72 = 2^3 \times 3^2
\]
When we take the square root of 72, we get:
\[
\sqrt{72} = \sqrt{2^3 \times 3^2} = \sqrt{2^3} \times \sqrt{3^2} = 2 \times \sqrt{2} \times 3 = 6\sqrt{2}
\] -
Square Root of 2 is Irrational: The term \(\sqrt{2}\) is known to be irrational. This was proven by the ancient Greeks, who showed that no two integers exist whose ratio equals \(\sqrt{2}\).
-
Combining Terms: Since \(\sqrt{2}\) is irrational, any multiple of \(\sqrt{2}\) (except zero) is also irrational. Therefore, \(6\sqrt{2}\) is irrational.
-
Conclusion: Because \(\sqrt{72}\) simplifies to \(6\sqrt{2}\), and \(\sqrt{2}\) is irrational, the square root of 72 is also irrational. It cannot be expressed as a fraction of two integers, and its decimal expansion is non-terminating and non-repeating.
Thus, the square root of 72 is classified as an irrational number due to its inability to be expressed as a simple fraction and the nature of its decimal expansion.
Applications and Examples
The square root of 72, or \(6\sqrt{2}\), has various applications in mathematics, science, engineering, and everyday life. Here are some detailed examples and applications:
-
Geometry: The square root of 72 can be used in geometric calculations. For instance, consider a right triangle with legs of lengths 6 and 6. The hypotenuse of this triangle can be calculated using the Pythagorean theorem:
\[
c = \sqrt{6^2 + 6^2} = \sqrt{36 + 36} = \sqrt{72} = 6\sqrt{2}
\]
This shows the hypotenuse is \(6\sqrt{2}\). -
Trigonometry: In trigonometry, the square root of 72 can be found in calculations involving certain angles and lengths in trigonometric functions. For example, it may appear when solving for the length of the sides in special right triangles.
-
Physics: The square root of 72 can be used in physics problems involving distance, velocity, and acceleration where the calculations result in this value. For example, if an object moves diagonally across a square field with each side of 6 units, the distance traveled diagonally is \(6\sqrt{2}\) units.
-
Engineering: Engineers may use the square root of 72 in design and structural analysis, particularly when dealing with diagonal bracing and components where this value naturally arises from geometric considerations.
-
Everyday Life: In practical applications, such as determining the diagonal of a rectangular object with sides of equal length, the square root of 72 can provide the necessary measurement. For instance, if you have a square table with each side measuring 6 feet, the diagonal would be \(6\sqrt{2}\) feet, or approximately 8.49 feet.
These examples demonstrate how the square root of 72, represented as \(6\sqrt{2}\), is a useful value in various fields and everyday scenarios.
Frequently Asked Questions
-
Can the square root of 72 be simplified?
Yes, the square root of 72 can be simplified to \(6\sqrt{2}\).
-
What is the square root of 72 rounded to its nearest tenth?
The square root of 72 rounded to its nearest tenth is approximately 8.5.
-
Does 72 have a square root?
Yes, the square root of 72 is approximately 8.48528137423857, which can be simplified to \(6\sqrt{2}\).
-
Is the square root of 72 rational or irrational?
The square root of 72 is an irrational number because it cannot be expressed as a simple fraction and its decimal form is non-repeating and non-terminating.
-
Is the square root of 72 a real number?
Yes, the square root of 72 is a real number.
-
Why is the square root of 72 irrational?
The square root of 72 is irrational because it cannot be expressed as a ratio of two integers. Its decimal expansion is non-repeating and non-terminating, which are the characteristics of an irrational number.
Cách Tốt Nhất Để Đơn Giản Hóa Căn Bậc Hai Của Một Số, Sqrt(72)
READ MORE:
Cách Tính Căn Bậc Hai của 72: Sqrt(72)