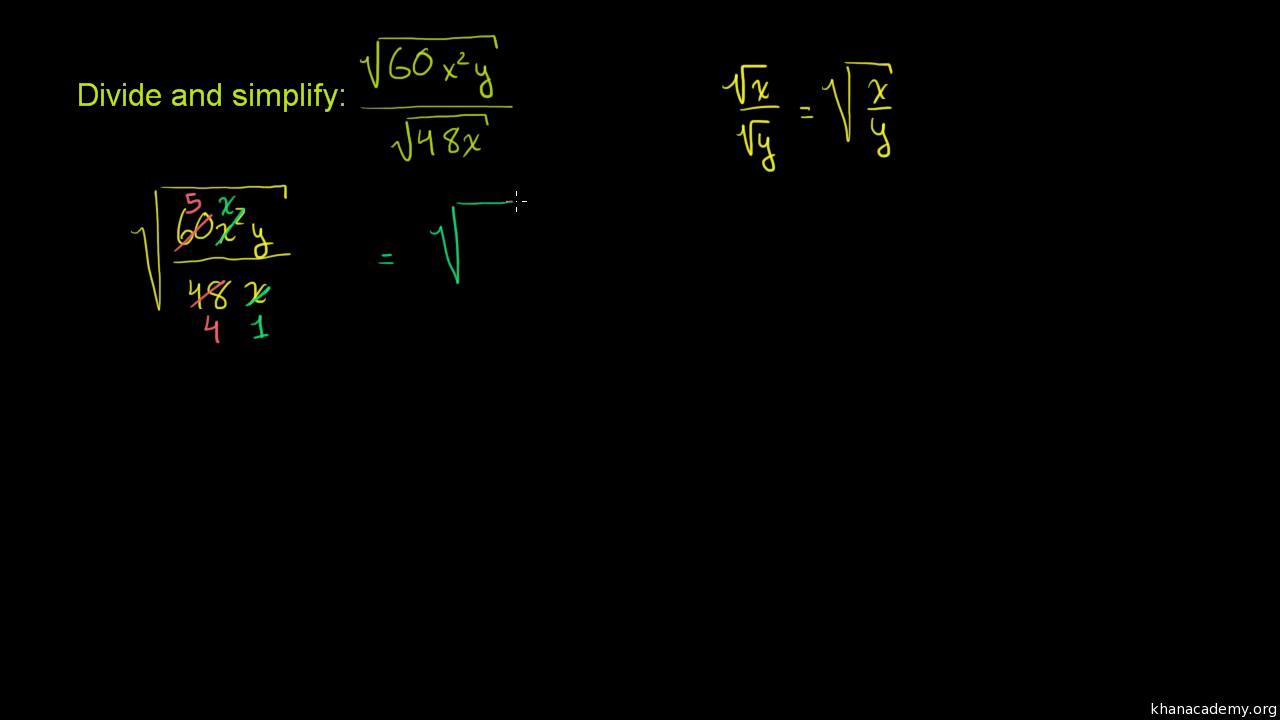Topic simplifying rational expressions step by step calculator: Welcome to our comprehensive guide on simplifying rational expressions step by step using a calculator. Whether you're new to algebra or looking to refresh your skills, this article will walk you through the process with clear explanations and examples. Discover how to simplify complex expressions effortlessly and improve your math proficiency.
Table of Content
- Simplifying Rational Expressions Step-by-Step Calculator
- Introduction to Simplifying Rational Expressions
- Understanding Rational Expressions and Their Components
- Step-by-Step Method for Simplifying Rational Expressions
- Common Challenges in Simplifying Rational Expressions
- Examples of Simplifying Rational Expressions with Detailed Solutions
- Using a Calculator to Simplify Rational Expressions
- Tips and Tricks for Efficiently Simplifying Rational Expressions
- Conclusion and Summary of Key Points
- YOUTUBE: Xem video để hiểu cách đơn giản hóa biểu thức hợp lý một cách chi tiết từng bước. Video này hướng dẫn bạn qua từng giai đoạn của quá trình, giúp bạn nắm vững cách đơn giản hóa biểu thức toán học phức tạp.
Simplifying Rational Expressions Step-by-Step Calculator
Here's a step-by-step guide to simplify rational expressions:
- Identify and factorize the numerator and denominator.
- Cancel out common factors, if possible.
- Check for any remaining factors that can be simplified.
- Verify the simplified expression is in its simplest form.
Example Calculation:
Simplify \( \frac{6x^2 - 2x}{4x^2 - 2} \) :
| Step 1: | Factor numerator: \( 2x(3x - 1) \) |
| Factor denominator: \( 2(2x^2 - 1) \) | |
| Step 2: | Cancel out common factors: \( \frac{x(3x - 1)}{2x(2x - 1)} \) |
| Step 3: | Simplify remaining expression if possible. |
| Step 4: | Verify: \( \frac{x(3x - 1)}{2x(2x - 1)} \) is in simplest form. |

READ MORE:
Introduction to Simplifying Rational Expressions
Simplifying rational expressions is a fundamental skill in algebra, essential for solving equations and understanding mathematical relationships. It involves reducing complex fractions to their simplest form by factoring and canceling common factors. Here's a step-by-step approach:
- Identify the numerator and denominator of the rational expression.
- Factor both the numerator and the denominator completely.
- Cancel out any common factors between the numerator and the denominator.
- Simplify the resulting expression if possible.
Using a calculator can expedite this process, ensuring accuracy and efficiency in your calculations.
Understanding Rational Expressions and Their Components
Rational expressions are fractions where the numerator and denominator are polynomials. The denominator of a rational expression must not be zero, as this would make the expression undefined. Rational expressions are written in the form:
\[ \text{Rational Expression} = \dfrac{A(x)}{B(x)} \]
where \( A(x) \) and \( B(x) \) are polynomials and \( B(x) \neq 0 \).
Let's break down the components of a rational expression:
- Numerator: The numerator is the polynomial expression above the fraction line. For example, in the rational expression \( \dfrac{6x + 1}{2x + 1} \), the numerator is \( 6x + 1 \).
- Denominator: The denominator is the polynomial expression below the fraction line. In the example \( \dfrac{6x + 1}{2x + 1} \), the denominator is \( 2x + 1 \).
It is crucial to identify the values of the variable that make the denominator zero, as these values are excluded from the domain of the rational expression. For instance, in the expression \( \dfrac{1}{x-2} \), the value \( x = 2 \) makes the denominator zero, thus \( x \neq 2 \).
Operations on Rational Expressions
Just like numerical fractions, rational expressions can undergo various arithmetic operations such as addition, subtraction, multiplication, and division.
Addition and Subtraction
To add or subtract rational expressions:
- Find a common denominator.
- Rewrite each expression with the common denominator.
- Add or subtract the numerators while keeping the common denominator.
- Simplify the resulting expression if possible.
Multiplication
To multiply rational expressions:
- Factorize the numerators and denominators.
- Multiply the numerators together and the denominators together.
- Cancel any common factors between the numerator and the denominator.
Division
To divide rational expressions:
- Multiply by the reciprocal of the divisor.
- Factorize the numerators and denominators.
- Cancel any common factors.
Examples
Here are a few examples to illustrate the simplification of rational expressions:
| Expression | Simplification Steps | Result |
|---|---|---|
| \( \dfrac{x^2 - 9}{x^2 + 6x + 9} \) |
|
\( \dfrac{x-3}{x+3} \) |
| \( \dfrac{x^2 - 4x + 4}{x^2 - 2x} \) |
|
\( \dfrac{x-2}{x} \) |
By understanding these fundamental components and operations, you will be better equipped to simplify and work with rational expressions efficiently.
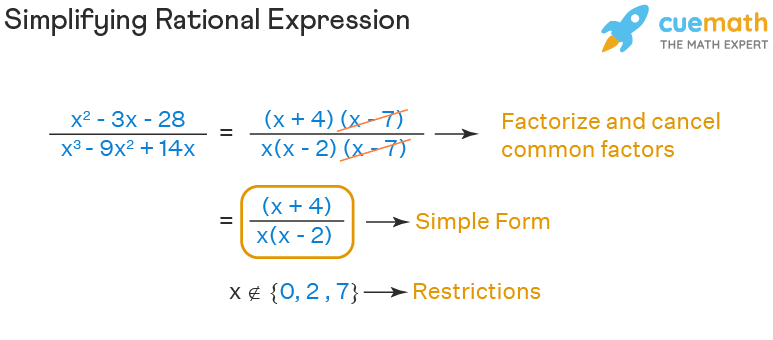
Step-by-Step Method for Simplifying Rational Expressions
Rational expressions are fractions where both the numerator and the denominator are polynomials. Simplifying these expressions involves reducing them to their simplest form by factoring and canceling common factors. Here’s a step-by-step method to simplify rational expressions:
-
Identify and Factorize: Factorize both the numerator and the denominator into their simplest polynomial forms.
Example: Simplify \( \dfrac{x^2 - 9}{x^2 - 6x + 9} \)
Factor both numerator and denominator:
\( \dfrac{(x - 3)(x + 3)}{(x - 3)(x - 3)} \)
-
Cancel Common Factors: Cancel out any common factors that appear in both the numerator and the denominator.
In our example, the common factor \( (x - 3) \) can be canceled out:
\( \dfrac{(x - 3)(x + 3)}{(x - 3)(x - 3)} = \dfrac{x + 3}{x - 3} \)
-
Simplify and Write Restrictions: Simplify the remaining expression and note any restrictions on the variable to avoid division by zero.
In our example:
Simplified form: \( \dfrac{x + 3}{x - 3} \)
Restrictions: \( x \neq 3 \)
This method applies to all rational expressions. Below are additional operations you might need when working with rational expressions:
- Adding/Subtracting Rational Expressions: Find a common denominator, combine the numerators, and simplify.
- Multiplying Rational Expressions: Multiply the numerators together and the denominators together, then simplify.
- Dividing Rational Expressions: Multiply by the reciprocal of the divisor and simplify.
Here’s an example of addition:
Simplify \( \dfrac{2}{x} + \dfrac{x}{x - 2} \)
- Find a common denominator: \( x(x - 2) \)
- Rewrite each fraction: \( \dfrac{2(x - 2)}{x(x - 2)} + \dfrac{x^2}{x(x - 2)} \)
- Combine the numerators: \( \dfrac{2x - 4 + x^2}{x(x - 2)} \)
- Simplify: \( \dfrac{x^2 + 2x - 4}{x(x - 2)} \)
By following these steps, you can efficiently simplify any rational expression.
Common Challenges in Simplifying Rational Expressions
Simplifying rational expressions can be a complex task due to several common challenges. Here are some of the most frequently encountered difficulties:
- Factoring Polynomials: One of the primary steps in simplifying rational expressions is factoring polynomials in the numerator and denominator. This can be challenging, especially for higher-degree polynomials or those that do not factor easily.
- Finding Common Denominators: When adding or subtracting rational expressions, finding a common denominator is crucial. This involves determining the least common multiple (LCM) of the denominators, which can be complex for expressions with multiple terms and factors.
- Canceling Common Factors: After factoring, it is essential to cancel common factors in the numerator and denominator. However, it is easy to overlook common factors or mistakenly cancel terms that are not common factors, leading to incorrect simplification.
- Dealing with Complex Fractions: Simplifying complex fractions, which are fractions within fractions, requires careful manipulation to combine and reduce them to simpler forms. This often involves finding a common denominator for the inner fractions and then simplifying the result.
- Handling Restrictions: Rational expressions have restrictions where the denominator cannot be zero. Identifying and correctly expressing these restrictions is crucial to avoid undefined expressions. It is important to remember to consider restrictions from both the original and simplified forms of the expression.
- Performing Arithmetic Operations: Adding, subtracting, multiplying, and dividing rational expressions follow similar rules to arithmetic operations on fractions but require careful attention to the algebraic terms involved. Errors in these operations can lead to incorrect results.
- Simplifying Resulting Expressions: After performing operations or combining expressions, further simplification may be necessary. This can involve additional factoring, canceling, and ensuring the final expression is in its simplest form.
By recognizing and addressing these challenges, you can improve your ability to simplify rational expressions accurately and efficiently.
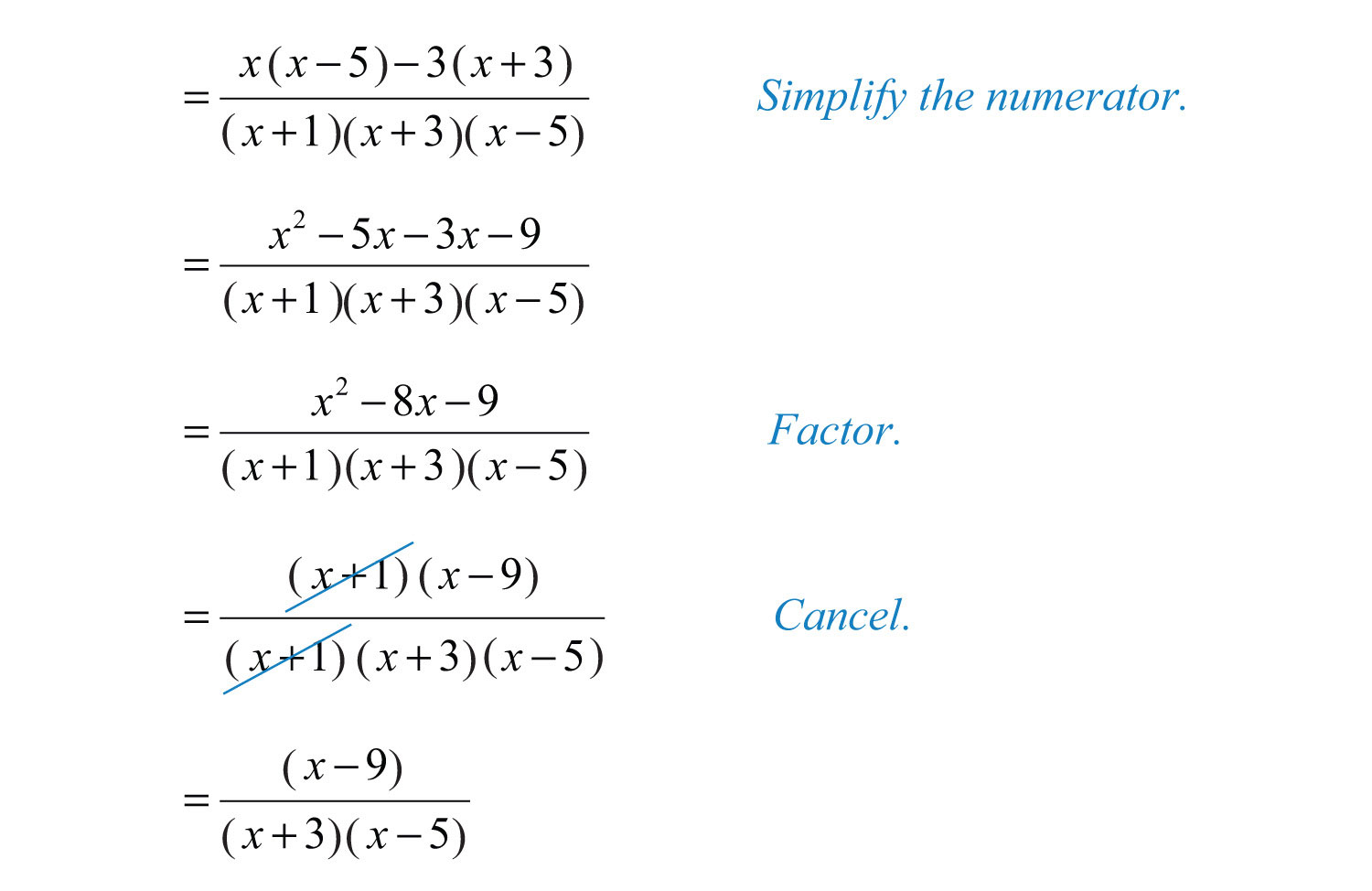
Examples of Simplifying Rational Expressions with Detailed Solutions
Here are some examples that demonstrate how to simplify rational expressions step-by-step. Each example includes detailed solutions to help you understand the process.
Example 1
Simplify the rational expression:
$$ \frac{4x^3 + 8x^2}{2x} $$
- Factor out the greatest common factor (GCF) from the numerator:
- Rewrite the expression:
- Cancel the common factors in the numerator and the denominator:
- The simplified expression is:
$$ 4x^3 + 8x^2 = 4x^2(x + 2) $$
$$ \frac{4x^2(x + 2)}{2x} $$
$$ \frac{4x^2(x + 2)}{2x} = \frac{2x(x + 2)}{1} $$
$$ 2x(x + 2) $$
Example 2
Simplify the rational expression:
$$ \frac{7x^2 + 28x}{x^2 + 8x + 16} $$
- Factor the numerator and the denominator:
- Rewrite the expression:
- Cancel the common factors:
- The simplified expression is:
Numerator: $$ 7x(x + 4) $$
Denominator: $$ (x + 4)(x + 4) $$
$$ \frac{7x(x + 4)}{(x + 4)(x + 4)} $$
$$ \frac{7x(x + 4)}{(x + 4)(x + 4)} = \frac{7x}{x + 4} $$
$$ \frac{7x}{x + 4} $$
Example 3
Simplify the rational expression:
$$ \frac{x^3 + 1}{x^2 + 7x + 6} $$
- Factor the numerator and the denominator:
- Rewrite the expression:
- Cancel the common factors:
- The simplified expression is:
Numerator: $$ (x + 1)(x^2 - x + 1) $$
Denominator: $$ (x + 1)(x + 6) $$
$$ \frac{(x + 1)(x^2 - x + 1)}{(x + 1)(x + 6)} $$
$$ \frac{(x + 1)(x^2 - x + 1)}{(x + 1)(x + 6)} = \frac{x^2 - x + 1}{x + 6} $$
$$ \frac{x^2 - x + 1}{x + 6} $$
These examples illustrate the importance of factoring and canceling common factors to simplify rational expressions effectively.
Using a Calculator to Simplify Rational Expressions
Simplifying rational expressions can often be made easier with the use of a calculator. Here is a step-by-step guide on how to use an online calculator to simplify rational expressions efficiently.
-
Choose a Reliable Calculator: Select a calculator that supports rational expressions. Some popular options include MathPapa, BYJU’S Rational Expression Calculator, and Calculator-Online.
-
Input the Expression: Enter the numerator and denominator of the rational expression into the respective fields of the calculator.
- For example, if you want to simplify \( \frac{x^2 - 4}{x^2 - x - 6} \), enter
x^2 - 4in the numerator field andx^2 - x - 6in the denominator field.
- For example, if you want to simplify \( \frac{x^2 - 4}{x^2 - x - 6} \), enter
-
Perform the Simplification: Click the 'Simplify' or equivalent button on the calculator interface. The calculator will then display the simplified form of the rational expression.
- The calculator will factorize the numerator and denominator where possible and cancel out common factors. For instance, \( \frac{x^2 - 4}{x^2 - x - 6} \) simplifies to \( \frac{(x - 2)(x + 2)}{(x - 3)(x + 2)} = \frac{x - 2}{x - 3} \) after canceling the common factor \( x + 2 \).
-
Review the Steps: Some calculators provide a step-by-step breakdown of the simplification process. Reviewing these steps can help you understand how the expression was simplified.
-
Practice with Examples: Use the calculator to practice simplifying different rational expressions. This will help you become familiar with the process and improve your manual simplification skills.
Using a calculator not only saves time but also helps in verifying the correctness of your manual calculations. Whether you're adding, subtracting, multiplying, or dividing rational expressions, these tools can handle a variety of operations and provide quick and accurate results.
Tips and Tricks for Efficiently Simplifying Rational Expressions
When simplifying rational expressions, there are several tips and tricks that can help you work more efficiently and accurately. Here are some of the best strategies to keep in mind:
-
Factor Completely:
Always start by factoring both the numerator and the denominator completely. Look for common factors, difference of squares, trinomials, and other factoring techniques.
For example, simplify \( \frac{x^2 - 4}{x^2 - x - 6} \):
- Factor the numerator: \( x^2 - 4 = (x - 2)(x + 2) \)
- Factor the denominator: \( x^2 - x - 6 = (x - 3)(x + 2) \)
- Cancel the common factor \( (x + 2) \): \( \frac{(x - 2)(x + 2)}{(x - 3)(x + 2)} = \frac{x - 2}{x - 3} \)
-
Identify and Cancel Common Factors:
Once factored, identify common factors in the numerator and the denominator and cancel them out. Be cautious not to cancel terms that are not factors.
-
Watch for Restricted Values:
Determine the values for which the denominator is zero and exclude these from the domain of the expression. These are known as the restricted values.
For example, in the expression \( \frac{1}{x-2} \), the value \( x = 2 \) is restricted because it makes the denominator zero.
-
Simplify Complex Fractions:
When dealing with complex fractions (fractions within fractions), simplify by multiplying the numerator and the denominator by the least common denominator (LCD) of all the fractions involved.
-
Use the Distributive Property:
Apply the distributive property where necessary to simplify expressions before canceling common factors.
For example, \( \frac{2x(x + 3)}{4x} \):
- Apply the distributive property: \( \frac{2x(x + 3)}{4x} = \frac{2x^2 + 6x}{4x} \)
- Cancel common factors: \( \frac{2(x^2 + 3x)}{4x} = \frac{x + 3}{2} \)
By following these tips and tricks, you can simplify rational expressions more efficiently and avoid common mistakes.
Conclusion and Summary of Key Points
In this guide, we've explored the comprehensive process of simplifying rational expressions, offering detailed explanations and examples to aid your understanding. Here, we summarize the key points discussed:
- Understanding Rational Expressions: A rational expression is a fraction where both the numerator and the denominator are polynomials. Simplifying these expressions involves reducing them to their simplest form.
- Components of Rational Expressions: Key components include the numerator, denominator, and the common factors which play a crucial role in simplification.
- Step-by-Step Simplification: The process involves factoring both the numerator and the denominator, identifying and canceling common factors, and ensuring the expression is in its lowest terms.
- Common Challenges: Challenges include dealing with complex polynomials, recognizing common factors, and ensuring the final expression is simplified correctly. It's important to be meticulous in the factorization process and double-check your work.
- Detailed Examples: Working through multiple examples helps solidify the understanding of the simplification process. Examples highlight different levels of complexity and demonstrate the practical application of the steps.
- Using Calculators: Calculators can be a valuable tool in simplifying rational expressions, especially for verifying solutions and handling more complex calculations efficiently.
- Tips and Tricks: Efficient simplification involves recognizing patterns, understanding polynomial factorization, and practicing regularly to enhance your skills. Additionally, leveraging online resources and tools can streamline the process.
By mastering these concepts and techniques, you will be well-equipped to tackle rational expressions with confidence and accuracy. Remember, practice is key to proficiency, so continue working on various problems to strengthen your understanding.
Xem video để hiểu cách đơn giản hóa biểu thức hợp lý một cách chi tiết từng bước. Video này hướng dẫn bạn qua từng giai đoạn của quá trình, giúp bạn nắm vững cách đơn giản hóa biểu thức toán học phức tạp.
Bạn Có Thể Đơn Giản Biểu Thức Hợp Lý Này Không? | Giải Thích Từng Bước
READ MORE:
Xem video để học cách đơn giản hóa một biểu thức đại số từ những bước cơ bản nhất. Mr. J sẽ hướng dẫn bạn từng bước để hiểu rõ hơn về biểu thức đại số và cách đơn giản hóa chúng.
Cách Đơn Giản Hóa Một Biểu Thức: Hướng Dẫn Cho Người Mới Bắt Đầu | Biểu Thức Đại Số | Toán Học với Mr. J