Topic simplifying rational expressions by factoring: Understanding how to simplify rational expressions by factoring is essential for mastering algebra. This process involves breaking down complex fractions into simpler forms by factoring both the numerator and the denominator. In this article, we'll guide you through the steps and techniques needed to effectively simplify rational expressions, ensuring you build a strong foundation in algebra.
Table of Content
- Simplifying Rational Expressions by Factoring
- Introduction to Rational Expressions
- Factoring Polynomials
- Simplifying Rational Expressions
- Common Denominators and Adding Rational Expressions
- Multiplying and Dividing Rational Expressions
- Complex Rational Expressions
- Domain and Restrictions
- Common Mistakes to Avoid
- Practice Problems and Solutions
- Conclusion and Key Takeaways
- YOUTUBE: Video hướng dẫn cách đơn giản hóa biểu thức hữu tỉ bằng cách phân tích nhân tử, giúp người xem hiểu rõ hơn về chủ đề này.
Simplifying Rational Expressions by Factoring
Simplifying rational expressions involves reducing the expression to its simplest form. This is achieved by factoring both the numerator and the denominator and then canceling out any common factors. Here, we will guide you through the process of simplifying rational expressions using factoring techniques.
Steps to Simplify Rational Expressions
- Factor the Numerator and Denominator: Break down both the numerator and the denominator into their prime factors.
- Identify Common Factors: Look for any common factors in the numerator and the denominator.
- Cancel Common Factors: Cancel out the common factors from the numerator and the denominator.
- Simplify the Expression: Write down the simplified form of the expression.
- State the Domain: Determine the values for which the original expression is undefined (i.e., where the denominator is zero) and exclude these from the domain.
Examples
Let's go through a couple of examples to illustrate these steps:
Example 1
Simplify the rational expression \( \frac{2x}{x^2} \).
- Factor the numerator and the denominator: \( \frac{2 \cdot x}{x \cdot x} \).
- Identify and cancel common factors: \( \frac{2 \cancel{x}}{\cancel{x} \cdot x} = \frac{2}{x} \).
- Simplified expression: \( \frac{2}{x} \).
- State the domain: \( x \neq 0 \).
Example 2
Simplify the rational expression \( \frac{(x + 3)(x + 4)}{(x + 3)(x + 2)} \).
- Factor the numerator and the denominator: \( \frac{(x + 3) \cdot (x + 4)}{(x + 3) \cdot (x + 2)} \).
- Identify and cancel common factors: \( \frac{\cancel{(x + 3)} \cdot (x + 4)}{\cancel{(x + 3)} \cdot (x + 2)} = \frac{x + 4}{x + 2} \).
- Simplified expression: \( \frac{x + 4}{x + 2} \).
- State the domain: \( x \neq -3, -2 \).
Important Points to Remember
- Always factor the numerator and denominator completely before canceling common factors.
- Only factors can be canceled, not terms. Be cautious not to cancel terms incorrectly.
- Check the original expression for values that make the denominator zero and exclude these from the domain.
- The simplified form should be equivalent to the original expression within the defined domain.
Practice Problems
- Simplify \( \frac{x^2 + 5x + 6}{x^2 + 2x + 1} \) and state the domain.
- Simplify \( \frac{4x^2 - 9}{2x^2 - x - 3} \) and state the domain.
READ MORE:
Introduction to Rational Expressions
Rational expressions are fractions where the numerator, the denominator, or both are polynomials. Simplifying these expressions involves factoring polynomials and canceling common factors. Understanding the domain of these expressions is crucial since any value that makes the denominator zero is excluded. By mastering these techniques, you can efficiently work with rational expressions in algebra.
Factoring Polynomials
Factoring polynomials is a fundamental skill in simplifying rational expressions. To factor a polynomial, we express it as a product of its simpler polynomial factors. This process is essential for identifying and canceling common factors in rational expressions. Here's a step-by-step guide to factoring polynomials:
- Identify the Greatest Common Factor (GCF): Determine the largest factor that is common to all terms in the polynomial.
- Factor out the GCF: Write the polynomial as a product of the GCF and the remaining polynomial.
- Factor the remaining polynomial: If the polynomial is a quadratic, apply techniques like factoring by grouping, using the difference of squares, or applying the quadratic formula if necessary.
- Check your factors: Multiply the factors to ensure they produce the original polynomial.
Let's apply these steps to an example:
Example: Factor the polynomial \(6x^2 + 11x - 10\).
- Step 1: Identify the GCF, which in this case is 1 (no common factor other than 1).
- Step 2: Since the GCF is 1, we proceed to factor the quadratic polynomial \(6x^2 + 11x - 10\).
- Step 3: Find two numbers that multiply to \(6 \cdot (-10) = -60\) and add to \(11\). These numbers are \(15\) and \(-4\).
- Step 4: Rewrite the middle term \(11x\) using these numbers: \(6x^2 + 15x - 4x - 10\).
- Step 5: Factor by grouping: \((6x^2 + 15x) + (-4x - 10) = 3x(2x + 5) - 2(2x + 5)\).
- Step 6: Factor out the common binomial factor: \((3x - 2)(2x + 5)\).
Thus, the polynomial \(6x^2 + 11x - 10\) factors to \((3x - 2)(2x + 5)\). By mastering these steps, you can simplify complex rational expressions effectively.
Simplifying Rational Expressions
Simplifying rational expressions involves reducing the expression to its simplest form by factoring the numerator and denominator and canceling common factors. This process makes the expression easier to work with and solve. Here is a step-by-step guide to simplifying rational expressions:
- Factor the Numerator and Denominator:
- Identify and factor out the greatest common factor (GCF) from the numerator and the denominator.
- Factor any trinomials, difference of squares, or other polynomial forms present.
- Identify Common Factors:
- Look for factors that are common to both the numerator and the denominator.
- Cancel Common Factors:
- Divide the numerator and the denominator by their common factors to simplify the expression.
- Simplify the Result:
- Rewrite the expression after canceling the common factors.
- State the Excluded Values:
- Identify any values of the variable that make the denominator zero, as these values are excluded from the domain of the rational expression.
Let's look at an example:
Example: Simplify the rational expression \(\frac{6x^2 + 15x}{3x}\).
- Factor the numerator and the denominator:
- Numerator: \(6x^2 + 15x = 3x(2x + 5)\)
- Denominator: \(3x\)
- Identify common factors:
- The common factor is \(3x\).
- Cancel common factors:
- \(\frac{3x(2x + 5)}{3x} = 2x + 5\)
- Simplify the result:
- The simplified expression is \(2x + 5\).
- State the excluded values:
- Since \(3x \neq 0\), \(x \neq 0\).
Therefore, the simplified form of the expression \(\frac{6x^2 + 15x}{3x}\) is \(2x + 5\) with \(x \neq 0\).
Common Denominators and Adding Rational Expressions
Adding rational expressions requires finding a common denominator, similar to adding numerical fractions. Here’s a step-by-step guide:
-
Identify the Denominators: Write down the denominators of the expressions you want to add.
- Example: For \( \frac{1}{x+2} + \frac{3}{x+3} \), the denominators are \( x+2 \) and \( x+3 \).
-
Find the Least Common Denominator (LCD): The LCD is the least common multiple of the denominators. For \( x+2 \) and \( x+3 \), the LCD is \( (x+2)(x+3) \).
-
Rewrite Each Expression: Adjust each fraction to have the LCD as the denominator.
- \( \frac{1}{x+2} \) becomes \( \frac{1 \cdot (x+3)}{(x+2)(x+3)} = \frac{x+3}{(x+2)(x+3)} \)
- \( \frac{3}{x+3} \) becomes \( \frac{3 \cdot (x+2)}{(x+2)(x+3)} = \frac{3(x+2)}{(x+2)(x+3)} \)
-
Add the Numerators: Combine the numerators over the common denominator.
- \( \frac{x+3}{(x+2)(x+3)} + \frac{3(x+2)}{(x+2)(x+3)} \) becomes \( \frac{(x+3) + 3(x+2)}{(x+2)(x+3)} \)
- Simplify the numerator: \( (x+3) + 3(x+2) = x + 3 + 3x + 6 = 4x + 9 \)
- So, \( \frac{(x+3) + 3(x+2)}{(x+2)(x+3)} = \frac{4x + 9}{(x+2)(x+3)} \)
-
Simplify if Possible: Check if the expression can be simplified further by canceling common factors.
By following these steps, you can add rational expressions effectively, ensuring a correct and simplified result.
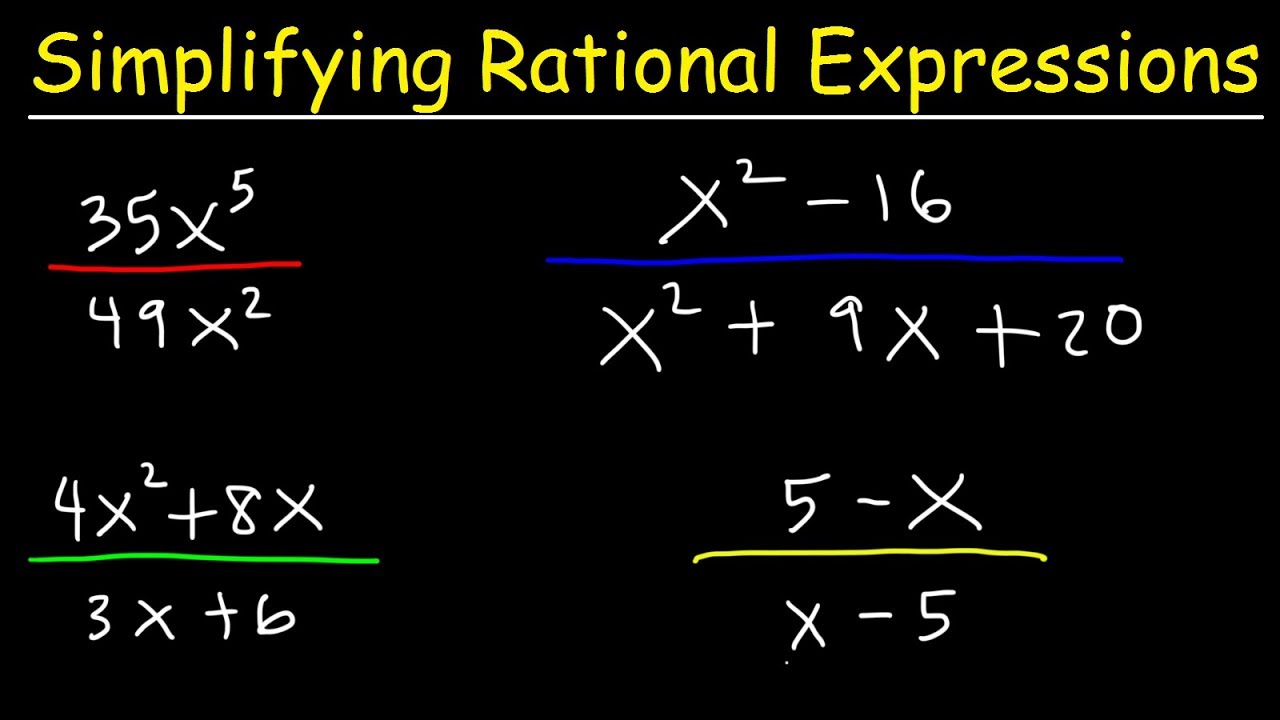
Multiplying and Dividing Rational Expressions
Multiplying and dividing rational expressions involve applying the same principles used for numerical fractions. These operations are performed by factoring polynomials in the numerators and denominators, simplifying, and then carrying out the multiplication or division. Below are the steps to follow:
- Factor the Numerators and Denominators: Factor each polynomial completely in both the numerators and the denominators.
- Identify and Cancel Common Factors: Look for common factors in the numerator and the denominator and cancel them out to simplify the expression.
- Multiply the Remaining Factors: For multiplication, multiply the remaining factors in the numerators together and the denominators together.
- Divide by Inverting: For division, multiply by the reciprocal of the divisor. This involves flipping the numerator and denominator of the divisor and then performing the multiplication step.
Here is an example:
Given the expressions \( \frac{6x^2}{3x} \times \frac{4}{2x} \), follow these steps:
- Factor: \( \frac{6x^2}{3x} = \frac{6 \cdot x \cdot x}{3 \cdot x} \) and \( \frac{4}{2x} = \frac{4}{2 \cdot x} \)
- Cancel Common Factors: Cancel \(3\) and \(x\) from the first fraction and \(2\) from the second fraction:
| \( \frac{6 \cdot x \cdot x}{3 \cdot x} \times \frac{4}{2 \cdot x} \) | \( = \frac{2x}{1} \times \frac{2}{x} \) |
| \( = \frac{2x \cdot 2}{1 \cdot x} \) | \( = \frac{4x}{x} \) |
| \( = 4 \) |
Here is another example involving division:
Given the expressions \( \frac{x^2 - 9}{2x + 6} ÷ \frac{x - 3}{x + 2} \), follow these steps:
- Factor: Factor the polynomials in the numerators and denominators: \( \frac{(x - 3)(x + 3)}{2(x + 3)} \div \frac{x - 3}{x + 2} \)
- Multiply by the Reciprocal: Flip the second fraction and multiply:
| \( \frac{(x - 3)(x + 3)}{2(x + 3)} \times \frac{x + 2}{x - 3} \) |
| Cancel the common factors \( (x - 3) \) and \( (x + 3) \) |
| \( = \frac{1}{2} \times (x + 2) \) |
| \( = \frac{x + 2}{2} \) |
Complex Rational Expressions
Complex rational expressions, also known as compound fractions, are fractions where the numerator, the denominator, or both, contain a rational expression themselves. Simplifying these expressions involves several steps to ensure they are in their simplest form.
- Identify and Factor:
- Examine both the numerator and the denominator to identify any common factors.
- Factor these expressions completely.
- Simplify Inner Fractions:
- If there are complex fractions within the expression, simplify these first.
- Combine any like terms and reduce to simplest form.
- Multiply by the Common Denominator:
- Find the least common denominator (LCD) of all the fractions involved.
- Multiply both the numerator and the denominator by this LCD to eliminate the inner fractions.
- Simplify the Resulting Expression:
- After eliminating the complex fractions, simplify the resulting rational expression as usual.
- Factor out any common terms and reduce to simplest form.
Let's consider an example to illustrate these steps:
\[ \frac{\frac{x+2}{x-1}}{\frac{3x}{2x+4}} \]
- Factor each part:
- Numerator: \( \frac{x+2}{x-1} \)
- Denominator: \( \frac{3x}{2(x+2)} \)
- Simplify inner fractions:
- LCD of the inner fractions is \( (x-1)(x+2) \).
- Multiply by the LCD:
- \[ \frac{(x+2)(2(x+2))}{(x-1)(3x)} \]
- Simplify the resulting expression:
- \[ \frac{2(x+2)^2}{3x(x-1)} \]
- Ensure no further simplification is possible.
By following these steps, you can simplify any complex rational expression to its simplest form.
Domain and Restrictions
When working with rational expressions, it is crucial to determine the domain of the expression. The domain consists of all the values that the variable can take without causing any mathematical errors, such as division by zero.
To find the domain of a rational expression, follow these steps:
- Identify the Denominator: Look at the rational expression and identify the polynomial in the denominator.
- Set the Denominator Equal to Zero: Solve the equation formed by setting the denominator equal to zero. This will help you find the values that make the denominator zero.
- Determine Restricted Values: The solutions to the equation in step 2 are the values that are not in the domain. These values make the denominator zero, which is undefined in mathematics.
- Express the Domain: Write the domain using interval notation or set-builder notation, excluding the restricted values found in step 3.
For example, consider the rational expression \( \frac{x+2}{x^2-4} \).
- Step 1: The denominator is \( x^2 - 4 \).
- Step 2: Setting the denominator equal to zero: \( x^2 - 4 = 0 \).
- Step 3: Solving for \( x \), we get \( x^2 = 4 \), so \( x = 2 \) and \( x = -2 \).
- Step 4: The domain is all real numbers except \( x = 2 \) and \( x = -2 \), which can be written as \( (-\infty, -2) \cup (-2, 2) \cup (2, \infty) \).
Understanding the domain is essential when simplifying rational expressions, as it helps avoid undefined values in calculations.
Common Mistakes to Avoid
When simplifying rational expressions by factoring, there are several common mistakes that can lead to incorrect results. Here are some key points to watch out for:
- Not Fully Factoring the Expression: Ensure that both the numerator and denominator are completely factored. Missing factors can lead to incorrect simplification.
- Canceling Incorrectly: Only cancel common factors that appear in both the numerator and the denominator. Remember that terms connected by addition or subtraction cannot be canceled individually.
- Ignoring Domain Restrictions: Always consider the values that make the denominator zero. Simplifying the expression does not change these restrictions. Include them in your final answer.
- Distributing Instead of Factoring: Be careful not to distribute terms when factoring is required. Factoring helps in identifying common factors that can be canceled.
- Forgetting to Check for Extraneous Solutions: Always recheck the simplified expression against the original domain. Some simplifications may introduce solutions that are not valid for the original expression.
By keeping these common mistakes in mind, you can ensure that your simplifications are accurate and mathematically sound.

Practice Problems and Solutions
Here are some practice problems to help you master simplifying rational expressions by factoring:
-
Problem: Simplify \(\frac{2x^2 - 8}{4x^2 - 16}\).
Solution:
- Factor the numerator and the denominator: \[ \frac{2(x^2 - 4)}{4(x^2 - 4)} = \frac{2(x - 2)(x + 2)}{4(x - 2)(x + 2)} \]
- Cancel the common factors \((x - 2)(x + 2)\): \[ \frac{2}{4} = \frac{1}{2} \]
- Final answer: \(\frac{1}{2}\) (for \(x \neq -2, 2\)).
-
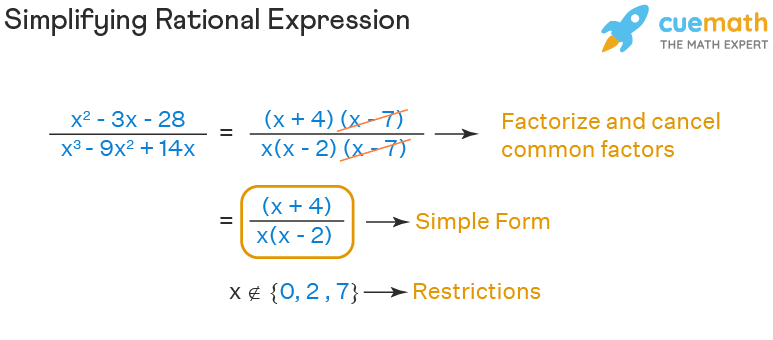
Problem: Simplify \(\frac{x^2 + 5x + 6}{x^2 - 4}\).
Solution:
- Factor both the numerator and the denominator: \[ \frac{(x + 2)(x + 3)}{(x - 2)(x + 2)} \]
- Cancel the common factor \((x + 2)\): \[ \frac{x + 3}{x - 2} \]
- Final answer: \(\frac{x + 3}{x - 2}\) (for \(x \neq -2, 2\)).
-
Problem: Simplify \(\frac{x^2 - 9}{x^2 - 6x + 9}\).
Solution:
- Factor the numerator and the denominator: \[ \frac{(x - 3)(x + 3)}{(x - 3)(x - 3)} \]
- Cancel the common factor \((x - 3)\): \[ \frac{x + 3}{x - 3} \]
- Final answer: \(\frac{x + 3}{x - 3}\) (for \(x \neq 3\)).
Practice these problems to gain a better understanding of how to simplify rational expressions by factoring.
Conclusion and Key Takeaways
In this guide, we explored the process of simplifying rational expressions through factoring. Here are the key points to remember:
- Factoring First: Always begin by factoring both the numerator and the denominator completely. This helps identify common factors.
- Canceling Common Factors: After factoring, cancel any common factors in the numerator and denominator. This step simplifies the expression while ensuring it's equivalent to the original for allowed values.
- Identifying Restrictions: Determine the values that make the denominator zero, as these are not included in the domain. Always state these restrictions clearly.
- Verifying Simplification: After simplifying, check your work by substituting values within the domain to ensure both the original and simplified expressions yield the same results.
Simplifying rational expressions by factoring is an essential skill in algebra, providing a foundation for solving more complex equations. Practice regularly to become proficient and always be mindful of the domain restrictions.
Video hướng dẫn cách đơn giản hóa biểu thức hữu tỉ bằng cách phân tích nhân tử, giúp người xem hiểu rõ hơn về chủ đề này.
Cách đơn giản hóa biểu thức hữu tỉ bằng cách phân tích nhân tử
READ MORE:
Video hướng dẫn cách đơn giản hoá các biểu thức hữu tỉ để thu hút người xem và sửa lỗi chính tả.
Đơn Giản Hoá Các Biểu Thức Hữu Tỉ