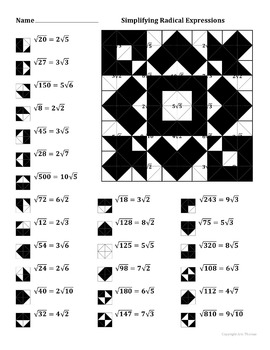
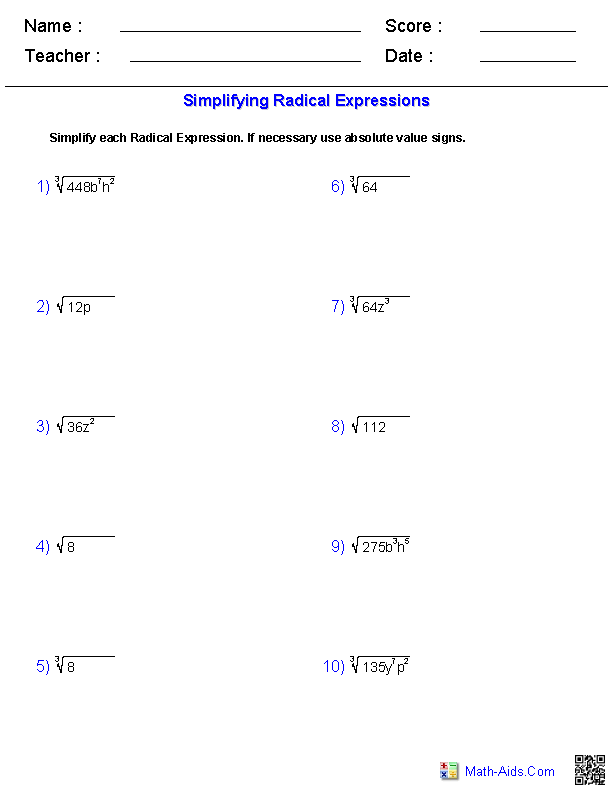Topic algebra 2 simplifying radical expressions: Unlock the secrets to mastering Algebra 2 with our comprehensive guide on simplifying radical expressions. Dive into essential techniques, step-by-step examples, and tips to enhance your understanding and skills. Perfect for students looking to excel in their math courses and simplify complex algebraic problems with ease.
Table of Content
- Simplifying Radical Expressions
- Introduction to Radical Expressions
- Prime Factorization and Radicals
- Product and Quotient Rules
- Simplifying Radical Expressions with Variables
- Examples of Simplifying Radicals
- Simplifying Radicals Involving Variables
- Special Cases and Common Mistakes
- Practice Problems and Solutions
- Advanced Techniques in Simplifying Radicals
- Conclusion and Summary
- YOUTUBE:
Simplifying Radical Expressions
Simplifying radical expressions involves breaking down the expression into its simplest form. Here are some rules and steps to help you simplify radical expressions effectively:
Steps to Simplify Radical Expressions
- Identify the Perfect Squares: Look for perfect square factors of the number under the radical (radicand).
- Rewrite the Radicand: Express the radicand as a product of its prime factors.
- Apply the Product Rule: Use the rule \( \sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b} \) to separate the factors under the radical.
- Simplify: Simplify the square roots of perfect square factors.
- Combine Like Terms: If there are variables, combine them into a simplified form.
Examples of Simplifying Radical Expressions
- Example 1: Simplify \( \sqrt{72} \)
\( \sqrt{72} = \sqrt{36 \cdot 2} = \sqrt{36} \cdot \sqrt{2} = 6\sqrt{2} \)
- Example 2: Simplify \( \sqrt{48} \)
\( \sqrt{48} = \sqrt{16 \cdot 3} = \sqrt{16} \cdot \sqrt{3} = 4\sqrt{3} \)
- Example 3: Simplify \( \sqrt{200} \)
\( \sqrt{200} = \sqrt{100 \cdot 2} = \sqrt{100} \cdot \sqrt{2} = 10\sqrt{2} \)
Special Rules for Simplifying Radical Expressions
| \( \sqrt{a \cdot b} \) | = \( \sqrt{a} \cdot \sqrt{b} \) |
| \( \sqrt{\frac{a}{b}} \) | = \( \frac{\sqrt{a}}{\sqrt{b}} \), where \( b \neq 0 \) |
| \( \sqrt{a} + \sqrt{b} \) | \( \neq \sqrt{a + b} \) |
| \( \sqrt{a} - \sqrt{b} \) | \( \neq \sqrt{a - b} \) |
Rationalizing the Denominator
To simplify a radical expression with a radical in the denominator, multiply the numerator and the denominator by the conjugate of the denominator. For example:
\( \frac{\sqrt{2} + \sqrt{3}}{\sqrt{5} - \sqrt{2}} \)
Multiply the numerator and the denominator by \( \sqrt{5} + \sqrt{2} \):
\( \frac{(\sqrt{2} + \sqrt{3})(\sqrt{5} + \sqrt{2})}{(\sqrt{5} - \sqrt{2})(\sqrt{5} + \sqrt{2})} = \frac{\sqrt{10} + \sqrt{15} + 2 + \sqrt{6}}{3} = \frac{2\sqrt{15}}{3} \)
Examples Involving Variables
- Example 1: Simplify \( \sqrt{12x^2y^4} \)
\( \sqrt{12x^2y^4} = \sqrt{4 \cdot 3 \cdot x^2 \cdot y^4} = 2xy^2\sqrt{3} \)
- Example 2: Simplify \( \sqrt{54a^{10}b^{16}c^7} \)
\( \sqrt{54a^{10}b^{16}c^7} = 3a^5b^8c^3\sqrt{6c} \)
By following these steps and rules, you can simplify any radical expression, making your algebra work cleaner and easier to manage.

READ MORE:
Introduction to Radical Expressions
Radical expressions are mathematical expressions that involve roots, such as square roots or cube roots. In algebra, simplifying radical expressions is an essential skill that helps in solving equations and understanding functions. This process involves rewriting the expression in its simplest form. Below, we outline the key concepts and steps involved in simplifying radical expressions.
Radical expressions include a radicand (the number or expression inside the radical symbol) and an index (which indicates the root to be taken). The most common radical is the square root, but other roots like cube roots are also used.
To simplify radical expressions:
- Identify and factor perfect squares or cubes: Look for factors that are perfect squares (e.g., 4, 9, 16) or perfect cubes (e.g., 8, 27) within the radicand.
- Apply the product rule for radicals: For any non-negative numbers \(a\) and \(b\), \( \sqrt{ab} = \sqrt{a} \cdot \sqrt{b} \). This allows us to break down the radicand into simpler components.
- Combine like terms: After breaking down the radicand, combine like terms and simplify further if possible.
- Rationalize the denominator: If the radical expression has a radical in the denominator, multiply the numerator and denominator by a conjugate to remove the radical.
Let's go through a step-by-step example:
Example: Simplify \( \sqrt{72} \)
- Identify perfect square factors of 72: \( 72 = 36 \times 2 \).
- Apply the product rule: \( \sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \cdot \sqrt{2} \).
- Simplify the expression: \( \sqrt{36} = 6 \), so \( \sqrt{72} = 6\sqrt{2} \).
This method can be applied to more complex radical expressions involving variables and higher indices.
By mastering these steps, you can simplify radical expressions efficiently and accurately, making it easier to solve algebraic problems.
Prime Factorization and Radicals
Prime factorization is a key technique in simplifying radical expressions. It involves breaking down a number into its prime factors, which can then be used to simplify the radical expression.
Here's a step-by-step process:
- Find Prime Factors: Break down the number under the radical (radicand) into its prime factors.
- Group Factors: Group the prime factors into pairs.
- Simplify: Each pair of prime factors can be simplified into a single number outside the radical.
Let's look at an example:
| Example: |
| \(\sqrt{72}\) |
| Step 1: Prime factorize 72: \(72 = 2 \times 2 \times 2 \times 3 \times 3\) |
| Step 2: Group the factors: \(\sqrt{(2 \times 2) \times (2 \times 3 \times 3)}\) |
| Step 3: Simplify the pairs: \(2 \times 3 \sqrt{2} = 6\sqrt{2}\) |
This process can be applied to any radical expression to simplify it by extracting perfect squares.
Here are some additional examples:
- \(\sqrt{98} = \sqrt{2 \times 7 \times 7} = 7\sqrt{2}\)
- \(\sqrt{200} = \sqrt{2 \times 2 \times 2 \times 5 \times 5} = 10\sqrt{2}\)
- \(\sqrt{48} = \sqrt{2 \times 2 \times 2 \times 2 \times 3} = 4\sqrt{3}\)
By understanding and applying prime factorization, you can simplify even the most complex radical expressions with ease.
Product and Quotient Rules
The product and quotient rules for radicals are fundamental tools in simplifying radical expressions. These rules help break down complex radicals into simpler forms.
Product Rule
The product rule states that the radical of a product is equal to the product of the radicals. Mathematically, this is represented as:
\[
\sqrt[n]{a \cdot b} = \sqrt[n]{a} \cdot \sqrt[n]{b}
\]
where \( n \) is a positive integer and \( a \) and \( b \) are non-negative real numbers.
Example:
Simplify \(\sqrt{24}\).
First, factor 24 into prime factors:
\[
\sqrt{24} = \sqrt{4 \cdot 6} = \sqrt{4} \cdot \sqrt{6} = 2\sqrt{6}
\]
Quotient Rule
The quotient rule states that the radical of a quotient is equal to the quotient of the radicals. Mathematically, this is represented as:
\[
\sqrt[n]{\frac{a}{b}} = \frac{\sqrt[n]{a}}{\sqrt[n]{b}}
\]
where \( n \) is a positive integer and \( a \) and \( b \) are non-negative real numbers.
Example:
Simplify \(\sqrt{\frac{12}{5}}\).
First, separate the radical into the numerator and the denominator:
\[
\sqrt{\frac{12}{5}} = \frac{\sqrt{12}}{\sqrt{5}}
\]
Then, simplify the numerator:
\[
\sqrt{12} = \sqrt{4 \cdot 3} = 2\sqrt{3}
\]
So, the simplified form is:
\[
\sqrt{\frac{12}{5}} = \frac{2\sqrt{3}}{\sqrt{5}}
\]
For further simplification, rationalize the denominator:
\[
\frac{2\sqrt{3}}{\sqrt{5}} \times \frac{\sqrt{5}}{\sqrt{5}} = \frac{2\sqrt{15}}{5}
\]
Key Points to Remember
- Only use the product and quotient rules for multiplication and division, not for addition or subtraction.
- Simplify inside the radical as much as possible before applying the rules.
- When dealing with variables, ensure that they are positive to avoid complications with absolute values.
These rules are essential for simplifying radical expressions and are widely used in solving algebraic equations involving radicals.
Simplifying Radical Expressions with Variables
In algebra, simplifying radical expressions involving variables follows similar principles as simplifying numerical radicals. Here are the steps:
- Combine Like Terms: Simplify any expressions under the radical by combining like terms.
- Factorization: Factor the expression inside the radical completely.
- Apply Radical Rules: Use properties of radicals to simplify. For example, \( \sqrt{ab} = \sqrt{a} \cdot \sqrt{b} \) and \( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \).
- Rationalize the Denominator: If needed, eliminate radicals from the denominator by multiplying numerator and denominator by the conjugate of the denominator.
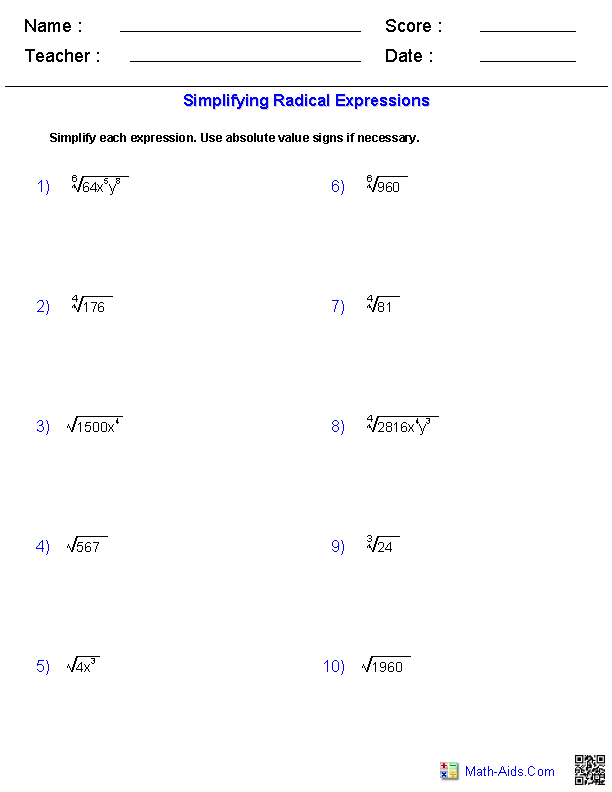
Examples of Simplifying Radicals
Here are some examples demonstrating the simplification of radical expressions:
- Simplify \( \sqrt{18} \):
- Simplify \( \sqrt{12x^2} \):
- Simplify \( \frac{\sqrt{27}}{\sqrt{3}} \):
| Step 1: | Identify the factors of 18: \( \sqrt{18} = \sqrt{9 \cdot 2} \). |
| Step 2: | Separate into perfect squares: \( \sqrt{9 \cdot 2} = \sqrt{9} \cdot \sqrt{2} \). |
| Step 3: | Compute the square root: \( \sqrt{9} = 3 \), so \( \sqrt{18} = 3\sqrt{2} \). |
| Step 1: | Factor out perfect squares: \( \sqrt{12x^2} = \sqrt{4 \cdot 3 \cdot x^2} \). |
| Step 2: | Separate into factors: \( \sqrt{4 \cdot 3 \cdot x^2} = 2x\sqrt{3} \). |
| Step 1: | Apply the quotient rule: \( \frac{\sqrt{27}}{\sqrt{3}} = \sqrt{\frac{27}{3}} \). |
| Step 2: | Simplify the fraction under the radical: \( \sqrt{\frac{27}{3}} = \sqrt{9} = 3 \). |
Simplifying Radicals Involving Variables
When simplifying radical expressions that involve variables, follow these steps:
- Factorization: Factor the expression under the radical completely.
- Use Properties of Radicals: Apply rules such as \( \sqrt{ab} = \sqrt{a} \cdot \sqrt{b} \) and \( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \).
- Simplify as Much as Possible: Combine like terms and simplify the coefficients.
- Rationalize if Needed: Eliminate radicals from the denominator using conjugates if necessary.
Special Cases and Common Mistakes
Simplifying radical expressions can sometimes be tricky due to special cases and common mistakes. Understanding these can help avoid errors and ensure accurate solutions.
Special Cases
- Perfect Squares and Cubes: When simplifying radicals, if the number under the radical is a perfect square (e.g., 4, 9, 16) or perfect cube (e.g., 8, 27), simplify directly. For example, \(\sqrt{16} = 4\) and \(\sqrt[3]{27} = 3\).
- Variables with Even Exponents: Variables raised to even exponents are perfect squares and can be simplified. For instance, \(\sqrt{x^4} = x^2\).
- Negative Radicands: Radicals with negative numbers under the square root sign are not real numbers. They are handled using imaginary numbers. For example, \(\sqrt{-9} = 3i\), where \(i\) is the imaginary unit.
- Nested Radicals: Sometimes, you encounter nested radicals. Simplify the inner radical first. For example, \(\sqrt{2 + \sqrt{3}}\).
Common Mistakes
- Incorrectly Simplifying Non-Perfect Squares: Don't simplify non-perfect squares directly. For example, \(\sqrt{20} \neq 2\sqrt{5}\) immediately without factoring. Instead, factor into \(\sqrt{4 \times 5} = 2\sqrt{5}\).
- Leaving Radicals in the Denominator: Always rationalize the denominator by multiplying the numerator and denominator by the conjugate if necessary. For example, \(\frac{1}{\sqrt{2}} = \frac{\sqrt{2}}{2}\).
- Combining Radicals Incorrectly: Remember that \(\sqrt{a} + \sqrt{b} \neq \sqrt{a + b}\). Radicals can only be combined when they have the same radicand.
- Forgetting to Simplify Completely: Always ensure that the radicand is simplified as much as possible. For instance, \(\sqrt{50} = \sqrt{25 \times 2} = 5\sqrt{2}\).
- Mistakes with Variables: When variables are involved, ensure that exponents are handled correctly. For example, \(\sqrt{x^6} = x^3\), not \(x^2\sqrt{x}\).
Examples
Let's look at a few examples to illustrate these points:
- Simplify \(\sqrt{75}\):
- Simplify \(\frac{3}{\sqrt{7}}\):
- Simplify \(\sqrt{50x^2}\):
\[
\sqrt{75} = \sqrt{25 \times 3} = 5\sqrt{3}
\]
\[
\frac{3}{\sqrt{7}} \times \frac{\sqrt{7}}{\sqrt{7}} = \frac{3\sqrt{7}}{7}
\]
\[
\sqrt{50x^2} = \sqrt{25 \times 2 \times x^2} = 5x\sqrt{2}
\]
By keeping these special cases and common mistakes in mind, you can simplify radical expressions accurately and efficiently.
Practice Problems and Solutions
Practicing with problems is essential to mastering the simplification of radical expressions. Below are a variety of problems along with step-by-step solutions to help you understand the process.
Problem 1: Simplify \( \sqrt{72} \)
- Find the largest perfect square factor of 72: \( 36 \times 2 \).
- Rewrite the expression using the product rule: \( \sqrt{72} = \sqrt{36 \times 2} \).
- Simplify the square root of the perfect square: \( \sqrt{36} = 6 \).
- Combine the results: \( \sqrt{72} = 6\sqrt{2} \).
Solution: \( \sqrt{72} = 6\sqrt{2} \)
Problem 2: Simplify \( \sqrt{50} \)
- Find the largest perfect square factor of 50: \( 25 \times 2 \).
- Rewrite the expression using the product rule: \( \sqrt{50} = \sqrt{25 \times 2} \).
- Simplify the square root of the perfect square: \( \sqrt{25} = 5 \).
- Combine the results: \( \sqrt{50} = 5\sqrt{2} \).
Solution: \( \sqrt{50} = 5\sqrt{2} \)
Problem 3: Simplify \( \sqrt{45} \)
- Find the largest perfect square factor of 45: \( 9 \times 5 \).
- Rewrite the expression using the product rule: \( \sqrt{45} = \sqrt{9 \times 5} \).
- Simplify the square root of the perfect square: \( \sqrt{9} = 3 \).
- Combine the results: \( \sqrt{45} = 3\sqrt{5} \).
Solution: \( \sqrt{45} = 3\sqrt{5} \)
Problem 4: Simplify \( \sqrt{12x^2y^4} \)
- Find the largest perfect square factor of 12: \( 4 \times 3 \).
- Rewrite the expression using the product rule: \( \sqrt{12x^2y^4} = \sqrt{4 \times 3 \times x^2 \times y^4} \).
- Simplify the square roots: \( \sqrt{4} = 2 \), \( \sqrt{x^2} = x \), \( \sqrt{y^4} = y^2 \).
- Combine the results: \( \sqrt{12x^2y^4} = 2xy^2\sqrt{3} \).
Solution: \( \sqrt{12x^2y^4} = 2xy^2\sqrt{3} \)
Problem 5: Simplify \( \sqrt{18} \)
- Find the largest perfect square factor of 18: \( 9 \times 2 \).
- Rewrite the expression using the product rule: \( \sqrt{18} = \sqrt{9 \times 2} \).
- Simplify the square root of the perfect square: \( \sqrt{9} = 3 \).
- Combine the results: \( \sqrt{18} = 3\sqrt{2} \).
Solution: \( \sqrt{18} = 3\sqrt{2} \)
Problem 6: Simplify \( \sqrt{128} \)
- Find the largest perfect square factor of 128: \( 64 \times 2 \).
- Rewrite the expression using the product rule: \( \sqrt{128} = \sqrt{64 \times 2} \).
- Simplify the square root of the perfect square: \( \sqrt{64} = 8 \).
- Combine the results: \( \sqrt{128} = 8\sqrt{2} \).
Solution: \( \sqrt{128} = 8\sqrt{2} \)

Advanced Techniques in Simplifying Radicals
In this section, we will explore some advanced techniques for simplifying radical expressions. These methods are useful when dealing with more complex expressions involving radicals.
1. Simplifying Radicals with Higher-Order Roots
When dealing with radicals that have higher-order roots, such as cube roots or fourth roots, the process is similar to that for square roots but requires handling higher powers. Here’s an example:
- Identify and factorize the radicand (the number inside the radical).
- Group the factors based on the root being considered.
- Extract the grouped factors from the radical.
Example: Simplify \( \sqrt[4]{16x^9y^3} \)
- Factorize the radicand: \( 16x^9y^3 = (2^4)(x^8)(x)(y^3) \)
- Group the factors: \( \sqrt[4]{(2^4)(x^8)(x)(y^3)} \)
- Extract the grouped factors: \( 2x^2 \sqrt[4]{xy^3} \)
2. Rational Exponents
Using rational exponents can sometimes simplify the process of dealing with radicals. A rational exponent represents both an exponent and a root. For example, \( x^{m/n} = \sqrt[n]{x^m} \).
Example: Simplify \( x^{3/2} \cdot x^{1/2} \)
- Add the exponents: \( x^{(3/2) + (1/2)} = x^{4/2} = x^2 \)
- The simplified form is \( x^2 \)
3. Multiplying and Dividing Radical Expressions
When multiplying or dividing radicals, use the properties of exponents and roots:
- Product Rule: \( \sqrt[n]{a} \cdot \sqrt[n]{b} = \sqrt[n]{ab} \)
- Quotient Rule: \( \sqrt[n]{\frac{a}{b}} = \frac{\sqrt[n]{a}}{\sqrt[n]{b}} \)
Example: Simplify \( \sqrt[3]{8x^6} \cdot \sqrt[3]{4x^3} \)
- Multiply the radicands: \( \sqrt[3]{8x^6 \cdot 4x^3} = \sqrt[3]{32x^9} \)
- Extract the cube roots: \( \sqrt[3]{32} \cdot \sqrt[3]{x^9} = 2x^3 \)
4. Handling Complex Radicals
For radicals involving complex expressions, break down the problem step-by-step:
Example: Simplify \( \sqrt{5 + 2\sqrt{6}} \)
- Assume the simplified form is \( \sqrt{a} + \sqrt{b} \).
- Square both sides to solve for \( a \) and \( b \): \( 5 + 2\sqrt{6} = a + b + 2\sqrt{ab} \).
- Compare rational and irrational parts to find \( a \) and \( b \).
- Simplify accordingly.
Through practice, identifying patterns and common techniques becomes easier.
5. Using Conjugates
To simplify expressions with radicals in the denominator, multiply by the conjugate:
Example: Simplify \( \frac{1}{\sqrt{3} + \sqrt{2}} \)
- Multiply numerator and denominator by the conjugate: \( \frac{1}{\sqrt{3} + \sqrt{2}} \cdot \frac{\sqrt{3} - \sqrt{2}}{\sqrt{3} - \sqrt{2}} \)
- Apply the difference of squares: \( \frac{\sqrt{3} - \sqrt{2}}{3 - 2} = \sqrt{3} - \sqrt{2} \)
By mastering these advanced techniques, you can confidently tackle any radical expression you encounter.
Conclusion and Summary
In this guide, we have explored the comprehensive methods of simplifying radical expressions in Algebra 2. Let's summarize the key points and techniques covered:
- Understanding Radical Expressions: We began by defining radicals and discussing their properties. We learned that a radical expression includes a root, such as a square root or cube root.
- Prime Factorization: Prime factorization is a crucial step in simplifying radicals. By breaking down a number into its prime factors, we can identify perfect squares and simplify the expression.
- Product and Quotient Rules: The product rule states that the square root of a product is equal to the product of the square roots of the factors. Similarly, the quotient rule states that the square root of a quotient is equal to the quotient of the square roots of the numerator and the denominator.
- Simplifying Radicals with Variables: When dealing with variables, it's essential to apply the product and quotient rules while considering the properties of exponents. We assume variables are positive to avoid complications with absolute values.
- Rationalizing the Denominator: To rationalize the denominator, we multiply the numerator and the denominator by a suitable radical that eliminates the radical in the denominator, making the expression rational.
- Special Cases and Common Mistakes: Special cases include dealing with higher-index roots and negative radicands, which may lead to imaginary numbers. Common mistakes often involve incorrect application of rules or overlooking perfect square factors.
- Practice Problems: Engaging with practice problems helps solidify the concepts and techniques. Solutions to these problems provide a step-by-step approach to ensure a thorough understanding.
- Advanced Techniques: Advanced techniques include simplifying complex radicals, using the properties of exponents effectively, and handling higher-index roots with more precision.
By mastering these techniques, you can simplify any radical expression with confidence and accuracy. Always remember to break down the problem into manageable steps, identify perfect square factors, and apply the product and quotient rules consistently. Happy simplifying!
Đơn Giản Hóa Biểu Thức Dưới Dạng Căn Với Biến Số, Số Mũ, Phân Số, Căn Bậc Ba - Đại Số
READ MORE:
Đơn Giản Hóa Biểu Thức Dưới Dạng Căn Với Biến Số