How to Get Rid of X Squared: Simple Solutions to Master Quadratic Equations

When solving equations involving x^2, there are several methods you can use depending on the context of the problem. Here are some common strategies:

1. Simplify and Combine Like Terms

Sometimes, you can eliminate the x^2 terms by simplifying and combining like terms.

1. Start with the equation: y + 2x^2 - 5 = 2(x^2 + 2)
2. Simplify the right side: y + 2x^2 - 5 = 2x^2 + 4
3. Subtract 2x^2 from both sides: y - 5 = 4
4. Solve for y: y = 9

2. Factoring

Factoring is useful for quadratic equations. For example:

1. Start with x^2 - 5x + 6 = 0
2. Factor the equation: (x - 2)(x - 3) = 0
3. Solve for x: x = 2 or x = 3

3. Using the Quadratic Formula

The quadratic formula is a reliable method for solving any quadratic equation:

Example:

1. For 2x^2 - 4x - 6 = 0, use a = 2, b = -4, and c = -6
2. Calculate the discriminant: b^2 - 4ac = (-4)^2 - 4(2)(-6) = 16 + 48 = 64
3. Apply the formula: x = \frac{{4 \pm 8}}{4}, giving x = 3 or x = -1

4. Completing the Square

This method transforms the quadratic equation into a perfect square trinomial:

1. Start with x^2 + 6x + 5 = 0
2. Rearrange to isolate the constant term: x^2 + 6x = -5
3. Add the square of half the coefficient of x to both sides: x^2 + 6x + 9 = 4
4. Factor the left side: (x + 3)^2 = 4
5. Take the square root of both sides: x + 3 = \pm 2
6. Solve for x: x = -1 or x = -5

5. Using Graphical Methods

Graphing the equation can provide a visual solution. Find the points where the graph intersects the x-axis.

These methods cover a wide range of approaches to removing or solving for x^2 in algebraic equations. Practice using each method to understand which one is most effective for different types of problems.

Solving equations with exponents, such as \(x^2\), can be challenging, but with the right approach, it becomes manageable. The key is to use algebraic methods to isolate the variable and eliminate the exponent. This process involves several steps, including combining like terms, factoring, and using the properties of exponents. Below, we outline the most effective strategies to get rid of \(x^2\) in equations.

1. Simplify and Combine Like Terms

Look for opportunities where exponent terms cancel each other out. For instance, in the equation \(y + 2x^2 - 5 = 2(x^2 + 2)\), simplify to get \(y + 2x^2 - 5 = 2x^2 + 4\). Subtract \(2x^2\) from both sides to remove the exponent, resulting in \(y - 5 = 4\), and solve for \(y\).

2. Use Inverse Operations

Apply inverse operations such as taking square roots or squaring both sides of the equation. For example, to solve \(\sqrt{x} + 1 = 5\), isolate the square root and square both sides to get \(x = 16\).

3. Factorization

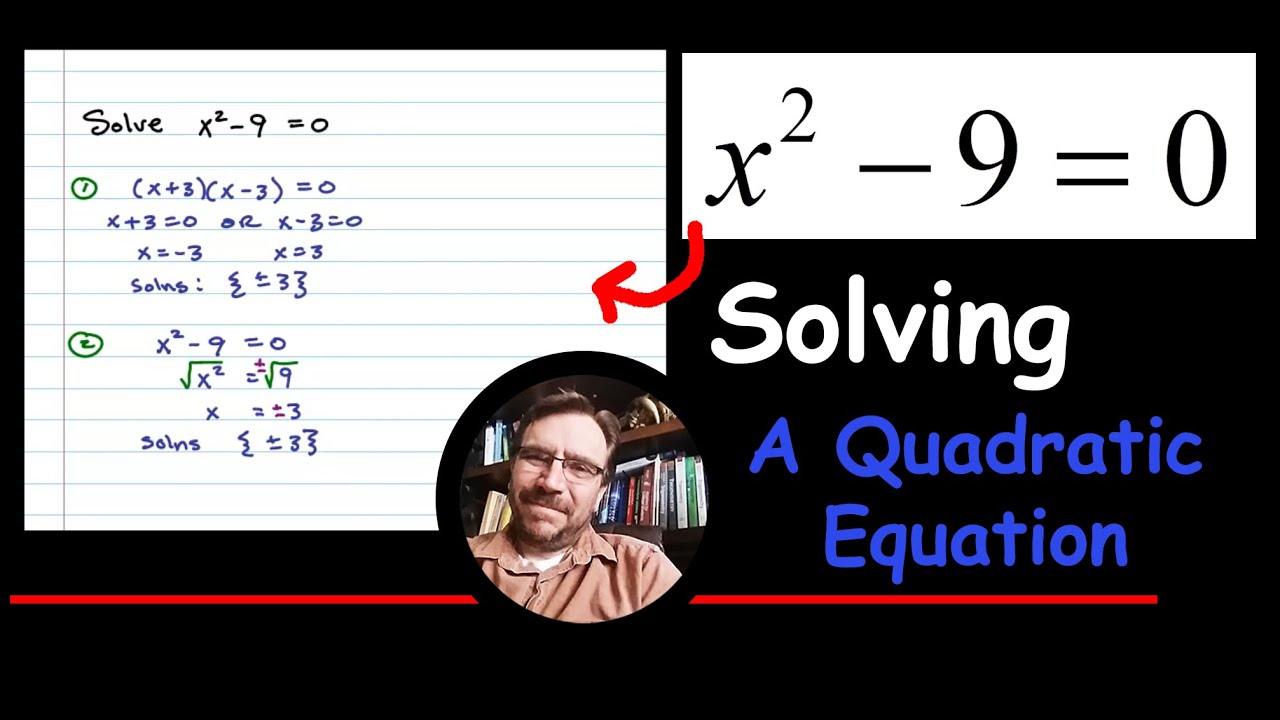
Identify and factor common polynomials. Use formulas like \(a^2 - b^2 = (a - b)(a + b)\) to simplify and solve the equation. For example, if the equation is \(x^2 - 9 = 0\), factor to \((x - 3)(x + 3) = 0\) and solve for \(x\).

4. Quadratic Formula

For more complex equations, use the quadratic formula \(x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}\). This is especially useful when the equation cannot be easily factored. For instance, in \(4x^2 + 8 = 24\), simplify to \(4x^2 = 16\), then \(x^2 = 4\), and solve for \(x = \pm 2\).

Squaring and square roots are fundamental concepts in mathematics that often appear in equations and problem-solving scenarios. Here's a detailed explanation to help you understand these concepts and how to work with them.

• What is Squaring?

  Squaring a number means multiplying it by itself. For example, the square of \( x \) is written as \( x^2 \) and is equal to \( x \times x \). If \( x = 3 \), then \( x^2 = 3 \times 3 = 9 \).

• What is a Square Root?

  The square root of a number is a value that, when multiplied by itself, gives the original number. It is denoted by the radical symbol \( \sqrt{} \). For example, the square root of 9 is 3, because \( 3 \times 3 = 9 \). This is written as \( \sqrt{9} = 3 \).

• Properties of Squares and Square Roots

  • Non-Negativity: For any real number \( x \), \( x^2 \geq 0 \) and \( \sqrt{x} \) is non-negative.
  • Inverses: Squaring and taking the square root are inverse operations. Thus, \( \sqrt{x^2} = x \) and \( (\sqrt{x})^2 = x \).

• Solving Equations Involving Squares and Square Roots

  To solve equations involving squares and square roots, follow these steps:

  1. Isolate the Square or Square Root: Move all terms involving squares or square roots to one side of the equation.
  2. Square Both Sides (if necessary): If the equation involves a square root, square both sides to eliminate the radical. For example, if \( \sqrt{x} = 3 \), then square both sides to get \( x = 9 \).
  3. Simplify and Solve: Simplify the resulting equation and solve for the variable. Be sure to check your solutions by substituting them back into the original equation to avoid extraneous solutions.

Understanding these basic principles of squaring and square roots is crucial for solving various mathematical problems and equations effectively.

Removing \( x^2 \) from equations can be crucial for solving various algebraic problems. Here are some effective methods to achieve this:

• Isolate and Square Root Method:
  1. Isolate the \( x^2 \) term on one side of the equation.
  2. Take the square root of both sides to solve for \( x \). Remember to consider both positive and negative roots.

  Example: Solve \( x^2 = 16 \). The solution is \( x = \pm 4 \).

• Completing the Square:
  1. Rearrange the equation in the form \( x^2 + bx + c = 0 \).
  2. Add and subtract the square of half the coefficient of \( x \) inside the equation.
  3. Rewrite the equation as a perfect square trinomial and solve for \( x \).

  Example: Solve \( x^2 - 4x + 4 = 0 \). The solution is \( x = 2 \).

• Quadratic Formula:
  1. Use the quadratic formula \( x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a} \) where \( ax^2 + bx + c = 0 \).
  2. Plug in the values of \( a \), \( b \), and \( c \) to find the values of \( x \).

  Example: Solve \( 2x^2 - 4x - 6 = 0 \). The solutions are \( x = 3 \) and \( x = -1 \).

By mastering these methods, you can effectively remove \( x^2 \) from equations and find solutions to various algebraic problems.

Eliminating x² from equations involves understanding the underlying principles of algebra and applying various techniques effectively. Below are step-by-step methods to achieve this:

1. Simplify and Combine Like Terms:

   Identify terms with x² and combine them if possible. For instance, in the equation \( y + 2x^2 - 5 = 2(x^2 + 2) \), simplify to get:

   \[ y + 2x^2 - 5 = 2x^2 + 4 \]

   Subtract \(2x^2\) from both sides to eliminate the squared term:

   \[ y - 5 = 4 \]

   Then solve for \( y \):

   \[ y = 9 \]

2. Factoring:

   Factorize polynomials to simplify the equation. Common factoring formulas are essential tools. For example:

   For the equation \( x^2 - 5x + 6 = 0 \), factorize to get:

   \[ (x - 2)(x - 3) = 0 \]

   Solve each factor for \( x \):

   \[ x - 2 = 0 \quad \text{or} \quad x - 3 = 0 \]

   Thus, \( x = 2 \) or \( x = 3 \).

3. Using the Quadratic Formula:

   For any quadratic equation of the form \( ax^2 + bx + c = 0 \), use the quadratic formula:

   \[ x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a} \]

   For example, solving \( 2x^2 + 3x - 2 = 0 \):

   \[ a = 2, \, b = 3, \, c = -2 \]

   Compute the discriminant \( \Delta \):

   \[ \Delta = b^2 - 4ac = 3^2 - 4 \cdot 2 \cdot (-2) = 9 + 16 = 25 \]

   Thus, the solutions are:

   \[ x = \frac{-3 \pm \sqrt{25}}{4} \]

   \[ x = \frac{-3 + 5}{4} = \frac{2}{4} = 0.5 \quad \text{and} \quad x = \frac{-3 - 5}{4} = \frac{-8}{4} = -2 \]

4. Completing the Square:

   This method transforms the equation into a perfect square trinomial. For example, to solve \( x^2 + 6x + 5 = 0 \):

   Rewrite the equation: \( x^2 + 6x = -5 \)

   Add and subtract \(\left(\frac{b}{2}\right)^2\) (where \( b \) is the coefficient of \( x \)):

   \[ x^2 + 6x + 9 = 4 \]

   Which can be written as:

   \[ (x + 3)^2 = 4 \]

   Take the square root of both sides:

   \[ x + 3 = \pm 2 \]

   Thus, \( x = -1 \) or \( x = -5 \).

These methods provide robust tools for solving equations involving \( x^2 \). Practice and familiarity with these techniques will enhance your problem-solving skills in algebra.

Understanding common mistakes when dealing with equations involving \( x^2 \) can save you time and frustration. Here are some frequent errors and tips to avoid them:

• Forgetting the Square Root Property:

  When you square both sides of an equation, it's crucial to remember that you might introduce extraneous solutions. Always check your solutions in the original equation.

• Misapplying the Distribution Property:

  Ensure correct distribution when dealing with expressions such as \((a + b)^2\). Remember that \((a + b)^2 = a^2 + 2ab + b^2\), not \(a^2 + b^2\).

• Incorrectly Simplifying Radicals:

  When dealing with radicals, be careful to simplify correctly. For instance, the square root of a sum, \(\sqrt{a + b}\), is not the same as \(\sqrt{a} + \sqrt{b}\).

• Linear Function Assumptions:

  Not all functions are linear. Avoid assuming that functions like \((x + y)^2 = x^2 + y^2\). Check for additional terms such as \(2xy\) in expansions.

• Equals Sign Misuse:

  When manipulating equations, ensure you understand the function's definition and context. Overuse of "doing the same thing to both sides" can lead to incorrect simplifications.

Practicing with examples and solving problems is a great way to understand how to eliminate \( x^2 \) from equations. Below are step-by-step solutions to various quadratic equations using different methods.

• Example 1: Solve \( x^2 = 25 \)

  Solution:

  1. Take the square root of both sides: \( x = \pm \sqrt{25} \)
  2. Simplify the square root: \( x = \pm 5 \)

• Example 2: Solve \( 3x^2 + 7 = 55 \)

  Solution:

  1. Subtract 7 from both sides: \( 3x^2 = 48 \)
  2. Divide both sides by 3: \( x^2 = 16 \)
  3. Take the square root of both sides: \( x = \pm 4 \)

• Example 3: Solve \( -2x^2 + 15 = x^2 - 12 \)

  Solution:

  1. Combine like terms: \( -2x^2 - x^2 = -12 - 15 \)
  2. Simplify: \( -3x^2 = -27 \)
  3. Divide both sides by -3: \( x^2 = 9 \)
  4. Take the square root of both sides: \( x = \pm 3 \)

• Example 4: Solve \( 7(-x^2 + 6) - 1 = -17 \)

  Solution:

  1. Distribute 7: \( -7x^2 + 42 - 1 = -17 \)
  2. Simplify: \( -7x^2 + 41 = -17 \)
  3. Subtract 41 from both sides: \( -7x^2 = -58 \)
  4. Divide both sides by -7: \( x^2 = \frac{58}{7} \)
  5. Take the square root of both sides: \( x = \pm \sqrt{\frac{58}{7}} \)

• Example 5: Solve \( x^2 + 3x + 9 = 5x - 8 \)

  Solution:

  1. Rewrite in standard form: \( x^2 - 2x + 17 = 0 \)
  2. Identify coefficients: \( a = 1 \), \( b = -2 \), \( c = 17 \)
  3. Apply the quadratic formula: \( x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a} \)
  4. Calculate: \( x = \frac{2 \pm \sqrt{-64}}{2} \)
  5. Simplify: \( x = 1 \pm 4i \)

Working through these examples will help solidify your understanding of eliminating \( x^2 \) and solving quadratic equations. Try solving similar problems to improve your skills further.

When dealing with complex equations involving \(x^2\), advanced techniques can be employed to simplify and solve these equations. Here, we explore several methods that are useful for handling these complex scenarios.

1. Factoring Complex Polynomials

For polynomials where \(x^2\) is part of a higher degree equation, factoring can simplify the equation. Consider the polynomial equation:

\(x^4 - 5x^2 + 4 = 0\)

By substituting \(y = x^2\), the equation becomes:

\(y^2 - 5y + 4 = 0\)

Factor the quadratic equation:

\((y - 4)(y - 1) = 0\)

Re-substitute \(x^2\) for \(y\):

\(x^2 = 4\) or \(x^2 = 1\)

Solve for \(x\):

\(x = \pm 2\) or \(x = \pm 1\)

2. Completing the Square

For equations that are not easily factorable, completing the square is a powerful method. Take the equation:

\(x^2 + 6x + 5 = 0\)

Rewrite the equation by adding and subtracting a suitable constant:

\(x^2 + 6x + 9 - 4 = 0\)

This can be simplified to:

\((x + 3)^2 = 4\)

Take the square root of both sides:

\(x + 3 = \pm 2\)

Solve for \(x\):

\(x = -1\) or \(x = -5\)

3. Using the Quadratic Formula

For any quadratic equation of the form \(ax^2 + bx + c = 0\), the quadratic formula provides a straightforward solution:

\(x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}\)

Consider the equation \(2x^2 - 4x - 6 = 0\). Using the quadratic formula:

\(x = \frac{4 \pm \sqrt{(-4)^2 - 4 \cdot 2 \cdot (-6)}}{2 \cdot 2}\)

Simplify inside the square root:

\(x = \frac{4 \pm \sqrt{64}}{4}\)

Which results in:

\(x = \frac{4 \pm 8}{4}\)

Thus, \(x = 3\) or \(x = -1\)

4. Substitution Methods for Higher Degree Equations

For higher degree equations or equations involving multiple variables, substitution can reduce the complexity. Consider the system:

\[
\begin{cases}
x^2 + y^2 = 25 \\
x + y = 7
\end{cases}
\]

Solve for one variable in terms of the other:

\(y = 7 - x\)

Substitute into the first equation:

\(x^2 + (7 - x)^2 = 25\)

Expand and simplify:

\(x^2 + 49 - 14x + x^2 = 25\)

Combine like terms:

\(2x^2 - 14x + 24 = 0\)

Divide by 2:

\(x^2 - 7x + 12 = 0\)

Factorize:

\((x - 3)(x - 4) = 0\)

So, \(x = 3\) or \(x = 4\)

Substitute back to find \(y\):

If \(x = 3\), then \(y = 4\); if \(x = 4\), then \(y = 3\)

5. Applying Numerical Methods

When exact solutions are difficult to find, numerical methods such as Newton's method can approximate the solutions. For an equation like \(x^2 - 2 = 0\), we can use the iterative formula:

\(x_{n+1} = x_n - \frac{f(x_n)}{f'(x_n)}\)

Where \(f(x) = x^2 - 2\) and \(f'(x) = 2x\). Starting with an initial guess \(x_0\), iterate using:

\(x_{n+1} = x_n - \frac{x_n^2 - 2}{2x_n}\)

For instance, if we start with \(x_0 = 1.5\), the iterations proceed as follows:

1. \(x_1 = 1.5 - \frac{1.5^2 - 2}{2 \cdot 1.5} = 1.4167\)
2. \(x_2 = 1.4167 - \frac{1.4167^2 - 2}{2 \cdot 1.4167} = 1.4142\)
3. Continue until desired accuracy is achieved.

Each of these techniques provides a different approach to solving complex equations involving \(x^2\). Mastering these methods will enhance your ability to tackle a wide range of mathematical problems.

After solving equations involving \(x^2\), it is crucial to verify the accuracy of your solutions. This ensures that the answers are correct and consistent with the original equation. Here are several steps and methods to check your solutions effectively:

1. Substitution Method

The simplest way to check if your solution is correct is by substituting the values of \(x\) back into the original equation.

For example, consider the equation:

\(x^2 - 5x + 6 = 0\)

If you found the solutions \(x = 2\) and \(x = 3\), substitute these values back into the equation:

• For \(x = 2\): \(2^2 - 5 \cdot 2 + 6 = 4 - 10 + 6 = 0\)
• For \(x = 3\): \(3^2 - 5 \cdot 3 + 6 = 9 - 15 + 6 = 0\)

Both values satisfy the equation, confirming that the solutions are correct.

2. Graphical Method

Another method to check your solutions is by graphing the equation and visually inspecting the points where the curve intersects the x-axis.

For instance, graph the equation \(x^2 - 5x + 6\). The x-intercepts of the graph represent the solutions to the equation.

You can plot the graph using a graphing calculator or software to confirm that the points \(x = 2\) and \(x = 3\) are where the curve crosses the x-axis.

3. Analytical Re-check

Revisit the steps you took to solve the equation. This includes:

• Verifying the factorization if you used factoring.
• Rechecking the completion of the square process.
• Ensuring you applied the quadratic formula correctly.

For example, if you solved \(x^2 - 5x + 6 = 0\) by factoring:

\(x^2 - 5x + 6 = (x - 2)(x - 3)\)

Confirm that the factors multiply back to the original equation:

\((x - 2)(x - 3) = x^2 - 3x - 2x + 6 = x^2 - 5x + 6\)

Since the factorization is correct, the solutions are verified.

4. Residual Analysis

Calculate the residuals, which are the differences between the left and right sides of the equation when your solutions are substituted.

For the equation \(x^2 - 5x + 6 = 0\) and solutions \(x = 2\) and \(x = 3\):

• For \(x = 2\): \(2^2 - 5 \cdot 2 + 6 = 0\), residual = \(0\)
• For \(x = 3\): \(3^2 - 5 \cdot 3 + 6 = 0\), residual = \(0\)

Zero residuals indicate accurate solutions.

5. Use of Software and Calculators

Utilize mathematical software or online calculators to double-check your solutions. These tools can solve equations and provide quick verification.

Input the equation \(x^2 - 5x + 6 = 0\) into a tool like WolframAlpha or a graphing calculator to confirm that the solutions are \(x = 2\) and \(x = 3\).

6. Peer Review and Collaboration

Discussing your solutions with peers or a mentor can provide additional verification. Different perspectives can uncover mistakes or confirm the correctness of your work.

Explain your method and results to someone else and see if they reach the same conclusion.

By employing these methods, you can confidently verify the accuracy of your solutions to equations involving \(x^2\). This comprehensive approach ensures that your answers are correct and reliable.

Understanding how to handle and simplify equations involving \(x^2\) is a fundamental skill in algebra and higher mathematics. Throughout this guide, we have explored various methods to effectively eliminate or solve for \(x^2\) in equations. Here, we summarize the key points and strategies discussed:

Key Takeaways

• Basic Techniques: Start with foundational methods such as factoring, using the quadratic formula, and completing the square. These are essential tools for solving most quadratic equations.
• Advanced Methods: For more complex equations, techniques like polynomial factorization, substitution methods, and numerical approaches (e.g., Newton's method) provide robust solutions.
• Verification: Always verify your solutions through substitution, graphical analysis, and residual checks. This ensures the accuracy and reliability of your results.

Practical Applications

Solving equations with \(x^2\) is not just an academic exercise but a practical skill used in various fields, including physics, engineering, and economics. Mastery of these techniques allows you to tackle real-world problems involving quadratic relationships.

For example, the trajectory of a projectile, which is modeled by a quadratic equation, can be analyzed and optimized using the methods discussed. Similarly, understanding the behavior of functions involving \(x^2\) is crucial in calculus and higher-level mathematics.

Steps for Continuous Learning

1. Practice Regularly: Regularly solve different types of quadratic equations to strengthen your understanding and problem-solving skills.
2. Explore Further: Delve into more complex topics, such as solving quadratic equations in multiple variables or exploring higher-degree polynomials.
3. Use Technology: Leverage graphing calculators and mathematical software to visualize solutions and explore new methods.
4. Collaborate and Discuss: Engaging with peers or mentors can provide new insights and help solidify your knowledge.

Final Thoughts

The ability to manage and eliminate \(x^2\) from equations opens up a world of mathematical possibilities. Whether you are preparing for academic exams, tackling engineering problems, or simply enhancing your mathematical toolkit, these skills are invaluable. Continue to explore and practice, and you'll find that solving equations involving \(x^2\) becomes second nature.

With the comprehensive techniques covered in this guide, you are well-equipped to approach and solve a wide range of equations. Embrace the challenge, and remember that each equation you solve brings you closer to mastering the art of algebra.