Topic 9-x squared: Explore the fascinating world of the quadratic expression \(9 - x^2\). This comprehensive guide delves into its mathematical properties, graphing techniques, real-life applications, and more. Whether you're a student, educator, or math enthusiast, discover how \(9 - x^2\) plays a pivotal role in algebra and beyond. Let's unlock the secrets together!
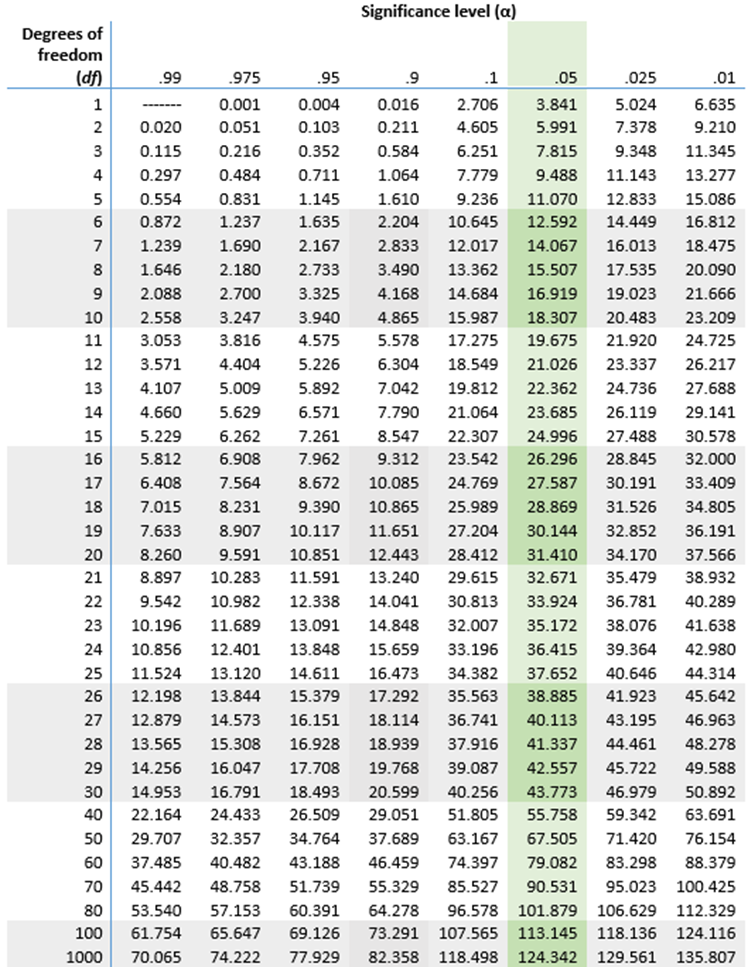
Table of Content
Understanding the Expression \(9 - x^2\)
The mathematical expression \(9 - x^2\) is commonly encountered in algebra, particularly when dealing with quadratic equations and the difference of squares. This expression can be simplified or factored in various ways, and it has significant applications in mathematics and physics.
Mathematical Interpretation
The expression \(9 - x^2\) can be interpreted and manipulated in several mathematical contexts:
- It is a quadratic expression that represents a parabola when plotted on a graph.
- It can be factored using the difference of squares formula: \(a^2 - b^2 = (a + b)(a - b)\).
- Here, it simplifies to: \(9 - x^2 = (3 + x)(3 - x)\).
Graphical Representation
When graphing \(y = 9 - x^2\), you get a parabola that opens downwards with its vertex at the point \((0, 9)\). The x-intercepts of the graph are at \(x = -3\) and \(x = 3\).
- Set \(y = 0\) to find the x-intercepts: \(0 = 9 - x^2\).
- Solving \(9 = x^2\), we get \(x = \pm3\).
Applications in Real Life
The expression \(9 - x^2\) can represent various physical phenomena:
- Physics: It can model the trajectory of an object under uniform acceleration when \(x\) represents time or horizontal distance.
- Engineering: Used in structural analysis and in describing certain types of curves and surfaces.
Example Problem
Let's solve the equation \(9 - x^2 = 0\).
- Rearrange to \(x^2 = 9\).
- Take the square root of both sides: \(x = \pm3\).
Visualizing \(9 - x^2\)
Below is a sample table of values for \(y = 9 - x^2\):
| x | y |
|---|---|
| -4 | -7 |
| -3 | 0 |
| 0 | 9 |
| 3 | 0 |
| 4 | -7 |
This table shows that the function has a maximum value at \(x = 0\) and decreases symmetrically as \(x\) moves away from zero.
Factoring \(9 - x^2\)
Using the difference of squares:
\[9 - x^2 = (3 + x)(3 - x)\]
Thus, the expression can be easily factored into two linear binomials.
READ MORE:
Introduction to 9 - x^2
The expression \(9 - x^2\) is a fundamental quadratic expression that appears frequently in algebra. It represents a classic example of a difference of squares, a concept used to simplify and solve various mathematical problems. Understanding this expression involves exploring its algebraic properties, graphical representation, and applications in different fields. Let's delve into each aspect to see why \(9 - x^2\) is so intriguing.
Algebraic Properties
The expression \(9 - x^2\) can be factored and simplified using the difference of squares formula. This formula states that:
\[a^2 - b^2 = (a + b)(a - b)\]
Applying this to \(9 - x^2\), we have:
\[9 - x^2 = (3 + x)(3 - x)\]
In this form, the expression is broken down into two linear factors, which can be useful for solving equations and simplifying expressions.
Graphical Representation
Graphing \(y = 9 - x^2\) results in a downward-opening parabola. The vertex of this parabola is at the point (0, 9), indicating the maximum value of the function. To understand how this graph behaves, consider the following table of values:
| x | y |
|---|---|
| -4 | -7 |
| -3 | 0 |
| 0 | 9 |
| 3 | 0 |
| 4 | -7 |
These values illustrate the parabolic shape, with the function reaching its highest point at \(y = 9\) and crossing the x-axis at \(x = -3\) and \(x = 3\).
Applications in Real Life
The quadratic expression \(9 - x^2\) finds applications in various real-world scenarios:
- Physics: It is used to model the trajectories of projectiles under the influence of gravity, where \(x\) can represent time or horizontal distance, and \(y\) can represent height.
- Engineering: Engineers use this expression to analyze structures and design curves that need to meet specific criteria, such as strength and stability.
- Economics: In economics, quadratic functions can model cost, revenue, and profit scenarios, helping businesses optimize their operations.
Step-by-Step Factorization
- Identify the coefficients in the expression \(a^2 - b^2\). Here, \(a = 3\) and \(b = x\).
- Apply the difference of squares formula: \[a^2 - b^2 = (a + b)(a - b)\].
- Substitute the values: \[9 - x^2 = (3 + x)(3 - x)\].
This factorized form is crucial for solving equations and finding the roots of the expression.
Conclusion
Understanding the expression \(9 - x^2\) opens doors to deeper insights into algebra and its applications. Whether you're graphing it, factoring it, or applying it in practical scenarios, this expression exemplifies the elegance and utility of quadratic equations in mathematics.
Mathematical Basics
Understanding the expression \(9 - x^2\) involves a foundation in quadratic expressions and their properties. Here, we explore the basic concepts related to this quadratic expression.
- Definition and Properties of Quadratic Expressions
- Difference of Squares Formula
- Factoring \(9 - x^2\)
- Roots of the Quadratic Expression
- \(3 + x = 0 \Rightarrow x = -3\)
- \(3 - x = 0 \Rightarrow x = 3\)
- Vertex and Axis of Symmetry
A quadratic expression is any polynomial of the form \(ax^2 + bx + c\), where \(a \neq 0\). In our case, \(9 - x^2\) is a quadratic expression in standard form where \(a = -1\), \(b = 0\), and \(c = 9\).
The difference of squares formula states that \(a^2 - b^2 = (a + b)(a - b)\). Applying this to \(9 - x^2\), we get:
\[9 - x^2 = 3^2 - x^2 = (3 + x)(3 - x)\]
This factorization is useful for simplifying and solving equations involving this expression.
Factoring \(9 - x^2\) involves recognizing it as a difference of squares:
\[9 - x^2 = (3 + x)(3 - x)\]
This factored form is essential for solving equations and understanding the properties of the expression.
The roots of the quadratic expression \(9 - x^2 = 0\) are found by setting each factor to zero:
Thus, the roots are \(x = -3\) and \(x = 3\).
The vertex form of a quadratic equation \(ax^2 + bx + c\) is useful for identifying the vertex and the axis of symmetry. For \(9 - x^2\), the vertex form is already given by:
\[y = 9 - x^2\]
The vertex of this parabola is at \((0, 9)\), and the axis of symmetry is \(x = 0\).
| Quadratic Expression | Standard Form | Factored Form | Vertex | Axis of Symmetry |
|---|---|---|---|---|
| \(9 - x^2\) | \(-x^2 + 0x + 9\) | \((3 + x)(3 - x)\) | (0, 9) | \(x = 0\) |
These fundamental concepts provide a solid understanding of the quadratic expression \(9 - x^2\) and its properties.
Graphical Analysis
Plotting \(9 - x^2\) on a Cartesian Plane
To plot the function \(9 - x^2\) on a Cartesian plane, we start by identifying key points and characteristics of the graph.
- The function \(9 - x^2\) is a downward-opening parabola.
- The vertex of the parabola is at \((0, 9)\), since the maximum value is achieved when \(x = 0\).
- The x-intercepts are found by setting the function equal to zero: \(9 - x^2 = 0 \Rightarrow x^2 = 9 \Rightarrow x = \pm 3\). Therefore, the x-intercepts are \((-3, 0)\) and \((3, 0)\).
Vertex and Axis of Symmetry
The vertex of the parabola \(y = 9 - x^2\) is at the point \((0, 9)\). This is the highest point on the graph, indicating that it opens downward.
The axis of symmetry is the vertical line that passes through the vertex. For the function \(y = 9 - x^2\), the axis of symmetry is the y-axis, or \(x = 0\).
Interpreting the Parabola's Shape and Direction
The general form of a quadratic function is \(y = ax^2 + bx + c\). For \(y = 9 - x^2\):
- The coefficient \(a\) is \(-1\), which is negative, indicating that the parabola opens downward.
- The value of \(c\) is \(9\), representing the y-intercept, where the graph crosses the y-axis.
The shape of the parabola is symmetrical with respect to the y-axis, and its width is determined by the coefficient of \(x^2\). In this case, the coefficient is \(-1\), giving it a standard width.
Graph Example
Below is a table of values to help visualize the graph of \(9 - x^2\):
| x | y |
|---|---|
| -3 | 0 |
| -2 | 5 |
| -1 | 8 |
| 0 | 9 |
| 1 | 8 |
| 2 | 5 |
| 3 | 0 |
Plot these points on the Cartesian plane and draw a smooth curve through them to complete the graph of \(y = 9 - x^2\).
Algebraic Manipulations
Algebraic manipulation of the expression \(9 - x^2\) involves several techniques, including solving quadratic equations, expanding and simplifying expressions, and completing the square. Below are detailed steps for these manipulations:
Solving Quadratic Equations Involving \(9 - x^2\)
To solve equations of the form \(9 - x^2 = 0\), follow these steps:
- Set the equation to zero: \(9 - x^2 = 0\).
- Rearrange the terms: \(9 = x^2\).
- Take the square root of both sides: \(x = \pm 3\).
This gives the solutions \(x = 3\) and \(x = -3\).
Expanding and Simplifying Expressions
Consider the expression \( (3 - x)(3 + x) \). This can be expanded using the distributive property:
\[
(3 - x)(3 + x) = 3 \cdot 3 + 3 \cdot x - x \cdot 3 - x \cdot x = 9 - x^2
\]Thus, \(9 - x^2\) is a difference of squares and simplifies to \((3 - x)(3 + x)\).
Completing the Square Method
To complete the square for the expression \(9 - x^2\), follow these steps:
- Rewrite the expression in the standard form of a quadratic: \( -x^2 + 9 \).
- Factor out the negative sign: \(-(x^2 - 9)\).
- Recognize that \(x^2 - 9\) is a difference of squares: \(-(x - 3)(x + 3)\).
Thus, \(9 - x^2\) can be expressed as \(-(x - 3)(x + 3)\).

Advanced Topics
The expression \( 9 - x^2 \) opens up several advanced mathematical concepts. Here, we delve into some of these topics in detail.
Integration and Differentiation of \( 9 - x^2 \)
- Differentiation:
To differentiate \( 9 - x^2 \), we use the power rule:
\[
\frac{d}{dx}(9 - x^2) = \frac{d}{dx}(9) - \frac{d}{dx}(x^2) = 0 - 2x = -2x
\] - Integration:
To integrate \( 9 - x^2 \), we use the power rule in reverse:
\[
\int (9 - x^2) \, dx = \int 9 \, dx - \int x^2 \, dx = 9x - \frac{x^3}{3} + C
\]
Complex Numbers and the Expression \( 9 - x^2 \)
- Roots in the Complex Plane:
For the equation \( 9 - x^2 = 0 \), solving for \( x \) gives \( x = \pm 3 \). However, for equations where the roots are not real, such as \( x^2 = 9 + 4 \), we get:
\[
x = \pm \sqrt{13}i
\]Here, \( i \) is the imaginary unit.
Solving Inequalities Involving \( 9 - x^2 \)
- Quadratic Inequality:
Consider the inequality \( 9 - x^2 \geq 0 \). We solve it by finding the roots \( x = \pm 3 \) and testing intervals:
\[
\begin{cases}
-3 \leq x \leq 3 & \text{(satisfies the inequality)} \\
\end{cases}
\] - Graphical Interpretation:
The inequality \( 9 - x^2 \geq 0 \) represents the region where the parabola \( y = 9 - x^2 \) lies above or on the x-axis. This region is bounded between the roots \( x = -3 \) and \( x = 3 \).
Implicit Differentiation Involving \( 9 - x^2 \)
- Concept:
Implicit differentiation is useful when dealing with equations where \( y \) is not isolated. For instance, if \( y^2 + x^2 = 9 \), differentiating both sides with respect to \( x \) gives:
\[
2y \frac{dy}{dx} + 2x = 0 \implies \frac{dy}{dx} = -\frac{x}{y}
\]
Practice Problems
Here are a variety of practice problems to help you master the concepts related to the expression \(9 - x^2\). These problems cover simplification, factorization, graphing, and real-world applications.
- Simplification and Factorization Exercises
- Simplify and factorize \(9 - x^2\).
- Simplify the following expressions by factoring:
- \(16 - y^2\)
- \(25 - z^2\)
- \(x^2 - 49\)
- Factor the expression \(9 - x^2\) completely.
- Verify your factorization by expanding the factors and comparing with the original expression.
- Graphing and Analyzing Quadratic Functions
- Plot the function \(y = 9 - x^2\) on a Cartesian plane.
- Identify the vertex and axis of symmetry.
- Determine the x-intercepts and y-intercept.
- Describe the shape and direction of the parabola.
- Compare the graph of \(y = 9 - x^2\) with the graph of \(y = -x^2\). What are the similarities and differences?
- Transform the graph of \(y = 9 - x^2\) by shifting it 3 units to the right and 2 units up. Write the equation of the new function and plot it.
- Plot the function \(y = 9 - x^2\) on a Cartesian plane.
- Real-World Application Scenarios
- Physics: A projectile is launched from a height of 9 meters with an initial horizontal velocity. The height \(h\) of the projectile as a function of horizontal distance \(x\) is given by \(h(x) = 9 - x^2\). Determine the maximum height and the range of the projectile.
- Engineering: A parabolic arch has a height of 9 meters at its center and is described by the equation \(y = 9 - x^2\). Calculate the width of the arch at ground level.
- Economics: The profit \(P\) from selling \(x\) units of a product is modeled by the equation \(P(x) = 9 - x^2\). Find the number of units that maximizes the profit and determine the maximum profit.
These problems are designed to reinforce your understanding and provide a comprehensive review of the concepts related to quadratic expressions and their applications. Practice regularly to enhance your skills and confidence in solving these types of problems.
Conclusion
In this guide, we have explored the quadratic expression \(9 - x^2\) from multiple perspectives, offering a comprehensive understanding of its properties, graphical behavior, algebraic manipulations, and real-world applications. Let's summarize the key concepts covered:
- Definition and Properties: The expression \(9 - x^2\) is a difference of squares, which can be factored as \((3 + x)(3 - x)\). This property is fundamental in simplifying and solving equations involving this expression.
- Graphical Analysis: The graph of \(y = 9 - x^2\) is a downward-facing parabola with its vertex at the point \((0, 9)\) and symmetry along the y-axis. This shape and direction are critical for understanding the behavior of the function across different values of \(x\).
- Algebraic Manipulations: We have delved into solving quadratic equations that include \(9 - x^2\), expanded and simplified related expressions, and used techniques such as completing the square to rewrite and solve these equations effectively.
- Real-World Applications: The concepts surrounding \(9 - x^2\) extend to various fields such as physics, where it models trajectories and parabolic motion; engineering, where it aids in structural analysis and design; and economics, where it helps in optimizing quadratic functions for maximum and minimum values.
- Advanced Topics: We explored the differentiation and integration of \(9 - x^2\), applications in complex numbers, and solving inequalities involving this expression, which provide a deeper mathematical context and tools for advanced studies.
- Practice Problems: A variety of exercises have been provided to reinforce the understanding and application of \(9 - x^2\), including simplification, factorization, graphing, and real-world problem scenarios.
For those interested in further exploration, additional resources and references are available to expand your knowledge and application skills regarding quadratic expressions and functions.
Understanding \(9 - x^2\) is more than just a mathematical exercise; it is a gateway to appreciating the beauty and utility of quadratic functions in both theoretical and practical contexts. We hope this guide has been both informative and inspiring.
For additional learning materials, please refer to the suggested resources and references below:





:max_bytes(150000):strip_icc()/Chi-SquareStatistic_Final_4199464-7eebcd71a4bf4d9ca1a88d278845e674.jpg)