Topic how to add 2 square roots: Learning how to add 2 square roots can simplify many mathematical problems and improve your calculation skills. In this article, we break down the process into easy-to-follow steps, ensuring you grasp the concept and apply it confidently. Master the technique of adding square roots with our comprehensive guide!
Table of Content
- How to Add Two Square Roots
- Introduction
- Understanding Square Roots
- Basic Principles of Adding Square Roots
- Step-by-Step Guide to Simplifying Square Roots
- Identifying Like Terms in Square Roots
- Combining Like Terms
- Examples of Adding Simplified Square Roots
- Special Cases in Adding Square Roots
- Common Mistakes to Avoid
- Practical Applications of Adding Square Roots
- Advanced Techniques for Complex Expressions
- YOUTUBE:
How to Add Two Square Roots
Adding square roots involves simplifying the square roots and combining like terms. Here's a detailed step-by-step guide:
Step 1: Simplify Each Square Root
Before adding the square roots, simplify each one as much as possible. For example:
- \(\sqrt{50} = \sqrt{25 \times 2} = 5\sqrt{2}\)
- \(\sqrt{18} = \sqrt{9 \times 2} = 3\sqrt{2}\)
Step 2: Check for Like Terms
Square roots can be added together only if they have the same radicand (the number inside the square root). In our example:
- Both \(\sqrt{50}\) and \(\sqrt{18}\) simplify to terms involving \(\sqrt{2}\).
Step 3: Combine Like Terms
Add the coefficients (numbers in front of the square roots) of the like terms:
- \(5\sqrt{2} + 3\sqrt{2} = (5 + 3)\sqrt{2} = 8\sqrt{2}\)
Example Problems
Let's look at a few example problems to solidify the concept:
-
\(\sqrt{12} + \sqrt{27}\)
- Simplify: \(\sqrt{12} = 2\sqrt{3}\) and \(\sqrt{27} = 3\sqrt{3}\)
- Combine like terms: \(2\sqrt{3} + 3\sqrt{3} = 5\sqrt{3}\)
-
\(\sqrt{45} + \sqrt{20}\)
- Simplify: \(\sqrt{45} = 3\sqrt{5}\) and \(\sqrt{20} = 2\sqrt{5}\)
- Combine like terms: \(3\sqrt{5} + 2\sqrt{5} = 5\sqrt{5}\)
Special Cases
If the square roots do not have the same radicand, they cannot be directly added. For instance:
- \(\sqrt{2} + \sqrt{3}\) cannot be simplified further and remains as is.
Conclusion
Adding square roots is straightforward when you simplify them first and then combine like terms. Remember, only square roots with the same radicand can be added together.

READ MORE:
Introduction
Adding square roots is a fundamental concept in mathematics that can help simplify complex equations and enhance your problem-solving abilities. In this section, we will introduce the basic principles of adding two square roots and provide a clear, step-by-step approach to mastering this technique. By understanding how to add square roots, you can tackle a variety of mathematical problems with confidence.
Square roots often appear in algebra, geometry, and various applications in science and engineering. Knowing how to add them correctly ensures accuracy in your calculations and helps you better understand the relationships between numbers. Let's dive into the basics of adding square roots:
- Identify the Square Roots: The first step is to identify the square roots you want to add. For example, let's consider \(\sqrt{8}\) and \(\sqrt{18}\).
- Simplify Each Square Root: Simplify each square root to its simplest form by factoring out the perfect squares. For instance, \(\sqrt{8} = \sqrt{4 \times 2} = 2\sqrt{2}\) and \(\sqrt{18} = \sqrt{9 \times 2} = 3\sqrt{2}\).
- Combine Like Terms: Once simplified, combine the square roots that have the same radicand. In our example, both terms have \(\sqrt{2}\), so we add the coefficients: \(2\sqrt{2} + 3\sqrt{2} = 5\sqrt{2}\).
- Verify the Result: Finally, verify the result to ensure accuracy. The sum of \(\sqrt{8}\) and \(\sqrt{18}\) is \(5\sqrt{2}\).
By following these steps, you can confidently add square roots and apply this knowledge to solve more complex mathematical problems. In the next sections, we will explore more detailed examples and special cases to further enhance your understanding.
Understanding Square Roots
Square roots are mathematical expressions that represent a value which, when multiplied by itself, gives the original number. The square root of a number \(x\) is written as \(\sqrt{x}\). For example, the square root of 16 is 4 because \(4 \times 4 = 16\). Understanding square roots is essential for various mathematical operations, including addition.
Here are some key concepts to understand square roots:
- Definition: The square root of a number \(x\) is a value \(y\) such that \(y^2 = x\). It is denoted as \(\sqrt{x}\).
- Perfect Squares: Numbers like 1, 4, 9, 16, and 25 are perfect squares because their square roots are integers. For instance, \(\sqrt{9} = 3\) and \(\sqrt{25} = 5\).
- Non-Perfect Squares: Numbers that are not perfect squares have square roots that are irrational numbers. For example, \(\sqrt{2}\) and \(\sqrt{3}\) are irrational and cannot be expressed as exact fractions.
- Simplifying Square Roots: Simplifying a square root involves factoring out the largest perfect square. For example, \(\sqrt{50} = \sqrt{25 \times 2} = 5\sqrt{2}\).
- Properties of Square Roots:
- \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\)
- \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\)
- \((\sqrt{a})^2 = a\)
Understanding these fundamental aspects of square roots is crucial for performing operations such as addition. In the following sections, we will delve into the specific steps and techniques for adding two square roots effectively.
Basic Principles of Adding Square Roots
Adding square roots requires an understanding of their properties and how they can be combined. Here are the basic principles to follow when adding square roots:
- Simplify the Square Roots: Before adding, simplify each square root as much as possible. For example, simplify \(\sqrt{12}\) to \(2\sqrt{3}\) and \(\sqrt{75}\) to \(5\sqrt{3}\).
- Identify Like Terms: Only square roots with the same radicand (the number inside the square root) can be added together. For instance, \(2\sqrt{3}\) and \(5\sqrt{3}\) can be added, but \(\sqrt{2}\) and \(\sqrt{3}\) cannot.
- Combine Like Terms: Add the coefficients (the numbers in front of the square roots) of like terms. For example:
- \(2\sqrt{3} + 5\sqrt{3} = (2 + 5)\sqrt{3} = 7\sqrt{3}\)
- Check for Further Simplification: After combining like terms, check if the resulting expression can be simplified further. In some cases, the combined term may have a factor that can be further simplified.
Let's look at a detailed example:
Example: Add \(\sqrt{18}\) and \(\sqrt{50}\)
- Simplify each square root:
- \(\sqrt{18} = \sqrt{9 \times 2} = 3\sqrt{2}\)
- \(\sqrt{50} = \sqrt{25 \times 2} = 5\sqrt{2}\)
- Identify like terms: Both terms have \(\sqrt{2}\) as the radicand.
- Combine like terms:
- \(3\sqrt{2} + 5\sqrt{2} = (3 + 5)\sqrt{2} = 8\sqrt{2}\)
By following these basic principles, you can accurately add square roots and simplify the expressions. Understanding these steps is crucial for solving more complex mathematical problems involving square roots.
Step-by-Step Guide to Simplifying Square Roots
Simplifying square roots is an essential skill for various mathematical operations, including addition. Here’s a detailed step-by-step guide to help you simplify square roots effectively:
- Identify the Radicand: The radicand is the number inside the square root symbol. For example, in \(\sqrt{50}\), the radicand is 50.
- Factor the Radicand: Break down the radicand into its prime factors or into factors that include perfect squares. For example:
- 50 can be factored as \(25 \times 2\)
- 72 can be factored as \(36 \times 2\) or \(9 \times 8\)
- Extract the Perfect Squares: Identify and extract the perfect square factors from the radicand. For example:
- \(\sqrt{50} = \sqrt{25 \times 2} = \sqrt{25} \times \sqrt{2} = 5\sqrt{2}\)
- \(\sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2}\)
- Simplify the Expression: Multiply the extracted square root of the perfect square by the remaining square root. For instance:
- \(\sqrt{50} = 5\sqrt{2}\)
- \(\sqrt{72} = 6\sqrt{2}\)
- Verify Your Simplification: Ensure that the remaining radicand is as simple as possible and cannot be factored further. For example, \(\sqrt{18}\) simplifies to \(3\sqrt{2}\), and no further simplification is needed.
Let’s look at a detailed example to illustrate these steps:
Example: Simplify \(\sqrt{200}\)
- Identify the radicand: The radicand is 200.
- Factor the radicand:
- 200 can be factored as \(100 \times 2\)
- Extract the perfect squares:
- \(\sqrt{200} = \sqrt{100 \times 2} = \sqrt{100} \times \sqrt{2} = 10\sqrt{2}\)
- Simplify the expression:
- \(\sqrt{200} = 10\sqrt{2}\)
- Verify your simplification:
- The simplified form \(10\sqrt{2}\) is correct and cannot be simplified further.
By following these steps, you can simplify any square root, making it easier to perform additional operations such as adding square roots. Practice with different numbers to become proficient in simplifying square roots.

Identifying Like Terms in Square Roots
When adding square roots, it is crucial to identify like terms. Like terms in square roots have the same radicand, which allows them to be combined. Here's a step-by-step guide to identifying like terms in square roots:
- Simplify Each Square Root: Begin by simplifying each square root to its simplest form. This helps in easily identifying like terms. For example:
- \(\sqrt{18} = 3\sqrt{2}\)
- \(\sqrt{50} = 5\sqrt{2}\)
- Compare the Radicands: Check the numbers inside the square roots (the radicands). If the radicands are the same, the square roots are like terms. For instance:
- In \(3\sqrt{2}\) and \(5\sqrt{2}\), the radicand is 2 in both terms.
- Combine the Coefficients: Once like terms are identified, add the coefficients (the numbers in front of the square roots) together. For example:
- \(3\sqrt{2} + 5\sqrt{2} = (3 + 5)\sqrt{2} = 8\sqrt{2}\)
- Handle Non-Like Terms Separately: Square roots with different radicands cannot be combined directly. They are considered non-like terms and should be kept separate. For example:
- \(\sqrt{3} + \sqrt{5}\) cannot be combined because the radicands (3 and 5) are different.
Let’s consider a detailed example to illustrate these steps:
Example: Identify and combine like terms in the expression \(\sqrt{8} + \sqrt{18} + \sqrt{32}\).
- Simplify each square root:
- \(\sqrt{8} = 2\sqrt{2}\)
- \(\sqrt{18} = 3\sqrt{2}\)
- \(\sqrt{32} = 4\sqrt{2}\)
- Compare the radicands:
- All terms have the radicand 2, so they are like terms.
- Combine the coefficients:
- \(2\sqrt{2} + 3\sqrt{2} + 4\sqrt{2} = (2 + 3 + 4)\sqrt{2} = 9\sqrt{2}\)
By following these steps, you can easily identify and combine like terms in square roots, simplifying your mathematical expressions effectively. Understanding how to identify like terms is crucial for performing accurate and efficient calculations involving square roots.
Combining Like Terms
Combining like terms in square roots is essential for simplifying expressions and performing accurate calculations. Here’s a detailed step-by-step guide to help you combine like terms effectively:
- Identify Like Terms: Like terms have the same radicand (the number inside the square root). For example, \(2\sqrt{3}\) and \(5\sqrt{3}\) are like terms because they both contain \(\sqrt{3}\).
- Simplify the Square Roots: Before combining, ensure each square root is simplified to its simplest form. For instance:
- \(\sqrt{50} = 5\sqrt{2}\)
- \(\sqrt{18} = 3\sqrt{2}\)
- Add the Coefficients: Once you have identified like terms and simplified the square roots, add the coefficients of the like terms. For example:
- \(5\sqrt{2} + 3\sqrt{2} = (5 + 3)\sqrt{2} = 8\sqrt{2}\)
- Combine Non-Like Terms Separately: Square roots with different radicands cannot be combined directly. They must be kept as separate terms in the expression. For example:
- \(\sqrt{3} + \sqrt{5}\) remains as it is because the radicands (3 and 5) are different.
Let’s look at a detailed example to illustrate these steps:
Example: Combine like terms in the expression \(2\sqrt{12} + \sqrt{75} + 3\sqrt{3}\).
- Simplify each square root:
- \(\sqrt{12} = \sqrt{4 \times 3} = 2\sqrt{3}\)
- \(\sqrt{75} = \sqrt{25 \times 3} = 5\sqrt{3}\)
- \(\sqrt{3}\) is already simplified.
- Identify like terms:
- All terms contain \(\sqrt{3}\), so they are like terms.
- Add the coefficients:
- \(2(2\sqrt{3}) + 5\sqrt{3} + 3\sqrt{3} = 4\sqrt{3} + 5\sqrt{3} + 3\sqrt{3} = (4 + 5 + 3)\sqrt{3} = 12\sqrt{3}\)
By following these steps, you can easily combine like terms in square roots, resulting in a simplified expression. Mastering this skill is crucial for solving various mathematical problems involving square roots efficiently and accurately.
Examples of Adding Simplified Square Roots
Adding simplified square roots can be straightforward once you understand the process of simplifying and combining like terms. Here are some detailed examples to illustrate this:
Example 1: Add \(\sqrt{8}\) and \(\sqrt{18}\)
- Simplify each square root:
- \(\sqrt{8} = \sqrt{4 \times 2} = 2\sqrt{2}\)
- \(\sqrt{18} = \sqrt{9 \times 2} = 3\sqrt{2}\)
- Identify like terms:
- Both terms have the same radicand (\(\sqrt{2}\)), so they are like terms.
- Add the coefficients:
- \(2\sqrt{2} + 3\sqrt{2} = (2 + 3)\sqrt{2} = 5\sqrt{2}\)
Example 2: Add \(\sqrt{50}\) and \(\sqrt{200}\)
- Simplify each square root:
- \(\sqrt{50} = \sqrt{25 \times 2} = 5\sqrt{2}\)
- \(\sqrt{200} = \sqrt{100 \times 2} = 10\sqrt{2}\)
- Identify like terms:
- Both terms have the same radicand (\(\sqrt{2}\)), so they are like terms.
- Add the coefficients:
- \(5\sqrt{2} + 10\sqrt{2} = (5 + 10)\sqrt{2} = 15\sqrt{2}\)
Example 3: Add \(\sqrt{72}\), \(\sqrt{18}\), and \(\sqrt{50}\)
- Simplify each square root:
- \(\sqrt{72} = \sqrt{36 \times 2} = 6\sqrt{2}\)
- \(\sqrt{18} = \sqrt{9 \times 2} = 3\sqrt{2}\)
- \(\sqrt{50} = \sqrt{25 \times 2} = 5\sqrt{2}\)
- Identify like terms:
- All terms have the same radicand (\(\sqrt{2}\)), so they are like terms.
- Add the coefficients:
- \(6\sqrt{2} + 3\sqrt{2} + 5\sqrt{2} = (6 + 3 + 5)\sqrt{2} = 14\sqrt{2}\)
Example 4: Add \(\sqrt{45}\) and \(\sqrt{20}\)
- Simplify each square root:
- \(\sqrt{45} = \sqrt{9 \times 5} = 3\sqrt{5}\)
- \(\sqrt{20} = \sqrt{4 \times 5} = 2\sqrt{5}\)
- Identify like terms:
- Both terms have the same radicand (\(\sqrt{5}\)), so they are like terms.
- Add the coefficients:
- \(3\sqrt{5} + 2\sqrt{5} = (3 + 2)\sqrt{5} = 5\sqrt{5}\)
These examples demonstrate the process of adding simplified square roots by identifying and combining like terms. By mastering this technique, you can handle more complex mathematical expressions involving square roots with ease.
Special Cases in Adding Square Roots
When adding square roots, certain special cases can make the process more complex. Below, we explore these cases and provide step-by-step guidance on how to handle them.
1. Adding Square Roots with Coefficients
When square roots have coefficients, you need to factor these into your calculations:
- Example: \(3\sqrt{2} + 5\sqrt{2}\)
- Combine the coefficients: \(3 + 5 = 8\)
- Result: \(8\sqrt{2}\)
2. Adding Square Roots with Different Radicands
If the square roots have different radicands, they cannot be directly added. Instead, you need to simplify them first:
- Example: \(\sqrt{18} + \sqrt{8}\)
- Simplify each square root:
- \(\sqrt{18} = \sqrt{9 \cdot 2} = 3\sqrt{2}\)
- \(\sqrt{8} = \sqrt{4 \cdot 2} = 2\sqrt{2}\)
- Now combine like terms: \(3\sqrt{2} + 2\sqrt{2} = 5\sqrt{2}\)
3. Rationalizing Square Roots
In some cases, you may need to rationalize the square roots before adding them:
- Example: \(\frac{1}{\sqrt{3}} + \frac{1}{\sqrt{12}}\)
- Rationalize each term:
- \(\frac{1}{\sqrt{3}} = \frac{\sqrt{3}}{3}\)
- \(\frac{1}{\sqrt{12}} = \frac{\sqrt{12}}{12} = \frac{2\sqrt{3}}{12} = \frac{\sqrt{3}}{6}\)
- Now combine like terms: \(\frac{\sqrt{3}}{3} + \frac{\sqrt{3}}{6} = \frac{2\sqrt{3} + \sqrt{3}}{6} = \frac{3\sqrt{3}}{6} = \frac{\sqrt{3}}{2}\)
4. Adding Square Roots of Perfect Squares
When adding square roots of perfect squares, simplify each term completely:
- Example: \(\sqrt{16} + \sqrt{25}\)
- Simplify each square root:
- \(\sqrt{16} = 4\)
- \(\sqrt{25} = 5\)
- Combine the results: \(4 + 5 = 9\)
5. Complex Numbers
When dealing with square roots of negative numbers, remember to use imaginary units:
- Example: \(\sqrt{-4} + \sqrt{-9}\)
- Express each term using \(i\):
- \(\sqrt{-4} = 2i\)
- \(\sqrt{-9} = 3i\)
- Combine the results: \(2i + 3i = 5i\)
By understanding these special cases, you can confidently handle any situation involving the addition of square roots.
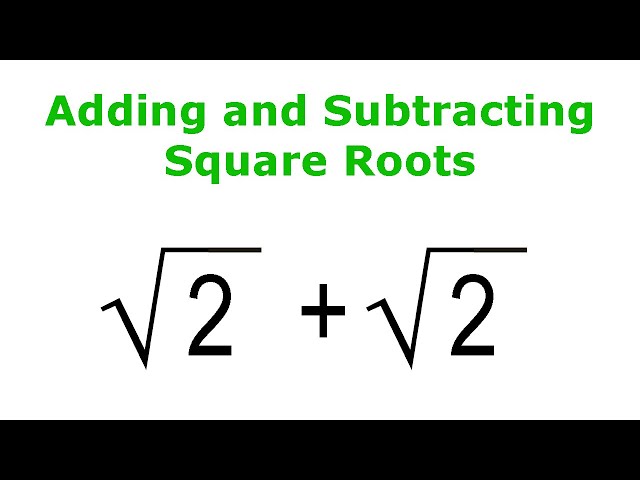
Common Mistakes to Avoid
When adding square roots, it's important to be mindful of common mistakes that can lead to incorrect results. Here are some frequent errors to watch out for and tips on how to avoid them:
- Adding Unlike Radicands Directly: One of the most common mistakes is trying to add square roots with different radicands. For example, \(\sqrt{2} + \sqrt{3}\) cannot be directly added. Ensure that the radicands are identical before combining them.
- Over-Simplification: Avoid simplifying square roots in a way that changes their values. For instance, improperly simplifying \(\sqrt{18}\) as \(\sqrt{9} \cdot \sqrt{2} = 3\sqrt{2}\) without considering that both parts must be factored correctly can lead to errors.
- Misapplying Algebraic Rules: It's crucial not to distribute the square root across addition inside the radicand. The square root of a sum, such as \(\sqrt{a + b}\), is not equal to the sum of the square roots, \(\sqrt{a} + \sqrt{b}\).
- Neglecting to Simplify First: Failing to simplify square roots before attempting to add them can result in missing opportunities for combining like terms. Always simplify each square root as much as possible to identify potential like terms.
- Incorrect Handling of Coefficients: Another common mistake is incorrectly combining coefficients. For example, when dealing with terms like \(3\sqrt{5} + 2\sqrt{5}\), ensure to add only the coefficients (3 + 2) while keeping the radicand the same, resulting in \(5\sqrt{5}\).
- Confusing Multiplication with Addition/Subtraction: Remember that while you can multiply square roots with different radicands, addition and subtraction require identical radicands. For example, \(\sqrt{2} \cdot \sqrt{3} = \sqrt{6}\), but you cannot add \(\sqrt{2} + \sqrt{3}\) directly.
By being aware of these common mistakes and practicing the correct methods, you can enhance your proficiency in adding square roots, leading to more accurate and efficient problem-solving in algebra.
Practical Applications of Adding Square Roots
Understanding how to add square roots is essential in various practical scenarios. Here are some detailed applications where adding square roots plays a crucial role:
1. Geometry and Architecture
In geometry, adding square roots is frequently used to calculate distances and areas. For instance, the Pythagorean theorem involves adding the squares of two sides to find the hypotenuse:
\[ c = \sqrt{a^2 + b^2} \]
In architecture, square roots help in determining the dimensions of structures. For example, when designing a diagonal brace for a rectangular frame, you can use the Pythagorean theorem to find the length of the brace by adding the squares of the lengths of the sides.
2. Physics and Engineering
In physics, the magnitude of a vector is found by adding the squares of its components and then taking the square root:
\[ |v| = \sqrt{v_x^2 + v_y^2 + v_z^2} \]
Engineering applications include calculating forces, where the resultant force from orthogonal components is determined using the same principle.
3. Finance and Economics
Square roots are used in financial formulas, such as calculating standard deviation to measure the volatility of stock returns:
\[ \sigma = \sqrt{\frac{1}{N}\sum_{i=1}^{N}(R_i - \mu)^2} \]
Here, \(\sigma\) is the standard deviation, \(R_i\) are the returns, \(\mu\) is the mean return, and \(N\) is the number of returns. Understanding these calculations helps in risk assessment and portfolio management.
4. Navigation
Pilots and navigators use square roots to calculate distances between two points on a map. For example, the distance formula in a two-dimensional plane is:
\[ d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2} \]
This formula helps in plotting the shortest path between two locations.
5. Statistics
In statistics, the variance and standard deviation of a data set are calculated using square roots. The standard deviation is the square root of the variance, providing insights into data dispersion:
\[ \sigma = \sqrt{\frac{1}{N}\sum_{i=1}^{N}(x_i - \bar{x})^2} \]
Where \(\sigma\) is the standard deviation, \(x_i\) are the data points, \(\bar{x}\) is the mean, and \(N\) is the number of data points.
6. Electrical Engineering
Square roots are fundamental in electrical engineering for calculating power in AC circuits. The root mean square (RMS) value of an AC current is found by taking the square root of the mean of the squares of the instantaneous values:
\[ I_{RMS} = \sqrt{\frac{1}{T}\int_0^T i^2(t) dt} \]
This calculation is crucial for designing and analyzing electrical systems.
7. Computer Graphics
In computer graphics, distances between points in 2D or 3D space are calculated using the distance formula, which involves adding square roots. This is essential for rendering and collision detection in games and simulations:
\[ d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2 + (z_2 - z_1)^2} \]
8. Accident Investigations
Police use square roots to determine the speed of vehicles before a collision based on skid marks. The formula used involves adding the square roots of distances:
\[ s = \sqrt{24d} \]
Where \(s\) is the speed in mph and \(d\) is the length of the skid marks in feet.
These examples illustrate how the concept of adding square roots extends beyond pure mathematics and is integral to various fields and everyday problem-solving.
Advanced Techniques for Complex Expressions
Adding complex square roots, especially those involving variables, requires a nuanced approach that goes beyond basic addition rules. Here are some advanced techniques to handle complex square root expressions effectively:
- Simplifying Radicals with Variables: When dealing with square roots involving variables, ensure each term is fully simplified. For instance, simplify expressions like \( \sqrt{50x^2} \) to \( 5x\sqrt{2} \) if possible. This helps in identifying like terms more easily.
- Rationalizing the Denominator: If a square root appears in the denominator, rationalize it by multiplying the numerator and the denominator by the conjugate of the denominator. For example:
\[
\frac{1}{\sqrt{2} + \sqrt{3}} \times \frac{\sqrt{2} - \sqrt{3}}{\sqrt{2} - \sqrt{3}} = \frac{\sqrt{2} - \sqrt{3}}{2 - 3} = \frac{\sqrt{2} - \sqrt{3}}{-1} = -\sqrt{2} + \sqrt{3}
\] - Using Algebraic Identities: Leverage algebraic identities to simplify expressions. For instance, use the difference of squares:
\[
(\sqrt{a} + \sqrt{b})(\sqrt{a} - \sqrt{b}) = a - b
\] - Combining Like Terms: Just like in basic addition of square roots, ensure to combine like terms. For instance:
\[
3\sqrt{5} + 4\sqrt{5} = (3 + 4)\sqrt{5} = 7\sqrt{5}
\] - Handling Nested Radicals: For expressions with nested radicals, simplify them step-by-step. For example:
\[
\sqrt{1 + 2\sqrt{3}} = \sqrt{(\sqrt{3} + 1)^2} = \sqrt{3} + 1
\] - Factoring Radicals: Sometimes, factoring the radicand can help in simplifying the expression. For example, \( \sqrt{72} = \sqrt{36 \times 2} = 6\sqrt{2} \).
- Using Conjugates for Complex Expressions: For complex expressions involving sums and differences of square roots, use conjugates to simplify. For example, to simplify \( \sqrt{a} + \sqrt{b} \) and \( \sqrt{a} - \sqrt{b} \):
\[
(\sqrt{a} + \sqrt{b})(\sqrt{a} - \sqrt{b}) = a - b
\]
By employing these advanced techniques, you can manage and simplify complex expressions involving square roots more effectively, enhancing your problem-solving skills in algebra and beyond.
Cộng Hai Căn Bậc Hai Bằng Cách Đơn Giản Hóa
READ MORE:
Cách Cộng và Trừ Căn Bậc Hai