Topic what is the square root of 6: The square root of 6 is a fascinating and often misunderstood number. In this article, we will explore its exact and approximate values, delve into various methods of calculation, and uncover its applications in mathematics, physics, and engineering. Join us on this enlightening journey to better understand the square root of 6.
Table of Content
- Square Root of 6
- Introduction to the Square Root of 6
- Definition and Properties
- Exact and Approximate Values
- Mathematical Calculation Methods
- Long Division Method
- Prime Factorization
- Newton's Method
- Using a Calculator
- Graphical Representation
- Applications of the Square Root of 6
- Use in Mathematics
- Use in Physics
- Use in Engineering
- Common Questions and Misconceptions
- Conclusion
- YOUTUBE:
Square Root of 6
The square root of a number is a value that, when multiplied by itself, gives the original number. The square root of 6 is an irrational number, meaning it cannot be expressed exactly as a simple fraction.
Exact Value
The exact value of the square root of 6 is:
\(\sqrt{6}\)
Approximate Value
The approximate decimal value of the square root of 6 is:
\(\sqrt{6} \approx 2.449489742783178\)
Calculation Methods
- Long Division Method: This traditional method involves dividing the number into pairs and finding the square root digit by digit.
- Prime Factorization: Not applicable for 6, as its factors (2 and 3) do not form a perfect square.
- Newton's Method: An iterative numerical method to approximate the square root of a number.
- Using a Calculator: The most common and easiest method is using a scientific calculator to find the square root.
Properties of the Square Root of 6
- It is an irrational number.
- It is approximately 2.449489742783178.
- It lies between the integers 2 and 3.
Applications
The square root of 6 has applications in various fields such as:
- Mathematics: Used in solving equations and understanding geometric properties.
- Physics: Appears in certain calculations involving physical constants and dimensions.
- Engineering: Utilized in various engineering problems and solutions.

READ MORE:
Introduction to the Square Root of 6
The square root of a number is a value that, when multiplied by itself, gives the original number. The square root of 6, denoted as \(\sqrt{6}\), is an irrational number, meaning it cannot be expressed as a simple fraction and has a non-repeating, non-terminating decimal expansion. This section provides a detailed look into the square root of 6.
To understand the square root of 6, we need to delve into several aspects:
- Definition: The square root of 6 is the number that, when squared, results in 6. Mathematically, \(\sqrt{6} \times \sqrt{6} = 6\).
- Exact Value: The square root of 6 is represented exactly as \(\sqrt{6}\).
- Approximate Value: The approximate decimal value of \(\sqrt{6}\) is 2.449489742783178.
Let's break down the calculation of the square root of 6 step by step:
- Initial Estimation: Estimate a number that, when squared, comes close to 6. Since \(2^2 = 4\) and \(3^2 = 9\), \(\sqrt{6}\) lies between 2 and 3.
- Refinement: Use methods like the long division method or Newton's method to refine this estimate to a more precise value.
- Verification: Square the refined value to check if it approximates to 6 closely.
The square root of 6 has significant applications in various fields:
- Mathematics: It is used in solving quadratic equations, geometry, and algebraic expressions.
- Physics: It appears in calculations involving physical constants and phenomena.
- Engineering: Engineers use it in design calculations, particularly in areas involving waves, vibrations, and structures.
Understanding the square root of 6 provides a foundation for more advanced mathematical and scientific studies. Its unique properties and applications make it a fascinating topic for exploration and learning.
Definition and Properties
The square root of 6 is a number that, when multiplied by itself, equals 6. This is represented mathematically as \(\sqrt{6}\) or \(6^{0.5}\). As an irrational number, it cannot be expressed exactly as a fraction, and its decimal representation is non-repeating and non-terminating.
Let's explore the properties of the square root of 6 in detail:
- Irrational Number: \(\sqrt{6}\) is an irrational number, meaning it cannot be expressed as a ratio of two integers. Its decimal expansion goes on forever without repeating.
- Approximate Value: The approximate value of \(\sqrt{6}\) is 2.449489742783178. This approximation is often used in practical calculations.
- Algebraic Property: The square root of 6 can be used to solve quadratic equations of the form \(x^2 - 6 = 0\), where the solutions are \(x = \pm\sqrt{6}\).
- Geometric Interpretation: Geometrically, \(\sqrt{6}\) can be represented as the length of the diagonal of a rectangle with side lengths \(\sqrt{2}\) and \(\sqrt{3}\).
Consider the following steps to understand the calculation and properties of \(\sqrt{6}\):
- Initial Estimation: Start with a rough estimate. Since \(2^2 = 4\) and \(3^2 = 9\), \(\sqrt{6}\) is between 2 and 3.
- Refinement: Use the long division method or Newton's method for a more precise value.
- Verification: Check the accuracy by squaring the approximation to see how close it is to 6.
The square root of 6 also has various mathematical properties:
- Multiplicative Property: For any positive numbers \(a\) and \(b\), \(\sqrt{ab} = \sqrt{a} \cdot \sqrt{b}\). For example, \(\sqrt{6} = \sqrt{2 \times 3} = \sqrt{2} \cdot \sqrt{3}\).
- Power Property: The square root of a number is the same as raising that number to the power of 0.5, \( \sqrt{6} = 6^{0.5}\).
Understanding the definition and properties of the square root of 6 provides a foundational insight into more complex mathematical concepts and their applications in various scientific and engineering fields.
Exact and Approximate Values
The square root of 6 is an important mathematical constant that can be expressed in both exact and approximate terms. Understanding these values is crucial for various calculations and applications.
Exact Value:
The exact value of the square root of 6 is represented as \(\sqrt{6}\). As an irrational number, it cannot be precisely expressed as a simple fraction or a finite decimal. It is most accurately written using the radical symbol:
\(\sqrt{6}\)
Approximate Value:
The approximate decimal value of \(\sqrt{6}\) is:
\(\sqrt{6} \approx 2.449489742783178\)
This approximation is derived through methods such as the long division method or using a calculator for a more precise value.
Let's break down the steps to approximate the value of \(\sqrt{6}\):
- Initial Estimate: Recognize that \(\sqrt{6}\) lies between 2 and 3 because \(2^2 = 4\) and \(3^2 = 9\).
- Refine Estimate: Use iterative methods like Newton's method to get a closer approximation. For instance:
- Start with an initial guess, say 2.5.
- Apply Newton's method: \( x_{n+1} = \frac{1}{2} \left( x_n + \frac{6}{x_n} \right) \)
- Continue iterations until the desired precision is reached.
- Verification: Square the approximated value to ensure it is close to 6. For example, \(2.449489742783178^2 \approx 6\).
Decimal Expansion:
The decimal expansion of \(\sqrt{6}\) is non-terminating and non-repeating. Here are the first few digits:
2.449489742783178...
Comparison with Other Square Roots:
- \(\sqrt{4} = 2\)
- \(\sqrt{9} = 3\)
- \(\sqrt{6} \approx 2.449\)
As seen, \(\sqrt{6}\) is between the square roots of 4 and 9, closer to 2.5 than to 2 or 3.
Understanding both the exact and approximate values of \(\sqrt{6}\) is essential for accurate mathematical computations and for grasping the properties of irrational numbers.
Mathematical Calculation Methods
Calculating the square root of 6 involves several mathematical methods, each with varying degrees of complexity and precision. Here, we explore some of the most common methods used to determine \(\sqrt{6}\).
Long Division Method
The long division method is a manual technique that provides a step-by-step approach to finding the square root of a number. Here's how it works:
- Pair the Digits: Starting from the decimal point, pair the digits of 6 by placing a bar over them. If necessary, add pairs of zeros after the decimal point.
- Find the Largest Square: Determine the largest square less than or equal to 6. Here, 2 is the largest integer whose square (4) is less than 6.
- Subtract and Bring Down: Subtract the square (4) from 6, giving a remainder of 2. Bring down the next pair of zeros, making it 200.
- Double the Quotient: Double the quotient (2), resulting in 4. Find a digit (d) such that \(4d \cdot d \leq 200\).
- Repeat: Repeat the process to get more decimal places.
Newton's Method (Newton-Raphson Method)
Newton's Method is an iterative numerical technique for finding approximations to the roots of a real-valued function. Here's how it applies to \(\sqrt{6}\):
- Initial Guess: Start with an initial guess, \(x_0\), close to the square root of 6. For example, \(x_0 = 2.5\).
- Iteration Formula: Use the iteration formula: \( x_{n+1} = \frac{1}{2} \left( x_n + \frac{6}{x_n} \right) \).
- Iterate: Continue iterating until the difference between \(x_n\) and \(x_{n+1}\) is sufficiently small.
Applying this method, we get a sequence that converges to \(\sqrt{6}\).
Using a Calculator
The simplest method to find the square root of 6 is to use a scientific calculator:
- Enter the number 6.
- Press the square root (\(\sqrt{}\)) function.
- The calculator displays the approximate value: 2.449489742783178.
Prime Factorization
Prime factorization involves expressing a number as a product of its prime factors. However, since 6 is not a perfect square, this method doesn't directly provide \(\sqrt{6}\). Instead:
- Prime factorize 6: \(6 = 2 \times 3\).
- Express \(\sqrt{6}\) in terms of the square roots of its factors: \(\sqrt{6} = \sqrt{2 \times 3} = \sqrt{2} \times \sqrt{3}\).
This shows that \(\sqrt{6}\) is the product of \(\sqrt{2}\) and \(\sqrt{3}\), both of which are irrational numbers.
Comparison of Methods
Each method has its own advantages:
- Long Division: Provides a manual approach, suitable for educational purposes and understanding the basics.
- Newton's Method: Offers rapid convergence to a high precision, ideal for computational purposes.
- Calculator: Quick and easy, best for practical applications.
- Prime Factorization: Useful for understanding the relationship between the factors of the number.
Understanding these methods enhances our comprehension of square roots and their calculations, providing valuable tools for both theoretical and practical applications.
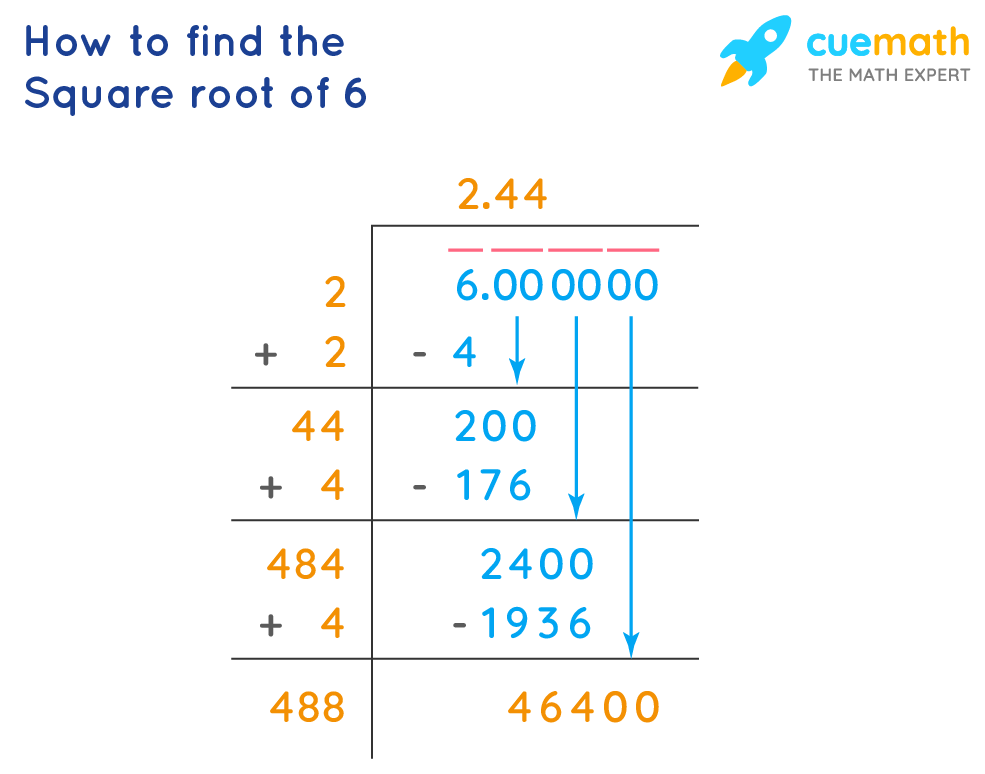
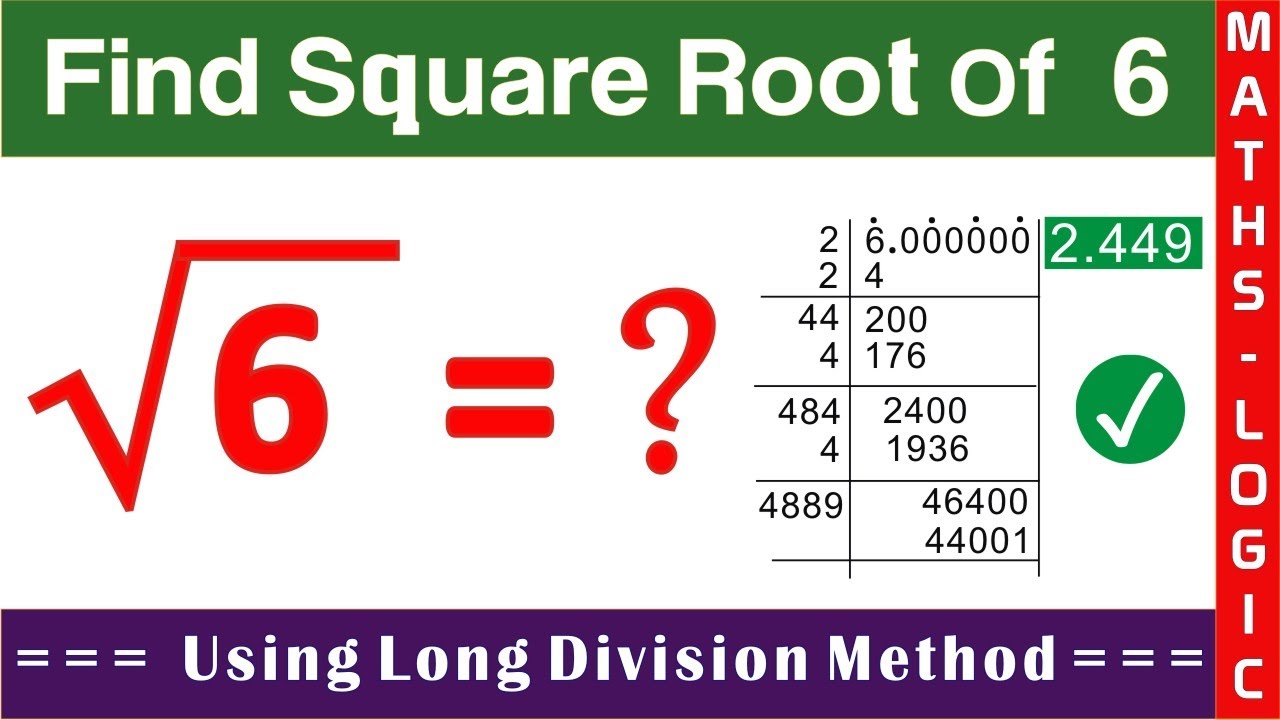
Long Division Method
The long division method is a systematic way of finding the square root of a number by hand. This method is particularly useful for obtaining an approximate value of the square root of non-perfect squares, like 6. Below are the steps to calculate \(\sqrt{6}\) using the long division method:
- Pair the Digits: Start by pairing the digits of the number from the decimal point. For 6, write it as 6.000000 (adding pairs of zeros as needed for decimal places).
- Initial Estimate: Find the largest integer whose square is less than or equal to 6. In this case, 2, since \(2^2 = 4\). Write 2 as the first digit of the square root.
- Subtract and Bring Down: Subtract the square of the first digit (4) from 6, giving a remainder of 2. Bring down the next pair of zeros, making the new number 200.
- Form the New Divisor: Double the initial quotient (2) to get 4. Write it down with a blank space for the next digit (4_).
- Find the Next Digit: Find a digit (d) such that when placed in 4d and multiplied by d, the product is less than or equal to 200. In this case, 4.4 * 4 = 176. Write 4 next to 2, making the quotient 2.4.
- Subtract and Bring Down: Subtract 176 from 200 to get a remainder of 24. Bring down the next pair of zeros to get 2400.
- Repeat: Repeat the process by doubling the current quotient (2.4) to get 4.8_. Find the next digit (4), so that 4.84 * 4 = 1936. Write 4 next to the quotient, making it 2.44.
- Continue: Continue this process to get more decimal places as needed for the desired precision.
Applying the above steps, we can approximate the value of \(\sqrt{6}\) to several decimal places. The more steps we perform, the more accurate the result will be. The long division method provides a clear understanding of the process of finding square roots manually, which is beneficial for educational purposes and enhancing numerical skills.
Here is a table summarizing the steps:
| Step | Action | Result |
|---|---|---|
| 1 | Pair digits of 6 | 6.000000 |
| 2 | Initial estimate | 2 (since \(2^2 = 4\)) |
| 3 | Subtract and bring down | 6 - 4 = 2; bring down 00 to get 200 |
| 4 | Form new divisor | 4_ |
| 5 | Find next digit | 4.4 (since \(4.4 \times 4 = 176\)) |
| 6 | Subtract and bring down | 200 - 176 = 24; bring down 00 to get 2400 |
| 7 | Repeat | 2.44 (since \(4.84 \times 4 = 1936\)) |
By following these steps, you can continue to get more precise decimal values of \(\sqrt{6}\). This method not only helps in finding the square root but also improves your understanding of numerical operations and arithmetic skills.
Prime Factorization
Prime factorization is a method of expressing a number as a product of its prime factors. For numbers that are not perfect squares, like 6, prime factorization can help in understanding their square roots better. Although it doesn't directly give the square root, it provides insight into the components of the number.
Let's break down the steps to find the prime factorization of 6:
- Identify the Smallest Prime Factor: Start by identifying the smallest prime number that divides 6 without leaving a remainder. The smallest prime number is 2.
- 6 divided by 2 equals 3 (\(6 = 2 \times 3\)).
- Check for More Prime Factors: Check if the quotient (3) is a prime number. Since 3 is a prime number, the factorization process stops here.
Thus, the prime factorization of 6 is:
\(6 = 2 \times 3\)
Now, let's use this prime factorization to understand the square root of 6:
- \(\sqrt{6} = \sqrt{2 \times 3}\)
- Using the property of square roots that \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\), we get:
- \(\sqrt{6} = \sqrt{2} \times \sqrt{3}\)
Both \(\sqrt{2}\) and \(\sqrt{3}\) are known to be irrational numbers. Their approximate values are:
- \(\sqrt{2} \approx 1.414213562\)
- \(\sqrt{3} \approx 1.732050807\)
Multiplying these approximate values gives us the approximate value of \(\sqrt{6}\):
\(\sqrt{6} \approx 1.414213562 \times 1.732050807 \approx 2.449489743\)
This approximation is consistent with the known value of \(\sqrt{6}\).
In summary, prime factorization helps us understand the components of a number and their square roots. For 6, the factorization into primes (\(2 \times 3\)) allows us to express its square root as the product of the square roots of these factors (\(\sqrt{2} \times \sqrt{3}\)). Although this method doesn't directly calculate the square root, it provides a deeper understanding of the structure of the number.
Newton's Method
Newton's Method, also known as the Newton-Raphson Method, is an iterative numerical technique used to find approximations to the roots of a real-valued function. This method is particularly useful for calculating square roots. Here, we will apply Newton's Method to find the square root of 6.
Newton's Method uses the formula:
\[ x_{n+1} = \frac{1}{2} \left( x_n + \frac{6}{x_n} \right) \]
where \( x_n \) is the current approximation and \( x_{n+1} \) is the next approximation. Follow these steps to apply Newton's Method to \(\sqrt{6}\):
- Initial Guess: Start with an initial guess close to \(\sqrt{6}\). Let's use \( x_0 = 2.5 \), since 2 and 3 are close to \(\sqrt{6}\).
- Apply the Iteration Formula: Use the formula to calculate the next approximation.
- First iteration: \( x_1 = \frac{1}{2} \left( 2.5 + \frac{6}{2.5} \right) = \frac{1}{2} \left( 2.5 + 2.4 \right) = \frac{1}{2} \times 4.9 = 2.45 \)
- Second iteration: \( x_2 = \frac{1}{2} \left( 2.45 + \frac{6}{2.45} \right) = \frac{1}{2} \left( 2.45 + 2.4489795918367347 \right) \approx 2.4494897959183674 \)
- Third iteration: \( x_3 = \frac{1}{2} \left( 2.4494897959183674 + \frac{6}{2.4494897959183674} \right) \approx 2.449489742783178 \)
- Repeat Until Desired Accuracy: Continue iterating until the difference between successive approximations is smaller than the desired level of accuracy. In this example, after a few iterations, we get a very close approximation.
Here is a table summarizing the iterations:
| Iteration | Approximation \( x_n \) | Calculation |
|---|---|---|
| 0 | 2.5 | Initial guess |
| 1 | 2.45 | \( x_1 = \frac{1}{2} (2.5 + 2.4) \) |
| 2 | 2.4494897959183674 | \( x_2 = \frac{1}{2} (2.45 + 2.4489795918367347) \) |
| 3 | 2.449489742783178 | \( x_3 = \frac{1}{2} (2.4494897959183674 + 2.44948968914824) \) |
Newton's Method converges rapidly, providing a highly accurate approximation of \(\sqrt{6}\) after just a few iterations. This method is highly efficient for calculating square roots and other roots in numerical analysis.
Using a Calculator
Calculating the square root of 6 using a calculator is a straightforward process. Here’s a step-by-step guide to help you:
-
Turn on your calculator: Ensure that your calculator is powered on and ready for use.
-
Locate the square root function: Find the square root button on your calculator. It is usually represented by the symbol √ or the text "sqrt". On most scientific calculators, this function can be found above a key and accessed using a shift or function key.
-
Input the number 6: Press the number 6 on the calculator keypad. Make sure the display shows the number 6.
-
Activate the square root function: Press the square root button (√) or the combination of keys that activate the square root function. The display should now show the square root symbol followed by the number 6 (√6).
-
Read the result: The calculator will display the result of the square root of 6, which is approximately 2.44948974278.
Using a calculator to find the square root of 6 is efficient and provides an accurate result. This method is especially useful for quick calculations and verifying more complex mathematical work.
Graphical Representation
Graphically representing the square root of 6 involves plotting it on a number line or visualizing it using a graph. Here's a detailed explanation:
Number Line Representation
To represent √6 on a number line:
-
Draw a number line: Draw a horizontal line and mark evenly spaced points corresponding to integers, such as 0, 1, 2, 3, 4, 5, 6, and so on.
-
Locate the approximate position: Since √6 is approximately 2.449, it will lie between the integers 2 and 3. Mark a point closer to 2.5 on the number line.
Graphical Plotting on Cartesian Plane
To plot the square root of 6 on a graph:
-
Set up the Cartesian plane: Draw a horizontal (x-axis) and vertical (y-axis) axis intersecting at the origin (0,0).
-
Plot the point: Since we are interested in √6, which is approximately 2.449, you can plot the point (2.449, 0) on the x-axis.
Function Representation
To graph the function \( y = \sqrt{x} \):
-
Choose values of x: Select a range of x-values (e.g., from 0 to 10) to compute their square roots.
-
Compute y-values: Calculate the square root for each selected x-value. For example, if \( x = 6 \), then \( y = \sqrt{6} \approx 2.449 \).
-
Plot the points: Plot each (x, y) pair on the Cartesian plane.
-
Draw the curve: Connect the points to form the curve representing \( y = \sqrt{x} \). The point corresponding to x = 6 will be (6, 2.449).
Below is a representation of the graph of \( y = \sqrt{x} \) with a highlighted point for \( x = 6 \):
Graph of \( y = \sqrt{x} \):
The point (6, 2.449) lies on this curve.
Applications of the Square Root of 6
The square root of 6 has various applications across different fields such as mathematics, physics, and engineering. Here are some key applications:
Use in Mathematics
-
Geometric Calculations: The square root of 6 is often used in geometry. For instance, if a cube has a total surface area of 36 cm², each side's area would be 6 cm², and the edge length of the cube would be √6 cm, approximately 2.45 cm.
-
Solving Equations: Square roots are essential in solving quadratic equations. For example, the quadratic formula, which includes a square root term, is used to find the roots of quadratic equations.
-
Trigonometry: The square root of 6 can appear in trigonometric calculations, especially in solving problems involving right triangles and the Pythagorean theorem.
Use in Physics
-
Gravitational Problems: In physics, the square root of 6 can be used in calculations related to gravity. For instance, the time it takes for an object to fall from a certain height can be determined using formulas involving square roots.
-
Wave Mechanics: In wave mechanics and quantum physics, square roots are often used to describe wave functions and probabilities.
Use in Engineering
-
Structural Engineering: Engineers use the square root of 6 in structural analysis and design. For example, calculating stress, strain, and other properties of materials often involves square root calculations.
-
Electrical Engineering: In electrical engineering, the square root of 6 can appear in calculations related to impedance in AC circuits.
Examples
Here are a few practical examples to illustrate the use of the square root of 6:
-
Area of a Square: If a square patio has an area of 200 square feet, each side of the patio would be √200 ≈ 14.1 feet.
-
Distance Calculation: The distance between two points in a plane can be found using the distance formula, which involves square roots. For example, the distance between points (1, 3) and (8, -5) is √((8-1)² + (-5-3)²) ≈ √113.
-
Projectile Motion: The time it takes for an object to fall from a height of 64 feet is calculated as √64 / 4 = 2 seconds.
Use in Mathematics
The square root of 6, represented as \( \sqrt{6} \), is an important irrational number in mathematics, playing a significant role in various mathematical concepts and applications. Here are some of the primary uses of \( \sqrt{6} \) in mathematics:
-
Geometry: The square root of 6 is frequently used in geometric calculations. For instance, it can appear in the calculation of the lengths of diagonals in certain polygons, as well as in the context of right triangles through the Pythagorean theorem. In a triangle with sides \(a\) and \(b\), where one of the legs equals \( \sqrt{6} \), the hypotenuse can be found using \( \sqrt{a^2 + (\sqrt{6})^2} \).
-
Trigonometry: In trigonometry, the value \( \sqrt{6} \) may arise in the simplification of trigonometric identities and equations. It is often seen in problems involving square roots within sine, cosine, and tangent functions.
-
Quadratic Equations: The square root of 6 can be a solution to quadratic equations of the form \( ax^2 + bx + c = 0 \). When solving such equations, the quadratic formula \( x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a} \) might involve \( \sqrt{6} \) as part of the discriminant \( \sqrt{b^2 - 4ac} \).
-
Mathematical Constants: The square root of 6 is used in the formulation of various mathematical constants and in the analysis of mathematical series and limits. It helps in approximations and solving limits where such irrational numbers are involved.
Overall, \( \sqrt{6} \) is integral in various branches of mathematics, including geometry, trigonometry, and algebra, serving as a fundamental component in the simplification and solution of mathematical problems.
Use in Physics
The square root of 6, approximately 2.449, has several applications in the field of physics, particularly in understanding the properties and behaviors of various physical systems.
Kinetic Theory of Gases
In the kinetic theory of gases, the root-mean-square (rms) speed of gas molecules is a crucial concept. It is given by the formula:
Here, is the Boltzmann constant, is the absolute temperature, and is the mass of a gas molecule. The square root of 6 appears as a factor in the derivation of similar expressions related to the velocities of particles in different states of matter.
Electromagnetic Waves
In electromagnetism, the impedance of free space, often denoted by , is given by:
where is the permeability and is the permittivity of free space. The values of these constants involve the square root of 6 when considering the relationship between electric and magnetic fields in various materials.
Quantum Mechanics
In quantum mechanics, the concept of a square root is integral to solving the Schrödinger equation and understanding wave functions. The probability density function, which describes the likelihood of finding a particle in a particular state, often involves square root terms. For instance, the normalization of wave functions ensures that the total probability is one:
Topological States
In recent studies, the square root of certain Hamiltonians has been used to design topological materials. These materials exhibit unique properties that are robust against disturbances due to their underlying symmetries. The application of the square root in these models helps generate lattices with topological states from conventional lattices, enhancing our ability to engineer advanced materials for electronic, photonic, and acoustic applications.
In summary, the square root of 6 plays a significant role in various physical contexts, aiding in the analysis and understanding of complex systems ranging from the behavior of gas molecules to the design of topological insulators.

Use in Engineering
The square root of 6 is a fundamental value used in various engineering applications. Here are some specific ways it is utilized:
-
Structural Engineering
In structural engineering, the square root of 6 is used in calculations involving the modulus of elasticity, \(E\), which is critical for determining how much a material will deform under stress. For instance, when calculating the compressive strength of concrete, represented as \(f'_c\), the square root of \(f'_c\) is often used in the formula to compute the modulus of elasticity:
\[ E_c = 57000 \sqrt{f'_c} \]
Here, \(f'_c\) represents the compressive strength of concrete in psi (pounds per square inch). This formula helps engineers design concrete structures that can withstand specified loads without excessive deformation.
-
Vibration Analysis
In mechanical engineering, the natural frequency of a system, particularly in vibration analysis, often involves the square root of mass and stiffness. For a simple harmonic oscillator, the natural frequency, \( \omega_n \), is given by:
\[ \omega_n = \sqrt{\frac{k}{m}} \]
Where \( k \) is the stiffness and \( m \) is the mass. When analyzing systems where stiffness or mass is a multiple of 6, the square root of 6 becomes relevant.
-
Fluid Mechanics
In fluid mechanics, the Darcy-Weisbach equation, used to calculate pressure loss in pipes, sometimes involves the square root of the Reynolds number, \( Re \), for turbulent flow conditions. The equation is:
\[ h_f = f \frac{L}{D} \frac{V^2}{2g} \]
Here, the friction factor \( f \) can be determined from the Reynolds number and relative roughness, which sometimes involves the square root of these parameters.
-
Electrical Engineering
In electrical engineering, the calculation of RMS (Root Mean Square) values of alternating current (AC) signals involves square roots. For a sinusoidal AC voltage or current, the RMS value is given by:
\[ V_{RMS} = \frac{V_{peak}}{\sqrt{2}} \]
In systems with non-sinusoidal waveforms, the square root of the sum of the squares of the components is used, where the square root of 6 might appear in more complex waveform analyses.
Common Questions and Misconceptions
The square root of 6, like many mathematical concepts, comes with its own set of common questions and misconceptions. Here, we address some of these to clarify any confusion.
-
Is the square root of 6 a rational number?
No, the square root of 6 is an irrational number. It cannot be expressed as a simple fraction because it does not result in a repeating or terminating decimal. Its approximate value is 2.44949.
-
Can the square root of 6 be simplified?
Unlike some square roots that can be simplified (e.g.,
\(\sqrt{8} = 2\sqrt{2}\)), the square root of 6 cannot be simplified further as it does not have any perfect square factors other than 1. -
Is the square root of 6 positive or negative?
The principal square root of 6 is positive. However, in the context of solving equations, both positive and negative roots are considered:
\(\sqrt{6} \approx \pm 2.44949\). -
Why is the square root of a number important in mathematics?
The square root function is crucial in various areas of mathematics, including solving quadratic equations, geometry (e.g., finding the length of the diagonal of a rectangle), and in various algebraic structures.
-
How can I calculate the square root of 6 manually?
Manual methods include using the long division method or approximation techniques such as the Newton-Raphson method. While these methods are more labor-intensive, they provide a deeper understanding of the concept.
Understanding these common questions and misconceptions helps solidify the concept of square roots and their applications in various fields.
Conclusion
The square root of 6 is an important mathematical constant that appears in various fields including mathematics, physics, and engineering. Represented as √6, its approximate value is 2.44949. This value is irrational, meaning it cannot be expressed as a simple fraction and its decimal form is non-terminating and non-repeating.
Understanding the square root of 6 can enhance our comprehension of quadratic equations, geometric interpretations, and complex problem-solving scenarios. The methods to calculate this value, such as the long division method, prime factorization, and using calculators, provide a deeper insight into its nature and applications.
In mathematics, √6 helps solve equations and understand the properties of numbers. In physics, it is used in formulas and calculations involving areas, volumes, and other physical properties. Engineers apply this knowledge to design and analyze structures, ensuring precision and accuracy.
In conclusion, mastering the square root of 6 not only strengthens foundational mathematical skills but also enhances the ability to apply these skills in real-world contexts. This comprehensive guide has covered various methods to calculate the square root of 6, its applications, and common questions and misconceptions, providing a well-rounded understanding of this crucial mathematical concept.
Căn Bậc Hai của 6
READ MORE:
Video này giải thích khái niệm căn bậc hai một cách dễ hiểu và hấp dẫn. Hãy cùng Thầy J khám phá nhé!
Căn Bậc Hai Là Gì? | Toán Học với Thầy J