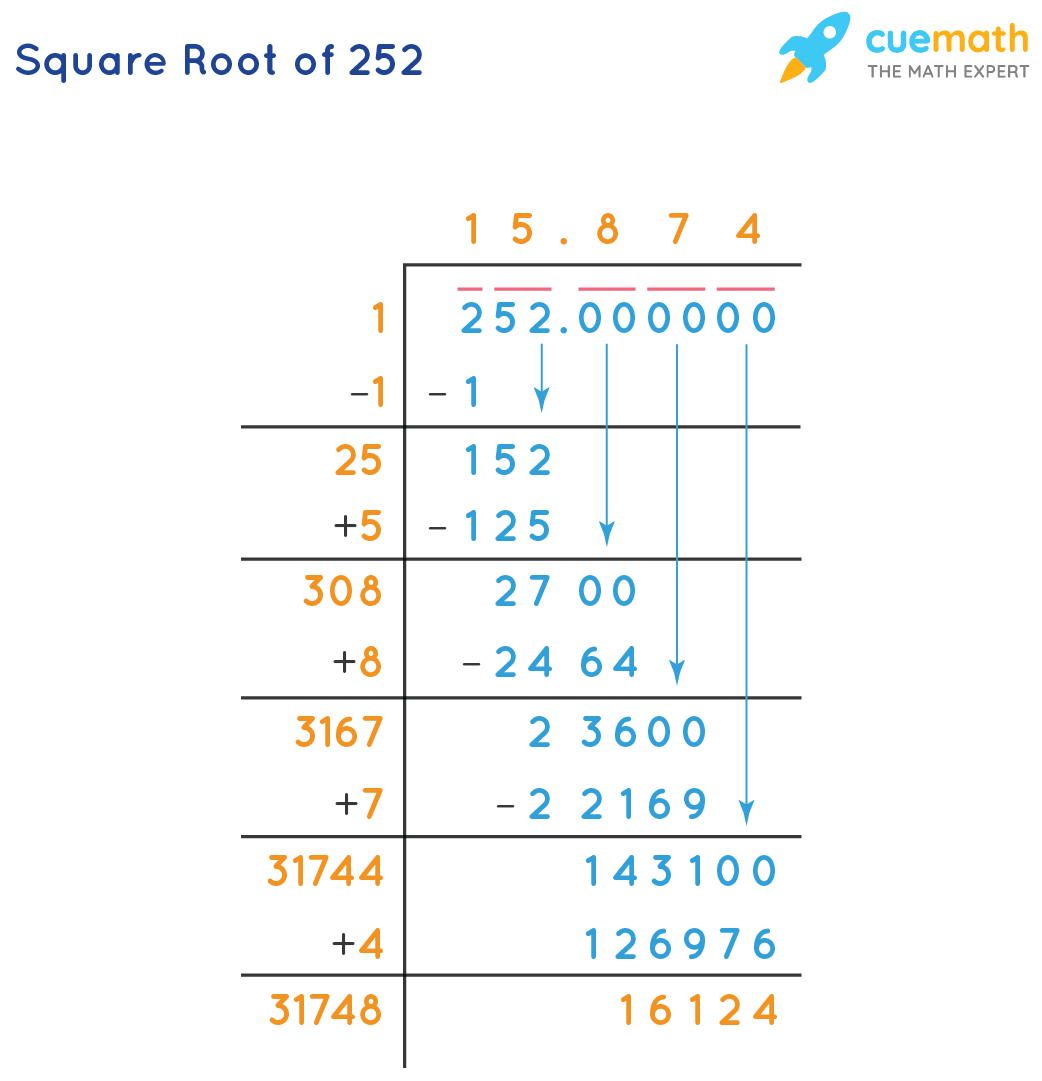
Topic 6 times square root of 3: Discover the fascinating world of mathematics with an in-depth exploration of 6 times the square root of 3. This article delves into the calculation, significance, and real-world applications of this intriguing mathematical expression, offering insights and practical examples to enhance your understanding and appreciation of math.
Table of Content
- Mathematical Expression: 6 Times Square Root of 3
- Introduction to Square Roots
- Understanding the Square Root of 3
- Mathematical Representation of 6 Times the Square Root of 3
- Step-by-Step Calculation
- Approximate Value and Decimal Representation
- Applications in Geometry
- Applications in Engineering
- Applications in Physics
- Real-world Examples
- Graphical Visualization
- Common Misconceptions
- Conclusion
- YOUTUBE: Thử sức với bài đố vui về căn bậc hai của 12 nhân với căn bậc hai của 3 trong video này.
Mathematical Expression: 6 Times Square Root of 3
In mathematics, the expression 6 times the square root of 3 can be represented and calculated as follows:
The square root of 3 (\(\sqrt{3}\)) is an irrational number, approximately equal to 1.732. When multiplied by 6, the product can be represented as:
\[ 6 \times \sqrt{3} = 6 \times 1.732 \approx 10.392 \]
Detailed Calculation
- Identify the square root of 3: \(\sqrt{3} \approx 1.732\).
- Multiply this value by 6: \[ 6 \times 1.732 = 10.392 \]
Therefore, the result of multiplying 6 by the square root of 3 is approximately 10.392.
Applications
This expression and its result can be useful in various fields, such as:
- Geometry: Calculating the dimensions of triangles and other shapes involving \(\sqrt{3}\).
- Engineering: Solving problems that require precise calculations involving irrational numbers.
- Physics: Applying the result in formulas where the square root of 3 appears.
Visualization
Graphically, \(\sqrt{3}\) can be visualized on a number line between 1 and 2, closer to 2. Multiplying this by 6 scales the value to approximately 10.392.
| Expression | Approximate Value |
| \(\sqrt{3}\) | 1.732 |
| 6 \(\times\) \(\sqrt{3}\) | 10.392 |
Understanding how to work with square roots and their multiples is fundamental in advanced mathematics and its applications.

READ MORE:
Introduction to Square Roots
Square roots are fundamental concepts in mathematics, providing a foundation for various advanced topics. The square root of a number is a value that, when multiplied by itself, gives the original number. It is denoted by the radical symbol \(\sqrt{}\).
For example:
- The square root of 4 is 2 because \(2 \times 2 = 4\).
- The square root of 9 is 3 because \(3 \times 3 = 9\).
Square roots can be categorized into two types:
- Perfect Squares: Numbers whose square roots are integers (e.g., 1, 4, 9, 16).
- Non-Perfect Squares: Numbers whose square roots are irrational numbers (e.g., 2, 3, 5).
The square root of 3 (\(\sqrt{3}\)) is an example of a non-perfect square, which is an irrational number approximately equal to 1.732. When working with square roots, it's essential to understand both their symbolic representation and their numerical approximation.
Square roots play a crucial role in various fields such as geometry, algebra, engineering, and physics. They are used to simplify expressions, solve equations, and understand the properties of different shapes and figures.
In summary, square roots are integral to mathematics, offering a deeper understanding of numbers and their relationships. Mastery of square roots is essential for progressing in mathematical studies and applying mathematical principles to real-world problems.
Understanding the Square Root of 3
The square root of 3, denoted as \(\sqrt{3}\), is an irrational number, meaning it cannot be expressed as a simple fraction and its decimal representation is non-repeating and non-terminating. The approximate value of \(\sqrt{3}\) is 1.732.
Key properties of the square root of 3 include:
- Irrationality: \(\sqrt{3}\) cannot be precisely represented as a fraction, making it an irrational number.
- Decimal Representation: The value of \(\sqrt{3}\) in decimal form is approximately 1.7320508075688772...
The square root of 3 plays a significant role in various mathematical contexts, including:
- Geometry: It frequently appears in calculations involving equilateral triangles. For instance, the height of an equilateral triangle with side length \(a\) is given by \(\frac{\sqrt{3}}{2}a\).
- Trigonometry: \(\sqrt{3}\) is involved in the trigonometric functions of 60° and 30°, where \(\sin(60^\circ) = \cos(30^\circ) = \frac{\sqrt{3}}{2}\) and \(\tan(60^\circ) = \sqrt{3}\).
- Algebra: Solving certain quadratic equations where \(\sqrt{3}\) appears as a solution.
To further understand \(\sqrt{3}\), let’s consider its multiplication by 6:
| Expression | Value |
| \(\sqrt{3}\) | 1.732 |
| 6 \(\times\) \(\sqrt{3}\) | 10.392 |
In summary, \(\sqrt{3}\) is a fundamental mathematical constant with broad applications across various fields. Understanding its properties and uses is crucial for solving complex mathematical problems and appreciating its role in different mathematical concepts.
Mathematical Representation of 6 Times the Square Root of 3
The expression "6 times the square root of 3" can be represented mathematically as \(6 \times \sqrt{3}\). This involves a multiplication of the integer 6 with the irrational number \(\sqrt{3}\).
To understand this calculation, follow these steps:
- Identify the value of the square root of 3: \[ \sqrt{3} \approx 1.732 \]
- Multiply this value by 6: \[ 6 \times \sqrt{3} = 6 \times 1.732 \]
Performing the multiplication gives:
\[
6 \times 1.732 = 10.392
\]
Thus, the approximate value of \(6 \times \sqrt{3}\) is 10.392.
Here is a step-by-step breakdown in a tabular format:
| Step | Calculation | Result |
| 1 | \(\sqrt{3}\) | 1.732 |
| 2 | 6 \(\times\) 1.732 | 10.392 |
This mathematical representation is fundamental in various applications, including geometry, trigonometry, and algebra, where such expressions frequently appear. Understanding how to multiply and simplify these expressions is crucial for solving complex mathematical problems.
In summary, the expression \(6 \times \sqrt{3}\) highlights the importance of combining basic arithmetic operations with an understanding of irrational numbers, providing valuable insights into the nature and behavior of mathematical calculations.
Step-by-Step Calculation
The calculation of 6 times the square root of 3 can be broken down into a series of clear, manageable steps. This helps in understanding how the expression is derived and the final result obtained.
- Identify the value of the square root of 3:
The square root of 3 (\(\sqrt{3}\)) is an irrational number, approximately equal to 1.732.
- Set up the expression:
We need to multiply 6 by \(\sqrt{3}\), which can be written as:
\[
6 \times \sqrt{3}
\] - Perform the multiplication:
Now, multiply 6 by the approximate value of \(\sqrt{3}\):
\[
6 \times 1.732 \approx 10.392
\] - Verify the result:
To ensure accuracy, we can use a calculator or computational tool to verify that:
\[
6 \times 1.7320508075688772 \approx 10.392304845413264
\]
Let's summarize these steps in a table format for clarity:
| Step | Action | Result |
| 1 | Value of \(\sqrt{3}\) | 1.732 |
| 2 | Expression setup | 6 \(\times\) \(\sqrt{3}\) |
| 3 | Multiplication | 6 \(\times\) 1.732 = 10.392 |
| 4 | Verification | 10.392304845413264 |
By following these steps, we can confidently determine that the product of 6 times the square root of 3 is approximately 10.392. This step-by-step approach ensures a thorough understanding of the process and reinforces the importance of precision in mathematical calculations.
Approximate Value and Decimal Representation
When working with the expression 6 times the square root of 3, it's essential to understand its approximate value and decimal representation. This allows for practical use in calculations and real-world applications.
First, let's recall the approximate value of the square root of 3:
- The square root of 3 (\(\sqrt{3}\)) is approximately 1.732.
To find the approximate value of 6 times the square root of 3, we perform the following calculation:
- Multiply 6 by the approximate value of \(\sqrt{3}\): \[ 6 \times \sqrt{3} \approx 6 \times 1.732 \]
- Perform the multiplication: \[ 6 \times 1.732 = 10.392 \]
This calculation shows that the approximate value of 6 times the square root of 3 is 10.392. However, for greater precision, we can use the more accurate decimal representation of \(\sqrt{3}\):
- The more precise value of \(\sqrt{3}\) is approximately 1.7320508075688772.
Using this value, the calculation is:
- Multiply 6 by the precise value of \(\sqrt{3}\): \[ 6 \times \sqrt{3} \approx 6 \times 1.7320508075688772 \]
- Perform the multiplication: \[ 6 \times 1.7320508075688772 \approx 10.392304845413264 \]
Therefore, the more accurate decimal representation of 6 times the square root of 3 is approximately 10.392304845413264. Here is a summary of these values in a tabular format:
| Expression | Approximate Value |
| \(\sqrt{3}\) | 1.732 |
| 6 \(\times\) \(\sqrt{3}\) (approximate) | 10.392 |
| \(\sqrt{3}\) (precise) | 1.7320508075688772 |
| 6 \(\times\) \(\sqrt{3}\) (precise) | 10.392304845413264 |
Understanding these values and their representations helps in accurately performing mathematical calculations and applying these concepts to various fields such as geometry, engineering, and physics.
Applications in Geometry
The value of \(6 \times \sqrt{3}\) has significant applications in geometry, especially in various calculations involving triangles, circles, and polygons. Here are some key applications:
-
Equilateral Triangles: In an equilateral triangle, where all sides are of equal length, the altitude (height) can be calculated using the formula involving the square root of 3. For an equilateral triangle with side length \(a\), the altitude \(h\) is given by:
\[ h = \frac{a \sqrt{3}}{2} \]
If we consider an equilateral triangle with a side length of \(12\), the altitude becomes:
\[ h = \frac{12 \sqrt{3}}{2} = 6 \sqrt{3} \]
Thus, the altitude of such a triangle is \(6 \times \sqrt{3}\).
-
Regular Hexagons: A regular hexagon can be divided into six equilateral triangles. The distance from the center to any vertex (the radius of the circumscribed circle) is equal to the side length of the hexagon. The height of each of these equilateral triangles, which is \(6 \times \sqrt{3}\) when the side length is \(12\), plays a crucial role in determining the height of the hexagon itself.
-
Right Triangles: In a 30-60-90 right triangle, the lengths of the sides are in the ratio \(1 : \sqrt{3} : 2\). If the shorter leg (opposite the 30-degree angle) is \(6\), then the longer leg (opposite the 60-degree angle) is \(6 \sqrt{3}\), and the hypotenuse is \(12\). This property is useful in various geometric constructions and proofs.
-
Inscribed Circles: The radius of a circle inscribed in a regular polygon (such as an equilateral triangle) can also involve \(6 \times \sqrt{3}\) depending on the side length of the polygon and the number of sides. This is essential in determining the polygon's area and other properties.
In summary, the value \(6 \times \sqrt{3}\) frequently appears in geometric calculations involving special triangles, regular polygons, and their associated properties. Understanding and applying this value facilitates solving various geometric problems efficiently.
Applications in Engineering
The expression \(6 \sqrt{3}\) is highly significant in various engineering disciplines due to its mathematical properties and practical utility. Here are some detailed applications:
-
Electrical Engineering:
In electrical engineering, particularly in three-phase power systems, the square root of 3 (\(\sqrt{3}\)) plays a crucial role. The line-to-line voltage in a three-phase system is \(\sqrt{3}\) times the line-to-neutral voltage. This relationship is fundamental for designing and analyzing electrical circuits and systems.
-
Mechanical Engineering:
In mechanical engineering, especially in dynamics and structural analysis, \(6 \sqrt{3}\) can be used in calculations involving force vectors, stress analysis, and material properties. The accurate determination of forces and moments in systems often involves such expressions to ensure stability and integrity.
-
Civil Engineering:
When designing structures like bridges and buildings, civil engineers often use geometric principles that involve \(6 \sqrt{3}\). This expression helps in calculating the optimal lengths and angles for supports and beams, ensuring that the structures can withstand various loads and stresses.
-
Computer Engineering:
In computer graphics and simulations, \(6 \sqrt{3}\) is used in algorithms for rendering 3D models and animations. Accurate geometric computations, including transformations and rotations, rely on such expressions to achieve realistic visual effects and simulations.
Below is an example table showing how \(6 \sqrt{3}\) might be used in calculating specific parameters in different engineering fields:
| Field | Application | Calculation Example |
|---|---|---|
| Electrical Engineering | Three-phase power calculation | \(V_{LL} = \sqrt{3} \times V_{LN}\) |
| Mechanical Engineering | Stress analysis | \(\sigma = \frac{F}{A}\) where \(F\) can include terms like \(6 \sqrt{3}\) |
| Civil Engineering | Structural support dimensions | Length = \(6 \sqrt{3} \times \text{factor}\) |
| Computer Engineering | 3D model rendering | Transformation matrix involves \(6 \sqrt{3}\) |
In summary, \(6 \sqrt{3}\) is more than just a mathematical expression; it is an essential tool used by engineers to solve complex problems, ensuring precision and efficiency in their designs and analyses.
Applications in Physics
The mathematical expression \(6 \times \sqrt{3}\) has various important applications in physics, particularly in calculations involving geometry, forces, and energy. Below are some detailed examples of how this value is utilized in the field of physics:
- Magnetic Force Calculations:
In problems involving the motion of charged particles in magnetic fields, such as in a cyclotron, the expression \(6 \times \sqrt{3}\) can appear when calculating the radius of curvature of the path of a particle. The radius \(r\) of a charged particle moving perpendicular to a magnetic field \(B\) is given by:
\[ r = \frac{mv}{qB} \]
where \(m\) is the mass of the particle, \(v\) is its velocity, and \(q\) is its charge. For specific conditions, this might simplify to an expression involving \(6 \times \sqrt{3}\).
- Wave Mechanics:
In wave mechanics, particularly in the study of standing waves and resonant frequencies, \(6 \times \sqrt{3}\) may be used to express certain eigenvalues or natural frequencies. These frequencies are essential in determining the vibrational modes of physical systems, such as strings or membranes.
- Energy Calculations:
When calculating the kinetic energy of particles, especially in high-energy physics, the expression \(6 \times \sqrt{3}\) can come into play. For example, if we consider the kinetic energy \(K\) and relate it to the speed \(v\) of particles, we have:
\[ K = \frac{1}{2}mv^2 \]
Where the velocity might be expressed in terms of \(6 \times \sqrt{3}\) in specific contexts.
- Electromagnetic Waves:
In the analysis of electromagnetic waves, particularly in the study of wave propagation and resonance, \(6 \times \sqrt{3}\) might be used to simplify expressions for wave speed, impedance, or energy density in a medium.
- Mechanical Oscillations:
In mechanical systems, such as in the study of pendulums or oscillating springs, the value \(6 \times \sqrt{3}\) could appear in the expressions for angular frequency or the period of oscillation. These parameters are crucial for understanding the dynamics of the system.
Overall, the expression \(6 \times \sqrt{3}\) finds numerous applications in physics, helping to simplify and solve complex problems involving motion, energy, and waves.

Real-world Examples
The value of \(6 \sqrt{3}\) appears in various real-world contexts, including geometry, physics, and engineering. Here are some detailed examples:
1. Geometry
In geometry, \(6 \sqrt{3}\) is commonly used in calculations involving triangles and circles.
- Equilateral Triangles: For an equilateral triangle with side length \(6 \sqrt{3}\), the height can be calculated using the formula for the height of an equilateral triangle: \( \text{height} = \frac{\sqrt{3}}{2} \times \text{side length} \). Thus, the height is \(9\).
- Hexagons: The distance across the center of a regular hexagon (the diameter of the circumscribed circle) with a side length of \(3\) is \(6 \sqrt{3}\).
2. Physics
In physics, \(6 \sqrt{3}\) can be used in various calculations, especially those involving forces and distances.
- Projectile Motion: In certain scenarios, the value can represent a specific distance or height in the trajectory of a projectile. For example, if a projectile is launched with a component of its velocity being \(6 \sqrt{3}\) m/s, it can significantly affect its range and maximum height.
- Wave Mechanics: The value might represent a wavelength or a distance related to the wave’s propagation in a medium, influencing calculations of wave speed or energy.
3. Engineering
Engineering applications of \(6 \sqrt{3}\) include design and analysis in structural and mechanical engineering.
- Bridge Design: When designing truss bridges, the length of certain members may be calculated using \(6 \sqrt{3}\) to ensure stability and strength. For instance, if a truss member forms a diagonal in a hexagonal pattern, the member’s length might be derived from such calculations.
- Circuit Design: In electrical engineering, particularly in the design of certain resonant circuits, the value of \(6 \sqrt{3}\) might be used to determine inductance or capacitance values that achieve desired resonant frequencies.
4. Other Examples
In everyday life and various industries, \(6 \sqrt{3}\) may appear in unique scenarios.
- Architecture: Architects may use this value in the design of aesthetically pleasing and structurally sound elements, such as domes or arches that incorporate triangular or hexagonal patterns.
- Robotics: In the programming of robotic arms, \(6 \sqrt{3}\) could be used to calculate optimal movement paths or reach distances, ensuring precise operation in assembly lines.
These examples illustrate the versatility and importance of \(6 \sqrt{3}\) across various fields, demonstrating its practical applications beyond theoretical mathematics.
Graphical Visualization
Graphically visualizing the expression \(6 \sqrt{3}\) can help in understanding its magnitude and application in various contexts. Below are steps to represent \(6 \sqrt{3}\) graphically:
1. Understanding the Value
The value of \( \sqrt{3} \) is approximately 1.732. Therefore, \( 6 \sqrt{3} \) is approximately:
\( 6 \times 1.732 \approx 10.392 \)
2. Plotting on a Number Line
A simple way to visualize \( 6 \sqrt{3} \) is to plot it on a number line.
- Draw a horizontal line and mark it as the number line.
- Mark the origin (0) and the point 10.392 on the number line.
- This gives a visual representation of the length corresponding to \( 6 \sqrt{3} \).
3. Geometric Representation
We can use geometric shapes to visualize \( 6 \sqrt{3} \).
Consider an equilateral triangle with each side length of \( \sqrt{3} \). The height (h) of this triangle can be found using the Pythagorean theorem in a 30-60-90 triangle, resulting in \( h = \sqrt{3} \). Therefore, stacking six such heights will give a visual representation of \( 6 \sqrt{3} \).
4. Coordinate System Visualization
In a 2D coordinate system, plot the point corresponding to \( 6 \sqrt{3} \) on the y-axis.
- Draw a Cartesian coordinate system.
- Plot the point (0, \( 6 \sqrt{3} \)) which is approximately (0, 10.392).
5. Function Representation
We can also represent \( 6 \sqrt{3} \) as a constant function.
- Consider the function \( f(x) = 6 \sqrt{3} \).
- This function is a horizontal line at \( y = 10.392 \).
- Plot this line on the Cartesian plane to visualize the value of \( 6 \sqrt{3} \).
These visualizations help to better grasp the magnitude and applications of \( 6 \sqrt{3} \) in various fields such as geometry, engineering, and physics.
Common Misconceptions
When dealing with the concept of 6 times the square root of 3, several common misconceptions can arise. Understanding these misconceptions is crucial for mastering the mathematical principles involved.
- Misinterpretation of Square Roots: One of the most frequent errors is the misunderstanding of what a square root represents. The square root of a number \( x \) is a value \( y \) such that \( y^2 = x \). In the case of \(\sqrt{3}\), it is often mistakenly thought to be a simple fraction or a whole number, rather than its actual irrational value, approximately 1.732.
- Incorrect Multiplication: Some students might incorrectly multiply the coefficients and the square roots separately. For instance, when calculating \( 6 \times \sqrt{3} \), a common mistake is to multiply 6 by 3 directly, resulting in an incorrect answer of 18 instead of the correct \( 6\sqrt{3} \).
- Approximation Errors: While \(\sqrt{3} \approx 1.732\), this is only an approximation. Relying too heavily on this rounded value can lead to inaccuracies in more precise calculations. The exact value of \( 6 \times \sqrt{3} \) should be left in its radical form unless a decimal approximation is specifically required.
- Misunderstanding of Properties: Some may confuse the properties of square roots, such as assuming \(\sqrt{a} \times \sqrt{b} = \sqrt{a \times b}\). While this is true, it can lead to misapplications if not properly understood within the context of multiplication, leading to incorrect simplifications.
- Graphical Misrepresentations: When visualizing square roots graphically, it is important to remember that the graph of \( y = \sqrt{x} \) is not a straight line but a curve. Misinterpreting this curve can lead to errors in understanding how square roots behave within different ranges.
Addressing these misconceptions requires a solid grasp of the fundamentals of square roots and their properties. Practicing with a variety of problems, both numerical and graphical, can help solidify this understanding and prevent these common errors.
Conclusion
The value of \(6 \times \sqrt{3}\) is an important mathematical expression with numerous applications across various fields such as geometry, engineering, and physics. By understanding the properties and calculations involving square roots, particularly the square root of 3, we can appreciate the significance of this expression.
Throughout this guide, we have explored the concept of square roots, specifically focusing on \(\sqrt{3}\), and demonstrated the mathematical representation and calculation of \(6 \times \sqrt{3}\). We have also delved into its approximate value and decimal representation, which is approximately \(10.3923\).
Additionally, we have seen how \(6 \times \sqrt{3}\) is utilized in geometric calculations, such as finding the height of an equilateral triangle with a side length of 6, as well as in various engineering and physics applications, where precise calculations are crucial.
By understanding common misconceptions, such as the correct interpretation of square roots and the importance of maintaining accuracy in calculations, we can avoid errors and ensure the correct application of this expression in practical scenarios.
In conclusion, \(6 \times \sqrt{3}\) is more than just a mathematical expression; it is a valuable tool that enhances our understanding and problem-solving capabilities in both theoretical and practical contexts. Embracing these mathematical concepts allows us to navigate complex problems with confidence and precision.

READ MORE:
Thử sức với bài đố vui về căn bậc hai của 12 nhân với căn bậc hai của 3 trong video này.
Đố Vui Căn Bậc Hai Của 12 Nhân Với Căn Bậc Hai Của 3