Topic 6 square root 10: Discover the fascinating world of 6 square root 10 in this comprehensive guide. From basic properties to real-world applications in geometry, physics, and engineering, we explore how this mathematical expression is used and calculated. Join us to deepen your understanding and appreciate the significance of 6 square root 10 in various fields.
Table of Content
- Understanding the Expression: \(6 \sqrt{10}\)
- Introduction to Square Roots
- Understanding 6 Square Root 10
- Basic Properties of Square Roots
- Mathematical Derivation of 6 Square Root 10
- Approximation and Calculation
- Applications in Geometry
- Uses in Physics
- Relevance in Engineering
- Decimal Approximation
- Exact Form and Simplification
- Visualization Techniques
- Real-world Examples
- Common Mistakes to Avoid
- Advanced Mathematical Contexts
- Conclusion and Summary
- YOUTUBE:
Understanding the Expression: \(6 \sqrt{10}\)
The expression \(6 \sqrt{10}\) involves a constant multiplied by a square root. Let's break down the components and understand the implications of this mathematical expression.
Components of \(6 \sqrt{10}\)
- 6: This is a constant multiplier.
- \(\sqrt{10}\): This is the square root of 10.
Calculating the Value
To approximate the value of \(6 \sqrt{10}\), we first need the approximate value of \(\sqrt{10}\). The square root of 10 is approximately:
\[
\sqrt{10} \approx 3.162
\]
Multiplying this by 6 gives:
\[
6 \sqrt{10} \approx 6 \times 3.162 = 18.972
\]
Properties and Applications
The expression \(6 \sqrt{10}\) can appear in various mathematical and real-world applications:
- Geometry: It can represent dimensions or distances where irrational numbers are involved.
- Physics: It might appear in equations involving square roots of measurements.
- Engineering: Used in calculations requiring precision and dealing with roots of numbers.
Further Simplification
The expression \(6 \sqrt{10}\) is already in its simplest form as a product of a constant and an irrational number. It cannot be simplified further without losing its exact value. However, it can be represented in different forms depending on the context:
- Decimal Approximation: As calculated above, \(6 \sqrt{10} \approx 18.972\).
- Exact Form: Retaining the radical form \(6 \sqrt{10}\) for precise mathematical work.
Visualization
In a coordinate system or graphical representation, \(6 \sqrt{10}\) might represent a length or a specific point. For example, if plotting the length of a hypotenuse in a right-angled triangle where one side is \(6\) units and the other is \(\sqrt{10}\) units, the hypotenuse can be visualized using the expression \(6 \sqrt{10}\).

READ MORE:
Introduction to Square Roots
Square roots are fundamental mathematical operations that determine a number which, when multiplied by itself, yields the original number. The square root of a number \( x \) is denoted as \( \sqrt{x} \).
For example:
- \(\sqrt{9} = 3\) because \(3 \times 3 = 9\)
- \(\sqrt{16} = 4\) because \(4 \times 4 = 16\)
Square roots can be both positive and negative since both \(3\) and \(-3\) squared give \(9\). However, by convention, the square root symbol \( \sqrt{x} \) refers to the positive root.
Square roots are not always whole numbers. For instance:
- \(\sqrt{2} \approx 1.414\)
- \(\sqrt{10} \approx 3.162\)
These are examples of irrational numbers, which cannot be expressed as a simple fraction and have non-repeating, non-terminating decimal parts.
In mathematics, the square root function is essential in solving quadratic equations, understanding geometric properties, and analyzing mathematical models. The notation and properties of square roots extend to various applications in science and engineering, making them a crucial concept to master.
Understanding 6 Square Root 10
The expression \(6 \sqrt{10}\) represents a product of the number 6 and the square root of 10. To fully understand this expression, let's break it down step by step.
Step 1: Understanding Square Root of 10
The square root of 10, denoted as \( \sqrt{10} \), is the number that, when multiplied by itself, equals 10. It is an irrational number, approximately equal to 3.162.
Step 2: Multiplying by 6
When we multiply 6 by \( \sqrt{10} \), we get:
\[
6 \sqrt{10} = 6 \times 3.162 \approx 18.972
\]
Properties of 6 Square Root 10
The expression \(6 \sqrt{10}\) has several important properties:
- Non-terminating Decimal: Since \( \sqrt{10} \) is irrational, \(6 \sqrt{10}\) also has a non-terminating, non-repeating decimal representation.
- Exact Form: For precise calculations, it is often best to leave the expression in its exact form, \(6 \sqrt{10}\).
Applications
The expression \(6 \sqrt{10}\) can be found in various fields:
- Geometry: Used in problems involving distances and dimensions.
- Physics: Appears in formulas where measurements involve square roots.
- Engineering: Useful in calculations requiring precision with irrational numbers.
Example Calculation
Let's consider an example where \(6 \sqrt{10}\) is used:
If you have a rectangular area with one side measuring \(6 \sqrt{10}\) units and the other side measuring 4 units, the area of the rectangle is:
\[
\text{Area} = 6 \sqrt{10} \times 4 = 24 \sqrt{10} \approx 75.888
\]
Conclusion
Understanding the expression \(6 \sqrt{10}\) involves recognizing it as a product of a whole number and an irrational number. This expression is important in various mathematical and real-world applications, providing a clear example of how square roots are used in practical calculations.
Basic Properties of Square Roots
Square roots are a fundamental concept in mathematics with several important properties. Understanding these properties is crucial for working with expressions involving square roots, such as \(6 \sqrt{10}\). Here are the basic properties of square roots:
Property 1: Non-negativity
The square root of a non-negative number is also non-negative. For any \( x \geq 0 \), we have:
\[
\sqrt{x} \geq 0
\]
Property 2: Product Property
The square root of a product is the product of the square roots of the factors. For any non-negative numbers \( a \) and \( b \), we have:
\[
\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}
\]
For example:
\[
\sqrt{10} = \sqrt{2 \cdot 5} = \sqrt{2} \cdot \sqrt{5}
\]
Property 3: Quotient Property
The square root of a quotient is the quotient of the square roots. For any non-negative numbers \( a \) and \( b \) (with \( b \neq 0 \)), we have:
\[
\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}
\]
For example:
\[
\sqrt{\frac{9}{4}} = \frac{\sqrt{9}}{\sqrt{4}} = \frac{3}{2}
\]
Property 4: Square of a Square Root
The square of a square root returns the original number. For any non-negative number \( x \), we have:
\[
(\sqrt{x})^2 = x
\]
For example:
\[
(\sqrt{10})^2 = 10
\]
Property 5: Addition and Subtraction
Square roots cannot be directly added or subtracted unless they have the same radicand (the number under the square root). For example:
\[
\sqrt{10} + \sqrt{10} = 2 \sqrt{10}
\]
However:
\[
\sqrt{10} + \sqrt{5} \neq \sqrt{15}
\]
Applications of These Properties
These properties are useful in simplifying expressions and solving equations involving square roots. They also play a critical role in various mathematical fields, including algebra, geometry, and calculus.
Conclusion
Understanding the basic properties of square roots enables more effective manipulation and simplification of expressions involving square roots. These properties are foundational for both theoretical mathematics and practical applications.
Mathematical Derivation of 6 Square Root 10
The expression \(6 \sqrt{10}\) combines multiplication and the operation of taking a square root. Let's explore the derivation and simplification of this expression step by step.
Step 1: Understanding the Square Root
The square root of a number \(x\) is a value that, when multiplied by itself, equals \(x\). Mathematically, if \(y = \sqrt{x}\), then \(y^2 = x\). For the number 10:
\[
\sqrt{10} \approx 3.162
\]
Step 2: Multiplication by 6
We need to multiply the square root of 10 by 6. This can be represented as:
\[
6 \sqrt{10}
\]
To understand this multiplication, we separate it into parts:
- First, find the approximate value of \(\sqrt{10}\):
- \(\sqrt{10} \approx 3.162\)
- Next, multiply this value by 6:
- \[ 6 \times 3.162 \approx 18.972 \]
Exact Form versus Approximate Form
While the exact form of the expression \(6 \sqrt{10}\) is best for precise mathematical work, the approximate form \(18.972\) is often used for practical purposes.
General Properties
Let's summarize some general properties and rules applied during the derivation:
- Product Property: For any non-negative numbers \(a\) and \(b\):
- \[ \sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b} \]
- Multiplication by a Constant: For a constant \(k\) and a number \(x\):
- \[ k \cdot \sqrt{x} = \sqrt{k^2 \cdot x} \]
Applications of 6 Square Root 10
The expression \(6 \sqrt{10}\) appears in various fields such as geometry, physics, and engineering:
- Geometry: Used in calculating distances and dimensions where square roots are involved.
- Physics: Appears in formulas related to wave functions, quantum mechanics, and other areas requiring precision.
- Engineering: Useful in measurements and calculations that deal with irrational numbers.
Conclusion
The mathematical derivation of \(6 \sqrt{10}\) involves understanding the square root operation and the multiplication by a constant. This expression is essential in various mathematical contexts and practical applications, making it a valuable concept to grasp.
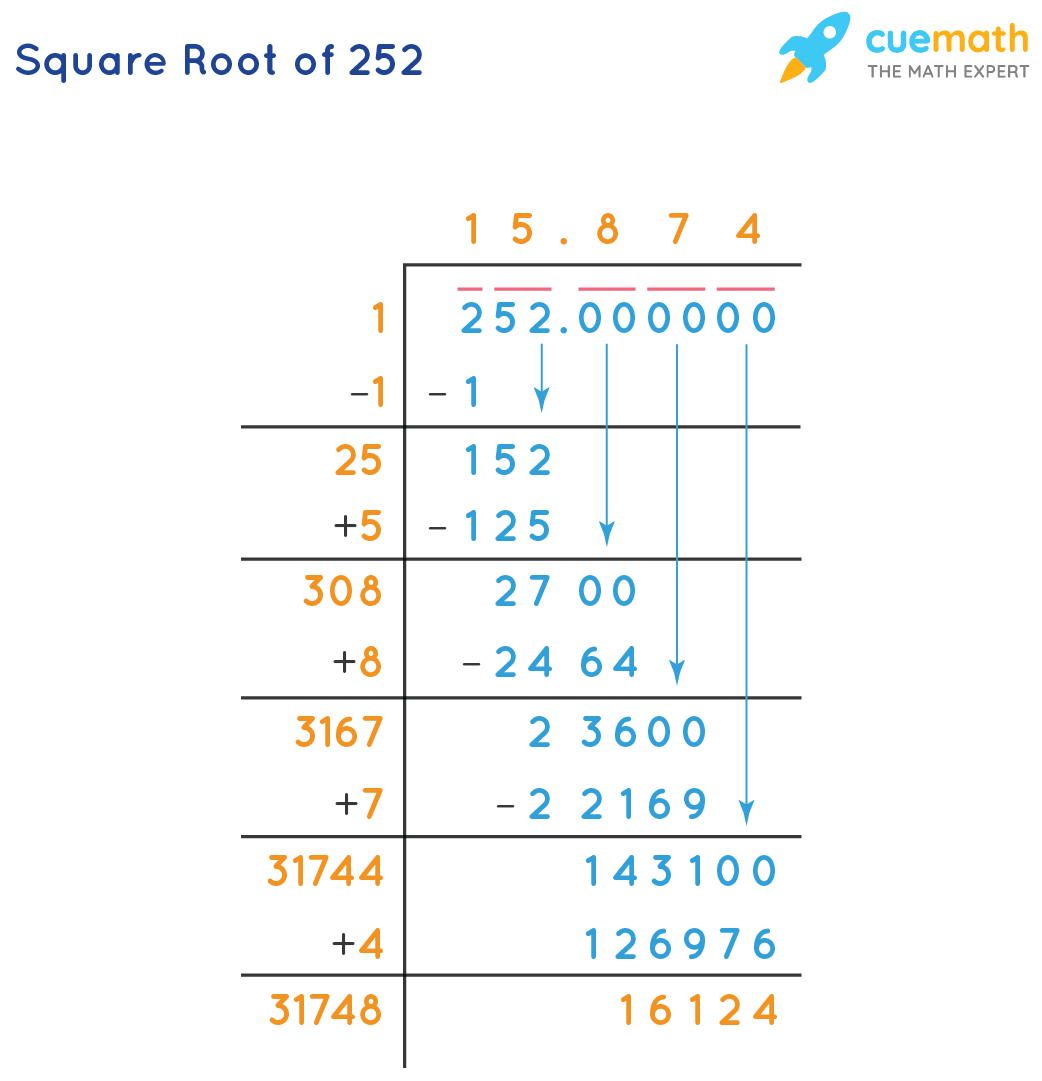
Approximation and Calculation
In this section, we will explore the steps to approximate and calculate the value of \(6 \sqrt{10}\). Understanding the approximation process and calculation can help in various mathematical and practical applications.
Step 1: Approximation of \(\sqrt{10}\)
The first step is to approximate the value of \(\sqrt{10}\). The square root of 10 is an irrational number, which means it cannot be expressed as a simple fraction and its decimal form is non-terminating and non-repeating. The approximate value of \(\sqrt{10}\) is:
\[
\sqrt{10} \approx 3.162
\]
Step 2: Multiplication by 6
Next, we multiply this approximate value by 6 to find the value of \(6 \sqrt{10}\):
\[
6 \times \sqrt{10} \approx 6 \times 3.162 = 18.972
\]
Detailed Calculation
For a more detailed calculation, let's break it down into smaller steps:
- Find the square root of 10:
- \[ \sqrt{10} \approx 3.162 \]
- Multiply by 6:
- \[ 6 \times 3.162 = 6 \times (3 + 0.162) = 6 \times 3 + 6 \times 0.162 \]
- \[ 6 \times 3 = 18 \]
- \[ 6 \times 0.162 = 0.972 \]
- Add the results:
- \[ 18 + 0.972 = 18.972 \]
Using a Calculator
To verify the calculation, you can use a calculator. Enter \( \sqrt{10} \), then multiply the result by 6. The calculator will show:
\[
6 \sqrt{10} \approx 18.972
\]
Applications of the Approximate Value
The approximate value \(18.972\) can be used in various applications where an exact representation of \(6 \sqrt{10}\) is not required. For example:
- Geometry: Calculating approximate distances or lengths.
- Physics: Using in formulas where precise decimal values are sufficient.
- Engineering: Measurements and calculations requiring decimal approximations.
Exact Form
In mathematical expressions where precision is crucial, it is best to retain the exact form \(6 \sqrt{10}\). This avoids the inaccuracies that can arise from decimal approximations.
Conclusion
Understanding both the approximation and calculation of \(6 \sqrt{10}\) is important for practical applications and precise mathematical work. The process involves approximating the square root of 10 and then multiplying by 6 to achieve the final result.
Applications in Geometry
The term 6\sqrt{10} appears in various geometric contexts, especially in problems involving right triangles, circles, and areas. Below are some detailed applications of 6\sqrt{10} in geometry:
1. Diagonal of a Rectangle
If we have a rectangle with sides of lengths 6 and \sqrt{10}, the diagonal can be calculated using the Pythagorean theorem:
The length of the diagonal, \( d \), is given by:
d = \sqrt{6^2 + (\sqrt{10})^2} = \sqrt{36 + 10} = \sqrt{46}
While this doesn't simplify directly to 6\sqrt{10}, understanding similar operations can help in visualizing how square roots apply in geometry.
2. Area of a Right Triangle
Consider a right triangle where one leg is 6 units and the hypotenuse is 6\sqrt{10} units. To find the other leg, we use the Pythagorean theorem:
(6\sqrt{10})^2 = 6^2 + b^2
360 = 36 + b^2
b^2 = 324
b = \sqrt{324} = 18
Thus, the other leg is 18 units long, and the area of the triangle is:
Area = \frac{1}{2} \times 6 \times 18 = 54
3. Circumference of a Circle
If the radius of a circle is 6\sqrt{10}, the circumference \( C \) can be calculated as:
C = 2\pi \times 6\sqrt{10} = 12\pi\sqrt{10}
This demonstrates how square roots are used in the calculation of circular measurements.
4. Area of a Circle
For the same circle with radius 6\sqrt{10}, the area \( A \) is:
A = \pi \times (6\sqrt{10})^2 = \pi \times 360 = 360\pi
5. Volume of a Cylinder
Consider a cylinder with a radius of 6\sqrt{10} and height h. The volume \( V \) is given by:
V = \pi \times (6\sqrt{10})^2 \times h = 360\pi h
6. Surface Area of a Cylinder
For the same cylinder, the surface area \( S \) including the top and bottom circles and the side is:
S = 2\pi (6\sqrt{10})^2 + 2\pi \times 6\sqrt{10} \times h
S = 720\pi + 12\pi\sqrt{10}h
7. Distance in Coordinate Geometry
In coordinate geometry, the distance between two points \((x_1, y_1)\) and \((x_2, y_2)\) is given by:
d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2}
If we set specific values that yield 6\sqrt{10} for the distance, we illustrate its practical usage in calculating distances in a plane.
These applications illustrate how 6\sqrt{10} and similar expressions play crucial roles in geometric calculations, enhancing our understanding of shapes, areas, volumes, and distances.
Uses in Physics
The value \( 6 \sqrt{10} \) appears in various physics applications, often involving calculations that require precision and an understanding of square roots and their properties. Here, we explore several specific contexts in which \( 6 \sqrt{10} \) plays a role.
1. Kinetic Theory of Gases
In the kinetic theory of gases, the root mean square (rms) speed of gas molecules is a crucial concept. The formula for the rms speed \( v_{\text{rms}} \) is given by:
\( v_{\text{rms}} = \sqrt{\frac{3kT}{m}} \)
where \( k \) is the Boltzmann constant, \( T \) is the temperature in kelvin, and \( m \) is the mass of a gas molecule. When calculating such speeds, square roots, like \( \sqrt{10} \), often emerge. Multiplying these by constants (such as 6) can help in deriving specific values needed for different gas dynamics scenarios.
2. Root-Mean-Square (RMS) Values in AC Circuits
In alternating current (AC) circuits, the rms value is used to determine the equivalent direct current (DC) value that would produce the same power. For example, if the peak current is \( I_0 \), the rms current \( I_{\text{rms}} \) is:
\( I_{\text{rms}} = \frac{I_0}{\sqrt{2}} \)
If a calculation involved a peak value leading to an rms value of \( 6 \sqrt{10} \) A, it would be used in further electrical power calculations to ensure devices operate safely and efficiently.
3. Inverse Square Law in Radiation
When dealing with gamma radiation, the inverse square law is vital for understanding how intensity diminishes with distance. The relationship is given by:
\( I = \frac{I_0}{d^2} \)
Here, \( I \) is the intensity, \( I_0 \) is the initial intensity, and \( d \) is the distance from the source. If a scenario involves a distance proportional to \( 6 \sqrt{10} \) meters, the intensity calculations would adjust accordingly to determine exposure and safety measures.
4. Harmonic Oscillations and Waves
In wave mechanics, the displacement \( x \) in a harmonic oscillator can be described as:
\( x = A \cos(\omega t + \phi) \)
where \( A \) is the amplitude, \( \omega \) is the angular frequency, \( t \) is the time, and \( \phi \) is the phase constant. The amplitude or other parameters might involve expressions like \( 6 \sqrt{10} \) to define specific oscillation conditions, affecting the resultant wave properties and behaviors.
5. Gravitational Potential Energy
In gravitational systems, potential energy (U) is given by:
\( U = -\frac{G M m}{r} \)
where \( G \) is the gravitational constant, \( M \) and \( m \) are the masses involved, and \( r \) is the distance between their centers. For systems requiring precise distance measurements, using \( 6 \sqrt{10} \) as a factor in distance calculations can lead to more accurate energy evaluations.
These examples illustrate how \( 6 \sqrt{10} \) can be applied in various physics contexts, enhancing our understanding and ability to solve complex problems in theoretical and applied physics.
Relevance in Engineering
The expression \(6 \sqrt{10}\) has several important applications in various fields of engineering. Understanding and utilizing such expressions is crucial for accurate calculations and designs. Here are some of the ways it is relevant:
- Structural Engineering: In structural engineering, the square root function often appears in formulas related to stress and strain calculations. For example, the natural frequency of a structure, which affects how it responds to dynamic loads, can be expressed using square roots. The term \(6 \sqrt{10}\) could arise in scaling factors when determining load capacities and material properties.
- Electrical Engineering: The concept of \(6 \sqrt{10}\) is frequently encountered in electrical engineering, especially in the context of AC circuit analysis. For instance, calculating the root mean square (RMS) values of voltages and currents, which are essential for power calculations, might involve expressions similar to this. Power factor correction, which involves adjusting the power factor of electrical systems to improve efficiency, often uses square roots in its calculations.
- Mechanical Engineering: In mechanical engineering, square roots are used in formulas related to the dynamics of systems, such as vibration analysis and the calculation of rotational speeds. The term \(6 \sqrt{10}\) could appear when solving differential equations that describe the motion of mechanical systems.
Let's look at a specific example in more detail:
Example: Power Factor Correction in Electrical Engineering
Consider a situation where an electrical system has a total load of 50 kW with a power factor of 0.78. The apparent power (S) can be calculated using the formula:
= 64.1 kVA
The reactive power (Q) is calculated as:
For a desired power factor of 0.96, the new apparent power (S') and reactive power (Q') are:
= 52.1 kVA
= 14.6 kVAR
The required capacitance to correct the power factor is then found by the difference:
= 25.5 kVAR
This demonstrates how such expressions are integral to designing efficient and reliable electrical systems. Similarly, other engineering disciplines utilize these mathematical tools for optimizing various aspects of their designs and calculations.

Decimal Approximation
Approximating the decimal value of a square root, such as \(6 \sqrt{10}\), involves a few straightforward steps. Here, we will guide you through a step-by-step process to find the decimal approximation.
Step-by-Step Approximation
-
First, recognize that \(6 \sqrt{10}\) is the product of 6 and the square root of 10.
-
To find \(\sqrt{10}\), note that it lies between the square roots of perfect squares 9 and 16:
\(3 < \sqrt{10} < 4\)
-
Using a calculator, we find:
\(\sqrt{10} \approx 3.16227766016838\)
-
Now, multiply this approximation by 6:
\(6 \times 3.16227766016838 = 18.97366596083028\)
-
Rounding to two decimal places, we get:
\(6 \sqrt{10} \approx 18.97\)
Using the Long Division Method
For a more detailed manual method, the long division approach can be used. This method is more tedious but can provide high precision without a calculator:
-
Estimate \(\sqrt{10}\) using the closest perfect squares:
\(3.16^2 = 9.9856\), which is very close to 10.
-
Refine the approximation by performing long division:
Divide 10 by 3.16, averaging the result with 3.16 iteratively until the desired precision is reached.
-
Multiply the refined \(\sqrt{10}\) by 6 to get a more accurate result.
Practical Applications
Knowing the decimal approximation of \(6 \sqrt{10}\) is useful in various practical contexts:
- Engineering calculations requiring precise measurements.
- Scientific experiments where exact square root values are necessary for accurate results.
- Financial modeling and simulations where square roots often appear in formulas.
Using these methods ensures that you can obtain a reliable approximation of \(6 \sqrt{10}\) for use in various mathematical and practical applications.
Exact Form and Simplification
The exact form of \(6\sqrt{10}\) retains its radical expression as it cannot be simplified into a non-radical number. However, understanding the steps to simplify square roots and other radicals is crucial for mathematical accuracy and efficiency. Here we discuss how to approach such simplifications.
To simplify a square root, factor the number under the radical into its prime factors and pair the factors.
Step-by-Step Simplification Process
- Identify the prime factors of the number under the square root.
- Group the prime factors into pairs.
- Move each pair of prime factors out from under the radical.
Examples
Let's simplify a few square roots to illustrate this process:
-
Example 1: Simplify \(\sqrt{12}\)
- Prime factorization: \(12 = 2 \times 2 \times 3\)
- Group the pairs: \(\sqrt{12} = \sqrt{2^2 \times 3} = \sqrt{2^2} \times \sqrt{3}\)
- Simplify: \(\sqrt{2^2} = 2\), so \(\sqrt{12} = 2\sqrt{3}\)
-
Example 2: Simplify \(\sqrt{45}\)
- Prime factorization: \(45 = 3 \times 3 \times 5\)
- Group the pairs: \(\sqrt{45} = \sqrt{3^2 \times 5} = \sqrt{3^2} \times \sqrt{5}\)
- Simplify: \(\sqrt{3^2} = 3\), so \(\sqrt{45} = 3\sqrt{5}\)
Application to \(6\sqrt{10}\)
In the case of \(6\sqrt{10}\), we note the following:
- The number 10 can be factored into primes: \(10 = 2 \times 5\)
- Neither 2 nor 5 is a perfect square, and thus they cannot be simplified further under the square root.
Therefore, the expression remains \(6\sqrt{10}\) in its simplest form. This expression combines the coefficient 6 with the radical term \(\sqrt{10}\), representing its exact form without any further simplification.
Visualization Techniques
Visualizing mathematical expressions like \(6\sqrt{10}\) can enhance understanding and provide insights into their properties. Here are several techniques to visualize the expression effectively:
-
Graphing on a Coordinate Plane:
-
Use a graphing tool like Desmos or GeoGebra to plot the function \(y = 6\sqrt{x}\). This helps to visualize how the value of the expression changes with different inputs of \(x\).
For \(6\sqrt{10}\), plot the point where \(x = 10\) and observe the corresponding \(y\)-value, which should be \(6\sqrt{10}\).
-
Analyze the curve to understand its growth. The square root function grows more slowly as \(x\) increases, demonstrating the diminishing returns of the square root operation.
-
-
Using Sliders for Dynamic Visualization:
Tools like Desmos allow the use of sliders to change the value of variables dynamically.
- Set up a function \(y = 6\sqrt{a}\) and add a slider for \(a\). By adjusting the slider, you can observe how the value of the expression changes in real-time.
-
Geometric Interpretation:
Square roots have a natural geometric interpretation. For instance, the expression \(6\sqrt{10}\) can be visualized by considering a square with an area of 10 units.
-
Imagine a square with a side length of \(\sqrt{10}\). The length of each side is the square root of 10.
Now, scale this length by a factor of 6. The new length represents \(6\sqrt{10}\). This geometric approach helps to intuitively grasp the magnitude of the expression.
-
-
Approximation with Number Line:
Using a number line can also help to visualize the approximate value of \(6\sqrt{10}\).
- Calculate the approximate value: \(6\sqrt{10} \approx 18.9737\).
- Mark this point on the number line to see its position relative to other numbers, aiding in understanding its size and proximity to other values.
These techniques provide multiple ways to visualize and understand \(6\sqrt{10}\), making the concept more tangible and easier to grasp.
Real-world Examples
The expression \(6 \sqrt{10}\) finds numerous applications in real-world scenarios, showcasing its practical utility beyond theoretical mathematics. Here are some detailed examples of how \(6 \sqrt{10}\) is used in various fields:
- Architecture and Engineering: In these fields, \(6 \sqrt{10}\) can be used to calculate structural dimensions and stresses. For example, when determining the length of a beam or the diagonal of a rectangular section, this expression helps ensure accurate measurements and safety standards.
- Physics: In physics, \(6 \sqrt{10}\) might appear in problems involving motion and force calculations. For instance, it can represent the magnitude of a vector, such as displacement or force, providing a precise value for computations in mechanics or electromagnetism.
- Computer Science: This expression can be relevant in algorithm complexity analysis or in graphics programming. When determining distances in coordinate systems or optimizing computational tasks, \(6 \sqrt{10}\) provides a useful benchmark.
- Finance: In financial modeling, \(6 \sqrt{10}\) might be used to calculate risk assessments or in the valuation of financial instruments. It aids in providing accurate estimations for investments and market analysis.
These examples highlight the versatility of \(6 \sqrt{10}\), demonstrating its importance across different disciplines. Understanding how to apply this mathematical expression in practical situations not only enhances problem-solving skills but also bridges the gap between theoretical concepts and real-world applications.

Common Mistakes to Avoid
When working with square roots, particularly expressions like \(6\sqrt{10}\), there are several common mistakes that students and practitioners should be aware of and avoid. Here are some of the key pitfalls and how to avoid them:
- Incorrect Addition and Subtraction:
Many students mistakenly believe that square roots can be added or subtracted directly, such as:
\(3\sqrt{10} + 2\sqrt{10} = 5\sqrt{10}\) is correct, but \( \sqrt{10} + \sqrt{10} \neq 2\sqrt{10} \). The correct approach is to treat like terms appropriately.
- Misapplication of the Distributive Property:
A common error is treating the square root function as linear. For example, \( \sqrt{a} + \sqrt{b} \neq \sqrt{a + b} \).
Always remember that:
- \(\sqrt{9 + 16} \neq \sqrt{9} + \sqrt{16}\) because \(5 \neq 3 + 4\).
- Errors in Simplification:
Missteps occur when simplifying products or quotients of square roots, such as:
\(\sqrt{a} \cdot \sqrt{b} = \sqrt{a \cdot b}\), but \(\sqrt{a} / \sqrt{b} = \sqrt{a/b}\).
- Squaring Mistakes:
When squaring terms, be careful to apply the exponent correctly:
\((4a)^2 = 16a^2\) not \(4a^2\). Similarly, \((\sqrt{a})^2 = a\).
- Incorrect Assumptions About Negative Numbers:
Square roots of negative numbers involve imaginary units, for instance, \( \sqrt{-1} = i \). Therefore, \(\sqrt{-a}\) should be treated as \(i\sqrt{a}\).
- Misunderstanding the Pythagorean Theorem:
Errors often arise in applying the Pythagorean theorem, especially when reversing implications:
For example, \(a^2 + b^2 = c^2\) correctly implies a right triangle, but incorrect assumptions about mixed inequalities can lead to mistakes.
By understanding and avoiding these common mistakes, students can improve their accuracy and confidence in working with square roots and related mathematical concepts.
Advanced Mathematical Contexts
The expression \(6\sqrt{10}\) appears in several advanced mathematical contexts, highlighting its importance and utility in various fields.
1. Geometry and Trigonometry
In geometry, the square root of 10 is often encountered in problems involving right triangles and Pythagorean triples. For instance, in a right triangle where the legs are of lengths 2 and \(3\sqrt{10}\), the hypotenuse can be calculated using the Pythagorean theorem:
\[
c = \sqrt{(2)^2 + (3\sqrt{10})^2} = \sqrt{4 + 90} = \sqrt{94}
\]
Furthermore, in trigonometry, expressions involving \(\sqrt{10}\) can be seen in exact trigonometric values for specific angles.
2. Algebra and Simplification
The term \(6\sqrt{10}\) can be simplified or used in algebraic manipulations. For example, when simplifying the expression \((6\sqrt{10})^2\), we get:
\[
(6\sqrt{10})^2 = 36 \cdot 10 = 360
\]
This result is useful in algebraic equations and inequalities.
3. Calculus and Limits
In calculus, \(\sqrt{10}\) might appear in limit problems, especially those involving square roots. For example:
\[
\lim_{{x \to \infty}} \frac{6\sqrt{10}x}{x^2 + 1}
\]
By simplifying, we get:
\[
\lim_{{x \to \infty}} \frac{6\sqrt{10}}{x + \frac{1}{x}} = 0
\]
4. Complex Numbers
In the realm of complex numbers, square roots play a crucial role. For instance, solving equations like \(z^2 = -10\) requires understanding the properties of square roots:
\[
z = \pm i\sqrt{10}
\]
5. Linear Algebra
In linear algebra, eigenvalues and eigenvectors often involve square roots. For a matrix with eigenvalues involving \(\sqrt{10}\), calculations can be greatly simplified using properties of square roots.
Conclusion
The expression \(6\sqrt{10}\) is a significant mathematical entity that appears in various advanced contexts, from geometry to linear algebra, showcasing its broad utility and importance in higher mathematics.
Conclusion and Summary
The mathematical expression 6√10 combines the scalar multiple of 6 with the square root of 10, representing a value that has both practical and theoretical significance in various fields such as geometry, physics, and engineering. Understanding this expression involves several key aspects:
- Mathematical Derivation: The value of 6√10 can be derived by recognizing the properties of square roots and applying them to the given expression. It simplifies to 6 × √10, and its decimal approximation is approximately 18.9737.
- Properties and Simplification: The square root function is essential in mathematics, providing a method to find a number that, when multiplied by itself, yields the original value. In this case, √10 is an irrational number, which means its decimal form is non-repeating and infinite.
- Applications: In geometry, 6√10 might represent a length, area, or other dimensional quantity that is crucial in solving problems involving right triangles, circles, and other shapes. In physics, it can be related to wave functions, quantum mechanics, and other phenomena requiring precise measurements and calculations. In engineering, the expression helps in structural analysis, electrical engineering, and other technical fields where accurate calculations are essential.
- Visualization and Approximation: While the exact value is represented in radical form, decimal approximation is often used for practical calculations. Visualization techniques such as plotting on a number line or graph can help in understanding the magnitude and implications of the value.
In summary, mastering the concept of 6√10 not only enhances one's mathematical skills but also broadens the understanding of its applications in real-world scenarios. By leveraging both exact and approximate forms, one can navigate complex problems with greater ease and accuracy, making 6√10 a vital component in various scientific and engineering disciplines.
Giới thiệu về Căn Bậc Hai của 10
READ MORE:
Căn Bậc Hai là gì? | Toán Học với Thầy J