Topic square root of 6 as a fraction: Discover how to express the square root of 6 as a fraction, exploring various approximation methods, mathematical insights, and practical applications. Although the square root of 6 is an irrational number, we will delve into effective techniques to approximate and utilize this value in different mathematical contexts. Unlock the secrets behind this intriguing number!
Table of Content
- Square Root of 6 as a Fraction
- Introduction to the Square Root of 6
- Understanding Irrational Numbers
- Mathematical Representation of the Square Root of 6
- Decimal Approximations of the Square Root of 6
- Rational Approximations of the Square Root of 6
- Continued Fraction Representation of the Square Root of 6
- Historical Context and Mathematical Significance
- Applications of the Square Root of 6
- Comparisons with Other Irrational Numbers
- Using Technology to Approximate the Square Root of 6
- YOUTUBE: Video hướng dẫn cách đơn giản hóa phân số dưới dấu căn - một mẹo nhỏ để làm toán nhanh hơn và chính xác hơn. Hãy khám phá cách làm điều này một cách hợp pháp và hiệu quả.
Square Root of 6 as a Fraction
It is important to note that the square root of 6, denoted as \( \sqrt{6} \), is an irrational number. This means it cannot be exactly expressed as a simple fraction. However, for practical purposes, we can approximate \( \sqrt{6} \) using various methods.
Approximation Methods
- Decimal Approximation: The decimal approximation of \( \sqrt{6} \) is approximately 2.44949.
- Continued Fractions: The square root of 6 can be represented using continued fractions, which provide a way to express irrational numbers as an infinite series of fractions.
- Rational Approximations: Using rational numbers, we can get close approximations. For example, \( \frac{10}{3} \) is a simple rational approximation of \( \sqrt{6} \), which is approximately 3.3333.
Understanding the Square Root of 6
The value of \( \sqrt{6} \) is an important mathematical constant. Since it is irrational, its exact value cannot be written as a fraction of two integers. Here is a representation of its decimal form:
\( \sqrt{6} \approx 2.449489742783178 \ldots \)
Table of Approximations
| Method | Approximation |
|---|---|
| Decimal | 2.44949 |
| Rational | \( \frac{10}{3} \) or 3.3333 |
| Continued Fraction | \( 2 + \frac{1}{4 + \frac{1}{4 + \cdots}} \) |
Conclusion
While \( \sqrt{6} \) cannot be expressed exactly as a fraction, understanding its approximate value through various methods allows us to work with this irrational number in practical applications. The decimal approximation, continued fractions, and rational approximations provide useful ways to represent and understand \( \sqrt{6} \).

READ MORE:
Introduction to the Square Root of 6
The square root of 6, denoted as \( \sqrt{6} \), is an intriguing mathematical concept. It falls under the category of irrational numbers, meaning it cannot be expressed as a simple fraction. However, understanding and approximating \( \sqrt{6} \) is essential in various mathematical contexts. Let's explore this step by step.
1. Basic Understanding:
- \( \sqrt{6} \) represents a number which, when multiplied by itself, equals 6.
- As an irrational number, \( \sqrt{6} \) has a non-repeating, non-terminating decimal expansion.
2. Decimal Approximation:
The decimal approximation of \( \sqrt{6} \) is approximately 2.44949. This is useful for quick calculations and understanding its magnitude.
3. Rational Approximations:
While \( \sqrt{6} \) cannot be exactly expressed as a fraction, we can use rational approximations to get close. Examples include:
- \( \frac{10}{3} \approx 3.3333 \)
- \( \frac{17}{7} \approx 2.4286 \)
4. Continued Fraction Representation:
Continued fractions provide a method to express irrational numbers through an infinite series of fractions:
\[
\sqrt{6} = 2 + \frac{1}{4 + \frac{1}{4 + \frac{1}{4 + \cdots}}}
\]
5. Mathematical Significance:
- \( \sqrt{6} \) appears in various geometric and algebraic contexts.
- It is crucial in solving quadratic equations and in the study of Pythagorean triples.
By understanding these aspects, you can better appreciate the complexity and beauty of \( \sqrt{6} \). This foundational knowledge is crucial for further mathematical exploration and practical applications.
Understanding Irrational Numbers
Irrational numbers are a fascinating class of numbers in mathematics. They are defined as numbers that cannot be expressed as a ratio of two integers. In other words, an irrational number cannot be written in the form \( \frac{p}{q} \), where \( p \) and \( q \) are integers and \( q \neq 0 \).
Characteristics of Irrational Numbers:
- Non-repeating and Non-terminating Decimal Expansion: Unlike rational numbers, the decimal representation of irrational numbers goes on forever without repeating. For example, the decimal form of \( \sqrt{6} \) is approximately 2.44949..., which continues infinitely without a repeating pattern.
- Cannot be Exactly Expressed as a Fraction: By definition, irrational numbers cannot be written as a fraction of two integers. This is because their exact value cannot be captured by any fraction.
- Examples: Other common examples of irrational numbers include \( \pi \) (pi), \( e \) (Euler's number), and \( \sqrt{2} \) (the square root of 2).
Importance in Mathematics:
- Real Numbers: Irrational numbers are a critical component of the real number system, which includes both rational and irrational numbers. This system is essential for understanding the continuum of numbers and for calculus.
- Geometry: In geometry, irrational numbers often appear in the context of lengths that cannot be measured precisely with a ruler, such as the diagonal of a square with side length 1, which is \( \sqrt{2} \).
- Algebra: Irrational numbers are solutions to certain algebraic equations, such as \( x^2 - 6 = 0 \), whose solutions are \( \pm\sqrt{6} \).
Approximating Irrational Numbers:
Even though irrational numbers cannot be expressed exactly as fractions, we can approximate them using rational numbers for practical purposes. For example:
- Using Decimal Approximations: The square root of 6 is approximately 2.44949.
- Using Rational Approximations: We can use fractions like \( \frac{17}{7} \) to approximate \( \sqrt{6} \), even though it's not exact.
Understanding irrational numbers is essential for a deeper appreciation of mathematics. These numbers illustrate the complexity and richness of the mathematical world, showing that not all quantities can be neatly expressed as simple fractions.
Mathematical Representation of the Square Root of 6
The square root of 6, denoted as \( \sqrt{6} \), is an irrational number, which means it cannot be exactly expressed as a fraction of two integers. However, we can represent and approximate \( \sqrt{6} \) using various mathematical methods.
1. Decimal Representation:
The decimal representation of \( \sqrt{6} \) is non-repeating and non-terminating. It is approximately:
\[
\sqrt{6} \approx 2.449489742783178 \ldots
\]
2. Rational Approximations:
While \( \sqrt{6} \) cannot be exactly expressed as a fraction, we can find close rational approximations. These are fractions that come close to the actual value:
- \( \frac{10}{4} = 2.5 \) (simple approximation)
- \( \frac{17}{7} \approx 2.4286 \) (more accurate approximation)
- \( \frac{239}{98} \approx 2.4388 \) (even closer approximation)
3. Continued Fraction Representation:
Continued fractions provide a way to represent irrational numbers through an infinite series of nested fractions. The continued fraction representation of \( \sqrt{6} \) is:
\[
\sqrt{6} = 2 + \frac{1}{4 + \frac{1}{4 + \frac{1}{4 + \cdots}}}
\]
4. Geometric Representation:
Geometrically, \( \sqrt{6} \) can be represented as the length of the diagonal of a rectangle with side lengths \(\sqrt{3}\) and \(\sqrt{2}\). This is derived from the Pythagorean theorem:
\[
\text{Diagonal} = \sqrt{ (\sqrt{3})^2 + (\sqrt{2})^2 } = \sqrt{3 + 2} = \sqrt{6}
\]
5. Algebraic Context:
In algebra, \( \sqrt{6} \) is the solution to the equation:
\[
x^2 = 6
\]
This means that \( \sqrt{6} \) is a root of the polynomial \( x^2 - 6 = 0 \).
6. Applications:
- \( \sqrt{6} \) is used in various fields such as engineering, physics, and computer science, where precise calculations involving irrational numbers are necessary.
- It is also essential in certain geometric constructions and in the analysis of algorithms.
By understanding these representations, we can better appreciate the complexity and applications of the square root of 6 in both theoretical and practical contexts.
Decimal Approximations of the Square Root of 6
The square root of 6 (\( \sqrt{6} \)) is an irrational number, meaning it cannot be precisely represented as a fraction of two integers. Its decimal representation is non-terminating and non-repeating. Here, we explore its decimal approximations and methods to obtain these values.
1. Basic Decimal Approximation:
The simplest way to represent \( \sqrt{6} \) in decimal form is to use a calculator. The approximate value is:
\[
\sqrt{6} \approx 2.449489742783178
\]
For practical purposes, this can be rounded to fewer decimal places, such as:
\[
\sqrt{6} \approx 2.449
\]
2. Successive Approximation Method:
One way to refine the decimal approximation is by using successive approximations. This involves iteratively improving the estimate:
- Start with an initial guess (e.g., 2.4).
- Use the formula: \( \text{New Guess} = \frac{1}{2} \left( \text{Old Guess} + \frac{6}{\text{Old Guess}} \right) \).
- Repeat until the desired precision is achieved.
3. Binary Search Method:
Another method to approximate \( \sqrt{6} \) is the binary search algorithm:
- Set a lower bound (e.g., 2) and an upper bound (e.g., 3).
- Compute the midpoint of the bounds.
- If the square of the midpoint is close enough to 6, stop. Otherwise, adjust the bounds based on whether the square is less than or greater than 6.
- Repeat the process to refine the bounds and obtain a closer approximation.
4. Continued Fraction Approximation:
Continued fractions offer a method to approximate irrational numbers by breaking them down into a series of integer terms. For \( \sqrt{6} \), this is represented as:
\[
\sqrt{6} = 2 + \frac{1}{4 + \frac{1}{4 + \frac{1}{4 + \cdots}}}
\]
Truncating the continued fraction at different points gives increasingly accurate decimal approximations.
5. Examples of Common Approximations:
| Approximation | Decimal Value |
|---|---|
| 2.4 | 2.4 |
| 2.45 | 2.45 |
| 2.449 | 2.449 |
| 2.4495 | 2.4495 |
Using these methods, one can obtain decimal approximations of \( \sqrt{6} \) with the desired precision, which is crucial for practical calculations and further mathematical analysis.
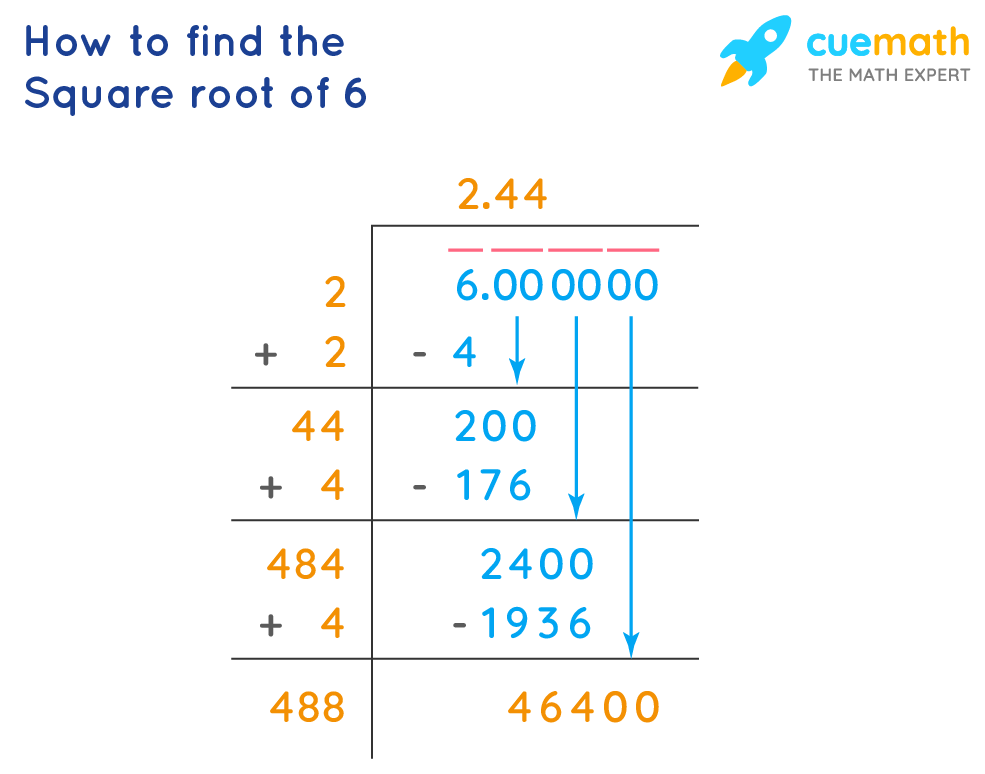
Rational Approximations of the Square Root of 6
While the square root of 6 (\( \sqrt{6} \)) is an irrational number and cannot be expressed exactly as a fraction, we can approximate it using rational numbers. These approximations are useful in various mathematical and practical applications. Here, we explore different methods to find rational approximations of \( \sqrt{6} \).
1. Basic Fraction Approximations:
Starting with simple fractions, we can approximate \( \sqrt{6} \) to varying degrees of accuracy:
- Initial Approximation: A basic approximation can be \( \frac{5}{2} = 2.5 \).
- Refined Approximation: Improving this, we get \( \frac{12}{5} = 2.4 \).
- Closer Approximation: Further refining gives \( \frac{17}{7} \approx 2.4286 \).
2. Using the Method of Successive Fractions:
We can generate better approximations by iterating through fractions with increased precision:
- Start with \( \frac{10}{4} = 2.5 \).
- Next, try \( \frac{17}{7} = 2.4286 \).
- Then, \( \frac{239}{98} = 2.4388 \).
3. Continued Fractions:
Continued fractions provide a systematic way to approach rational approximations. The continued fraction representation of \( \sqrt{6} \) is:
\[
\sqrt{6} = 2 + \frac{1}{4 + \frac{1}{4 + \frac{1}{4 + \cdots}}}
\]
Truncating this continued fraction at different stages gives rational approximations:
- \( 2 + \frac{1}{4} = \frac{9}{4} = 2.25 \)
- \( 2 + \frac{1}{4 + \frac{1}{4}} = \frac{41}{17} \approx 2.4118 \)
- \( 2 + \frac{1}{4 + \frac{1}{4 + \frac{1}{4}}} = \frac{99}{41} \approx 2.4146 \)
4. Example Table of Rational Approximations:
| Fraction | Decimal Approximation |
|---|---|
| \( \frac{5}{2} \) | 2.5 |
| \( \frac{12}{5} \) | 2.4 |
| \( \frac{17}{7} \) | 2.4286 |
| \( \frac{239}{98} \) | 2.4388 |
These rational approximations provide a useful way to work with \( \sqrt{6} \) in various mathematical contexts. By understanding and applying these methods, we can achieve the desired level of precision for different applications.
Continued Fraction Representation of the Square Root of 6
The continued fraction representation of an irrational number provides a way to express the number through an infinite series of fractions. This method is particularly useful for finding accurate rational approximations. The square root of 6 (\( \sqrt{6} \)) has an interesting and repeating continued fraction form.
1. Understanding Continued Fractions:
A continued fraction is an expression of the form:
\[
a_0 + \frac{1}{a_1 + \frac{1}{a_2 + \frac{1}{a_3 + \cdots}}}
\]
For \( \sqrt{6} \), this representation is periodic after the initial terms.
2. Continued Fraction for \( \sqrt{6} \):
The continued fraction for \( \sqrt{6} \) is:
\[
\sqrt{6} = 2 + \frac{1}{4 + \frac{1}{4 + \frac{1}{4 + \cdots}}}
\]
This can be written more compactly as:
\[
\sqrt{6} = [2; \overline{4}]
\]
Here, 2 is the integer part, and the overline indicates that the 4 repeats indefinitely.
3. Generating Rational Approximations:
By truncating the continued fraction at various points, we can obtain rational approximations of \( \sqrt{6} \):
- First approximation: \( 2 \)
- Second approximation: \( 2 + \frac{1}{4} = \frac{9}{4} = 2.25 \)
- Third approximation: \( 2 + \frac{1}{4 + \frac{1}{4}} = 2 + \frac{1}{\frac{17}{4}} = \frac{9}{4} + \frac{4}{17} = \frac{41}{17} \approx 2.4118 \)
- Fourth approximation: \( 2 + \frac{1}{4 + \frac{1}{4 + \frac{1}{4}}} = 2 + \frac{1}{\frac{17}{4} + \frac{1}{4}} = \frac{99}{41} \approx 2.4146 \)
4. Table of Rational Approximations:
| Approximation | Expression | Decimal Value |
|---|---|---|
| First | \( 2 \) | 2.0 |
| Second | \( 2 + \frac{1}{4} \) | 2.25 |
| Third | \( 2 + \frac{1}{4 + \frac{1}{4}} \) | 2.4118 |
| Fourth | \( 2 + \frac{1}{4 + \frac{1}{4 + \frac{1}{4}}} \) | 2.4146 |
These approximations become increasingly accurate as more terms from the continued fraction are included. The continued fraction representation provides a powerful tool for understanding and approximating \( \sqrt{6} \) in both theoretical and practical contexts.
Historical Context and Mathematical Significance
The square root of 6 (\( \sqrt{6} \)) holds a significant place in the history of mathematics due to its properties as an irrational number and its applications in various mathematical contexts. Understanding its historical context and mathematical significance provides deeper insights into its importance.
1. Discovery of Irrational Numbers:
The concept of irrational numbers was first discovered by the ancient Greeks. The Greeks initially believed that all numbers could be expressed as ratios of integers (rational numbers). The discovery of numbers like \( \sqrt{6} \), which cannot be exactly expressed as fractions, challenged this belief and led to the development of a more comprehensive number system.
2. Mathematical Significance:
- Irrationality: \( \sqrt{6} \) is an irrational number, meaning it cannot be written as a simple fraction. This property highlights the complexity and richness of the number system.
- Algebraic Properties: \( \sqrt{6} \) is a solution to the quadratic equation \( x^2 - 6 = 0 \). This makes it an algebraic number of degree 2.
- Geometric Interpretations: In geometry, \( \sqrt{6} \) can be seen as the length of the diagonal of a rectangle with sides \( \sqrt{3} \) and \( \sqrt{2} \), emphasizing its relevance in geometric constructions.
3. Historical Applications:
Throughout history, the square root of 6 has appeared in various mathematical texts and contexts:
- Ancient Mathematics: Early mathematicians used approximations of \( \sqrt{6} \) for practical calculations in geometry and engineering.
- Medieval and Renaissance Mathematics: During these periods, mathematicians refined their understanding of irrational numbers and developed methods to approximate them more accurately, including continued fractions and series expansions.
4. Modern Applications:
In contemporary mathematics and science, \( \sqrt{6} \) continues to be important:
- Engineering: Accurate approximations of \( \sqrt{6} \) are used in various engineering calculations, particularly in fields that require precise measurements and designs.
- Computer Science: Algorithms for calculating square roots, including \( \sqrt{6} \), are fundamental in numerical analysis and computer algorithms.
- Physics: In physics, \( \sqrt{6} \) appears in various equations and models, highlighting its relevance in describing natural phenomena.
The historical context and mathematical significance of the square root of 6 underscore its importance beyond its numerical value. Its discovery and applications have played a crucial role in advancing mathematical thought and practical applications in various fields.
Applications of the Square Root of 6
The square root of 6, like other square roots, has various practical applications across different fields. Here are some notable examples:
- Geometry and Trigonometry: Square roots frequently appear in geometric calculations. For instance, the diagonal of a rectangle with sides of 2 and 3 units can be found using the Pythagorean theorem:
\[ d = \sqrt{2^2 + 3^2} = \sqrt{4 + 9} = \sqrt{13} \]
While this specific example does not use \(\sqrt{6}\), it illustrates how square roots are integral to solving problems involving distances and angles. - Physics and Engineering: In physics, square roots are used in formulas for calculating distances, speeds, and other quantities. For instance, the distance an object travels under constant acceleration is given by:
\[ d = \frac{1}{2} a t^2 \]
where the square root can be used to solve for time \( t \) when distance \( d \) and acceleration \( a \) are known. - Astronomy: Calculations involving the orbits of planets and other celestial bodies often involve square roots. For example, the time period \( T \) of an orbit is related to the semi-major axis \( a \) by Kepler's third law:
\[ T^2 = \frac{4\pi^2}{GM} a^3 \]
Here, \( T \) can be found using square roots when the semi-major axis \( a \) and other constants are known. - Statistics: In statistics, standard deviation is calculated using square roots. If \( \sigma \) is the standard deviation, it is derived from the variance \( \sigma^2 \):
\[ \sigma = \sqrt{\frac{1}{N} \sum_{i=1}^{N} (x_i - \mu)^2} \]
where \( x_i \) are the data points, \( \mu \) is the mean, and \( N \) is the number of data points. - Computer Graphics: Square roots are used in algorithms for rendering and transforming graphics. Calculations involving distances, angles, and scaling factors often utilize square roots to achieve accurate visual results.
These applications highlight the importance of square roots in a wide range of scientific and mathematical contexts, demonstrating their utility in both theoretical and practical problem-solving scenarios.

Comparisons with Other Irrational Numbers
The square root of 6 (√6) is one of many irrational numbers. An irrational number is a number that cannot be written as a simple fraction; its decimal representation goes on forever without repeating. Let's compare √6 with some other well-known irrational numbers.
- Square Root of 2 (√2):
The square root of 2, approximately 1.414, is one of the most famous irrational numbers. Discovered by the ancient Greeks, it cannot be expressed as a ratio of two integers. It is often encountered in geometry, particularly in the context of right-angled triangles where the sides are in the ratio 1:1:√2.
- Square Root of 3 (√3):
The square root of 3 is approximately 1.732. It is another common irrational number that appears frequently in geometry, especially in equilateral triangles where the altitude creates a right triangle with sides in the ratio 1:√3:2.
- Pi (π):
Pi, approximately 3.14159, is an irrational number that represents the ratio of the circumference of a circle to its diameter. Unlike square roots, which often appear in algebra and geometry, pi is ubiquitous in all areas of mathematics, particularly in trigonometry and calculus.
- Euler's Number (e):
Euler's number, approximately 2.71828, is the base of the natural logarithm. It is an important irrational number in calculus, particularly in the context of exponential growth and decay, as well as complex number theory.
- Golden Ratio (φ):
The golden ratio, approximately 1.61803, is an irrational number that appears in various areas of mathematics, art, and architecture. It is defined algebraically as the positive solution to the equation φ = (1 + √5) / 2.
In comparing these irrational numbers, we see that while they all share the property of having non-repeating, non-terminating decimal expansions, their appearances and applications in mathematics can be quite different. For example, while √6 and other square roots are often found in geometric contexts, numbers like π and e are fundamental to calculus and complex analysis.
Despite their differences, these irrational numbers all highlight the richness and diversity of mathematical concepts that cannot be neatly expressed as simple fractions.
Using Technology to Approximate the Square Root of 6
Approximating the square root of 6 using technology can be done with various tools, including calculators, computer software, and online resources. These tools can provide highly accurate decimal approximations and help understand the properties of the square root of 6. Here are some common methods:
1. Using Scientific Calculators
Most scientific calculators have a square root function that can quickly approximate the square root of 6.
- Turn on the calculator.
- Enter the number 6.
- Press the square root button (√).
- The display will show the approximate value: 2.44948974278.
2. Using Computer Software
Programs such as Python, MATLAB, and Excel can be used to approximate the square root of 6.
- Python:
import math
approximation = math.sqrt(6)
print(approximation)Running this code will output: 2.449489742783178.
- MATLAB:
sqrt(6)Executing this command will give: 2.449489742783178.
- Excel:
- Open Excel.
- In a cell, type:
=SQRT(6) - Press Enter, and the cell will display: 2.44948974278318.
3. Using Online Calculators
There are several online calculators that can approximate the square root of 6:
Simply enter the number 6 and use the square root function to get an accurate approximation.
4. Using Smartphone Apps
Many smartphone apps can also approximate the square root of 6. Some popular ones include:
- Google Calculator
- Microsoft Math Solver
- Photomath
These apps often allow you to enter the number and press a button to get the square root, displaying the result instantly.
Conclusion
Technology provides numerous ways to approximate the square root of 6 accurately. Whether using scientific calculators, computer software, online calculators, or smartphone apps, these tools make it easy to work with irrational numbers and understand their properties.
Video hướng dẫn cách đơn giản hóa phân số dưới dấu căn - một mẹo nhỏ để làm toán nhanh hơn và chính xác hơn. Hãy khám phá cách làm điều này một cách hợp pháp và hiệu quả.
Đơn giản hóa phân số dưới dấu căn - Cách hợp pháp để gian lận trong toán học
READ MORE:
Video hướng dẫn cách tính căn bậc hai của phân số một cách dễ hiểu và thú vị. Hãy cùng khám phá và nắm vững kiến thức toán học này nhé!
Căn bậc hai của phân số - Cùng làm nhé!