3 Square Root 9: Comprehensive Guide to Understanding and Applying

The expression 3 × √9 involves multiplication and the square root operation. Let's break it down step by step:

Step-by-Step Calculation

• First, identify the square root of 9.
• The square root of 9 is 3 because 3 × 3 = 9.
• Next, multiply the result by 3.
• So, 3 × 3 = 9.

Therefore, the value of 3 × √9 is:

$$

3
×

9

=
9

General Rule for Square Roots

The square root of a number is a value that, when multiplied by itself, gives the original number. Mathematically, it is represented as:

$$


x

×

x

=
x

Example Calculations

Here are a few more examples to illustrate how square roots and multiplications work:

• 2 × √4 = 2 × 2 = 4
• 4 × √16 = 4 × 4 = 16
• 5 × √25 = 5 × 5 = 25

Using MathJax for Mathematical Expressions

MathJax is a JavaScript display engine that produces high-quality mathematical typesetting in web pages. Here’s how you can represent mathematical expressions using MathJax:

  $3\times \sqrt{9}=9$
  

This allows for clear and accurate representation of mathematical formulas.

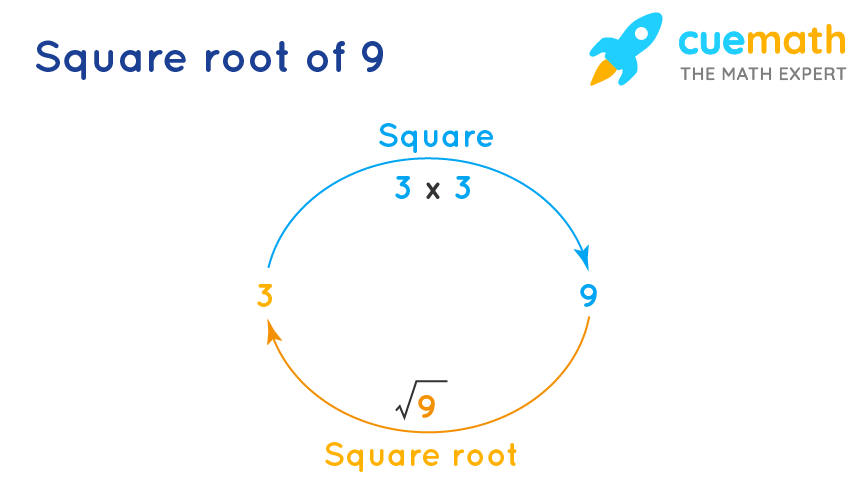
Square roots are a fundamental concept in mathematics that provide a way to find the side length of a square whose area matches a given value. Specifically, the square root of a number \( x \), denoted as \( \sqrt{x} \), is a value that, when multiplied by itself, gives \( x \). For example, \( \sqrt{9} \) equals 3 because \( 3 \times 3 = 9 \).

In the expression \( 3 \times \sqrt{9} \), we are multiplying the number 3 by the square root of 9. This can be interpreted as taking three times the side length of a square whose area is 9 square units, resulting in 9 units of linear measurement.

Understanding square roots is crucial in various fields such as geometry, physics, engineering, and even everyday calculations. It allows us to determine dimensions, solve equations involving areas and volumes, and comprehend complex mathematical functions.

When we encounter the expression \( 3 \times \sqrt{9} \), it represents a mathematical operation involving multiplication and a square root. Here's how we interpret and compute it:

1. We start with the square root of 9. The square root of a number \( x \), denoted as \( \sqrt{x} \), is the value that, when multiplied by itself, yields \( x \). Therefore, \( \sqrt{9} = 3 \) because \( 3 \times 3 = 9 \).
2. Next, we multiply this result by 3. So, \( 3 \times \sqrt{9} = 3 \times 3 = 9 \).

This expression simplifies to 9. It showcases the basic arithmetic operations involving multiplication and square roots, which are foundational in mathematics and applicable in various real-world contexts.

Square roots are a fundamental concept in mathematics, particularly in algebra and geometry. Here are some important properties:

1. Definition: The square root of a number \( a \), denoted as \( \sqrt{a} \), is a value \( x \) such that \( x^2 = a \) and \( x \geq 0 \).
2. Non-negative Result: The square root of a non-negative real number is always non-negative. For example, \( \sqrt{9} = 3 \) and \( \sqrt{0} = 0 \).
3. Multiplication Property: For any non-negative real numbers \( a \) and \( b \), \( \sqrt{ab} = \sqrt{a} \times \sqrt{b} \).
4. Power Rule: \( (\sqrt{a})^n = \sqrt{a^n} \) for any non-negative real number \( a \) and positive integer \( n \).
5. Radical Simplification: The square root expression can often be simplified by factoring out perfect squares from under the radical.
6. Relationship to Squares: The square root function is the inverse of the squaring function, meaning \( (\sqrt{a})^2 = a \).

These properties are foundational in solving equations, simplifying expressions, and understanding geometric relationships involving lengths and areas.

To calculate \( 3 \times \sqrt{9} \), follow these steps:

1. Identify that \( \sqrt{9} \) equals 3, because \( 3^2 = 9 \).
2. Multiply 3 by the result of \( \sqrt{9} \):

	\( 3 \times \sqrt{9} = 3 \times 3 \)		\( = 9 \)

Therefore, \( 3 \times \sqrt{9} = 9 \).

Square roots play a crucial role in various real-world applications across different fields. Understanding how to calculate and use square roots can be immensely beneficial in solving practical problems. Below are some of the significant applications of square roots:

• 1. Architecture and Construction

  Architects and engineers use square roots to determine the dimensions of various structures. For example, to ensure the stability and balance of buildings, the Pythagorean theorem, which involves square roots, is applied to calculate the lengths of sides in right-angled triangles.

  Example: Calculating the length of a diagonal in a rectangular room.

  If a room has a length of 6 meters and a width of 8 meters, the diagonal (d) can be found using the formula:

  \[
  d = \sqrt{6^2 + 8^2} = \sqrt{36 + 64} = \sqrt{100} = 10 \text{ meters}
  \]

• 2. Finance and Economics

  In finance, square roots are used to calculate the standard deviation of investment returns, which helps in assessing the risk associated with different investments.

  Example: Finding the standard deviation of a set of returns.

  If the returns are 4%, 6%, 8%, and 10%, the standard deviation (σ) is calculated as:

  \[
  \sigma = \sqrt{\frac{(4-7)^2 + (6-7)^2 + (8-7)^2 + (10-7)^2}{4}}
  \]

• 3. Physics

  Square roots are frequently used in physics, particularly in equations involving areas and volumes, such as calculating the period of a pendulum or the energy levels in quantum mechanics.

  Example: Calculating the period of a simple pendulum.

  The period (T) of a pendulum is given by:

  \[
  T = 2\pi \sqrt{\frac{L}{g}}
  \]

  where L is the length of the pendulum and g is the acceleration due to gravity.

• 4. Medicine

  In medical imaging, square roots are used to process and interpret data from various scanning technologies such as MRI and CT scans, helping to create clear and accurate images of the human body.

  Example: Enhancing image quality in MRI scans by calculating the square root of intensity values.

• 5. Computer Graphics

  Square roots are essential in computer graphics for rendering images, calculating distances between points, and applying transformations.

  Example: Calculating the Euclidean distance between two points in 3D space.

  If the coordinates of two points are (x1, y1, z1) and (x2, y2, z2), the distance (d) is given by:

  \[
  d = \sqrt{(x2 - x1)^2 + (y2 - y1)^2 + (z2 - z1)^2}
  \]

These examples illustrate the diverse and practical applications of square roots in everyday life, highlighting their importance in various fields and the need for a solid understanding of this mathematical concept.

Understanding how to work with square roots through examples and practice problems can help solidify your knowledge and improve your problem-solving skills. Below are detailed examples and practice problems involving square roots, including the expression \( 3 \times \sqrt{9} \).

Example 1: Basic Calculation

Let's start with a simple calculation involving the expression \( 3 \times \sqrt{9} \).

1. First, find the square root of 9:

   \[
   \sqrt{9} = 3
   \]

2. Next, multiply the result by 3:

   \[
   3 \times 3 = 9
   \]

3. Therefore, \( 3 \times \sqrt{9} = 9 \).

Example 2: Solving a Quadratic Equation

Solve the quadratic equation \( x^2 = 25 \).

1. Take the square root of both sides of the equation:

   \[
   x = \pm \sqrt{25}
   \]

2. Simplify the square root:

   \[
   x = \pm 5
   \]

3. The solutions to the equation are \( x = 5 \) and \( x = -5 \).

Example 3: Finding the Length of a Side in a Right Triangle

Given a right triangle with legs of lengths 6 and 8, find the length of the hypotenuse.

1. Use the Pythagorean theorem:

   \[
   c = \sqrt{a^2 + b^2}
   \]

2. Substitute the given values:

   \[
   c = \sqrt{6^2 + 8^2} = \sqrt{36 + 64} = \sqrt{100} = 10
   \]

3. The length of the hypotenuse is 10.

Practice Problems

• Problem 1:

  Simplify \( 2 \times \sqrt{16} \).

  Solution: \( 2 \times 4 = 8 \).

• Problem 2:

  Solve the equation \( x^2 = 49 \).

  Solution: \( x = \pm 7 \).

• Problem 3:

  Find the square root of 81.

  Solution: \( \sqrt{81} = 9 \).

• Problem 4:

  Given a right triangle with legs of lengths 5 and 12, find the length of the hypotenuse.

  Solution: \( c = \sqrt{5^2 + 12^2} = \sqrt{25 + 144} = \sqrt{169} = 13 \).

• Problem 5:

  Simplify \( 4 \times \sqrt{25} \).

  Solution: \( 4 \times 5 = 20 \).

These examples and practice problems are designed to enhance your understanding of square roots and their applications. Practice regularly to master these concepts.

Square roots can sometimes be tricky, and it's easy to make mistakes when working with them. Here are some common mistakes to watch out for, along with tips on how to avoid them:

• 1. Incorrect Simplification of Square Roots

  One common mistake is simplifying square roots incorrectly. For instance, assuming that the square root of a sum is the sum of the square roots:

  \[
  \sqrt{a + b} \neq \sqrt{a} + \sqrt{b}
  \]

  For example, \(\sqrt{9 + 16} \neq \sqrt{9} + \sqrt{16}\).

  Instead, calculate each square root separately and then perform the addition or subtraction.

• 2. Forgetting to Consider Both Positive and Negative Roots

  When solving equations that involve square roots, remember that both the positive and negative roots are solutions:

  \[
  x^2 = 25 \implies x = \pm 5
  \]

  Always consider both the positive and negative solutions when dealing with square roots in equations.

• 3. Misinterpreting the Square Root Symbol

  Another common mistake is misinterpreting the square root symbol, \(\sqrt{}\), as a regular number or operation.

  For example, \(\sqrt{9} = 3\), not \(9\).

  Always remember that the square root of a number is a value that, when multiplied by itself, gives the original number.

• 4. Ignoring the Order of Operations

  When expressions involve square roots, it's crucial to follow the correct order of operations (PEMDAS/BODMAS).

  For example, in the expression \(3 \times \sqrt{9} + 2\), calculate the square root first, then multiply by 3, and finally add 2:

  \[
  3 \times \sqrt{9} + 2 = 3 \times 3 + 2 = 9 + 2 = 11
  \]

• 5. Confusing Squaring with Taking the Square Root

  Sometimes, students confuse squaring a number with taking the square root. Remember, squaring a number and taking the square root are inverse operations:

  \[
  (\sqrt{a})^2 = a \quad \text{and} \quad \sqrt{a^2} = |a|
  \]

  For example, \(\sqrt{3^2} = 3\) and \((\sqrt{9})^2 = 9\).

By being mindful of these common mistakes and following the proper steps, you can improve your accuracy when working with square roots and avoid potential errors.

Square roots are fundamental in mathematics, but they also lead to more advanced concepts that extend their utility and applications. Here are some advanced topics related to square roots:

• 1. Complex Numbers and Imaginary Units

  Square roots are not limited to positive numbers. The concept extends to complex numbers using the imaginary unit \(i\), where \(i\) is defined as the square root of -1:

  \[
  i = \sqrt{-1}
  \]

  Using this definition, the square roots of negative numbers can be expressed in terms of \(i\). For example:

  \[
  \sqrt{-9} = \sqrt{9 \times (-1)} = \sqrt{9} \times \sqrt{-1} = 3i
  \]

• 2. Higher-Order Roots

  While square roots are second-order roots, the concept generalizes to higher-order roots, such as cube roots and fourth roots. For example, the cube root of \(a\) is written as:

  \[
  \sqrt[3]{a}
  \]

  and it represents a number that, when cubed, equals \(a\).

• 3. The Square Root Function and Its Properties

  The square root function \(f(x) = \sqrt{x}\) has unique properties and characteristics. It is defined for all non-negative real numbers and is a monotonically increasing function. The graph of \(f(x) = \sqrt{x}\) is a half-parabola opening to the right.

• 4. Solving Equations Involving Square Roots

  Equations involving square roots can be solved using various techniques. For example, to solve an equation like \( \sqrt{x + 3} = 5 \), follow these steps:

  1. Square both sides to eliminate the square root:

     \[
     (\sqrt{x + 3})^2 = 5^2 \implies x + 3 = 25
     \]

  2. Solve the resulting linear equation:

     \[
     x + 3 = 25 \implies x = 22
     \]

• 5. Rationalizing the Denominator

  Rationalizing the denominator involves removing square roots from the denominator of a fraction. For example, to rationalize the denominator of \(\frac{1}{\sqrt{2}}\), multiply both the numerator and the denominator by \(\sqrt{2}\):

  \[
  \frac{1}{\sqrt{2}} \times \frac{\sqrt{2}}{\sqrt{2}} = \frac{\sqrt{2}}{2}
  \]

• 6. Applications in Calculus

  Square roots appear in various calculus problems, including limits, derivatives, and integrals. For instance, to find the derivative of \(f(x) = \sqrt{x}\), use the chain rule:

  \[
  f'(x) = \frac{d}{dx} (x^{1/2}) = \frac{1}{2} x^{-1/2} = \frac{1}{2\sqrt{x}}
  \]

• 7. Numerical Methods for Approximating Square Roots

  When an exact value is difficult to obtain, numerical methods such as the Newton-Raphson method can approximate square roots. For example, to approximate \(\sqrt{a}\), the iterative formula is:

  \[
  x_{n+1} = \frac{1}{2} \left( x_n + \frac{a}{x_n} \right)
  \]

  This method converges rapidly to the true value of \(\sqrt{a}\) with a good initial guess.

Exploring these advanced concepts related to square roots can deepen your understanding and reveal their broader applications in mathematics and science.

Technology has made it easier than ever to compute square roots accurately and efficiently. Various tools and software can help you calculate square roots quickly, whether for simple or complex calculations. Here are some ways to use technology to compute square roots:

• 1. Scientific Calculators

  Most scientific calculators have a dedicated square root button (\(\sqrt{\ }\)) that allows you to compute the square root of a number directly. For example, to find the square root of 9:

  1. Turn on the calculator.
  2. Press the \(\sqrt{\ }\) button.
  3. Enter 9 and press the equals (=) button.
  4. The display will show 3, indicating that \(\sqrt{9} = 3\).

• 2. Graphing Calculators

  Graphing calculators, like the TI-84, provide advanced functions, including square roots. To calculate \(3 \times \sqrt{9}\) using a graphing calculator:

  1. Turn on the calculator.
  2. Enter 3.
  3. Press the multiplication (\(\times\)) button.
  4. Press the \(\sqrt{\ }\) button.
  5. Enter 9 and press the equals (=) button.
  6. The display will show 9, confirming that \(3 \times \sqrt{9} = 9\).

• 3. Online Calculators

  There are numerous online calculators available for computing square roots. Websites like WolframAlpha, Calculator.net, and Mathway offer free tools. To use an online calculator:

  1. Open your web browser and go to the online calculator website.
  2. Enter the expression \(3 \times \sqrt{9}\) into the input field.
  3. Click the calculate or equals button.
  4. The result, 9, will be displayed, confirming \(3 \times \sqrt{9} = 9\).

• 4. Spreadsheet Software

  Spreadsheet software like Microsoft Excel, Google Sheets, and LibreOffice Calc can also compute square roots. To calculate \(3 \times \sqrt{9}\) in Excel:

  1. Open Excel and select a cell.
  2. Type the formula =3*SQRT(9) into the cell.
  3. Press Enter.
  4. The cell will display 9, showing that \(3 \times \sqrt{9} = 9\).

• 5. Programming Languages

  Programming languages like Python, JavaScript, and MATLAB provide functions to compute square roots. For example, to calculate \(3 \times \sqrt{9}\) in Python:

  1. Open your Python IDE or a Jupyter notebook.
  2. Type the following code:

     import math
     result = 3 * math.sqrt(9)
     print(result)

  3. Run the code.
  4. The output will be 9, indicating that \(3 \times \sqrt{9} = 9\).

By leveraging these technological tools, you can easily and accurately compute square roots, saving time and reducing the risk of errors in your calculations.

Understanding square roots is a fundamental aspect of mathematics that extends to various advanced concepts and practical applications. Throughout this guide, we have explored the basic definition and properties of square roots, the specific calculation of \( 3 \times \sqrt{9} \), and how to avoid common mistakes when working with square roots. Additionally, we have delved into advanced topics and the use of technology to compute square roots.

• Basic Concepts

  Square roots represent a number that, when multiplied by itself, gives the original number. For example, \( \sqrt{9} = 3 \) because \( 3 \times 3 = 9 \). The expression \( 3 \times \sqrt{9} \) simplifies to \( 3 \times 3 = 9 \).

• Common Mistakes

  We identified several common mistakes, such as incorrectly simplifying square roots, ignoring the order of operations, and confusing squaring with taking the square root. By understanding and avoiding these errors, you can improve your mathematical accuracy.

• Advanced Concepts

  Advanced topics related to square roots include complex numbers, higher-order roots, the properties of the square root function, solving equations, rationalizing the denominator, and applications in calculus. These concepts extend the utility of square roots beyond basic arithmetic.

• Using Technology

  Various technological tools, such as scientific and graphing calculators, online calculators, spreadsheet software, and programming languages, make computing square roots easier and more efficient. These tools help save time and reduce the risk of errors.

By mastering the fundamentals of square roots and exploring their advanced applications, you can enhance your mathematical skills and apply these concepts effectively in both academic and real-world scenarios. Keep practicing and using technology to aid your understanding, and you'll find that working with square roots becomes increasingly intuitive and straightforward.