Topic x squared - 1: The expression "x squared - 1" is a fundamental concept in algebra with applications in various mathematical problems. This article will explore its significance, methods of factorization, and practical examples to enhance understanding. Learn how to solve equations involving this expression and see its use in real-world scenarios.
Table of Content
- Understanding and Solving x2 - 1
- Introduction to "x squared - 1"
- Basic Concepts
- Solving Quadratic Equations
- Difference of Squares Formula
- Factoring x^2 - 1
- Completing the Square
- Applications in Algebra
- Graphical Representations
- Common Mistakes and Misconceptions
- Practical Examples and Exercises
- YOUTUBE: Video khám phá liệu phương trình 1^x có thể bằng 2 hay không, dành cho những ai yêu thích toán học và muốn tìm hiểu sâu hơn.
Understanding and Solving x2 - 1
The expression x2 - 1 is a fundamental algebraic expression that can be factored and solved using various methods. Below, we explore different aspects of this expression, its properties, and methods to solve or manipulate it.
Factoring x2 - 1
The expression x2 - 1 is a difference of squares, which can be factored as follows:
\[ x^2 - 1 = (x + 1)(x - 1) \]
This factorization is derived from the formula for the difference of squares: \[ a^2 - b^2 = (a + b)(a - b) \]. Here, \( a = x \) and \( b = 1 \).
Solving the Equation x2 - 1 = 0
To find the roots of the equation x2 - 1 = 0, we set the factored form equal to zero:
\[ (x + 1)(x - 1) = 0 \]
This gives us two solutions:
- \( x + 1 = 0 \implies x = -1 \)
- \( x - 1 = 0 \implies x = 1 \)
Graphing x2 - 1
The graph of the function y = x2 - 1 is a parabola that opens upwards with its vertex at (0, -1). The x-intercepts, also known as the roots, are at \( x = 1 \) and \( x = -1 \).

Applications
The expression x2 - 1 appears in various mathematical contexts, including algebraic manipulations, calculus, and solving real-world problems involving quadratic equations. Understanding how to factor and solve such expressions is crucial in many areas of mathematics and science.
Example Problem
Factor and solve the following equation:
\[ x^2 - 4 = 0 \]
Solution:
- Recognize it as a difference of squares: \[ x^2 - 4 = (x + 2)(x - 2) \]
- Set each factor to zero and solve for \( x \):
- \( x + 2 = 0 \implies x = -2 \)
- \( x - 2 = 0 \implies x = 2 \)
- The solutions are \( x = -2 \) and \( x = 2 \).
By understanding the structure and properties of quadratic expressions like x2 - 1, solving related equations becomes more intuitive and straightforward.
Further Learning Resources

READ MORE:
Introduction to "x squared - 1"
The expression \(x^2 - 1\) is a fundamental concept in algebra and mathematics. It represents a quadratic expression that can be factored using the difference of squares method. Understanding this expression and its applications is essential for solving various mathematical problems.
- \(x^2 - 1\) is a polynomial that can be expressed as the difference of two squares.
- Using the formula \(a^2 - b^2 = (a - b)(a + b)\), we can factor \(x^2 - 1\) into \((x - 1)(x + 1)\).
- This factorization is useful in simplifying equations and solving for variable values.
- The expression also appears in various algebraic contexts, such as solving quadratic equations, completing the square, and in calculus.
- Practical applications include geometry problems, physics equations, and optimization problems.
By mastering the manipulation and understanding of \(x^2 - 1\), students and professionals can enhance their mathematical problem-solving skills and apply them to a wide range of disciplines.
Basic Concepts
The expression \(x^2 - 1\) is a fundamental concept in algebra, often encountered in polynomial equations and algebraic factoring. Understanding this expression involves several key principles:
- Polynomial Representation: The term \(x^2 - 1\) is a polynomial of degree 2. It can be expressed in the standard form \(ax^2 + bx + c\), where \(a = 1\), \(b = 0\), and \(c = -1\).
- Difference of Squares: The expression \(x^2 - 1\) is a classic example of the difference of squares. It can be factored into \((x + 1)(x - 1)\), using the formula \(a^2 - b^2 = (a + b)(a - b)\), where \(a = x\) and \(b = 1\).
- Roots of the Equation: Solving \(x^2 - 1 = 0\) yields the roots \(x = 1\) and \(x = -1\). These are the points where the polynomial intersects the x-axis.
- Graphical Interpretation: The graph of the function \(y = x^2 - 1\) is a parabola that opens upwards with its vertex at \((0, -1)\). The roots \(x = 1\) and \(x = -1\) are the points where the parabola crosses the x-axis.
- Applications: The difference of squares is widely used in simplifying algebraic expressions, solving quadratic equations, and in calculus for finding limits and integrals involving polynomial functions.
| Expression | Factorization | Roots | Graph Shape |
|---|---|---|---|
| \(x^2 - 1\) | \((x + 1)(x - 1)\) | 1, -1 | Parabola opening upwards |
By mastering these concepts, students can effectively tackle a wide range of mathematical problems involving polynomials and quadratic equations.
Solving Quadratic Equations
Solving quadratic equations involves finding the values of \( x \) that satisfy the equation \( ax^2 + bx + c = 0 \). This can be achieved through various methods, each with its own steps and applications. Here, we will explore these methods in detail.
Methods of Solving Quadratic Equations
- Factoring
- Using the Quadratic Formula
- Completing the Square
- Graphing
Factoring
To factor a quadratic equation, we rewrite it in the form \( (x + p)(x + q) = 0 \). The solutions are then \( x = -p \) and \( x = -q \). Here is a step-by-step process:
- Rewrite the quadratic equation in standard form \( ax^2 + bx + c = 0 \).
- Find two numbers that multiply to \( ac \) and add to \( b \).
- Rewrite the middle term using these two numbers.
- Factor by grouping.
- Set each factor to zero and solve for \( x \).
Using the Quadratic Formula
The quadratic formula is a universal method for solving any quadratic equation. The formula is given by:
\[
x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}
\]
Steps to use the quadratic formula:
- Identify the coefficients \( a \), \( b \), and \( c \) in the quadratic equation \( ax^2 + bx + c = 0 \).
- Calculate the discriminant \( \Delta = b^2 - 4ac \).
- Determine the number of solutions based on the discriminant:
- If \( \Delta > 0 \), there are two distinct real solutions.
- If \( \Delta = 0 \), there is one real solution.
- If \( \Delta < 0 \), there are two complex solutions.
- Substitute \( a \), \( b \), and \( c \) into the formula and solve for \( x \).
Completing the Square
This method involves transforming the quadratic equation into a perfect square trinomial. Here are the steps:
- Rewrite the equation in the form \( ax^2 + bx = -c \).
- Divide all terms by \( a \) (if \( a \neq 1 \)).
- Add and subtract \( \left(\frac{b}{2a}\right)^2 \) inside the equation.
- Factor the perfect square trinomial.
- Solve for \( x \) by taking the square root of both sides.
Graphing
Graphing the quadratic equation \( y = ax^2 + bx + c \) provides a visual method of finding the roots. The points where the graph intersects the x-axis are the solutions to the equation.
Steps to solve by graphing:
- Plot the quadratic function on a graph.
- Identify the x-intercepts (if any).
- The x-values of the intercepts are the solutions to the equation.
Difference of Squares Formula
The difference of squares formula is a fundamental algebraic identity used to simplify expressions and solve equations. It states that any expression of the form \( a^2 - b^2 \) can be factored into \( (a + b)(a - b) \). This identity is crucial for solving quadratic equations, simplifying algebraic expressions, and understanding polynomial behavior.
Here is a step-by-step explanation of the formula:
- Start with the expression \( a^2 - b^2 \).
- Recognize that it can be rewritten as \( (a)^2 - (b)^2 \).
- Apply the difference of squares formula: \( a^2 - b^2 = (a + b)(a - b) \).
This formula can be applied in various contexts:
- Factorization: For example, \( x^2 - 1 \) can be factored as \( (x + 1)(x - 1) \).
- Simplifying Rational Expressions: Rational expressions involving squares can be simplified using this formula.
- Solving Equations: Equations of the form \( a^2 - b^2 = 0 \) can be easily solved by factoring and setting each factor to zero.
| Example | Factorization |
|---|---|
| \( x^2 - 16 \) | \( (x + 4)(x - 4) \) |
| \( 4x^2 - 25 \) | \( (2x + 5)(2x - 5) \) |
| \( x^4 - 1 \) | \( (x^2 + 1)(x^2 - 1) \) |
The difference of squares formula is versatile and appears frequently in algebra, making it an essential tool for students and mathematicians alike.
Factoring x^2 - 1
Factoring the expression \(x^2 - 1\) is a straightforward application of the difference of squares formula. The difference of squares states that \(a^2 - b^2 = (a + b)(a - b)\). In this case, \(a\) is \(x\) and \(b\) is 1. Here's a detailed, step-by-step breakdown of how to factor \(x^2 - 1\).
- Identify the difference of squares: \(x^2 - 1\) can be written as \((x)^2 - (1)^2\).
- Apply the difference of squares formula: \((x)^2 - (1)^2 = (x + 1)(x - 1)\).
Thus, the factored form of \(x^2 - 1\) is \((x + 1)(x - 1)\).
This method is useful for solving quadratic equations and simplifying algebraic expressions. With practice, recognizing and applying the difference of squares becomes easier.
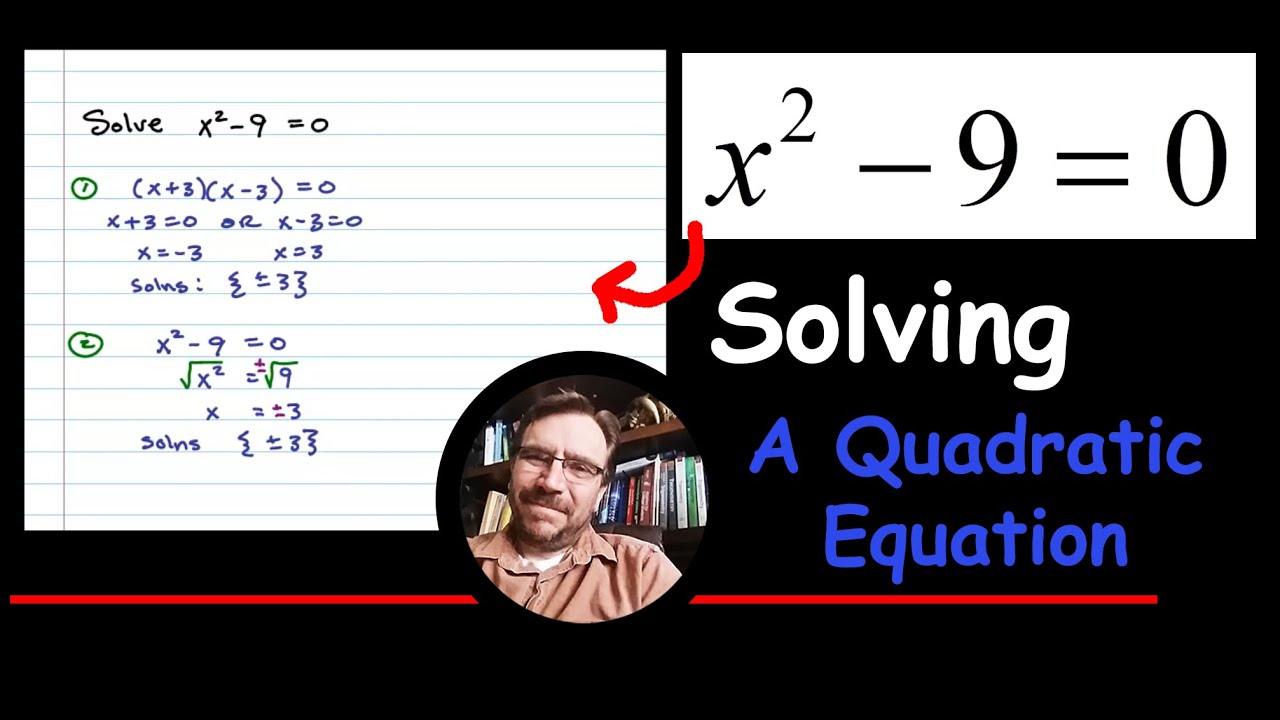
Completing the Square
Completing the square is a method used to solve quadratic equations by transforming the equation into a perfect square trinomial. This technique is particularly useful when the quadratic formula is not preferred or when dealing with specific types of equations. The steps involved in completing the square are as follows:
- Start with the quadratic equation in the form \(ax^2 + bx + c = 0\).
- Move the constant term \(c\) to the other side of the equation: \(ax^2 + bx = -c\).
- If the leading coefficient \(a\) is not 1, divide every term by \(a\) to normalize the equation: \(x^2 + \frac{b}{a}x = -\frac{c}{a}\).
- Take half of the coefficient of \(x\), square it, and add it to both sides of the equation. This transforms the left side into a perfect square trinomial: \(x^2 + \frac{b}{a}x + \left(\frac{b}{2a}\right)^2 = -\frac{c}{a} + \left(\frac{b}{2a}\right)^2\).
- Rewrite the left side as a squared binomial: \(\left(x + \frac{b}{2a}\right)^2 = -\frac{c}{a} + \left(\frac{b}{2a}\right)^2\).
- Solve for \(x\) by taking the square root of both sides and then isolating \(x\): \(x + \frac{b}{2a} = \pm \sqrt{-\frac{c}{a} + \left(\frac{b}{2a}\right)^2}\).
- Simplify to find the values of \(x\).
For example, to solve \(x^2 - 4x - 1 = 0\) by completing the square:
- Move the constant term: \(x^2 - 4x = 1\).
- Add \(\left(\frac{-4}{2}\right)^2 = 4\) to both sides: \(x^2 - 4x + 4 = 1 + 4\).
- Rewrite the left side as a squared binomial: \((x - 2)^2 = 5\).
- Solve for \(x\): \(x - 2 = \pm \sqrt{5}\), so \(x = 2 \pm \sqrt{5}\).
Completing the square not only provides a method for solving quadratics but also gives insights into the properties of quadratic functions, including their vertex form and graphing.
Applications in Algebra
The expression \( x^2 - 1 \) is a fundamental quadratic form that finds numerous applications in algebra. Below are some key applications, explained in detail:
-
Simplifying Algebraic Expressions
The expression \( x^2 - 1 \) can be factored using the difference of squares formula:
\[ x^2 - 1 = (x + 1)(x - 1) \]
This factorization simplifies many algebraic operations, such as simplifying rational expressions.
-
Solving Equations
Setting \( x^2 - 1 \) equal to zero provides a simple quadratic equation:
\[ x^2 - 1 = 0 \]
Solving this equation involves factoring and setting each factor to zero:
\[ (x + 1)(x - 1) = 0 \]
This gives the solutions:
\[ x = 1 \text{ and } x = -1 \]
-
Inequalities
To solve inequalities involving \( x^2 - 1 \), such as \( x^2 - 1 \leq 0 \), we can factor and analyze the critical points:
\[ x^2 - 1 \leq 0 \rightarrow (x + 1)(x - 1) \leq 0 \]
The solutions are found by testing intervals around the roots \( x = 1 \) and \( x = -1 \), resulting in:
\[ -1 \leq x \leq 1 \]
-
Graphing
The graph of the function \( y = x^2 - 1 \) is a parabola that opens upwards, with its vertex at (0, -1) and x-intercepts at (1, 0) and (-1, 0). This visualization helps in understanding the behavior of quadratic functions.
x y -2 3 -1 0 0 -1 1 0 2 3 -
Applications in Calculus
The function \( f(x) = x^2 - 1 \) is often used to illustrate basic concepts in calculus, such as finding limits, derivatives, and integrals. For example, the derivative of \( f(x) \) is:
\[ f'(x) = 2x \]
This derivative helps in understanding the slope and concavity of the function.
-
Number Theory
In number theory, the expression \( x^2 - 1 \) is used in problems involving integer solutions and factorization properties. For example, it can help in finding Pythagorean triples.
Graphical Representations
The quadratic function \(x^2 - 1\) is a fundamental equation in algebra, and its graphical representation provides significant insights into its properties and applications.
To graph the equation \(x^2 - 1\), follow these steps:
- Identify the vertex: The equation \(x^2 - 1\) is in the standard form \(ax^2 + bx + c\) where \(a = 1\), \(b = 0\), and \(c = -1\). The vertex form of a quadratic function is \(a(x - h)^2 + k\), where \((h, k)\) is the vertex. For \(x^2 - 1\), the vertex is at \((0, -1)\).
- Determine the axis of symmetry: The axis of symmetry for \(x^2 - 1\) is the vertical line \(x = 0\), which passes through the vertex.
- Find the intercepts:
- Y-intercept: Set \(x = 0\), then \(y = x^2 - 1 = -1\). So, the y-intercept is \((0, -1)\).
- X-intercepts: Set \(y = 0\), then solve \(x^2 - 1 = 0\). This gives \(x^2 = 1\), so \(x = \pm 1\). The x-intercepts are \((1, 0)\) and \((-1, 0)\).
- Plot the points: Plot the vertex \((0, -1)\), y-intercept \((0, -1)\), and x-intercepts \((1, 0)\) and \((-1, 0)\).
- Draw the parabola: Since the coefficient of \(x^2\) is positive, the parabola opens upwards. Draw a smooth curve through the plotted points, making sure it is symmetrical about the axis of symmetry.
Here is a table summarizing the critical points:
| Point | Coordinates |
|---|---|
| Vertex | (0, -1) |
| Y-intercept | (0, -1) |
| X-intercepts | (1, 0) and (-1, 0) |
The graph of \(y = x^2 - 1\) illustrates the fundamental properties of quadratic functions:
- The parabola is symmetric about the y-axis.
- The vertex represents the minimum point of the function.
- The function has two x-intercepts, showing where it crosses the x-axis.
The graphical representation helps in visualizing the behavior of the quadratic function, understanding its roots, and analyzing its vertex and symmetry.

Common Mistakes and Misconceptions
When dealing with \(x^2 - 1\), there are several common mistakes and misconceptions that students often encounter. Understanding and addressing these can help in mastering the topic.
- Misunderstanding the Difference of Squares:
One of the most frequent mistakes is not recognizing that \(x^2 - 1\) is a difference of squares, which can be factored into \((x + 1)(x - 1)\). Students might incorrectly try to apply other factoring techniques.
- Incorrectly Solving \(x^2 - 1 = 0\):
Another common error is in solving the equation \(x^2 - 1 = 0\). Some students might forget to set the equation to zero first or might only find one solution, \(x = 1\), and miss the other solution, \(x = -1\).
- Ignoring the Zero Product Property:
When solving \((x + 1)(x - 1) = 0\), students sometimes overlook the zero product property, which states that if a product is zero, at least one of the factors must be zero. Thus, \(x + 1 = 0\) or \(x - 1 = 0\), leading to the solutions \(x = -1\) and \(x = 1\).
- Order of Operations Errors:
Order of operations is crucial in algebra. For example, when expanding expressions involving \(x^2\), ensure that students apply the operations in the correct sequence to avoid errors.
- Factoring Mistakes:
Students may incorrectly factor \(x^2 - 1\) as \(x(x - 1)\) or some other incorrect form. Remind them of the correct factorization into \((x + 1)(x - 1)\).
- Misconceptions with Square Roots:
When dealing with square roots, students might mistakenly think \(\sqrt{x^2 - 1} = x - 1\). Correct them by explaining that square roots and squares cancel out under certain conditions, and remind them of the correct handling of such expressions.
By addressing these common mistakes and misconceptions, students can develop a stronger understanding of how to work with \(x^2 - 1\) and similar algebraic expressions.
Practical Examples and Exercises
The equation \(x^2 - 1\) offers numerous opportunities for practical application and practice. Below are some examples and exercises designed to help reinforce your understanding of this concept.
Example 1: Factoring \(x^2 - 1\)
Factor the expression \(x^2 - 1\).
- Solution: Recognize that \(x^2 - 1\) is a difference of squares.
- Use the formula \(a^2 - b^2 = (a - b)(a + b)\).
- Here, \(a = x\) and \(b = 1\).
- Thus, \(x^2 - 1 = (x - 1)(x + 1)\).
Example 2: Solving the Equation \(x^2 - 1 = 0\)
Solve for \(x\).
- Set the equation to zero: \(x^2 - 1 = 0\).
- Factor the expression: \((x - 1)(x + 1) = 0\).
- Set each factor to zero: \(x - 1 = 0\) or \(x + 1 = 0\).
- Solve each equation: \(x = 1\) or \(x = -1\).
- Thus, the solutions are \(x = 1\) and \(x = -1\).
Exercise 1: Graphing \(y = x^2 - 1\)
Graph the function \(y = x^2 - 1\) and identify its key features.
- Plot the vertex: The vertex is at \((0, -1)\).
- Determine the axis of symmetry: The axis of symmetry is the y-axis, or \(x = 0\).
- Find the x-intercepts: Set \(y = 0\) to get \(x^2 - 1 = 0\), which gives \(x = \pm 1\).
- Find the y-intercept: Set \(x = 0\) to get \(y = -1\).
- Draw the parabola opening upwards.
Exercise 2: Application in Geometry
Use the equation \(x^2 - 1\) in a geometric context.
- Consider a square with side length \(x\). The area is \(x^2\).
- If one unit is subtracted from the area, we have \(x^2 - 1\).
- For a side length \(x = 2\), calculate the new area: \(2^2 - 1 = 4 - 1 = 3\).
Practice Problems
- Simplify the expression: \( (3x)^2 - 1 \).
- Solve for \(x\): \( 4x^2 - 4 = 0 \).
- Factor the expression: \( 9x^2 - 1 \).
Solutions
- For \( (3x)^2 - 1 \): Apply the difference of squares to get \( (3x - 1)(3x + 1) \).
- For \( 4x^2 - 4 = 0 \): Factor to get \( 4(x^2 - 1) = 0 \), then \( 4(x - 1)(x + 1) = 0 \). Solutions are \( x = 1 \) and \( x = -1 \).
- For \( 9x^2 - 1 \): Use the difference of squares to get \( (3x - 1)(3x + 1) \).
Video khám phá liệu phương trình 1^x có thể bằng 2 hay không, dành cho những ai yêu thích toán học và muốn tìm hiểu sâu hơn.
Có thể 1^x=2?
READ MORE:
Video hướng dẫn cách giải phương trình bậc hai bằng phương pháp hoàn thành bình phương, phù hợp cho những ai muốn học toán nâng cao và nắm vững kỹ thuật này.
Giải phương trình bậc hai bằng cách hoàn thành bình phương
x-1=sec(squared)x.jpg)