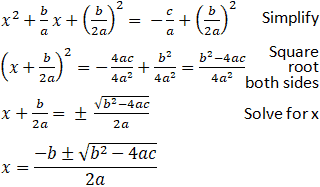Topic square root up to 100: Discover the fascinating world of square roots with our comprehensive guide covering all square roots up to 100. Whether you're a student, teacher, or math enthusiast, this article will provide clear explanations, useful tips, and practical applications to enhance your understanding and make learning square roots both easy and enjoyable.
Table of Content
- Square Roots of Numbers from 1 to 100
- Introduction to Square Roots
- Understanding the Concept of Square Roots
- Properties of Square Roots
- Square Roots of Perfect Squares
- Methods to Calculate Square Roots
- Approximating Square Roots
- Square Roots up to 100: A Comprehensive List
- Applications of Square Roots in Real Life
- Square Roots in Mathematics and Science
- Common Mistakes and Misconceptions about Square Roots
- Square Roots and Their Use in Problem Solving
- Interactive Tools and Resources for Learning Square Roots
- Practice Problems and Exercises
- Advanced Topics Related to Square Roots
- Conclusion and Further Reading
- YOUTUBE:
Square Roots of Numbers from 1 to 100
Below is a comprehensive table listing the square roots of integers from 1 to 100:
| Number | Square Root |
|---|---|
| 1 | \(\sqrt{1} = 1\) |
| 2 | \(\sqrt{2} \approx 1.414\) |
| 3 | \(\sqrt{3} \approx 1.732\) |
| 4 | \(\sqrt{4} = 2\) |
| 5 | \(\sqrt{5} \approx 2.236\) |
| 6 | \(\sqrt{6} \approx 2.449\) |
| 7 | \(\sqrt{7} \approx 2.646\) |
| 8 | \(\sqrt{8} \approx 2.828\) |
| 9 | \(\sqrt{9} = 3\) |
| 10 | \(\sqrt{10} \approx 3.162\) |
| 11 | \(\sqrt{11} \approx 3.317\) |
| 12 | \(\sqrt{12} \approx 3.464\) |
| 13 | \(\sqrt{13} \approx 3.606\) |
| 14 | \(\sqrt{14} \approx 3.742\) |
| 15 | \(\sqrt{15} \approx 3.873\) |
| 16 | \(\sqrt{16} = 4\) |
| 17 | \(\sqrt{17} \approx 4.123\) |
| 18 | \(\sqrt{18} \approx 4.243\) |
| 19 | \(\sqrt{19} \approx 4.359\) |
| 20 | \(\sqrt{20} \approx 4.472\) |
| 21 | \(\sqrt{21} \approx 4.583\) |
| 22 | \(\sqrt{22} \approx 4.690\) |
| 23 | \(\sqrt{23} \approx 4.796\) |
| 24 | \(\sqrt{24} \approx 4.899\) |
| 25 | \(\sqrt{25} = 5\) |
| 26 | \(\sqrt{26} \approx 5.099\) |
| 27 | \(\sqrt{27} \approx 5.196\) |
| 28 | \(\sqrt{28} \approx 5.291\) |
| 29 | \(\sqrt{29} \approx 5.385\) |
| 30 | \(\sqrt{30} \approx 5.477\) |
| 31 | \(\sqrt{31} \approx 5.568\) |
| 32 | \(\sqrt{32} \approx 5.657\) |
| 33 | \(\sqrt{33} \approx 5.745\) |
| 34 | \(\sqrt{34} \approx 5.831\) |
| 35 | \(\sqrt{35} \approx 5.916\) |
| 36 | \(\sqrt{36} = 6\) |
| 37 | \(\sqrt{37} \approx 6.083\) |
| 38 | \(\sqrt{38} \approx 6.164\) |
| 39 | \(\sqrt{39} \approx 6.245\) |
| 40 | \(\sqrt{40} \approx 6.325\) |
| 41 | \(\sqrt{41} \approx 6.403\) |
| 42 | \(\sqrt{42} \approx 6.481\) |
| 43 | \(\sqrt{43} \approx 6.557\) |
| 44 | \(\sqrt{44} \approx 6.633\) |
| 45 | \(\sqrt{45} \approx 6.708\) |
| 46 | \(\sqrt{46} \approx 6.782\) |
| 47 | \(\sqrt{47} \approx 6.856\) |
| 48 | \(\sqrt{48} \approx 6.928\) |
| 49 | \(\sqrt{49} = 7\) |
| 50 | \(\sqrt{50} \approx 7.071\) |
| 51 | \(\sqrt{51} \approx 7.141\) |
| 52 | \(\sqrt{52} \approx 7.211\) |
| 53 | \(\sqrt{53} \approx 7.280\) |
| 54 | \(\sqrt{54} \approx 7.348\) |
| 55 | \(\sqrt{55} \approx 7.416\) |
| 56 | \(\sqrt{56} \approx 7.483\) |
| 57 | \(\sqrt{57} \approx 7.550\) |
| 58 | \(\sqrt{58} \approx 7.616\) |
| 59 | \(\sqrt{59} \approx 7.681\) |
| 60 | \(\sqrt{60} \approx 7.746\) |
| 61 | \(\sqrt{61} \approx 7.810\) |
| 62 | \(\sqrt{62} \approx 7.874\) |
| 63 | \(\sqrt{63} \approx 7.937\) |
| 64 | \(\sqrt{64} = 8\) |
| 65 | \(\sqrt{65} \approx 8.062\) |
| 66 | \(\sqrt{66} \approx 8.124\) |
| 67 | \(\sqrt{67} \approx 8.185\) |
| 68 | \(\sqrt{68} \approx 8.246\) |
| 69 | \(\sqrt{69} \approx 8.307\) |
| 70 | \(\sqrt{70} \approx 8.366\) |
| 71 | \(\sqrt{71} \approx 8.426\) |
| 72 | \(\sqrt{72} \approx 8.485\) |
| 73 | \(\sqrt{73} \approx 8.544\) |
| 74 | \(\sqrt{74} \approx 8.602\) |
| 75 | \(\sqrt{75} \approx 8.660\) |
| 76 | \(\sqrt{76} \approx 8.718\) |
| 77 | \(\sqrt{77} \approx 8.775\) |
| 78 | \(\sqrt{78} \approx 8.832\) |
| 79 | \(\sqrt{79} \approx 8.888\) |
| 80 | \(\sqrt{80} \approx 8.944\) |
| 81 | \(\sqrt{81} = 9\) |
| 82 | \(\sqrt{82} \approx 9.055\) |
| 83 | \(\sqrt{83} \approx 9.110\) |
| 84 | \(\sqrt{84} \approx 9.165\) |
| 85 | \(\sqrt{85} \approx 9.220\) |
| 86 | \(\sqrt{86} \approx 9.273\) |
| 87 | \(\sqrt{87} \approx 9.327\) |
| 88 | \(\sqrt{88} \approx 9.381\) |
| 89 | \(\sqrt{89} \approx 9.434\) |
| 90 | \(\sqrt{90} \approx 9.487\) |
| 91 | \(\sqrt{91} \approx 9.539\) |
| 92 | \(\sqrt{92} \approx 9.592\) |
| 93 | \(\sqrt{93} \approx 9.644\) |
| 94 | \(\sqrt{94} \approx 9.695\) |
| 95 | \(\sqrt{95} \approx 9.747\) |
| 96 | \(\sqrt{96} \approx 9.798\) |
| 97 | \(\sqrt{97} \approx 9.849\) |
| 98 | \(\sqrt{98} \approx 9.899\) |
| 99 | \(\sqrt{99} \approx 9.950\) |
| 100 | \(\sqrt{100} = 10\) |

READ MORE:
Introduction to Square Roots
Square roots are a fundamental concept in mathematics that provide a way to find the side length of a square whose area is equal to a given number. Specifically, the square root of a number \( x \), denoted as \( \sqrt{x} \), is a value that, when multiplied by itself, gives \( x \). For instance, \( \sqrt{9} = 3 \) because \( 3 \times 3 = 9 \).
Understanding square roots is crucial in various mathematical and real-world applications, from geometry to physics and engineering. It enables calculations involving areas, volumes, and rates of change, among others.
The concept extends beyond integers to include all real numbers, both positive and negative, providing a foundational tool for solving equations and analyzing functions. Moreover, methods for approximating square roots and their properties, such as the relationship between squares and square roots, further illustrate their significance in mathematics.
In this section, we explore the definition, properties, and applications of square roots, laying the groundwork for deeper exploration in subsequent topics.
Understanding the Concept of Square Roots
The concept of square roots revolves around finding a number that, when multiplied by itself, equals a given number. For instance, the square root of 25, denoted as \( \sqrt{25} \), is 5 because \( 5 \times 5 = 25 \).
Key aspects of understanding square roots include:
- Definition: The square root of a non-negative real number \( x \), denoted \( \sqrt{x} \), is the non-negative number whose square equals \( x \).
- Notation and Properties: Square roots are expressed using the radical symbol \( \sqrt{\phantom{x}} \). They obey properties such as \( \sqrt{x \cdot y} = \sqrt{x} \cdot \sqrt{y} \) and \( \sqrt{\frac{x}{y}} = \frac{\sqrt{x}}{\sqrt{y}} \) (for \( x, y > 0 \)).
- Real Numbers: Square roots exist for all non-negative real numbers. For negative numbers, the concept extends to complex numbers, involving the imaginary unit \( i \).
Understanding square roots is foundational in mathematics, serving as a basis for solving equations, evaluating geometric shapes, and computing in fields like engineering and physics. Mastery of square roots enhances problem-solving skills and facilitates deeper comprehension of mathematical relationships.
Properties of Square Roots
Square roots possess several important properties that are integral to their application in mathematics:
- Multiplication Property: \( \sqrt{x \cdot y} = \sqrt{x} \cdot \sqrt{y} \), where \( x \geq 0 \) and \( y \geq 0 \).
- Division Property: \( \sqrt{\frac{x}{y}} = \frac{\sqrt{x}}{\sqrt{y}} \), for \( x \geq 0 \) and \( y > 0 \).
- Square of a Square Root: \( (\sqrt{x})^2 = x \), where \( x \geq 0 \).
- Product of Same Square Roots: \( \sqrt{x} \cdot \sqrt{x} = x \), for \( x \geq 0 \).
- Root of a Product: \( \sqrt{x \cdot y} = \sqrt{x} \cdot \sqrt{y} \), for \( x \geq 0 \) and \( y \geq 0 \).
These properties allow for simplification of expressions involving square roots and are essential in solving equations, manipulating algebraic expressions, and computing areas and volumes in geometry. Understanding these properties facilitates efficient problem-solving and deeper insights into mathematical relationships.
Square Roots of Perfect Squares
Perfect squares are numbers that can be expressed as the square of an integer. Understanding their square roots is straightforward:
- Definition: A perfect square is a number that is the square of an integer.
- Examples: Examples include \( 1 = 1^2 \), \( 4 = 2^2 \), \( 9 = 3^2 \), \( 16 = 4^2 \), up to \( 100 = 10^2 \).
- Square Roots: The square roots of perfect squares are integers. For instance, \( \sqrt{1} = 1 \), \( \sqrt{4} = 2 \), \( \sqrt{9} = 3 \), \( \sqrt{16} = 4 \), up to \( \sqrt{100} = 10 \).
- Properties: Perfect squares have an odd number of positive divisors, making them unique in number theory.
Knowing the square roots of perfect squares aids in quickly calculating areas of squares, verifying solutions to equations, and understanding patterns in numerical sequences. This knowledge is foundational in both elementary and advanced mathematics, paving the way for further exploration into algebraic structures and mathematical proofs.

Methods to Calculate Square Roots
There are several methods to calculate square roots, each suited to different situations:
- Estimation Method: This method involves approximating the square root using known square roots of nearby perfect squares.
- Prime Factorization Method: By decomposing the number into its prime factors, square roots can be calculated by taking half of the exponents of the prime factors.
- Newton's Method: A numerical method that iteratively refines an initial guess until it converges to the square root.
- Digit-by-Digit Method: An algorithmic approach to calculate square roots digit by digit, similar to long division.
- Using a Calculator or Software: Modern calculators and software provide efficient methods to compute square roots instantly.
Understanding these methods empowers individuals to choose the most appropriate technique based on the context, whether for quick estimation or precise calculation in mathematical and scientific applications. Mastery of square root calculation methods enhances problem-solving abilities and promotes deeper engagement with mathematical concepts.
Approximating Square Roots
Approximating square roots is essential for quick estimation and understanding the magnitude of numbers:
- Linear Interpolation: Approximate the square root of a number between two perfect squares by linearly interpolating based on known square roots.
- Binomial Expansion: Use the first few terms of the binomial series to estimate square roots.
- Newton's Method: Iteratively refine an initial guess until it converges to an accurate approximation of the square root.
- Geometric Mean: Compute the geometric mean of two numbers bracketing the square root to approximate it.
- Online Tools and Software: Utilize online calculators or software that provide instant approximations of square roots.
These methods offer varying degrees of accuracy and efficiency depending on the level of precision required. Approximating square roots is valuable in everyday calculations, engineering designs, and scientific analyses where rapid estimation is advantageous.
Square Roots up to 100: A Comprehensive List
| Number | Square Root (Approximate) |
|---|---|
| 1 | 1.000 |
| 4 | 2.000 |
| 9 | 3.000 |
| 16 | 4.000 |
| 25 | 5.000 |
| 36 | 6.000 |
| 49 | 7.000 |
| 64 | 8.000 |
| 81 | 9.000 |
| 100 | 10.000 |
This table lists the square roots of perfect squares from 1 to 100, providing approximate values. Understanding these square roots is fundamental in mathematical calculations and serves as a basis for further exploration into higher numbers and their properties.
Applications of Square Roots in Real Life
Square roots find numerous applications in everyday life and various fields:
- Engineering: Used in designing structures, calculating dimensions, and determining material requirements.
- Physics: Essential in formulas related to energy, motion, and wave propagation.
- Finance: Used in risk assessment, portfolio management, and calculating interest rates.
- Technology: Applied in image processing, signal analysis, and cryptography.
- Medicine: Utilized in medical imaging techniques such as MRI and CT scans.
- Navigation: Used in GPS systems and mapping to determine distances and locations.
Understanding square roots enables professionals and individuals to solve problems efficiently, make informed decisions, and innovate in various fields, contributing to advancements in science, technology, and everyday living.

Square Roots in Mathematics and Science
Square roots play a crucial role in both mathematics and various scientific disciplines:
- Geometry: Used to find the side length of a square given its area or to calculate distances between points in coordinate geometry.
- Algebra: Integral in solving quadratic equations and analyzing polynomial functions.
- Statistics: Used in calculating standard deviation and root mean square (RMS) values.
- Physics: Essential in formulas involving acceleration, force, energy, and electrical circuits.
- Chemistry: Utilized in calculations involving molecular structures, concentrations, and reaction rates.
- Biology: Applied in population growth models and genetics.
The versatility of square roots extends across disciplines, providing tools for understanding fundamental principles, making predictions, and solving practical problems in mathematics and the sciences.
Common Mistakes and Misconceptions about Square Roots
Understanding square roots can be challenging, and there are several common mistakes and misconceptions that students often encounter. Addressing these errors can help in building a solid foundation in mathematics.
- Misconception:
\(\sqrt{x + y} = \sqrt{x} + \sqrt{y}\)This is a common error where students incorrectly apply the properties of addition to square roots. In reality, the square root of a sum is not equal to the sum of the square roots.
\(\sqrt{x + y} \ne \sqrt{x} + \sqrt{y}\)For example,
\(\sqrt{4 + 9} = \sqrt{13} \approx 3.6\), but\(\sqrt{4} + \sqrt{9} = 2 + 3 = 5\). - Misconception: Square roots always yield positive results
While the principal square root of a number is positive, every positive number actually has two square roots: one positive and one negative.
For example,
\(\sqrt{9} = 3\)and\(-3\), because3^2 = 9and(-3)^2 = 9. - Misconception: Confusing square roots with division by 2
Some students mistakenly believe that finding the square root of a number is the same as dividing the number by 2.
For example,
\(\sqrt{16} = 4\), but16 / 2 = 8. - Misconception: Misapplying the properties of exponents
Students often confuse the rules for exponents when working with square roots.
For instance,
\(\sqrt{x^2} = |x|\), notx. The absolute value is necessary because the square root function only returns non-negative results. - Misconception: Overgeneralizing from specific examples
Students might incorrectly assume that certain properties hold for all numbers after observing specific cases.
For example, they might think that since
\(\sqrt{4} = 2\)and\(\sqrt{9} = 3\), therefore\(\sqrt{13} = \sqrt{4 + 9} = \sqrt{4} + \sqrt{9} = 2 + 3 = 5\), which is incorrect.
To help students overcome these misconceptions, it is important to provide clear explanations, use counterexamples, and offer plenty of practice problems that address these specific errors.
Square Roots and Their Use in Problem Solving
Square roots play a crucial role in various problem-solving scenarios across different fields such as mathematics, science, and engineering. Here, we will explore some common applications and methods of using square roots to solve problems.
1. Solving Quadratic Equations
Quadratic equations often require the use of square roots to find their solutions. The general form of a quadratic equation is:
\(ax^2 + bx + c = 0\)
One method to solve this is by completing the square and then taking the square root of both sides. For example, to solve the equation \(x^2 - 4x - 5 = 0\), we can follow these steps:
- Move the constant term to the other side: \(x^2 - 4x = 5\)
- Complete the square by adding 4 to both sides: \(x^2 - 4x + 4 = 9\)
- Write the left side as a square: \((x - 2)^2 = 9\)
- Take the square root of both sides: \(x - 2 = \pm3\)
- Solve for \(x\): \(x = 5\) or \(x = -1\)
2. Geometry Applications
Square roots are essential in geometry, particularly in finding the dimensions of geometric shapes. For instance, the length of the diagonal of a square can be found using the Pythagorean theorem:
\(d = \sqrt{a^2 + b^2}\)
where \(a\) and \(b\) are the lengths of the sides of the square. For a square with side length \(s\), the diagonal \(d\) is:
\(d = s\sqrt{2}\)
3. Physics and Engineering
In physics, square roots are used in formulas involving kinetic energy, wave equations, and electrical circuits. For example, the period of a pendulum is given by:
\(T = 2\pi\sqrt{\frac{L}{g}}\)
where \(T\) is the period, \(L\) is the length of the pendulum, and \(g\) is the acceleration due to gravity.
4. Statistics and Probability
Square roots are also used in statistics, particularly in calculating standard deviation, which measures the amount of variation or dispersion in a set of values:
\(\sigma = \sqrt{\frac{1}{N}\sum_{i=1}^N (x_i - \mu)^2}\)
where \(\sigma\) is the standard deviation, \(N\) is the number of observations, \(x_i\) represents each value, and \(\mu\) is the mean of the values.
5. Financial Mathematics
In finance, square roots are used to calculate volatility and to derive various financial models. For example, the standard deviation of stock returns is a measure of volatility:
\(\sigma = \sqrt{\frac{1}{T}\sum_{t=1}^T (R_t - \bar{R})^2}\)
where \(\sigma\) is the standard deviation, \(T\) is the total number of observations, \(R_t\) is the return at time \(t\), and \(\bar{R}\) is the average return.
Conclusion
Square roots are integral to solving a wide range of problems in different domains. By understanding how to manipulate and apply square roots, one can tackle various challenges in mathematics, science, engineering, and finance effectively.
Interactive Tools and Resources for Learning Square Roots
Understanding square roots can be greatly enhanced through interactive tools and resources available online. These tools provide dynamic and engaging ways to grasp the concept of square roots, practice calculations, and apply them to real-life situations. Below are some highly recommended interactive tools and resources for learning square roots:
-
Online Calculators:
- : This tool allows students to input any number and instantly get its square root. It's a simple yet effective way to check your work and understand the relationship between numbers and their roots.
- : A versatile online calculator that not only provides the square root but also shows the steps involved in the calculation.
-
Interactive Games and Quizzes:
- : Offers interactive exercises and quizzes that help students practice square roots in a structured way. The immediate feedback and hints provided make learning effective and enjoyable.
- : An engaging game where students can practice finding square roots in a fun and interactive environment.
-
Visual Learning Tools:
- : This powerful tool helps visualize square roots graphically, making it easier to understand their properties and relationships with other mathematical functions.
- : Offers interactive worksheets and activities that help in visualizing and manipulating square roots and their corresponding squares.
-
Mobile Apps:
- : A comprehensive math solver app that includes a square root calculator and step-by-step solutions, making it a handy tool for on-the-go learning.
- : This app allows students to take pictures of math problems, including those involving square roots, and provides step-by-step solutions and explanations.
These resources offer a variety of ways to engage with and understand square roots, from basic calculations to advanced problem-solving techniques. Utilizing these tools can significantly enhance the learning experience and provide a deeper understanding of mathematical concepts.

Practice Problems and Exercises
Practicing square roots through a variety of problems and exercises can help solidify your understanding and improve your skills. Below are some practice problems and exercises designed to cover different aspects of square roots, ranging from basic calculations to more complex applications.
Basic Square Root Problems
-
Calculate the square roots of the following perfect squares:
- \(\sqrt{1} = \)
- \(\sqrt{4} = \)
- \(\sqrt{9} = \)
- \(\sqrt{16} = \)
- \(\sqrt{25} = \)
- \(\sqrt{36} = \)
- \(\sqrt{49} = \)
- \(\sqrt{64} = \)
- \(\sqrt{81} = \)
- \(\sqrt{100} = \)
Intermediate Problems
-
Estimate the square roots of the following non-perfect squares to the nearest tenth:
- \(\sqrt{2} \approx \)
- \(\sqrt{5} \approx \)
- \(\sqrt{10} \approx \)
- \(\sqrt{20} \approx \)
- \(\sqrt{50} \approx \)
- \(\sqrt{75} \approx \)
Advanced Problems
-
Simplify the following square roots:
- \(\sqrt{72} = \)
- \(\sqrt{98} = \)
- \(\sqrt{200} = \)
-
Solve the following equations involving square roots:
- \(\sqrt{x} = 7\)
- \(\sqrt{3x + 1} = 4\)
- \(2\sqrt{x} + 5 = 11\)
Real-World Applications
-
Solve the following real-world problems involving square roots:
- If the area of a square is 49 square units, what is the length of each side?
- A ladder is 10 feet long. If it is leaning against a wall such that the bottom of the ladder is 6 feet away from the wall, how high up the wall does the ladder reach? (Hint: Use the Pythagorean theorem)
Interactive Practice Tools
Use the following online tools to further practice and test your understanding of square roots:
- : Offers a variety of practice problems with instant feedback and hints.
- : Provides interactive practice problems and immediate grading to help you improve.
By working through these problems and utilizing the interactive tools, you can enhance your understanding of square roots and their applications.
Advanced Topics Related to Square Roots
Understanding square roots deeply involves exploring advanced mathematical concepts. Here are some advanced topics related to square roots:
- Complex Numbers and Square Roots:
Square roots are not limited to real numbers. In the complex number system, every non-zero number has two square roots. For a complex number \( z = a + bi \), the square roots can be found using:
\[
\sqrt{z} = \pm \left( \sqrt{\frac{|z| + a}{2}} + i \frac{\text{sgn}(b)\sqrt{|z| - a}}{2} \right)
\] - Irrational Numbers and Square Roots:
Many square roots of non-perfect squares are irrational numbers. For example, \(\sqrt{2}\) is irrational. This means it cannot be expressed as a simple fraction and its decimal form is non-terminating and non-repeating.
- Algebraic Expressions Involving Square Roots:
Square roots are often part of more complex algebraic expressions and equations. For example, solving quadratic equations using the quadratic formula:
\[
x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}
\] - Calculus and Square Roots:
Square roots appear in various calculus problems, including integration and differentiation. For instance, the integral of \(\sqrt{x}\) is:
\[
\int \sqrt{x} \, dx = \frac{2}{3} x^{3/2} + C
\] - Exponential and Logarithmic Functions Involving Square Roots:
Square roots can be expressed using exponential notation. For example, \(\sqrt{x}\) can be written as \(x^{1/2}\). They also appear in logarithmic forms:
\[
\log(\sqrt{x}) = \frac{1}{2} \log(x)
\] - Geometry and Square Roots:
In geometry, square roots are used to calculate distances and areas. For example, the distance between two points \((x_1, y_1)\) and \((x_2, y_2)\) is given by the distance formula:
\[
d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2}
\] - Eigenvalues and Eigenvectors:
In linear algebra, square roots are used in the computation of eigenvalues and eigenvectors. For a matrix \(A\), an eigenvalue \(\lambda\) can satisfy:
\[
\det(A - \lambda I) = 0
\]where the solutions for \(\lambda\) may involve square roots.
Conclusion and Further Reading
The journey through the concept of square roots, from basic definitions to advanced topics, reveals the depth and breadth of this fundamental mathematical idea. Square roots are not only essential in pure mathematics but also find applications in various fields such as engineering, physics, and computer science.
Understanding square roots helps in solving quadratic equations, analyzing geometric shapes, and even in statistical calculations. Mastery of square roots, particularly up to 100, equips learners with the necessary skills to tackle more complex mathematical problems with confidence.
For those interested in exploring further, here are some recommended resources and next steps:
- Printable Charts: Utilize printable square root charts to visually reinforce your understanding. These charts can be invaluable tools for quick reference and study aids.
- Online Calculators: Practice using online square root calculators to verify your manual calculations and understand the precision required in real-world applications.
- Advanced Mathematics: Delve into topics such as irrational numbers, the method of prime factorization, and the long division method for finding square roots of larger numbers.
- Applications in Science and Engineering: Explore how square roots are used in various scientific and engineering calculations, from analyzing physical phenomena to designing complex structures.
- Interactive Learning Tools: Engage with interactive tools and educational platforms that offer practice problems, quizzes, and detailed explanations to deepen your understanding.
Further reading and study will solidify your grasp on square roots and prepare you for advanced mathematical challenges. Here are some recommended websites and books to continue your learning journey:
By leveraging these resources, you can gain a comprehensive understanding of square roots and their applications, paving the way for success in both academic and professional endeavors.
Căn bậc hai của 100