Topic square root quadratic equation calculator: Discover how a square root quadratic equation calculator can simplify solving complex mathematical problems. This tool helps you find real and complex roots quickly and accurately. Whether you're a student or a math enthusiast, understanding and utilizing this calculator can enhance your problem-solving skills and boost your confidence in tackling quadratic equations.
Table of Content
- Square Root Quadratic Equation Calculator
- Introduction to Quadratic Equations
- Steps to Solve Quadratic Equations
- Solving Quadratic Equations by Square Root Method
- Graphing Quadratic Equations
- Examples
- Online Quadratic Equation Calculators
- YOUTUBE: Cách tính căn bậc hai của phương trình bậc hai bằng máy tính khoa học.
Square Root Quadratic Equation Calculator
Quadratic equations are mathematical expressions of the form \(ax^2 + bx + c = 0\), where \(a\), \(b\), and \(c\) are constants. Solving these equations often involves finding the values of \(x\) that satisfy the equation. One common method is using the square root approach.
Quadratic Formula
The quadratic formula provides the solution to a quadratic equation:
\[ x = \frac{{-b \pm \sqrt{{b^2 - 4ac}}}}{2a} \]
Steps to Solve Using Square Root
- Ensure the quadratic equation is in the standard form \(ax^2 + bx + c = 0\).
- Calculate the discriminant \(\Delta\): \[ \Delta = b^2 - 4ac \]
- Determine the number and type of roots:
- If \(\Delta > 0\), there are two real and distinct roots.
- If \(\Delta = 0\), there is one real root (a repeated root).
- If \(\Delta < 0\), there are two complex roots.
- Apply the quadratic formula to find the roots:
- For real roots: \[ x = \frac{{-b \pm \sqrt{\Delta}}}{2a} \]
- For complex roots: \[ x = \frac{{-b \pm i\sqrt{|\Delta|}}}{2a} \]
Example Calculation
Consider the quadratic equation \(2x^2 - 4x - 6 = 0\). Follow these steps:
- Identify \(a = 2\), \(b = -4\), and \(c = -6\).
- Calculate the discriminant: \[ \Delta = (-4)^2 - 4(2)(-6) = 16 + 48 = 64 \]
- Since \(\Delta > 0\), there are two real roots.
- Apply the quadratic formula:
\[ x = \frac{{4 \pm \sqrt{64}}}{4} = \frac{{4 \pm 8}}{4} \]
- First root: \[ x = \frac{{4 + 8}}{4} = 3 \]
- Second root: \[ x = \frac{{4 - 8}}{4} = -1 \]
Online Calculators
Several online calculators can solve quadratic equations using the square root method:
Conclusion
Using the square root method to solve quadratic equations is a fundamental technique in algebra. With online calculators, this process is simplified, providing quick and accurate results.
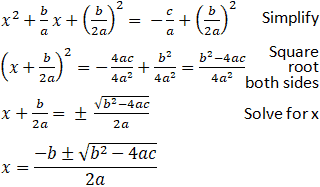
READ MORE:
Introduction to Quadratic Equations
A quadratic equation is a second-order polynomial equation in a single variable \(x\), with the general form:
\[ ax^2 + bx + c = 0 \]
where \(a\), \(b\), and \(c\) are constants, and \(a \neq 0\). Quadratic equations are fundamental in algebra and have a wide range of applications in various fields such as physics, engineering, and economics.
Key Components of Quadratic Equations
- Coefficient \(a\): The quadratic coefficient, which multiplies the \(x^2\) term.
- Coefficient \(b\): The linear coefficient, which multiplies the \(x\) term.
- Coefficient \(c\): The constant term.
Solving Quadratic Equations
There are several methods to solve quadratic equations, including:
- Factoring: Expressing the quadratic equation as a product of two binomials.
- Completing the Square: Rewriting the equation in the form \((x + p)^2 = q\).
- Using the Quadratic Formula: Applying the formula: \[ x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a} \]
- Graphing: Plotting the quadratic function and identifying the points where it intersects the x-axis.
Discriminant and Nature of Roots
The discriminant of a quadratic equation is given by:
\[ \Delta = b^2 - 4ac \]
The value of the discriminant determines the nature of the roots:
- \(\Delta > 0\): Two distinct real roots.
- \(\Delta = 0\): One real root (a repeated root).
- \(\Delta < 0\): Two complex roots.
Example
Consider the quadratic equation \(2x^2 - 4x - 6 = 0\). To solve this using the quadratic formula:
- Identify \(a = 2\), \(b = -4\), and \(c = -6\).
- Calculate the discriminant: \[ \Delta = (-4)^2 - 4(2)(-6) = 16 + 48 = 64 \]
- Since \(\Delta > 0\), there are two real roots.
- Apply the quadratic formula:
\[ x = \frac{4 \pm \sqrt{64}}{4} = \frac{4 \pm 8}{4} \]
- First root: \[ x = \frac{4 + 8}{4} = 3 \]
- Second root: \[ x = \frac{4 - 8}{4} = -1 \]
Thus, the solutions are \(x = 3\) and \(x = -1\).
Quadratic equations are a vital part of algebra, and understanding how to solve them is crucial for students and professionals in many fields.
Steps to Solve Quadratic Equations
Detailed steps to solve quadratic equations using various methods:
1. Using the Quadratic Formula
The quadratic formula is a universal method for solving quadratic equations of the form \( ax^2 + bx + c = 0 \). Follow these steps:
- Ensure the equation is in standard form \( ax^2 + bx + c = 0 \).
- Identify the coefficients \( a \), \( b \), and \( c \).
- Calculate the discriminant: \(\Delta = b^2 - 4ac\).
- Analyze the discriminant:
- \(\Delta > 0\): Two real and distinct roots.
- \(\Delta = 0\): One real root.
- \(\Delta < 0\): Two complex roots.
- Apply the quadratic formula: \[ x = \frac{{-b \pm \sqrt{{b^2 - 4ac}}}}{2a} \]
- Simplify the solutions to find the roots of the equation.
2. Solving by Factoring
This method is applicable when the quadratic equation can be factored easily:
- Rewrite the equation in standard form \( ax^2 + bx + c = 0 \).
- Factor the quadratic expression if possible.
- Set each factor equal to zero.
- Solve the resulting linear equations to find the roots.
3. Completing the Square
This method involves rewriting the quadratic equation in a perfect square form:
- Start with the standard form \( ax^2 + bx + c = 0 \).
- Divide all terms by \( a \) to simplify the equation (if \( a \neq 1 \)).
- Move the constant term to the other side of the equation.
- Take half of the coefficient of \( x \), square it, and add to both sides of the equation.
- Rewrite the left side as a perfect square trinomial.
- Take the square root of both sides.
- Solve for \( x \) by isolating the variable.
4. Solving by Square Root Method
This method is useful for equations that can be written in the form \( ax^2 = k \):
- Ensure the equation is in the form \( ax^2 = k \).
- Isolate \( x^2 \) by dividing both sides by \( a \).
- Take the square root of both sides, considering both the positive and negative roots.
- Solve for \( x \) by isolating the variable.
5. Graphical Method
Using graphs can provide a visual representation of the roots:
- Rewrite the quadratic equation in standard form \( ax^2 + bx + c = 0 \).
- Plot the quadratic function \( y = ax^2 + bx + c \).
- Identify the points where the graph intersects the x-axis. These points are the roots of the equation.
These methods offer various approaches to solve quadratic equations, catering to different types of problems and preferences in solving techniques.
Solving Quadratic Equations by Square Root Method
The square root method is an effective technique for solving quadratic equations that can be written in the form \( ax^2 = c \). This method involves isolating the \( x^2 \) term and then taking the square root of both sides. Here are the detailed steps:
- Start with the quadratic equation in the form \( ax^2 + c = 0 \) or \( ax^2 = c \).
- Isolate the \( x^2 \) term by moving \( c \) to the other side of the equation:
\( ax^2 = -c \) or \( x^2 = \frac{c}{a} \) (if \( a \) is not 1)
- Take the square root of both sides. Remember to include both the positive and negative roots:
\( x = \pm \sqrt{\frac{c}{a}} \)
- Simplify the square root expression, if possible.
Let's look at a few examples:
- Example 1: Solve \( x^2 = 25 \)
- Take the square root of both sides: \( x = \pm \sqrt{25} \)
- Simplify: \( x = \pm 5 \)
So, the solutions are \( x = 5 \) and \( x = -5 \).
- Example 2: Solve \( 2x^2 = 18 \)
- Divide both sides by 2: \( x^2 = 9 \)
- Take the square root of both sides: \( x = \pm \sqrt{9} \)
- Simplify: \( x = \pm 3 \)
So, the solutions are \( x = 3 \) and \( x = -3 \).
- Example 3: Solve \( x^2 - 16 = 0 \)
- Move 16 to the other side: \( x^2 = 16 \)
- Take the square root of both sides: \( x = \pm \sqrt{16} \)
- Simplify: \( x = \pm 4 \)
So, the solutions are \( x = 4 \) and \( x = -4 \).
In conclusion, the square root method is a straightforward way to solve quadratic equations that can be easily manipulated into the form \( x^2 = c \). This method is especially useful when the quadratic equation does not have a linear term (i.e., \( bx \) is missing).
Graphing Quadratic Equations
Graphing quadratic equations helps visualize their solutions and understand their properties. A quadratic equation in the form \( ax^2 + bx + c = 0 \) produces a parabolic graph. Here's a step-by-step guide to graphing quadratic equations:
-
Identify the coefficients:
Given the quadratic equation \( ax^2 + bx + c = 0 \), identify the values of \( a \), \( b \), and \( c \).
-
Find the vertex:
The vertex (h, k) of the parabola can be found using the formula:
\[ h = -\frac{b}{2a} \]
Substitute \( h \) back into the equation to find \( k \):
\[ k = a(h)^2 + b(h) + c \]
-
Determine the axis of symmetry:
The line \( x = h \) is the axis of symmetry for the parabola.
-
Calculate the y-intercept:
Set \( x = 0 \) in the equation to find the y-intercept \( c \).
\[ y = c \]
-
Find the x-intercepts (roots):
Use the quadratic formula to find the x-intercepts, if they exist:
\[ x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a} \]
Analyze the discriminant (\( \Delta = b^2 - 4ac \)) to determine the nature of the roots:
- \( \Delta > 0 \): Two distinct real roots.
- \( \Delta = 0 \): One real root (the vertex touches the x-axis).
- \( \Delta < 0 \): No real roots (the parabola does not intersect the x-axis).
-
Plot key points:
Plot the vertex, axis of symmetry, y-intercept, and x-intercepts (if any). Use additional points if needed to accurately shape the parabola.
-
Draw the parabola:
Connect the points with a smooth, U-shaped curve to complete the graph of the quadratic equation.
Here's an example to illustrate these steps:
- Example: Graph the equation \( y = 2x^2 + 4x - 6 \)
- Identify coefficients: \( a = 2 \), \( b = 4 \), \( c = -6 \)
- Vertex: \( h = -\frac{4}{2(2)} = -1 \), \( k = 2(-1)^2 + 4(-1) - 6 = -8 \)
- Axis of symmetry: \( x = -1 \)
- y-intercept: \( y = -6 \)
- Discriminant: \( \Delta = 4^2 - 4(2)(-6) = 16 + 48 = 64 \) (two real roots)
- x-intercepts: \( x = \frac{-4 \pm \sqrt{64}}{4} = \frac{-4 \pm 8}{4} \rightarrow x = 1 \text{ and } x = -3 \)
- Plot points and draw the parabola through \((-1, -8)\), \((0, -6)\), \((1, 0)\), and \((-3, 0)\).
By following these steps, you can accurately graph any quadratic equation and gain insights into its solutions and characteristics.

Examples
-
Example 1: Real roots
We have the quadratic equation \( x^2 - 4x + 4 = 0 \).
- Identify coefficients: \( a = 1, b = -4, c = 4 \).
- Calculate the discriminant: \( \Delta = (-4)^2 - 4 \cdot 1 \cdot 4 = 0 \).
- Since \( \Delta = 0 \), there is one real root.
- Apply the quadratic formula: \( x = \frac{{-(-4) \pm \sqrt{0}}}{{2 \cdot 1}} = \frac{{4 \pm 0}}{2} = 2 \).
Thus, the equation has a double root at \( x = 2 \).
-
Example 2: Complex roots
Consider the equation \( x^2 + 2x + 5 = 0 \).
- Identify coefficients: \( a = 1, b = 2, c = 5 \).
- Calculate the discriminant: \( \Delta = 2^2 - 4 \cdot 1 \cdot 5 = -16 \).
- Since \( \Delta < 0 \), there are two complex roots.
- Apply the quadratic formula: \( x = \frac{{-2 \pm \sqrt{-16}}}{{2 \cdot 1}} = \frac{{-2 \pm 4i}}{2} = -1 \pm 2i \).
Hence, the roots are \( -1 + 2i \) and \( -1 - 2i \).
Online Quadratic Equation Calculators
-
Symbolab offers a comprehensive quadratic equation calculator that not only solves quadratic equations but also provides step-by-step solutions. It supports both real and complex roots, making it suitable for a wide range of quadratic equations.
-
MathPapa's quadratic equation solver is user-friendly and efficient. It instantly computes solutions upon input, displaying results neatly with explanations where applicable. It's a great tool for quick calculations and learning purposes.
-
Calculator Soup provides a straightforward quadratic formula calculator that computes roots accurately. It's designed for simplicity, offering quick results for standard quadratic equations with clear outputs.
-
Good Calculators features a quadratic equation calculator that handles various forms of quadratic equations. It's particularly useful for educational purposes, as it breaks down solutions step-by-step, aiding in understanding.
Cách tính căn bậc hai của phương trình bậc hai bằng máy tính khoa học.
HƯỚNG DẪN TÍNH CĂN BẬC HAI CỦA PHƯƠNG TRÌNH BẬC HAI BẰNG MÁY TÍNH | Hướng dẫn
READ MORE:
Giải phương trình bậc hai bằng máy tính: Hướng dẫn chi tiết cách sử dụng máy tính để giải phương trình bậc hai.
Giải phương trình bậc hai bằng máy tính