Topic square root with a 3: Discover the fascinating world of the square root with a 3, an essential concept in mathematics. This article explores the properties, calculations, and real-world applications of √3, providing a comprehensive understanding for learners and enthusiasts alike. Join us as we delve into the intriguing details of this irrational number.
Table of Content
- Square Root of 3
- Introduction
- Definition and Representation
- Decimal and Radical Forms
- Rationality of the Square Root of 3
- Calculating the Square Root of 3
- Long Division Method
- Applications in Trigonometry
- Comparison with Other Square Roots
- Special Functions and Continued Fractions
- Importance in Mathematics
- FAQs on the Square Root of 3
- YOUTUBE: Cách tính căn bậc hai của số 3 bởi Kumar Patel và các ứng dụng của nó.
Square Root of 3
The square root of 3 is a significant mathematical constant with numerous applications in various fields. It is represented using the radical symbol as , and its value is approximately 1.732.
Decimal and Exponential Forms
The square root of 3 can be expressed in different forms:
- Decimal form: 1.7320508075688772...
- Radical form:
- Exponential form: 31/2 or 30.5
Rationality
The square root of 3 is an irrational number because its decimal representation is non-terminating and non-repeating. This means it cannot be expressed as a simple fraction.
Finding the Square Root of 3
The value of the square root of 3 can be found using various methods, including the long division method. Here's a step-by-step process:
- Start with the number 3, and represent it as 3.000000...
- Find a number which, when squared, is less than or equal to 3 (in this case, 1).
- Use long division to continue the process, bringing down pairs of zeros and finding successive approximations.
This process results in the value 1.7320508075688772...
Geometric Interpretations
The square root of 3 appears in various geometric contexts, such as:
- The height of an equilateral triangle with side length 2.
- The space diagonal of a unit cube.
- The distance between parallel sides of a regular hexagon with side length 1.
Trigonometric Applications
In trigonometry, the square root of 3 is used in the calculations involving 30-60-90 triangles. For example, in a 30-60-90 triangle, the side opposite the 60-degree angle is times the hypotenuse.
Examples and Problems
- John wonders if - is the same as . The former is a real number, while the latter is imaginary.
- Micheal travels at an average speed of 50 km/hr. The distance covered in 1 hour is 50 x 1.732 = 86.603 km.
- If the area of a circle is 3π square inches, its radius is inches, approximately 1.732 inches.
Additional Notes
The value of is crucial in various mathematical calculations and applications, making it a fundamental constant in geometry, trigonometry, and algebra.

READ MORE:
Introduction
The square root of 3, often represented as , is a fundamental mathematical constant that appears frequently in various fields of mathematics and science. Its approximate value is 1.7320508075688772, and it is an irrational number, meaning its decimal representation is non-terminating and non-repeating. This section explores the properties, calculations, and significance of the square root of 3 in detail.
The square root of 3 can be expressed in multiple forms:
- Decimal form: 1.7320508075688772...
- Radical form:
- Exponent form: 31/2 or 30.5
As an irrational number, the square root of 3 cannot be expressed as a simple fraction. This property makes it essential for various mathematical proofs and calculations. Understanding how to find and use the square root of 3 is crucial for students and professionals working with geometry, algebra, and trigonometry.
In geometry, the square root of 3 is significant in relation to shapes like equilateral triangles and hexagons. For instance, the height of an equilateral triangle with side length 2 is , and the space diagonal of a unit cube is also . These geometric applications highlight the importance of understanding and calculating the square root of 3 accurately.
In trigonometry, the square root of 3 frequently appears in expressions involving trigonometric functions. For example, it is used to calculate the lengths of sides in 30-60-90 triangles and is found in the sines and cosines of various angles.
Overall, the square root of 3 is a versatile and essential mathematical constant, with applications ranging from simple geometric calculations to complex algebraic expressions.
Definition and Representation
The square root of 3 is a mathematical constant that is widely used in various fields. It is the number which, when multiplied by itself, gives the original number 3. This can be written in several forms:
- Decimal form: 1.7320508075688772...
- Radical form:
- Exponent form: 31/2 or 30.5
The square root of 3 is an irrational number, meaning it cannot be expressed as a simple fraction. Its decimal representation is non-terminating and non-repeating, making it essential in various mathematical contexts.
In mathematics, the square root of 3 is denoted by the radical symbol, and it is often encountered in geometric and algebraic applications. The representation of the square root of 3 in different forms allows for flexibility in mathematical calculations and problem-solving.
To further understand this concept, consider the following examples:
- The square root of 3 in decimal form is approximately 1.7320508075688772...
- Using the radical form, it is written as .
- In exponential form, it is represented as 31/2 or 30.5.
These representations are crucial for various mathematical operations and proofs, highlighting the importance of understanding the square root of 3.
Decimal and Radical Forms
The square root of 3 is a significant mathematical constant and can be represented in various forms. The most common representations are the decimal form and the radical form. These forms are essential for different mathematical calculations and applications.
- Decimal Form: The square root of 3 is approximately 1.7320508075688772. This decimal representation is non-terminating and non-repeating, indicating that √3 is an irrational number.
- Radical Form: The square root of 3 is written as . This form is used frequently in algebraic expressions and geometric calculations.
To find the value of the square root of 3, we often use the long division method. This step-by-step process helps in calculating the precise decimal value:
- Write the number 3 as 3.000000, grouping the zeros after the decimal point in pairs.
- Identify a number which, when multiplied by itself, is less than or equal to 3. In this case, the number is 1.
- Divide 3 by 1, placing 1 in the quotient and subtracting to get a remainder of 2.
- Bring down a pair of zeros to make the dividend 200.
- Double the quotient (1) to get 2, and find a number (X) such that 2X × X is less than or equal to 200. Here, X is 7.
- Continue this process, repeating the steps to get a more accurate decimal value of the square root of 3.
Understanding both the decimal and radical forms of √3 is crucial for various mathematical contexts, including geometry, trigonometry, and algebra. These forms allow for precise calculations and facilitate a deeper comprehension of mathematical concepts.
Rationality of the Square Root of 3
The square root of 3 is an irrational number, which means it cannot be expressed as a simple fraction. This property is important in mathematics because it defines the nature of the number and its decimal representation.
The definition of an irrational number is one that has a non-terminating, non-repeating decimal expansion. The square root of 3, approximately 1.7320508075688772, meets this criterion. Here’s a detailed explanation:
- Decimal Representation: The square root of 3 in decimal form is 1.7320508075688772… This expansion continues infinitely without repeating, showcasing its irrational nature.
- Radical Form: In its radical form, it is represented as . This form is crucial for various algebraic manipulations and geometric interpretations.
To further understand the irrationality of the square root of 3, consider the following steps:
- Assume that the square root of 3 is rational. This would mean it could be expressed as a fraction \(\frac{a}{b}\), where \(a\) and \(b\) are integers with no common factors.
- Then, \((\frac{a}{b})^2 = 3\), which implies \(a^2 = 3b^2\).
- This equation shows that \(a^2\) is a multiple of 3, which means \(a\) must also be a multiple of 3. Let \(a = 3k\) for some integer \(k\).
- Substitute \(a = 3k\) into the equation \(a^2 = 3b^2\), resulting in \((3k)^2 = 3b^2\), or \(9k^2 = 3b^2\).
- Divide both sides by 3 to get \(3k^2 = b^2\). This indicates that \(b^2\) is also a multiple of 3, meaning \(b\) must be a multiple of 3.
- Since both \(a\) and \(b\) are multiples of 3, this contradicts the assumption that \(\frac{a}{b}\) is in its simplest form.
Thus, the assumption that the square root of 3 is rational leads to a contradiction, proving that \(\sqrt{3}\) is indeed irrational.
Understanding the irrationality of the square root of 3 is fundamental in higher mathematics, particularly in number theory and various proof techniques.
Calculating the Square Root of 3
The square root of 3 (√3) is an irrational number, which means it cannot be expressed exactly as a simple fraction. Its approximate value is 1.732. One common method to calculate the square root of 3 is the long division method. Here are the detailed steps to perform this calculation:
Long Division Method
Write down the number 3 and pair its digits from right to left. Since 3 has only one digit, you can consider it as 3.000000 for simplicity.
Find a number (y) such that y² is less than or equal to 3. The largest such number is 1 because 1² = 1 and 2² = 4 (which is greater than 3).
Subtract the square of this number from the given number: 3 - 1² = 3 - 1 = 2.
Bring down a pair of zeros next to the remainder to get 200.
Double the divisor (which is 1), and write it down with a blank digit next to it: 2_.
Find a digit (x) to fill the blank such that (2x * x) is less than or equal to 200. In this case, the digit is 7 because 27 * 7 = 189, and the next digit (8) would result in a product greater than 200.
Write down 7 in the quotient and subtract 189 from 200 to get 11. Bring down the next pair of zeros to get 1100.
Repeat the process: double the current divisor (27), making it 54, and find the next digit (x) such that (54x * x) is less than or equal to 1100. The digit is 2 because 542 * 2 = 1084.
Write down 2 in the quotient and subtract 1084 from 1100 to get 16. Bring down the next pair of zeros to get 1600.
Continue this process to get more decimal places. The quotient obtained through this process gives the square root of 3.
Following these steps, the approximation of the square root of 3 is found to be 1.732, accurate up to several decimal places.
Example Calculation
Let's go through a few steps of this method:
Initial number: 3.000000
1 is the largest number whose square is less than 3.
Subtract 1² (1) from 3 to get 2. Bring down 00 to make it 200.
Double the divisor (1) to get 2_. Find x such that (2x * x) ≤ 200. x = 7, because 27 * 7 = 189.
Write 7 in the quotient, making it 1.7. Subtract 189 from 200 to get 11. Bring down 00 to make it 1100.
Double the current divisor (27) to get 54_. Find x such that (54x * x) ≤ 1100. x = 2, because 542 * 2 = 1084.
Write 2 in the quotient, making it 1.72. Subtract 1084 from 1100 to get 16. Bring down 00 to make it 1600.
Continuing this process provides a more accurate value of the square root of 3.
The long division method is a systematic way to find the square root of both perfect and imperfect squares, and it can be extended to find the value to as many decimal places as needed.
Long Division Method
The long division method is a systematic way to find the square root of a number. Here are the steps to find the square root of 3 using this method:
- Step 1: Start by setting up the number 3 under the long division bar. Pair the digits from right to left. Since 3 is a single digit, we consider it as 3.000000 for the division.
- Step 2: Find the largest number whose square is less than or equal to 3. The largest number is 1 because 12 = 1.
- Step 3: Write 1 as the divisor and quotient. Subtract 12 (which is 1) from 3 to get the remainder 2. Bring down two zeros to make it 200.
- Step 4: Double the quotient (which is 1) to get 2, and place it as the new divisor's ten's place.
- Step 5: Determine a digit X such that 2X * X ≤ 200. The largest such digit is 7 because 27 * 7 = 189.
- Step 6: Place 7 in the quotient and subtract 189 from 200 to get 11. Bring down two more zeros to make it 1100.
- Step 7: Double the current quotient (which is 17) to get 34. Determine a digit Y such that 34Y * Y ≤ 1100. The largest such digit is 3 because 343 * 3 = 1029.
- Step 8: Place 3 in the quotient and subtract 1029 from 1100 to get 71. Bring down two more zeros to make it 7100.
- Step 9: Continue this process, doubling the current quotient, determining the new digit, and bringing down pairs of zeros until you reach the desired level of accuracy.
Following these steps, we find that the square root of 3 is approximately 1.732050807568877...
Here is a summary of the steps in a table for clarity:
| Step | Action | Quotient | Divisor | Remainder |
|---|---|---|---|---|
| 1 | Initial Setup | 3.000000 | ||
| 2 | 1 * 1 = 1 | 1 | 1 | 2 |
| 3 | 200 - 189 = 11 | 1.7 | 27 | 11 |
| 4 | 1100 - 1029 = 71 | 1.73 | 343 | 71 |
| 5 | Continue process... | 1.732 |
This method helps in achieving the square root of non-perfect squares like 3 accurately up to the desired decimal places.
Applications in Trigonometry
The square root of 3, approximately equal to 1.732, is a significant value in trigonometry. It often appears in various trigonometric identities, functions, and problems. Here are some key applications:
- Special Angles: The square root of 3 appears in the trigonometric values of special angles. For example:
- For a 60° angle:
\(\sin 60^\circ = \frac{\sqrt{3}}{2}\)
\(\tan 60^\circ = \sqrt{3}\)
- For a 30° angle:
\(\cos 30^\circ = \frac{\sqrt{3}}{2}\)
\(\cot 30^\circ = \sqrt{3}\)
- For a 60° angle:
- Equilateral Triangles: In an equilateral triangle with side length \(a\):
- The height (altitude) is \(\frac{\sqrt{3}}{2}a\).
- The area is \(\frac{\sqrt{3}}{4}a^2\).
- Trigonometric Identities: The square root of 3 is used in various trigonometric identities. For instance:
- \(\tan 60^\circ = \frac{\sin 60^\circ}{\cos 60^\circ} = \frac{\frac{\sqrt{3}}{2}}{\frac{1}{2}} = \sqrt{3}\)
- \(\sin 60^\circ = \cos 30^\circ = \frac{\sqrt{3}}{2}\)
- Law of Sines and Cosines: The square root of 3 is also present in the Law of Sines and the Law of Cosines when dealing with specific angles and triangle properties.
Understanding these applications is essential for solving many trigonometric problems. The square root of 3 often simplifies complex expressions and aids in finding solutions to geometric and trigonometric challenges.
Comparison with Other Square Roots
The square root of 3, denoted as \(\sqrt{3}\), is an important irrational number in mathematics. Comparing it with other square roots helps in understanding its relative magnitude and applications. Here, we will compare \(\sqrt{3}\) with \(\sqrt{2}\), \(\sqrt{5}\), and other notable square roots.
Square Root of 2
The square root of 2, approximately 1.414, is smaller than the square root of 3. Both numbers are irrational, but they have different applications in geometry and trigonometry.
- \(\sqrt{2} \approx 1.414\)
- \(\sqrt{3} \approx 1.732\)
- Comparison: \(\sqrt{2} < \sqrt{3}\)
Square Root of 5
The square root of 5, approximately 2.236, is larger than the square root of 3. This comparison helps in understanding the growth of square roots as the number inside the radical increases.
- \(\sqrt{3} \approx 1.732\)
- \(\sqrt{5} \approx 2.236\)
- Comparison: \(\sqrt{3} < \sqrt{5}\)
Square Root of 4
The square root of 4 is exactly 2, which is an integer and larger than the square root of 3. This comparison highlights the difference between irrational and rational square roots.
- \(\sqrt{3} \approx 1.732\)
- \(\sqrt{4} = 2\)
- Comparison: \(\sqrt{3} < \sqrt{4}\)
Table of Common Square Roots
| Number | Square Root | Approximate Value |
|---|---|---|
| 2 | \(\sqrt{2}\) | 1.414 |
| 3 | \(\sqrt{3}\) | 1.732 |
| 4 | \(\sqrt{4}\) | 2.000 |
| 5 | \(\sqrt{5}\) | 2.236 |
| 6 | \(\sqrt{6}\) | 2.449 |
| 7 | \(\sqrt{7}\) | 2.646 |
| 8 | \(\sqrt{8}\) | 2.828 |
In summary, the square root of 3 is an important irrational number that lies between the square root of 2 and the square root of 4. Understanding its position relative to other square roots is valuable for various mathematical applications.
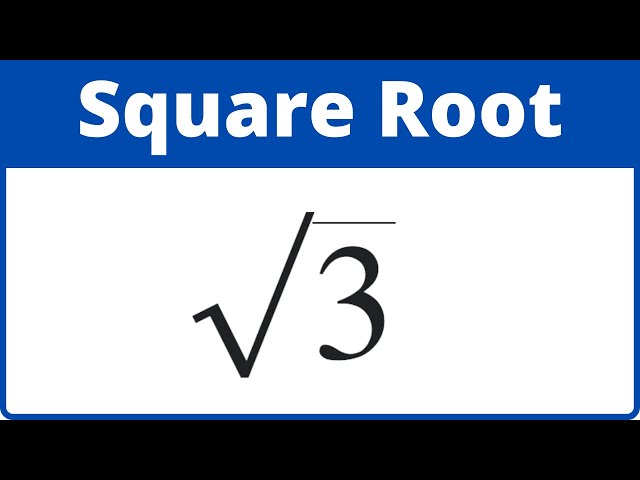
Special Functions and Continued Fractions
The square root of 3 (\(\sqrt{3}\)) has interesting representations in terms of special functions and continued fractions. These mathematical tools provide insights and approximations for \(\sqrt{3}\) that are useful in various contexts.
Special Functions
One way to approximate \(\sqrt{3}\) is through binomial expansions and iterative methods. For instance, using the binomial expansion, we can approximate \(\sqrt{3}\) as follows:
\[
\sqrt{3} = (1 + 2)^{1/2} \approx 1 + \frac{1}{2}(2) - \frac{1}{8}(2^2) + \frac{1}{16}(2^3) - \cdots
\]
Each term in the expansion adds a higher level of precision, converging quickly to the actual value of \(\sqrt{3}\).
Continued Fractions
Continued fractions provide another way to represent and approximate \(\sqrt{3}\). The continued fraction for \(\sqrt{3}\) is given by:
\[
\sqrt{3} = [1; \overline{1, 2}]
\]
This means that the continued fraction for \(\sqrt{3}\) is periodic and can be written as:
\[
\sqrt{3} = 1 + \cfrac{1}{1 + \cfrac{1}{2 + \cfrac{1}{1 + \cfrac{1}{2 + \cfrac{1}{1 + \cfrac{1}{2 + \cdots}}}}}}
\]
To compute the convergents of this continued fraction, we use the recurrence relations:
\[
P_k = a_k P_{k-1} + P_{k-2}
\]
\[
Q_k = a_k Q_{k-1} + Q_{k-2}
\]
where \(P_k\) and \(Q_k\) are the numerator and denominator of the k-th convergent, and \(a_k\) are the coefficients from the continued fraction. For \(\sqrt{3}\), the coefficients \(a_k\) are 1 and 2, alternating. The initial values are \(P_{-2} = 0\), \(P_{-1} = 1\), \(Q_{-2} = 1\), and \(Q_{-1} = 0\).
Some of the first few convergents for \(\sqrt{3}\) are:
- \(\frac{1}{1}\)
- \(\frac{2}{1}\)
- \(\frac{5}{3}\)
- \(\frac{7}{4}\)
- \(\frac{19}{11}\)
- \(\frac{26}{15}\)
Each convergent is a better approximation of \(\sqrt{3}\), with the sequence converging to the true value.
Matrix Representation
Another approach involves using matrix methods to approximate \(\sqrt{3}\). The matrix method uses the key matrix \(\mathbf{M} = \begin{pmatrix} 1 & 1 \\ 2 & 3 \end{pmatrix}\). Repeatedly squaring this matrix provides progressively better approximations of \(\sqrt{3}\). Applying \(\mathbf{M}^n\) to the initial vector \(\begin{pmatrix} 0 \\ 1 \end{pmatrix}\) gives a vector whose components can be used to form a good approximation of \(\sqrt{3}\).
For example, starting with:
\[
\mathbf{M}^1 = \begin{pmatrix} 1 & 1 \\ 2 & 3 \end{pmatrix}
\]
The approximation from \(\mathbf{M}^1\) is \(\frac{5}{3} \approx 1.6667\).
Further squaring the matrix gives better approximations:
\[
\mathbf{M}^2 = \begin{pmatrix} 7 & 10 \\ 20 & 29 \end{pmatrix}
\]
The approximation from \(\mathbf{M}^2\) is \(\frac{29}{17} \approx 1.7059\), which is closer to the actual value of \(\sqrt{3}\).
These methods showcase the richness of mathematical techniques available for approximating irrational numbers like \(\sqrt{3}\).
Importance in Mathematics
The square root of 3 is a significant number in various branches of mathematics, particularly due to its irrationality and its frequent appearance in geometric contexts and algebraic structures.
Here are some key areas where the square root of 3 plays an important role:
- Geometry: The square root of 3 is a critical component in the geometry of equilateral triangles and 30-60-90 triangles. In a 30-60-90 triangle, the sides are in the ratio 1 : √3 : 2. This ratio is fundamental in many geometric constructions and proofs.
- Trigonometry: The square root of 3 appears in trigonometric functions, especially those involving 30° and 60° angles. For example, the tangent of 60° is √3, and the sine of 60° is (√3)/2. These values are essential in solving various trigonometric equations and problems.
- Algebra: In algebra, the square root of 3 is often encountered when solving quadratic equations. For instance, the solutions to the equation \(x^2 - 3 = 0\) are \(x = ±√3\).
- Number Theory: The irrationality of the square root of 3 is a significant topic in number theory. Proving that √3 is irrational demonstrates that it cannot be expressed as a simple fraction, which has implications for the study of rational and irrational numbers.
- Applications in Real Life: The square root of 3 is used in various practical applications, such as calculating distances in two-dimensional and three-dimensional spaces using the Pythagorean theorem. It's also used in engineering, physics, and computer graphics to perform accurate calculations.
To summarize, the square root of 3 is a fundamental mathematical constant that appears in diverse mathematical disciplines, highlighting its importance and utility in both theoretical and applied mathematics.
FAQs on the Square Root of 3
-
What is the square root of 3?
The square root of 3 is approximately \( \sqrt{3} \approx 1.732 \). This value is an irrational number, meaning its decimal representation is non-terminating and non-repeating.
-
Is the square root of 3 a rational or irrational number?
The square root of 3 is an irrational number because it cannot be expressed as a ratio of two integers. Its decimal form goes on forever without repeating.
-
How can you calculate the square root of 3?
There are several methods to calculate the square root of 3, including the long division method and the use of a calculator. For manual calculations, the long division method is often used to find an approximate value.
-
What are some applications of the square root of 3 in mathematics?
The square root of 3 appears in various mathematical contexts, including geometry (calculating the height of an equilateral triangle), trigonometry (appearing in sine and cosine of 60 degrees), and algebra (solving quadratic equations).
-
Can the square root of 3 be simplified further?
No, \( \sqrt{3} \) is already in its simplest radical form and cannot be simplified further.
-
What is the significance of the square root of 3 in real-world applications?
The square root of 3 is significant in real-world applications such as engineering, physics, and architecture. It is used in calculations involving triangular shapes and in determining the dimensions of certain geometric figures.
-
How does the square root of 3 compare to other square roots?
Compared to other square roots, \( \sqrt{3} \) is unique because it is an irrational number and has a specific value that is frequently encountered in various mathematical problems. For instance, it is larger than \( \sqrt{2} \approx 1.414 \) but smaller than \( \sqrt{4} = 2 \).
-
What are some properties of the square root of 3?
Properties of \( \sqrt{3} \) include its irrationality, its appearance in the solutions of quadratic equations, and its role in the geometric properties of equilateral triangles and hexagons.
-
Can you express the square root of 3 in exponential form?
Yes, the square root of 3 can be expressed in exponential form as \( 3^{1/2} \) or \( 3^{0.5} \).
-
How can you represent the square root of 3 geometrically?
Geometrically, \( \sqrt{3} \) can be represented as the height of an equilateral triangle with side length 2, or as the distance between two opposite vertices of a regular hexagon with unit side length.
Cách tính căn bậc hai của số 3 bởi Kumar Patel và các ứng dụng của nó.
Cách tính căn bậc hai của số 3 bởi Kumar Patel | Video hướng dẫn
READ MORE:
Căn bậc ba - Harold & Kumar
Căn bậc ba - Harold & Kumar