Topic square root zero: The square root of zero is a unique and fascinating mathematical concept. In this article, we will explore its properties, significance in various mathematical contexts, and its applications in geometry. By understanding the square root of zero, we can gain deeper insights into fundamental principles of mathematics.
Table of Content
- Square Root of Zero
- Introduction to Square Root
- Understanding the Square Root of Zero
- Mathematical Explanation
- Properties of Square Roots
- Applications of Square Roots in Geometry
- Perfect Squares and Square Roots
- Principal Square Root
- Square Roots in Different Mathematical Contexts
- Calculating Square Roots
- Common Misconceptions
- Geometric Construction of Square Roots
- YOUTUBE: Xem video này để hiểu về căn bậc hai của số không và những điều cơ bản về nó.
Square Root of Zero
The square root of zero is a fundamental mathematical concept often encountered in various branches of mathematics and science. The square root function is the inverse operation of squaring a number. When we square a number, we multiply it by itself. The square root of a number is the value that, when multiplied by itself, gives the original number.
Mathematical Definition
The square root of zero is expressed mathematically as:
In exponential form, it can be represented as:
\(0^{1/2} = 0\)
This is because any number raised to the power of zero is zero itself:
\(0^2 = 0\)
Explanation
To understand this, consider the definition of a square root. The square root of a number is a value that, when multiplied by itself, results in the original number. For zero:
\(x \times x = 0\)
Here, \(x\) must be zero because any non-zero number squared will not result in zero.
Properties and Applications
- The square root of zero is always zero, regardless of the mathematical context (real numbers, complex numbers, etc.).
- In linear algebra, a zero determinant indicates a singular matrix, which has implications in solving linear equations and matrix operations.
- In calculus, the square root function is continuous and differentiable, with the derivative of \(\sqrt{x}\) at \(x = 0\) being undefined because it involves division by zero.
Examples in Mathematics
Here are a few contexts where the square root of zero appears:
- Basic Arithmetic: Simplifying expressions like \(\sqrt{0} = 0\).
- Algebra: Solving equations involving square roots where one of the solutions may be zero.
- Calculus: Finding limits and continuity at points where the function involves the square root of zero.
Conclusion
The concept of the square root of zero is simple yet crucial in understanding more complex mathematical theories and applications. It serves as a foundational element in arithmetic, algebra, calculus, and beyond.

READ MORE:
Introduction to Square Root
The concept of the square root is fundamental in mathematics, representing a value that, when multiplied by itself, yields the original number. For any non-negative number \(x\), the square root is denoted as \(\sqrt{x}\). Here are some key points about square roots:
- The square root of a positive number has two values: a positive and a negative root. For example, \(\sqrt{4}\) is both 2 and -2 because \(2^2 = 4\) and \((-2)^2 = 4\).
- The square root of zero is unique and equal to zero. Mathematically, \(\sqrt{0} = 0\).
- Square roots are used extensively in various fields such as geometry, physics, and engineering to solve equations and understand relationships between quantities.
Understanding square roots involves recognizing their properties and how they function within different mathematical contexts. Below is a table illustrating some common square roots:
| Number | Square Root |
|---|---|
| 0 | 0 |
| 1 | 1 |
| 4 | 2 |
| 9 | 3 |
| 16 | 4 |
The square root function is essential in solving quadratic equations, optimizing functions, and analyzing geometric shapes. It helps simplify complex expressions and provides insights into the nature of numbers.
Understanding the Square Root of Zero
The square root of zero (\(\sqrt{0}\)) is a simple yet intriguing concept in mathematics. It is defined as the number which, when multiplied by itself, gives zero. Let's break down the understanding step by step:
- Definition: The square root of a number \(x\) is a number \(y\) such that \(y^2 = x\). For zero, this means finding a number \(y\) where \(y^2 = 0\).
- Unique Solution: In the case of zero, there is only one number that satisfies this equation: zero itself. Therefore, \(\sqrt{0} = 0\).
- Mathematical Representation: Using Mathjax, we can represent this as:
\[\sqrt{0} = 0\]
- Properties: Understanding the properties of zero helps in grasping its square root:
- Additive Identity: Zero is the additive identity, meaning any number plus zero remains unchanged: \(a + 0 = a\).
- Multiplicative Property: Any number multiplied by zero equals zero: \(a \times 0 = 0\).
- Applications: The concept of zero and its square root is fundamental in various areas of mathematics and its applications:
- Solving Equations: In quadratic equations, the square root of zero can be a potential solution when the discriminant is zero.
- Geometry: In geometric interpretations, zero often represents a point or an absence of dimension.
Understanding the square root of zero lays the foundation for more complex mathematical concepts and helps in simplifying various mathematical expressions and problems.
Mathematical Explanation
The square root of a number \( x \), denoted as \( \sqrt{x} \), is a value that, when multiplied by itself, gives \( x \). Specifically, for zero, the square root \( \sqrt{0} \) is defined as a non-negative number \( y \) such that \( y \times y = 0 \).
In mathematical terms:
- \( \sqrt{0} = 0 \) because \( 0 \times 0 = 0 \).
Zero is unique in that its square root is itself, which is unlike any other real number where positive and negative square roots exist. This uniqueness arises because zero is the only number that, when squared, remains zero.
Furthermore, the square root function \( \sqrt{x} \) is typically defined only for non-negative real numbers. Hence, \( \sqrt{0} \) conventionally refers to the non-negative square root, which is 0.
Properties of Square Roots
Understanding the properties of square roots provides insights into their behavior and applications:
- Non-negative Values: The square root \( \sqrt{x} \) of a non-negative number \( x \geq 0 \) is always non-negative.
- Principal Square Root: For any positive number \( x > 0 \), \( \sqrt{x} \) denotes the principal (non-negative) square root.
- Squaring: Squaring both sides of \( \sqrt{x} = y \) gives \( x = y^2 \).
- Product Property: \( \sqrt{ab} = \sqrt{a} \cdot \sqrt{b} \) for non-negative real numbers \( a \) and \( b \).
- Quotient Property: \( \frac{\sqrt{a}}{\sqrt{b}} = \sqrt{\frac{a}{b}} \) for \( b > 0 \).
- Zero: \( \sqrt{0} = 0 \).
- Negative Numbers: The square root \( \sqrt{x} \) is not defined for negative real numbers \( x \).
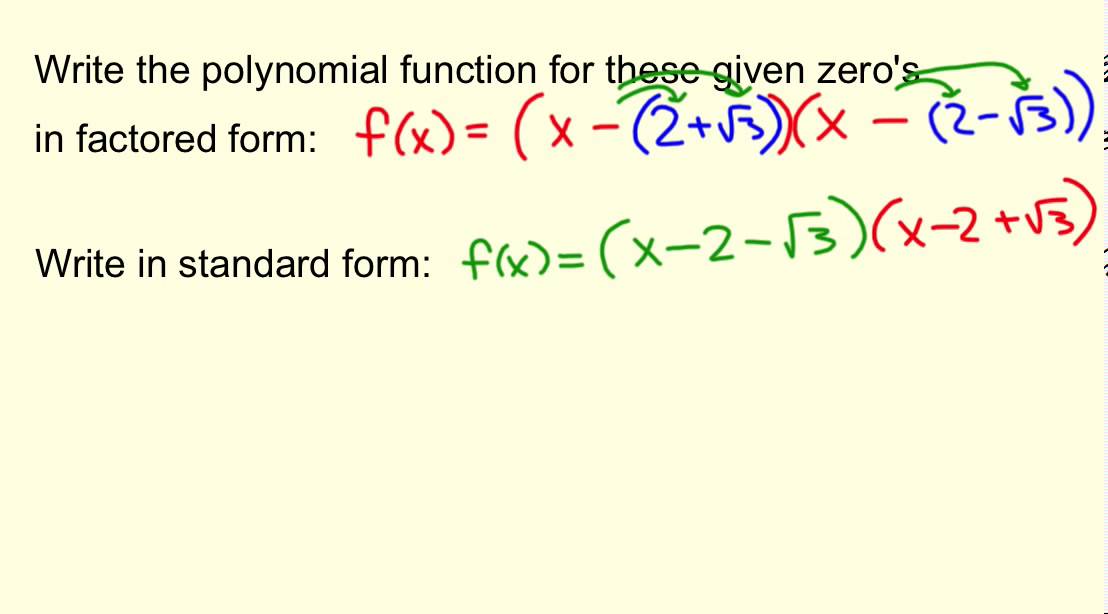
Applications of Square Roots in Geometry
Square roots are integral in various geometric contexts, contributing to both theoretical understanding and practical calculations:
- Distance Calculation: In Euclidean geometry, the distance between two points \( (x_1, y_1) \) and \( (x_2, y_2) \) is given by \( \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2} \).
- Area of Squares: The side length \( s \) of a square is related to its area \( A \) by \( s = \sqrt{A} \).
- Pythagorean Theorem: In right triangles, the theorem states \( a^2 + b^2 = c^2 \), where \( c \) is the hypotenuse length, hence \( c = \sqrt{a^2 + b^2} \).
- Circle Properties: The radius \( r \) of a circle is connected to its area \( A \) by \( r = \sqrt{\frac{A}{\pi}} \).
- Volume of Cubes and Cuboids: The side length \( s \) of a cube or the dimensions of a cuboid are determined by cube roots, extending the concept of square roots in three dimensions.
Perfect Squares and Square Roots
Perfect squares and their square roots play a fundamental role in mathematics, offering insights into both arithmetic and geometric contexts:
- Definition: A perfect square is a number that can be expressed as \( n^2 \), where \( n \) is an integer.
- Examples: Examples of perfect squares include \( 1 \) (\( 1^2 \)), \( 4 \) (\( 2^2 \)), \( 9 \) (\( 3^2 \)), \( 16 \) (\( 4^2 \)), etc.
- Square Roots: The square root of a perfect square \( n^2 \) is \( n \).
- Properties:
- The product of two perfect squares \( (m^2)(n^2) \) is also a perfect square \( (mn)^2 \).
- The square root of a product is the product of the square roots: \( \sqrt{mn} = \sqrt{m} \cdot \sqrt{n} \).
- The square root function \( \sqrt{x} \) is defined for non-negative real numbers.
- Applications: Perfect squares and square roots are used in various fields including algebra, geometry, physics, and computer science.
Principal Square Root
The principal square root refers to the non-negative square root of a non-negative real number:
- Definition: For a non-negative number \( x \geq 0 \), the principal square root \( \sqrt{x} \) is the non-negative number \( y \) such that \( y^2 = x \).
- Symbol: It is denoted as \( \sqrt{x} \).
- Properties:
- For \( x \geq 0 \), \( \sqrt{x} \geq 0 \).
- The principal square root is a specific value among the possible square roots of \( x \).
- Examples include \( \sqrt{9} = 3 \) (since \( 3^2 = 9 \)) and \( \sqrt{25} = 5 \) (since \( 5^2 = 25 \)).
- Usage: In mathematics and everyday applications, the principal square root is often used to find the length of sides of squares, calculate distances, and solve quadratic equations.
Square Roots in Different Mathematical Contexts
Square roots are utilized across various branches of mathematics, each with unique applications and implications:
- Algebra: In algebra, square roots are essential for solving quadratic equations and understanding the properties of polynomials.
- Geometry: In geometry, square roots are used to calculate distances, find side lengths of squares and rectangles, and explore relationships in triangles (like in the Pythagorean theorem).
- Analysis: In mathematical analysis, square roots are employed in the study of limits, continuity, and differentiation.
- Numerical Methods: Square roots are crucial in numerical methods for solving equations, interpolation, and optimization problems.
- Physics: In physics, square roots are encountered in formulas related to energy, acceleration, and waves.
- Computer Science: Square roots are used in various algorithms, especially in computational geometry, graphics, and simulations.
- Probability and Statistics: Square roots appear in statistical formulas, such as calculating standard deviations and root mean squares.

Calculating Square Roots
To calculate the square root of a number, you can use several methods:
- Estimation Method: Approximate the square root based on known perfect squares.
- Prime Factorization Method: Express the number as a product of prime factors and simplify.
- Newton's Method: Use an iterative approach to converge on the square root.
Here's a step-by-step example using Newton's Method:
| Step 1: | Start with an initial guess, \( x_0 \). |
| Step 2: | Improve the guess using the formula: \( x_{n+1} = \frac{1}{2} \left( x_n + \frac{a}{x_n} \right) \), where \( a \) is the number whose square root is being calculated. |
| Step 3: | Repeat Step 2 until the difference between \( x_{n+1} \) and \( x_n \) is within an acceptable tolerance level. |
This method and others provide various approaches to find the square root of a number efficiently.
Common Misconceptions
There are several misconceptions about the square root of zero:
- Zero is its own square root: Some believe that the square root of zero is zero itself, but in reality, both \( 0 \) and \( -0 \) are considered square roots of zero.
- Only positive square roots exist: While in many contexts we consider the principal (positive) square root, every non-negative real number has both a positive and a negative square root.
- Imaginary square roots for zero: It's sometimes misunderstood that zero has imaginary square roots. In fact, zero's square roots are purely real numbers.
- Square root of zero is undefined: Contrary to this misconception, the square root of zero is well-defined and equals zero.
Understanding these misconceptions clarifies the nature of square roots, especially when dealing with zero.
Geometric Construction of Square Roots
Geometrically, the square root of a number can be constructed using the following method:
- Draw a Unit Square: Start with a square where each side measures 1 unit.
- Construct the Diagonal: Draw a diagonal that connects one corner of the square to the opposite corner.
- Measure the Diagonal: The length of this diagonal is the square root of 2 units.
This geometric construction demonstrates visually how the square root of a number corresponds to the length of a diagonal in a square.
Xem video này để hiểu về căn bậc hai của số không và những điều cơ bản về nó.
What is the square root of zero? | Video Title in Vietnamese
READ MORE:
Xem video này để khám phá bí ẩn của căn bậc không và các khái niệm liên quan.
The Mystery Of The 0th Root | Bí ẩn của căn bậc không