Topic square root 7 times square root 7: The product of the square root of 7 and the square root of 7 is a fundamental mathematical operation that simplifies to the number 7. Understanding this concept is essential for various algebraic applications, including solving equations and simplifying expressions. This article delves into the properties and significance of this operation, providing clear examples and practical applications.
Table of Content
- Understanding the Multiplication of Square Roots
- Introduction to Square Roots
- Properties of Square Roots
- Calculating Square Roots
- Example: Calculating √7 Using Long Division
- Understanding the Square Root of 7
- Mathematical Representation
- Calculation Methods
- Perfect and Imperfect Squares
- Long Division Method
- Practical Applications and Examples
- FAQs on Square Roots
- YOUTUBE:
Understanding the Multiplication of Square Roots
The multiplication of two square roots follows specific mathematical rules. In this case, we will explore the multiplication of the square root of 7 by itself.
Mathematical Explanation
To multiply the square root of 7 by itself, we can use the product rule for radicals:
\[
\sqrt{7} \times \sqrt{7}
\]
According to the product rule, we can combine the radicals:
\[
\sqrt{7 \times 7}
\]
This simplifies to:
\[
\sqrt{49}
\]
Since the square root of 49 is 7, we have:
\[
\sqrt{7} \times \sqrt{7} = 7
\]
Steps to Calculate the Square Root
- Combine the two radicals using the product rule.
- Multiply the numbers under the radicals.
- Simplify the resulting radical if possible.
Long Division Method for Finding Square Roots
The square root of a number can also be found using the long division method. Here is how you can find the square root of 7:
- Write the number 7 in decimal form as 7.0000.
- Find a number whose square is less than or equal to 7. In this case, 2x2=4, which is less than 7.
- Subtract 4 from 7 to get a remainder of 3 and a quotient of 2.
- Double the quotient to get 4 and use it as the new divisor.
- Bring down two zeros to make 300 the new dividend.
- Find a number (n) such that (40+n) x n is less than or equal to 300. Here, 46 x 6 = 276, which is less than 300.
- Subtract 276 from 300 to get a remainder of 24 and a quotient of 26.
- Repeat the process to find the value of the square root up to three decimal places. The value of the square root of 7 is approximately 2.645.
Example Problem
Consider simplifying the expression: 2√7 + 5√7.
Solution:
\[
2\sqrt{7} + 5\sqrt{7} = 7\sqrt{7}
\]
Conclusion
By understanding and applying these rules, we can accurately multiply and simplify square roots, as well as find their values using methods such as long division.

READ MORE:
Introduction to Square Roots
Square roots are mathematical operations that determine what number, when multiplied by itself, equals the original number. The concept of square roots is fundamental in various fields of mathematics and is essential for solving quadratic equations, geometry problems, and more. For example, the square root of 7 is denoted as √7, and its approximate value is 2.645.
Properties of Square Roots
- Radical Form: The square root of 7 is written as √7.
- Decimal Form: The approximate value of √7 is 2.645.
- Irrational Number: Since 7 is not a perfect square, √7 is an irrational number, meaning it cannot be expressed as a simple fraction.
Calculating Square Roots
There are various methods to calculate square roots, including:
- Prime Factorization: This method involves breaking down the number into its prime factors and simplifying the radical expression.
- Long Division Method: This is a step-by-step approach to find the square root of non-perfect squares to a desired level of accuracy.
Example: Calculating √7 Using Long Division
To calculate √7 using the long division method, follow these steps:
- Write the number 7 in decimal form as 7.0000.
- Find a number whose square is less than or equal to 7. In this case, 2^2 = 4.
- Subtract 4 from 7 to get the remainder 3. Bring down a pair of zeros to get 300.
- Double the quotient (2) to get 4, and use it as the new divisor (40).
- Find a number (x) such that (40 + x) * x is less than or equal to 300. Here, x is 6, because 46 * 6 = 276.
- Subtract 276 from 300 to get the remainder 24. Bring down another pair of zeros to get 2400.
- Repeat the process to get more decimal places. After several iterations, you get √7 ≈ 2.645.
The process can be continued to achieve the desired level of precision. The value obtained, 2.645, is a close approximation of the square root of 7.
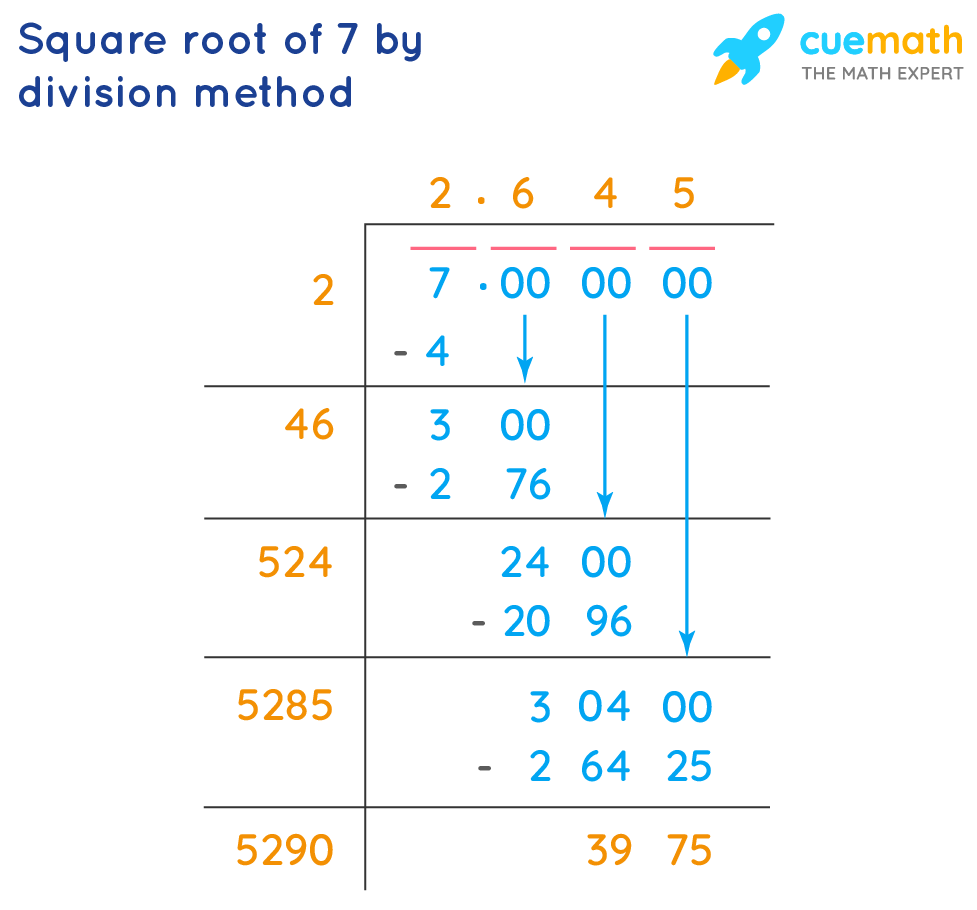
Understanding the Square Root of 7
The square root of 7, denoted as \( \sqrt{7} \), is an irrational number. It cannot be expressed as a simple fraction and has a non-repeating, non-terminating decimal expansion. The approximate value of \( \sqrt{7} \) is 2.645.
To find the square root of 7, we use the long division method:
- Write the number 7 as 7.0000.
- Find the largest number whose square is less than or equal to 7. In this case, 2 × 2 = 4.
- Subtract 4 from 7, giving a remainder of 3. Bring down two zeros, making the new number 300.
- Double the quotient (2), resulting in 4. Determine a number (x) such that (40 + x) × x ≤ 300. Here, 46 × 6 = 276.
- Subtract 276 from 300 to get a remainder of 24. Bring down the next pair of zeros, resulting in 2400.
- Continue this process to approximate the value of \( \sqrt{7} \) to three decimal places as 2.645.
Mathematically, the positive and negative square roots of 7 are represented as \( \sqrt{7} \) and \( -\sqrt{7} \). This means both \( \sqrt{7} \times \sqrt{7} = 7 \) and \( -\sqrt{7} \times -\sqrt{7} = 7 \).
Here is a simple representation of the value:
| \( \sqrt{7} \) | \( \approx 2.645 \) |
Understanding square roots is crucial in various mathematical applications, including solving equations and understanding geometrical concepts.
Mathematical Representation
In mathematics, the square root of a number is a value that, when multiplied by itself, gives the original number. For instance, the square root of 7, denoted as \( \sqrt{7} \), can be represented and calculated through various methods. Below is a step-by-step explanation of these methods using MathJax for mathematical clarity.
Product Rule for Radicals
The product of two square roots is equal to the square root of the product of the numbers. This can be expressed as:
\[ \sqrt{a} \times \sqrt{b} = \sqrt{a \times b} \]
For the square root of 7 times the square root of 7, it can be simplified as:
\[ \sqrt{7} \times \sqrt{7} = \sqrt{7 \times 7} = \sqrt{49} = 7 \]
Exponential Representation
Square roots can also be expressed using exponents. The square root of a number \( a \) is equivalent to raising \( a \) to the power of \( \frac{1}{2} \). Thus, the square root of 7 can be written as:
\[ \sqrt{7} = 7^{\frac{1}{2}} \]
Multiplying the square root of 7 by itself using exponents gives:
\[ 7^{\frac{1}{2}} \times 7^{\frac{1}{2}} = 7^{1} = 7 \]
Decimal and Fractional Forms
The decimal form of the square root of 7 is approximately 2.645. Therefore:
\[ \sqrt{7} \approx 2.645 \]
Multiplying this value by itself gives:
\[ 2.645 \times 2.645 \approx 7 \]
Long Division Method
Another precise way to calculate the square root of a number is the long division method. Here is a brief outline of the steps involved:
- Write the number 7 as 7.0000 to determine the root value up to 3 decimal places.
- Find a number whose square is less than or equal to 7. The closest value is 2, as \( 2^2 = 4 \).
- Subtract 4 from 7, giving a remainder of 3. Bring down a pair of zeros to get 300.
- Double the quotient (2) to get 4. Find a digit that, when appended to 4, forms a new divisor that goes into 300. The closest digit is 6, as \( 46 \times 6 = 276 \).
- Continue this process to refine the quotient to 2.645.
Binomial Expansion Method
Binomial expansion can also be used to approximate the square root of a number. For small values of x, the binomial expansion is:
\[ (1 + x)^{\frac{1}{2}} \approx 1 + \frac{x}{2} \]
For the square root of 7, using x = 0.0015625, the expansion provides a close approximation to the value 2.645.
Conclusion
Through these methods, the square root of 7, whether represented using radicals, exponents, decimals, or through long division, consistently yields the value 7 when multiplied by itself, demonstrating its fundamental mathematical properties.
Calculation Methods
The calculation of the square root of 7 times the square root of 7 can be approached in several ways. Here, we'll cover the product rule for radicals, the long division method, and some additional insights.
Product Rule for Radicals
To multiply the square root of 7 by itself, we use the product rule for radicals:
- Combine the square roots under a single radical: \( \sqrt{7} \times \sqrt{7} = \sqrt{7 \times 7} \).
- Simplify the expression under the radical: \( \sqrt{49} \).
- Since the square root of 49 is 7, we get \( \sqrt{49} = 7 \).
Long Division Method
The long division method can be used to find the square root of 7 in a step-by-step manner:
- Write 7 as 7.000000. Consider pairs of digits starting from the decimal point.
- Determine a number whose square is less than or equal to 7. Here, 2 is the largest such number (since \(2^2 = 4\)).
- Write 2 as the quotient and subtract 4 from 7 to get the remainder 3. Bring down the next pair of zeros to get 300.
- Double the quotient (2) to get 4 and place it as the new divisor’s tens digit (40). Find a number such that \(40n \times n\) is less than or equal to 300. Here, 6 is that number (since \(46 \times 6 = 276\)).
- Subtract 276 from 300 to get 24. Bring down the next pair of zeros to get 2400.
- Double the quotient (26) to get 52 and place it as the new divisor’s tens digit (520). Find a number such that \(520n \times n\) is less than or equal to 2400. Here, 4 is that number (since \(524 \times 4 = 2096\)).
- Repeat this process to find the quotient to the desired decimal places.
Through this process, we approximate \( \sqrt{7} \approx 2.645 \).
Square Root Properties
Additionally, it's important to understand the properties of square roots:
- Positive and Negative Roots: Every positive number has two square roots, one positive and one negative. For example, \( \sqrt{7} \approx 2.645 \) and \( -\sqrt{7} \approx -2.645 \).
- Perfect vs. Imperfect Squares: The square root of a perfect square is an integer (e.g., \( \sqrt{49} = 7 \)), while the square root of an imperfect square is a non-integer (e.g., \( \sqrt{7} \approx 2.645 \)).
Perfect and Imperfect Squares
Understanding perfect and imperfect squares is essential in grasping the concept of square roots. Let's explore these concepts in detail:
Perfect Squares
A perfect square is an integer that is the square of another integer. In other words, if \( n \) is an integer, then \( n^2 \) is a perfect square. For example:
- \( 1^2 = 1 \)
- \( 2^2 = 4 \)
- \( 3^2 = 9 \)
- \( 4^2 = 16 \)
- \( 5^2 = 25 \)
Imperfect Squares
An imperfect square, also known as a non-perfect square, is a number that is not the square of an integer. These numbers do not have exact integer square roots. For example:
- \( 2 \) (since \( \sqrt{2} \) is approximately \( 1.414 \))
- \( 3 \) (since \( \sqrt{3} \) is approximately \( 1.732 \))
- \( 5 \) (since \( \sqrt{5} \) is approximately \( 2.236 \))
- \( 7 \) (since \( \sqrt{7} \) is approximately \( 2.646 \))
Square Root of 7
The square root of 7 is an example of an imperfect square. It cannot be simplified to an exact integer. The value of \( \sqrt{7} \) is approximately \( 2.646 \), and when multiplied by itself, it gives us back the original number:
\[ \sqrt{7} \times \sqrt{7} = 7 \]
Understanding Perfect vs. Imperfect Squares
To distinguish between perfect and imperfect squares, consider the following points:
- Check if the number is an integer and can be written as the product of an integer with itself. If yes, it's a perfect square.
- If the number cannot be expressed as the product of an integer with itself and its square root is a non-repeating, non-terminating decimal, it is an imperfect square.
Examples and Practice
Let's look at a few examples to better understand the concept:
| Number | Square Root | Perfect or Imperfect |
|---|---|---|
| 9 | \( \sqrt{9} = 3 \) | Perfect |
| 8 | \( \sqrt{8} \approx 2.828 \) | Imperfect |
| 16 | \( \sqrt{16} = 4 \) | Perfect |
| 10 | \( \sqrt{10} \approx 3.162 \) | Imperfect |
Long Division Method
The long division method is a systematic way to find the square root of a number. Here is a detailed step-by-step guide to finding the square root of 7 using the long division method:
- Represent the number 7 as 7.000000 to determine the square root value up to 3 decimal places. Group the digits after the decimal point in pairs (7.00 00 00).
- Find the largest number whose square is less than or equal to 7. The number is 2 because \(2^2 = 4\). Write 2 as the quotient, and subtract 4 from 7, giving a remainder of 3.
- Bring down the first pair of zeros, making the new dividend 300. Write a decimal point in the quotient after 2.
- Double the quotient obtained so far (2). This gives 4, which becomes the new divisor.
- Find a number (X) such that \(4X \times X \leq 300\). The number is 6 because \(46 \times 6 = 276\). Write 6 in the quotient next to 2, giving 2.6.
- Subtract 276 from 300, giving a remainder of 24. Bring down the next pair of zeros to make the new dividend 2400.
- Double the current quotient (26), which gives 52. Find a number (Y) such that \(52Y \times Y \leq 2400\). The number is 4 because \(524 \times 4 = 2096\). Write 4 in the quotient, giving 2.64.
- Continue this process until you reach the desired number of decimal places. For the next step, double the quotient (264), making it 528, and find a number (Z) such that \(528Z \times Z \leq 24000\).
By repeating these steps, you find that the square root of 7 is approximately 2.645.
| Step | Action | Result |
|---|---|---|
| 1 | Find largest integer whose square is ≤ 7 | 2 (since \(2^2 = 4\)) |
| 2 | Subtract 4 from 7, bring down 00 | 3.00 |
| 3 | Double quotient (2), new divisor is 4_ | 4_ |
| 4 | Find X such that \(4X \times X \leq 300\) | 6 (since \(46 \times 6 = 276\)) |
| 5 | Subtract 276 from 300, bring down 00 | 24.00 |
| 6 | Double quotient (26), new divisor is 52_ | 52_ |
| 7 | Find Y such that \(52Y \times Y \leq 2400\) | 4 (since \(524 \times 4 = 2096\)) |
Continue this process to achieve a more accurate value of the square root of 7, approximating it to 2.645.
Practical Applications and Examples
Square roots are fundamental in various practical applications across different fields. Below are some real-world examples and uses of square roots:
- Finance: In finance, square roots are used to calculate stock market volatility, which measures the extent of price fluctuations. This involves taking the variance of stock returns and finding its square root to assess investment risk.
- Architecture and Engineering: Engineers use square roots to determine the natural frequency of structures like bridges and buildings. This helps predict how structures will react to different loads, such as wind or traffic.
- Science: Square roots are employed in various scientific calculations, such as determining the velocity of moving objects, the amount of radiation absorbed by materials, and the intensity of sound waves.
- Statistics: In statistics, the standard deviation, which measures data variability, is the square root of the variance. This helps statisticians understand data distribution and make informed decisions.
- Geometry: In geometry, square roots are crucial for calculating the area and perimeter of shapes, as well as solving problems involving right triangles using the Pythagorean theorem.
- Computer Science: Square roots are used in programming for tasks such as encryption algorithms, image processing, and game physics. These applications are critical for data security and creating realistic graphics.
- Cryptography: Cryptographic algorithms often utilize square roots for digital signatures, key exchange systems, and secure communication channels, ensuring data integrity and security.
- Navigation: In navigation, square roots help compute distances between points on maps and estimate directions, aiding pilots and sailors in planning their routes accurately.
- Electrical Engineering: Square roots are used to calculate power, voltage, and current in electrical circuits, essential for designing and maintaining electrical systems and devices.
- Cooking: Recipes may require adjusting ingredient amounts for larger batches. The square root of the scaling factor helps maintain the correct balance of flavors when scaling recipes up or down.
- Photography: The aperture of a camera lens, which controls light entry, is expressed using the f-number. The area of the aperture is proportional to the square of the f-number, affecting exposure and image quality.
- Computer Graphics: In 2D and 3D graphics, square roots are used to calculate distances between points and lengths of vectors, essential for rendering accurate images and animations.
- Telecommunication: Signal strength in wireless communication decreases with distance, following the inverse square law. Understanding this helps in optimizing signal transmission and reception.
These examples highlight the importance of square roots in various fields, demonstrating their practical utility in solving real-world problems and enhancing technological advancements.
FAQs on Square Roots
This section addresses some common questions about square roots, their properties, and applications.
-
What is a square root?
A square root of a number is a value that, when multiplied by itself, gives the original number. It is denoted by the symbol √. For example, the square root of 25 is 5, because 5 × 5 = 25.
-
What are perfect squares?
Perfect squares are numbers whose square roots are whole numbers. For example, 1, 4, 9, 16, 25, and 36 are perfect squares because their square roots are 1, 2, 3, 4, 5, and 6 respectively.
-
What are imperfect squares?
Imperfect squares are numbers that do not have whole numbers as their square roots. For example, 2, 3, 5, and 7 are imperfect squares. Their square roots are irrational numbers: √2 ≈ 1.414, √3 ≈ 1.732, √5 ≈ 2.236, and √7 ≈ 2.646.
-
How do you find the square root of a number?
There are several methods to find the square root of a number, including:
- Prime factorization: Decompose the number into its prime factors and pair them.
- Long division method: A systematic approach to finding the square root, especially useful for non-perfect squares.
- Using a calculator: Most calculators have a square root function.
-
What is the square root of 7 times the square root of 7?The square root of 7 times the square root of 7 is 7. This is because √7 × √7 = 7, according to the property of square roots that √a × √a = a.
-
What are the practical applications of square roots?
Square roots are used in various fields such as:
- Geometry: Calculating areas and dimensions.
- Algebra: Solving quadratic equations.
- Physics: Determining physical quantities like speed and energy.
- Statistics: Standard deviation and variance calculations.
Căn Bậc Hai của 7
READ MORE:
Đơn giản hóa căn bậc hai #7