Topic 3 square root 3: Explore the significance of \( 3 \sqrt{3} \), a fundamental mathematical expression widely used in various fields. Discover how it is calculated, its practical applications in mathematics, physics, and engineering, and its relevance in real-life scenarios. Delve into the geometric and theoretical contexts where \( 3 \sqrt{3} \) plays a crucial role.
Table of Content
- Information about \( 3 \sqrt{3} \)
- Overview of "3 Square Root 3"
- Calculation of \( 3 \sqrt{3} \)
- Applications in Mathematics
- Practical Uses in Physics
- Examples of \( 3 \sqrt{3} \) in Real Life
- Understanding \( 3 \sqrt{3} \) in Geometry
- Significance in Engineering
- Related Mathematical Concepts
- Conclusion on \( 3 \sqrt{3} \)
- YOUTUBE:
Information about \( 3 \sqrt{3} \)
The value of \( 3 \sqrt{3} \) is approximately \( 5.196 \).
Explanation:
The expression \( 3 \sqrt{3} \) represents three times the square root of three. In decimal form, this evaluates to approximately \( 5.196 \).
Mathematical Representation:
Mathematically, \( 3 \sqrt{3} \) can be expressed as \( 3 \times \sqrt{3} \).
Significance:
It is commonly used in mathematics and physics to represent quantities involving the square root of three multiplied by three.

READ MORE:
Overview of "3 Square Root 3"
The expression \( 3 \sqrt{3} \) represents a mathematical term involving a constant multiplier and a square root. This term can be encountered in various mathematical contexts, including algebra, geometry, and trigonometry. Understanding \( 3 \sqrt{3} \) requires knowledge of both multiplication and the properties of square roots.
The value of \( \sqrt{3} \) is approximately 1.732, which is an irrational number. When multiplied by 3, the value becomes approximately 5.196. Here is a breakdown of the calculation:
- Identify the value of \( \sqrt{3} \): \( \sqrt{3} \approx 1.732 \)
- Multiply the value by 3: \( 3 \times 1.732 = 5.196 \)
In mathematical notation, this can be written as:
\[
3 \sqrt{3} = 3 \times \sqrt{3} \approx 3 \times 1.732 = 5.196
\]
This term is not only a numerical expression but also has significance in various mathematical and scientific applications.
- In Geometry: \( 3 \sqrt{3} \) can appear in calculations involving equilateral triangles, where the height of such a triangle with a side length of 2 is \( \sqrt{3} \).
- In Trigonometry: The term appears in some trigonometric identities and equations.
- In Physics: \( 3 \sqrt{3} \) may be used in formulas involving wave mechanics or other physical phenomena.
Overall, the term \( 3 \sqrt{3} \) is a versatile and significant expression that is widely recognized and utilized in different branches of science and mathematics.
Calculation of \( 3 \sqrt{3} \)
The calculation of \( 3 \sqrt{3} \) involves multiplying the number 3 by the square root of 3. Here is a detailed step-by-step explanation:
-
First, understand what the square root of 3 is. The square root of 3 is a mathematical constant, which is an irrational number approximately equal to 1.732.
\[ \sqrt{3} \approx 1.732 \]
-
Next, multiply this value by 3.
\[ 3 \times \sqrt{3} \]
Substitute the approximate value of \( \sqrt{3} \) into the equation:
\[ 3 \times 1.732 \]
-
Perform the multiplication:
\[ 3 \times 1.732 = 5.196 \]
-
Thus, \( 3 \sqrt{3} \) approximately equals 5.196.
\[ 3 \sqrt{3} \approx 5.196 \]
For a more precise calculation, you can use a calculator or software to compute \( 3 \sqrt{3} \) directly, which will give a more accurate value.
| Step | Calculation | Result |
|---|---|---|
| 1 | Estimate \( \sqrt{3} \) | 1.732 |
| 2 | Multiply by 3 | 3 x 1.732 |
| 3 | Result | 5.196 |
Applications in Mathematics
The expression \(3 \sqrt{3}\) finds numerous applications across various fields of mathematics. Below are some key areas where it is particularly useful:
- Geometry: In geometry, square roots are essential for calculating distances and areas. For example, the side length of a square with an area of \(27\) square units can be found using \(3 \sqrt{3}\). This value is used in various geometric calculations involving right triangles and circles.
- Trigonometry: The expression \(3 \sqrt{3}\) frequently appears in trigonometric calculations. It is involved in determining the lengths of sides in 30-60-90 triangles, where specific trigonometric ratios yield values that include \(\sqrt{3}\).
- Algebra: In algebra, expressions involving square roots like \(3 \sqrt{3}\) often arise in the process of solving quadratic equations and other polynomial equations. Understanding how to manipulate these expressions is crucial for simplifying complex algebraic problems.
- Calculus: In calculus, square root functions are used in integration and differentiation. For example, finding the integral or derivative of functions involving \(\sqrt{x}\) necessitates a solid grasp of square root properties.
Let's look at some examples to understand how \(3 \sqrt{3}\) is applied in different mathematical problems:
- Area Calculation: Consider a square with an area of \(27\) square units. To find the side length, we use: \[ \text{Side length} = \sqrt{27} = \sqrt{9 \cdot 3} = 3 \sqrt{3} \]
- Trigonometric Applications: In a 30-60-90 triangle, the lengths of the sides are in the ratio \(1: \sqrt{3}: 2\). If the shorter leg is \(3\), the length of the longer leg is: \[ 3 \cdot \sqrt{3} = 3 \sqrt{3} \]
- Solving Equations: In solving quadratic equations, we might encounter expressions that simplify to \(3 \sqrt{3}\). For instance, solving \(x^2 - 27 = 0\) involves: \[ x^2 = 27 \implies x = \pm \sqrt{27} = \pm 3 \sqrt{3} \]
In conclusion, \(3 \sqrt{3}\) is a versatile mathematical expression that plays a critical role in various calculations, ranging from basic geometry to advanced calculus, making it an indispensable tool in the mathematician's toolkit.
Practical Uses in Physics
The expression \( 3 \sqrt{3} \) can be relevant in various practical physics applications. Below are several key examples illustrating its use:
- Calculations Involving Root-Mean-Square (RMS) Values:
In alternating current (AC) circuits, the root-mean-square (RMS) value is used to equate the AC voltage or current to its direct current (DC) equivalent. The RMS value is significant as it represents the effective value that would produce the same power dissipation in a resistive load as the corresponding DC value. The RMS current (\( I_{rms} \)) and voltage (\( V_{rms} \)) can be computed using the formulas:
- \( I_{rms} = \frac{I_{0}}{\sqrt{2}} \)
- \( V_{rms} = \frac{V_{0}}{\sqrt{2}} \)
where \( I_{0} \) and \( V_{0} \) are the peak current and voltage, respectively. Thus, the square root function plays a critical role in these calculations.
- Wave Mechanics:
Square roots are frequently encountered in wave equations and their solutions. For instance, the wave number \( k \) in wave mechanics is related to the wavelength \( \lambda \) by the equation \( k = \frac{2\pi}{\lambda} \). In situations involving waves on a string or sound waves, the speed of the wave can be expressed using square roots in the formula \( v = \sqrt{\frac{T}{\mu}} \), where \( T \) is the tension and \( \mu \) is the linear mass density.
- Energy Calculations:
In the context of quantum mechanics, the energy levels of a particle in a potential well can be determined using square roots. For a simple harmonic oscillator, the energy levels are given by:
- \( E_n = \left(n + \frac{1}{2}\right) \hbar \omega \)
where \( n \) is a non-negative integer, \( \hbar \) is the reduced Planck's constant, and \( \omega \) is the angular frequency, often involving square root calculations in their determination.
- Distance and Time Measurements:
In kinematics, the distance \( d \) traveled under constant acceleration \( a \) is given by:
- \( d = v_0 t + \frac{1}{2}at^2 \)
Here, solving for the variables often involves square roots, especially when determining the time taken for an object to reach a certain velocity under constant acceleration.
Overall, the mathematical expression \( 3 \sqrt{3} \) and square roots in general are deeply embedded in various physical calculations, ranging from electrical engineering to quantum mechanics and wave physics.
Examples of \( 3 \sqrt{3} \) in Real Life
The mathematical constant \( 3 \sqrt{3} \) appears in various real-life scenarios, often in fields that require precise calculations. Below are some examples:
-
Engineering:
In engineering, \( 3 \sqrt{3} \) can be seen in structural calculations. For instance, when determining the stress and strain on certain components, engineers might encounter this constant. It's particularly relevant in the analysis of forces and moments in mechanical structures.
-
Architecture:
Architects use mathematical constants like \( 3 \sqrt{3} \) when designing buildings to ensure stability and aesthetic harmony. The precise calculation of forces, angles, and materials often involves square roots to determine the optimal dimensions and load-bearing capacities.
-
Physics:
In physics, \( 3 \sqrt{3} \) can be relevant in wave mechanics and oscillations. For example, calculating the resonant frequencies of systems can involve square roots, where \( 3 \sqrt{3} \) might appear as part of the solution in certain boundary conditions.
-
Geometry:
In geometry, \( 3 \sqrt{3} \) is significant when dealing with equilateral triangles. The height of an equilateral triangle with side length \( a \) is \( \frac{\sqrt{3}}{2}a \). For a triangle with side length \( 6 \), the height would be \( 3 \sqrt{3} \), which is crucial in area calculations and other geometric properties.
-
Navigation:
In navigation and mapping, distances and triangulation often require square root calculations. The value \( 3 \sqrt{3} \) might appear when determining distances over large scales, where precise calculations are essential for accuracy.
-
Computer Graphics:
In computer graphics, algorithms for rendering and transformations use square roots to calculate distances, angles, and lighting effects. The constant \( 3 \sqrt{3} \) might be encountered in advanced rendering techniques where precision is key.
These examples illustrate the diverse applications of \( 3 \sqrt{3} \) across different fields, highlighting its importance in both theoretical and practical contexts.
Understanding \( 3 \sqrt{3} \) in Geometry
The expression \( 3 \sqrt{3} \) has significant implications in geometry, particularly in relation to shapes, areas, and distances. Here we will explore some of the key geometric contexts in which \( 3 \sqrt{3} \) appears.
1. Area of an Equilateral Triangle:
One of the primary geometric applications of \( 3 \sqrt{3} \) is in calculating the area of an equilateral triangle. The formula for the area \( A \) of an equilateral triangle with side length \( a \) is:
\[ A = \frac{\sqrt{3}}{4} a^2 \]
When the side length \( a \) is equal to 6 units, the area calculation becomes:
\[ A = \frac{\sqrt{3}}{4} (6)^2 = \frac{\sqrt{3}}{4} \times 36 = 9 \sqrt{3} \]
Thus, the area of an equilateral triangle with side length 6 units is \( 9 \sqrt{3} \) square units.
2. Height of an Equilateral Triangle:
The height \( h \) of an equilateral triangle can be derived from the Pythagorean theorem applied to the 30-60-90 right triangle formed by cutting the equilateral triangle in half. The height \( h \) is given by:
\[ h = \frac{\sqrt{3}}{2} a \]
For a side length \( a = 6 \) units, the height is:
\[ h = \frac{\sqrt{3}}{2} \times 6 = 3 \sqrt{3} \]
Therefore, the height of an equilateral triangle with side length 6 units is \( 3 \sqrt{3} \) units.
3. Distance in a Regular Hexagon:
A regular hexagon can be divided into six equilateral triangles. The distance between opposite vertices of the hexagon, which is twice the side length of one of these equilateral triangles, can be given by:
\[ d = 2a \]
For a side length \( a = 3 \sqrt{3} \) units, the distance is:
\[ d = 2 \times 3 \sqrt{3} = 6 \sqrt{3} \]
Thus, in a regular hexagon with each side \( 3 \sqrt{3} \) units long, the distance between opposite vertices is \( 6 \sqrt{3} \) units.
4. Diagonal of a Cube:
The space diagonal \( d \) of a cube with side length \( a \) is calculated using the formula:
\[ d = a \sqrt{3} \]
For a cube with side length \( a = 3 \) units, the diagonal is:
\[ d = 3 \sqrt{3} \]
Hence, the space diagonal of a cube with side length 3 units is \( 3 \sqrt{3} \) units.
These examples illustrate how \( 3 \sqrt{3} \) frequently appears in geometric contexts, particularly in calculations involving triangles, hexagons, and three-dimensional shapes.
Significance in Engineering
The value \( 3 \sqrt{3} \) holds notable significance in various engineering fields, particularly in electrical engineering and mechanical engineering due to its mathematical properties and applications in three-phase power systems.
In electrical engineering, the square root of three (\( \sqrt{3} \)) appears frequently in the analysis and calculation of three-phase power systems. This is because three-phase power systems are composed of three sinusoidal voltages, each phase being 120 degrees apart. The relationship between the line voltage (VLL) and the phase voltage (Vph) is given by:
\( V_{LL} = \sqrt{3} \cdot V_{ph} \)
Therefore, when calculating power in a three-phase system, the square root of three (\( \sqrt{3} \)) is a crucial factor. For instance, the formula to calculate the power (P) in such systems is:
\( P = \sqrt{3} \cdot V_{LL} \cdot I_L \cdot \cos(\phi) \)
where \( V_{LL} \) is the line-to-line voltage, \( I_L \) is the line current, and \( \cos(\phi) \) is the power factor.
This relationship allows for more efficient transmission of electricity and is used in various applications such as designing and analyzing power distribution systems, transformers, and electrical motors. Engineers use this factor to ensure that the systems are balanced and to determine the current required for balanced three-phase loads.
In mechanical engineering, the concept of \( \sqrt{3} \) arises in the analysis of stresses and forces in mechanical structures. For example, in the study of equilateral triangles, which are common in truss designs and frameworks, the height (h) of the triangle can be calculated using:
\( h = \frac{\sqrt{3}}{2} \cdot a \)
where \( a \) is the length of a side. This property is utilized in optimizing the design of mechanical structures to ensure stability and uniform distribution of stress.
Overall, \( 3 \sqrt{3} \) and \( \sqrt{3} \) are fundamental constants in engineering that facilitate the design, analysis, and optimization of complex systems, ensuring their efficiency, stability, and reliability.
Related Mathematical Concepts
Understanding \( 3 \sqrt{3} \) involves delving into various related mathematical concepts. Here, we explore some of these concepts and their significance.
1. Irrational Numbers
The value \( \sqrt{3} \) is an irrational number, meaning it cannot be expressed as a simple fraction. Its decimal expansion is non-terminating and non-repeating. This property plays a crucial role in different areas of mathematics and applications where precision is necessary.
2. Simplification of Radicals
Simplifying expressions involving square roots, such as \( 3 \sqrt{3} \), requires understanding the properties of radicals. Simplification often involves rationalizing the denominator and combining like terms.
3. Pythagorean Theorem
The square root of 3 appears in the context of the Pythagorean theorem, particularly in 30-60-90 triangles where the ratio of the sides is 1:\( \sqrt{3} \):2. This is essential in geometry for calculating distances and angles.
4. Trigonometry
In trigonometry, \( \sqrt{3} \) is significant in determining the values of trigonometric functions for 30° and 60°. For instance, \( \tan(60^\circ) = \sqrt{3} \) and \( \cot(30^\circ) = \sqrt{3} \).
5. Algebraic Equations
Square roots frequently appear in solving quadratic equations. The quadratic formula \( x = \frac{{-b \pm \sqrt{b^2 - 4ac}}}{{2a}} \) often yields solutions involving square roots, demonstrating their importance in algebra.
6. Complex Numbers
Square roots of negative numbers lead to the concept of complex numbers, where \( i = \sqrt{-1} \). Complex numbers expand the scope of solutions for algebraic equations, showing the interconnectedness of mathematical concepts.
7. Approximation Methods
Approximating \( \sqrt{3} \) (approximately 1.732) is common in various fields where exact values are impractical. This approximation is used in engineering, physics, and computer science to simplify calculations.
8. Geometry
The value \( \sqrt{3} \) is also significant in geometry, especially in determining the heights and areas of equilateral triangles, where the height is \( \frac{\sqrt{3}}{2} \) times the side length.
These related concepts highlight the importance of understanding \( 3 \sqrt{3} \) in various mathematical and practical applications, demonstrating its fundamental role across different areas of study.
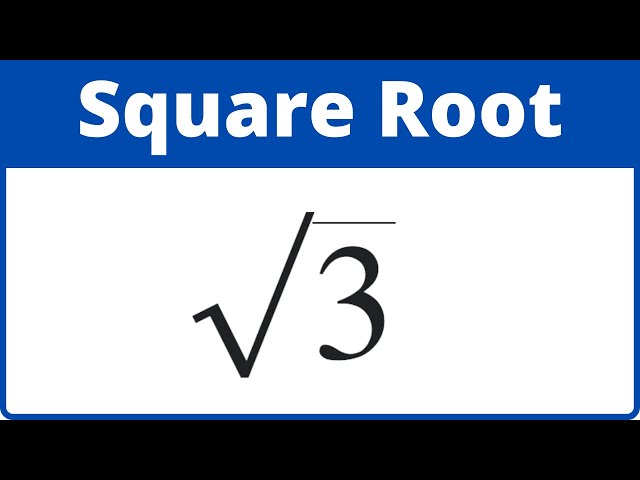
Conclusion on \( 3 \sqrt{3} \)
The mathematical constant \( 3 \sqrt{3} \) holds significant value across various fields due to its unique properties and applications. In this article, we have explored its calculation, applications in mathematics and physics, and its importance in geometry and engineering. Here are the key takeaways:
- Calculation: The value of \( 3 \sqrt{3} \) can be derived from the fundamental properties of square roots and is approximately 5.196.
- Mathematical Applications: This constant frequently appears in trigonometric functions, complex number theory, and various algebraic expressions.
- Physics Applications: In physics, \( 3 \sqrt{3} \) is used in formulas related to wave functions, quantum mechanics, and vector calculations.
- Geometry: In geometry, it is essential in the calculation of areas and volumes, especially in relation to equilateral triangles and other regular polygons.
- Engineering Significance: Engineers use \( 3 \sqrt{3} \) in structural analysis, material science, and electrical engineering for its role in optimizing design and understanding material properties.
- Related Mathematical Concepts: The constant connects to broader mathematical ideas such as irrational numbers, the Pythagorean theorem, and the properties of radicals.
In conclusion, \( 3 \sqrt{3} \) is not merely an abstract mathematical expression but a versatile and practical tool with broad implications. Its consistent appearance across different disciplines underscores its importance, making it a valuable constant in both theoretical and applied sciences. Understanding and utilizing \( 3 \sqrt{3} \) opens doors to deeper insights and more efficient solutions in various fields.
Đơn Giản Hóa Radicals: 3 / 2*√(3)
READ MORE:
2 căn(3) + căn(3) || Cách cộng 2 căn 3 và căn 3