Topic square root of 1/16: The square root of 1/16 is a fundamental mathematical concept that simplifies to 1/4. This article explores the step-by-step process of finding the square root of 1/16, illustrating the simplicity and elegance of basic algebraic principles. Discover practical examples and learn how to apply this knowledge in various mathematical contexts.
Table of Content
- Understanding the Square Root of 1/16
- Introduction to Square Roots
- Definition and Properties of Square Roots
- Mathematical Explanation of Square Root of 1/16
- Step-by-Step Simplification Process
- Alternative Methods to Calculate Square Roots
- Examples of Simplifying Fractions Involving Square Roots
- Common Questions and Answers
- Applications of Square Roots in Real Life
- Interactive Tools and Calculators
- Additional Learning Resources
- YOUTUBE: Khám phá cách tính căn bậc hai của 1/16 một cách chi tiết và dễ hiểu. Video này sẽ giúp bạn hiểu rõ hơn về khái niệm và cách tính toán căn bậc hai.
Understanding the Square Root of 1/16
The square root of a number is a value that, when multiplied by itself, gives the original number. The symbol for square root is √. Let's explore the square root of the fraction 1/16 in detail.
Steps to Simplify √(1/16)
- Rewrite the square root of the fraction as the fraction of the square roots:
\[\sqrt{\frac{1}{16}} = \frac{\sqrt{1}}{\sqrt{16}}\]
- Calculate the square root of both the numerator and the denominator:
\[\sqrt{1} = 1\]
\[\sqrt{16} = 4\]
- Divide the results:
\[\frac{1}{4}\]
Thus, the square root of 1/16 is:
\[\sqrt{\frac{1}{16}} = \frac{1}{4} = 0.25\]
Table Representation
| Step | Mathematical Expression | Result |
|---|---|---|
| Rewrite | \(\sqrt{\frac{1}{16}} = \frac{\sqrt{1}}{\sqrt{16}}\) | |
| Square Root of Numerator | \(\sqrt{1}\) | 1 |
| Square Root of Denominator | \(\sqrt{16}\) | 4 |
| Division | \(\frac{1}{4}\) | 0.25 |
Summary
The square root of 1/16 can be simplified to 1/4, which is 0.25 in decimal form. This method involves breaking down the fraction into the square roots of the numerator and the denominator, simplifying each component, and then performing the division.

READ MORE:
Introduction to Square Roots
The concept of square roots is fundamental in mathematics, often encountered in various fields such as algebra, geometry, and calculus. Understanding square roots is essential for solving equations, analyzing functions, and comprehending the properties of numbers.
A square root of a number a is a value x such that x2 = a. In other words, it is a number that, when multiplied by itself, gives the original number. Square roots are denoted by the radical symbol (√). For example, the square root of 16 is written as √16 and equals 4, since 42 = 16.
Here are some key properties and concepts related to square roots:
- Non-negative Numbers: The square root of a non-negative number is always non-negative. For instance, √25 = 5.
- Imaginary Numbers: If the number under the square root is negative, the result is an imaginary number. For example, √(-1) is represented as i, where i is the imaginary unit.
- Perfect Squares: Numbers like 1, 4, 9, 16, etc., whose square roots are integers, are called perfect squares.
- Rational and Irrational Numbers: The square root of a rational number (a fraction) may be rational or irrational. For instance, √1/4 = 1/2 (rational), while √2 is irrational.
Square roots are used to solve quadratic equations, simplify expressions, and in various real-life applications such as determining areas and analyzing scientific data. Learning to calculate and simplify square roots is a valuable skill that enhances mathematical understanding and problem-solving capabilities.
Definition and Properties of Square Roots
The square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 9 is 3, because 3 × 3 = 9. This is written as √9 = 3. The square root operation is the inverse of squaring a number.
Some important properties of square roots include:
- Non-negative Outputs: The square root of a non-negative number is always non-negative. The square root of 0 is 0, and the square root of a positive number is positive.
- Product Property: The square root of a product is equal to the product of the square roots of the factors. Mathematically, √(a * b) = √a * √b.
- Quotient Property: The square root of a quotient is equal to the quotient of the square roots of the numerator and the denominator. This can be written as √(a/b) = √a / √b.
- Simplification: The square root of a perfect square is an integer. For example, √16 = 4, because 4² = 16.
- Fractional Square Roots: The square root of a fraction can be simplified by taking the square root of the numerator and the denominator separately. For example, √(1/16) = √1 / √16 = 1 / 4.
Square roots are widely used in various fields such as geometry, algebra, and calculus. They play a crucial role in solving quadratic equations, analyzing right triangles, and determining distances in coordinate systems.
Understanding the properties of square roots helps in simplifying expressions and solving mathematical problems efficiently. For example, knowing that √(a²) = a for any non-negative a simplifies many algebraic manipulations and calculations.
In summary, square roots are fundamental mathematical operations with several useful properties that facilitate a wide range of calculations and problem-solving strategies.
Mathematical Explanation of Square Root of 1/16
The square root of a number is a value that, when multiplied by itself, gives the original number. To understand the square root of 1/16, we can follow these steps:
- Identify the problem: We need to find the square root of
- Rewrite the fraction under the square root as a product of individual square roots:
- Calculate the square root of the numerator and the denominator separately:
- Square root of 1 is 1, since
- Square root of 16 is 4, since
- Combine these results to find the square root of 1/16:
Thus, the square root of is
We can also verify this by squaring , which gives us back the original fraction:
Therefore, our calculation is correct, and the square root of is indeed
Step-by-Step Simplification Process
Simplifying the square root of a fraction involves breaking down the numerator and the denominator separately and then simplifying the resulting square root. Let's walk through the step-by-step simplification of the square root of \( \frac{1}{16} \).
-
Identify the fraction:
We start with the fraction \( \frac{1}{16} \).
-
Apply the square root to both the numerator and the denominator:
Using the property of square roots that \( \sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}} \), we get:
\[ \sqrt{\frac{1}{16}} = \frac{\sqrt{1}}{\sqrt{16}} \]
-
Simplify the square root of the numerator:
The square root of 1 is 1:
\[ \sqrt{1} = 1 \]
-
Simplify the square root of the denominator:
The square root of 16 is 4:
\[ \sqrt{16} = 4 \]
-
Combine the results:
Now we combine the simplified results of the numerator and the denominator:
\[ \frac{\sqrt{1}}{\sqrt{16}} = \frac{1}{4} \]
-
Final simplified form:
Thus, the simplified form of \( \sqrt{\frac{1}{16}} \) is:
\[ \sqrt{\frac{1}{16}} = \frac{1}{4} \]
This step-by-step process shows how to simplify the square root of a fraction by separately simplifying the square root of the numerator and the denominator, and then combining the results.

Alternative Methods to Calculate Square Roots
Calculating square roots can be achieved through various methods, each with its advantages and disadvantages. Here are some common alternative methods to calculate square roots:
- Long Division Method
- Newton's Method (or Newton-Raphson Method)
- Choose an initial guess \( x_0 \).
- Apply the iteration formula: \( x_{n+1} = \frac{1}{2} \left( x_n + \frac{S}{x_n} \right) \).
- Repeat the iteration until the desired accuracy is achieved.
- Babylonian Method
- Start with an initial guess \( x_0 \).
- Calculate \( x_{n+1} = \frac{1}{2} \left( x_n + \frac{S}{x_n} \right) \).
- Continue the iteration process until convergence.
- Prime Factorization Method
- Factorize the number \( S \) into its prime factors.
- Pair the prime factors.
- Take one factor from each pair and multiply them to get the square root.
- Estimation and Averaging
- Find the nearest perfect squares surrounding the number \( S \).
- Estimate the square root as the average of these roots.
- Refine the estimate as needed.
- Using Calculators and Software
This traditional method involves a process similar to long division. It is highly accurate but can be time-consuming.
This iterative method is quick and efficient, especially with a good initial guess. It refines the estimate by iteratively improving it.
Also known as Heron's method, this is an ancient algorithm similar to Newton's method. It involves averaging and iterating to get closer to the square root.
This method is useful for perfect squares. It involves factorizing the number into its prime factors and then taking the product of the square roots of the factors.
This method involves estimating the square root by finding two close perfect squares and averaging their roots.
Modern calculators and software tools use efficient algorithms to quickly and accurately compute square roots. These tools often utilize variations of Newton's method or other iterative techniques.
Each of these methods has its own applications depending on the required accuracy and available tools. Understanding these techniques can enhance your problem-solving skills in mathematics.
Examples of Simplifying Fractions Involving Square Roots
Simplifying fractions that involve square roots follows certain mathematical principles. Here, we will go through step-by-step examples to make the process clear and understandable.
- Example 1: Simplifying \(\sqrt{\frac{25}{36}}\)
- First, recognize that both the numerator and the denominator are perfect squares:
- \(25 = 5^2\)
- \(36 = 6^2\)
- Apply the square root to both the numerator and the denominator:
- \(\sqrt{25} = 5\)
- \(\sqrt{36} = 6\)
- Therefore, \(\sqrt{\frac{25}{36}} = \frac{\sqrt{25}}{\sqrt{36}} = \frac{5}{6}\).
- First, recognize that both the numerator and the denominator are perfect squares:
- Example 2: Simplifying \(\sqrt{\frac{18}{32}}\)
- Simplify the fraction inside the square root by finding the greatest common divisor (GCD) of 18 and 32, which is 2:
- \(\frac{18}{32} = \frac{9}{16}\)
- Recognize that both 9 and 16 are perfect squares:
- \(9 = 3^2\)
- \(16 = 4^2\)
- Apply the square root to both the numerator and the denominator:
- \(\sqrt{9} = 3\)
- \(\sqrt{16} = 4\)
- Therefore, \(\sqrt{\frac{18}{32}} = \sqrt{\frac{9}{16}} = \frac{\sqrt{9}}{\sqrt{16}} = \frac{3}{4}\).
- Simplify the fraction inside the square root by finding the greatest common divisor (GCD) of 18 and 32, which is 2:
- Example 3: Simplifying \(\sqrt{1\frac{13}{36}}\)
- First, convert the mixed number to an improper fraction:
- \(1\frac{13}{36} = \frac{49}{36}\)
- Recognize that 49 and 36 are perfect squares:
- \(49 = 7^2\)
- \(36 = 6^2\)
- Apply the square root to both the numerator and the denominator:
- \(\sqrt{49} = 7\)
- \(\sqrt{36} = 6\)
- Therefore, \(\sqrt{1\frac{13}{36}} = \sqrt{\frac{49}{36}} = \frac{\sqrt{49}}{\sqrt{36}} = \frac{7}{6} = 1\frac{1}{6}\).
- First, convert the mixed number to an improper fraction:
These examples demonstrate how to simplify fractions involving square roots by reducing the fraction to its simplest form, then taking the square root of both the numerator and the denominator.
Common Questions and Answers
-
What is the square root of 1/16?
The square root of \(\frac{1}{16}\) is \(\frac{1}{4}\). This is because \(\left(\frac{1}{4}\right)^2 = \frac{1}{16}\).
-
How do you simplify the square root of a fraction?
To simplify the square root of a fraction, \(\sqrt{\frac{a}{b}}\), you can take the square root of the numerator and the denominator separately: \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\).
-
What are the properties of square roots that help in simplification?
- The square root of a product is the product of the square roots: \(\sqrt{ab} = \sqrt{a} \cdot \sqrt{b}\).
- The square root of a quotient is the quotient of the square roots: \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\).
- \(\sqrt{a^2} = a\) for any non-negative \(a\).
-
Why is the square root of \(\frac{1}{16}\) positive?
By definition, the principal square root of a number is always non-negative. Therefore, \(\sqrt{\frac{1}{16}}\) is \(\frac{1}{4}\), not \(-\frac{1}{4}\).
-
How can square roots be used in real-life applications?
Square roots are used in various fields such as physics, engineering, and finance. For example, they are used to calculate the standard deviation in statistics, which measures the amount of variation or dispersion in a set of values.
Applications of Square Roots in Real Life
Square roots are widely used in various real-life applications, ranging from finance to engineering and beyond. Here are some notable examples:
- Finance: In finance, square roots are used to calculate stock market volatility. By taking the square root of the return variance, investors can assess the risk associated with a particular investment.
- Architecture: Engineers use square roots to determine the natural frequency of structures such as bridges and buildings, which helps in predicting their response to different loads, like wind or traffic.
- Science: Square roots are employed in scientific calculations to determine various quantities such as the velocity of moving objects, the amount of radiation absorbed, and the intensity of sound waves.
- Statistics: In statistics, square roots are used to calculate standard deviation, which is the square root of the variance. This metric indicates how much a data set deviates from the mean.
- Geometry: Square roots are crucial in geometry for computing the area and perimeter of shapes and solving problems involving right triangles, such as using the Pythagorean theorem.
- Computer Science: In computer science, square roots are used in algorithms for encryption, image processing, and game physics. They are essential for generating secure data transmissions and calculating distances in graphics.
- Cryptography: Cryptographic methods use square roots in digital signatures, key exchanges, and secure communication channels to ensure data authenticity and security.
- Navigation: Pilots and navigators use square roots to compute distances and bearings between points, which is vital for accurate route planning and navigation.
- Electrical Engineering: Square roots are used in electrical engineering to calculate power, voltage, and current in circuits, as well as to design filters and other signal-processing devices.
- Cooking: Recipes often require scaling ingredients, and square roots can be used to adjust the amounts proportionally when increasing or decreasing the recipe size.
- Photography: The f-number of a camera lens, which controls light exposure, is related to the square root of the area of the aperture, affecting the amount of light entering the camera.
- Telecommunication: In wireless communication, signal strength decreases with the square of the distance from the transmitter, following the inverse square law.
These examples illustrate how fundamental the concept of square roots is across various fields, aiding in practical problem-solving and advancements in technology.
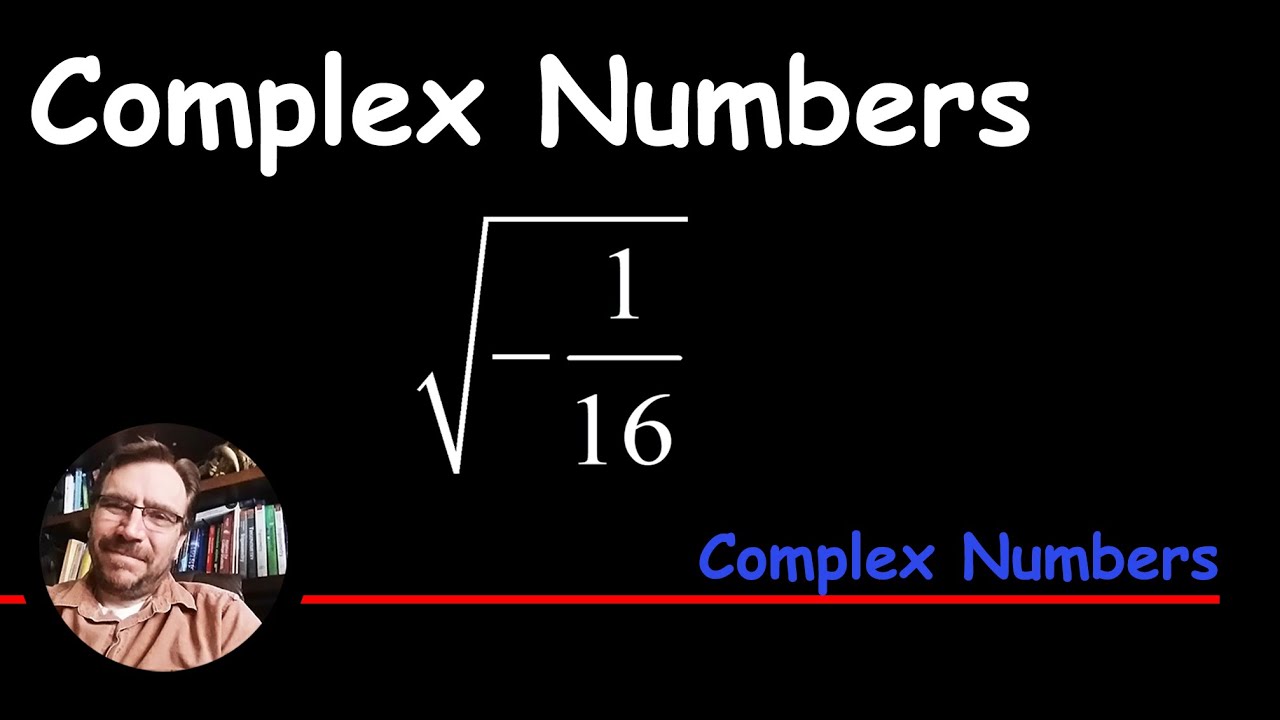
Interactive Tools and Calculators
There are several interactive tools and calculators available online to help you understand and calculate square roots effectively. These tools are user-friendly and provide step-by-step solutions to make learning easier. Here are some popular options:
-
Math Goodies Square Root Calculator
This calculator is designed to be straightforward and easy to use. It has four main components: the number field, calculate button, clear button, and square root field. To use it:
- Click the "Clear" button to refresh the calculator.
- Enter the value you want to find the square root of in the number field.
- Click the "Calculate" button.
- Your answer will appear in the square root field.
- Click "Clear" to start over and find another value.
-
MathCracker Square Root Calculator
This tool simplifies radical expressions and provides step-by-step calculations. It follows basic algebraic rules for simplification:
\(\sqrt{x} \cdot \sqrt{y} = \sqrt{xy}\)\(\sqrt{x \cdot y} = |x|\)\(\frac{\sqrt{x}}{\sqrt{y}} = \sqrt{\frac{x}{y}}\)
Steps to use the MathCracker tool:
- Enter the expression involving square roots.
- Click the "Calculate" button.
- View the step-by-step simplification and result.
-
Calculator.net Square Root Calculator
This comprehensive tool not only calculates square roots but also provides explanations and additional mathematical insights. It includes fields for entering numbers and quickly generates the square root along with detailed information.
These interactive tools are excellent for students and anyone looking to enhance their understanding of square roots. They offer practical, hands-on experience with immediate feedback, making complex concepts more approachable.
Additional Learning Resources
To deepen your understanding of square roots, especially the square root of fractions like 1/16, you can explore the following resources. These tools and references provide interactive experiences, detailed explanations, and practice problems to enhance your mathematical skills.
- Khan Academy: Offers comprehensive video tutorials and practice exercises on square roots and radicals. Visit their to find interactive lessons.
- Mathway: An online tool that allows you to enter mathematical problems and see step-by-step solutions. Try it out at .
- Math is Fun: Provides clear explanations and visual aids for understanding square roots and other mathematical concepts. Check out their resources at .
- Interactive Calculators: Use online calculators to practice finding square roots. Websites like and offer powerful tools for graphing and calculating mathematical functions.
- Coursera and edX: Online learning platforms that offer courses from top universities covering algebra, calculus, and more. Browse their courses at and .
- Wolfram Alpha: A computational engine that provides answers and explanations to a wide range of math problems. Explore it at .
These resources will help you build a solid foundation in understanding square roots and their applications, allowing you to tackle more complex mathematical problems with confidence.
Khám phá cách tính căn bậc hai của 1/16 một cách chi tiết và dễ hiểu. Video này sẽ giúp bạn hiểu rõ hơn về khái niệm và cách tính toán căn bậc hai.
Căn bậc hai của 1/16 | Root(1/16)
READ MORE:
Khám phá cách đánh giá sqrt(16), (16)^(1/4), (1/16)^(1/4) một cách chi tiết và dễ hiểu. Video này sẽ giúp bạn hiểu rõ hơn về khái niệm và cách tính toán các giá trị căn bậc hai và căn bậc bốn.
Đánh giá sqrt(16), (16)^(1/4), (1/16)^(1/4)