Is the Square Root of 10 Irrational? Discover the Mathematical Truth

The square root of 10 is often a topic of interest in mathematics, particularly in the context of rational and irrational numbers. This number is denoted as $\sqrt{10}$ and has intriguing properties.

Understanding Rational and Irrational Numbers

A rational number is a number that can be expressed as the quotient or fraction $\frac{a}{b}$, where $a$ and $b$ are integers and $b$ is not zero. An irrational number, on the other hand, cannot be expressed as a simple fraction; it has a decimal expansion that neither terminates nor repeats.

Is the Square Root of 10 Rational or Irrational?

To determine if $\sqrt{10}$ is irrational, we need to understand its properties:

• First, consider the decimal expansion of $\sqrt{10}$. It is approximately 3.162277660168379... and continues without repeating or terminating.
• Prime factorization of 10 is $2\times 5$, which do not form a perfect square.

Proof of Irrationality

The square root of a non-perfect square is always irrational. Here’s a brief proof:

1. Assume $\sqrt{10}$ is rational, which means it can be expressed as $\frac{a}{b}$, where $a$ and $b$ are coprime integers.
2. Then, $(\frac{a}{b}{)}^2=10$, implying $a^2=10b^2$.
3. Thus, $a^2$ must be divisible by 10. Therefore, $a$ must also be divisible by 10.
4. Let $a=10k$. Substituting, we get ${(}^{10}$.
5. This simplifies to $100k^2=10b^2$, leading to $10k^2=b^2$.
6. This implies $b$ is also divisible by 10, which contradicts the initial assumption that $a$ and $b$ are coprime.

Therefore, $\sqrt{10}$ is irrational.

Conclusion

In conclusion, the square root of 10 is an irrational number because it cannot be expressed as a fraction of two integers and its decimal expansion is non-terminating and non-repeating.

The square root of 10, often denoted as \( \sqrt{10} \), is a number that has intrigued mathematicians for centuries. This number is significant not only in theoretical mathematics but also in various practical applications. The question of whether \( \sqrt{10} \) is irrational is crucial for understanding the nature of numbers and their properties.

In this article, we will delve into the concept of irrationality and provide a comprehensive explanation of why \( \sqrt{10} \) is considered irrational. We will explore the mathematical proofs, historical context, and its implications in different fields. By the end of this article, you will have a clear understanding of the irrationality of the square root of 10 and its relevance in mathematics.

1. Definition of Rational and Irrational Numbers: We will begin by defining what makes a number rational or irrational.
2. Mathematical Proof of Irrationality: A step-by-step proof demonstrating the irrationality of \( \sqrt{10} \).
3. Decimal Expansion of Square Root of 10: Examining the non-repeating, non-terminating decimal expansion of \( \sqrt{10} \).
4. Prime Factorization Method: Understanding why the prime factorization method supports the irrationality of \( \sqrt{10} \).
5. Historical Context and Discovery: A look into the historical background and discovery of irrational numbers, including \( \sqrt{10} \).
6. Applications in Mathematics: How the irrationality of \( \sqrt{10} \) is applied in various mathematical contexts.
7. Common Misconceptions: Addressing and correcting common misunderstandings about irrational numbers.
8. Examples of Other Irrational Numbers: Comparing \( \sqrt{10} \) with other well-known irrational numbers.
9. Conclusion: Summarizing the key points and the importance of recognizing \( \sqrt{10} \) as an irrational number.

To understand why the square root of 10 is considered irrational, it is essential first to define what rational and irrational numbers are.

Rational Numbers: A rational number is any number that can be expressed as the quotient or fraction \( \frac{p}{q} \) of two integers, where \( p \) and \( q \) are integers and \( q \neq 0 \). Examples of rational numbers include:

• \( \frac{1}{2} \)
• \( -\frac{3}{4} \)
• \( 5 \) (since it can be written as \( \frac{5}{1} \))
• \( 0.75 \) (since it can be written as \( \frac{3}{4} \))

In decimal form, rational numbers either terminate (e.g., 0.5) or repeat periodically (e.g., 0.333... = \( \frac{1}{3} \)).

Irrational Numbers: An irrational number, on the other hand, cannot be expressed as a simple fraction. Irrational numbers have non-terminating and non-repeating decimal expansions. This means that no matter how many digits you calculate, the decimal part will never form a repeating pattern. Examples of irrational numbers include:

• \( \pi \) (pi), which is approximately 3.14159...
• \( e \) (Euler's number), which is approximately 2.71828...
• \( \sqrt{2} \), which is approximately 1.41421...

Specifically, the square root of a non-perfect square is always irrational. Since 10 is not a perfect square, \( \sqrt{10} \) cannot be expressed as a fraction of two integers, making it irrational.

The properties of rational and irrational numbers are fundamental in mathematics, helping us understand the structure and behavior of numbers on the real number line. In the following sections, we will explore a mathematical proof that \( \sqrt{10} \) is indeed irrational and examine its decimal expansion.

The irrationality of the square root of 10 can be demonstrated through a proof by contradiction. This method involves assuming the opposite of what we want to prove and showing that this assumption leads to a contradiction, thereby proving the original statement.

1. Assume that \( \sqrt{10} \) is rational. This means it can be expressed as a fraction \( \frac{a}{b} \) in its lowest terms, where \( a \) and \( b \) are coprime integers (i.e., their greatest common divisor is 1).
2. According to the assumption, we have: \[ \sqrt{10} = \frac{a}{b} \] Squaring both sides, we get: \[ 10 = \frac{a^2}{b^2} \] \[ 10b^2 = a^2 \]
3. This equation implies that \( a^2 \) is divisible by 10. Therefore, \( a \) must also be divisible by 10 (since the square of a number divisible by 10 is also divisible by 10). Let \( a = 10k \), where \( k \) is an integer.
4. Substitute \( a = 10k \) into the equation \( 10b^2 = a^2 \): \[ 10b^2 = (10k)^2 \] \[ 10b^2 = 100k^2 \] \[ b^2 = 10k^2 \]
5. This equation implies that \( b^2 \) is also divisible by 10, and therefore, \( b \) must be divisible by 10.
6. We have now shown that both \( a \) and \( b \) are divisible by 10, which contradicts our original assumption that \( \frac{a}{b} \) is in its lowest terms. Therefore, our initial assumption that \( \sqrt{10} \) is rational must be false.
7. Hence, \( \sqrt{10} \) is irrational.

This proof by contradiction clearly demonstrates that the square root of 10 cannot be expressed as a fraction of two integers, confirming its irrationality.

The decimal expansion of \( \sqrt{10} \) provides further evidence of its irrationality. Unlike rational numbers, which have either terminating or repeating decimal expansions, irrational numbers have non-terminating and non-repeating decimal expansions. Let us examine the decimal expansion of \( \sqrt{10} \) step by step.

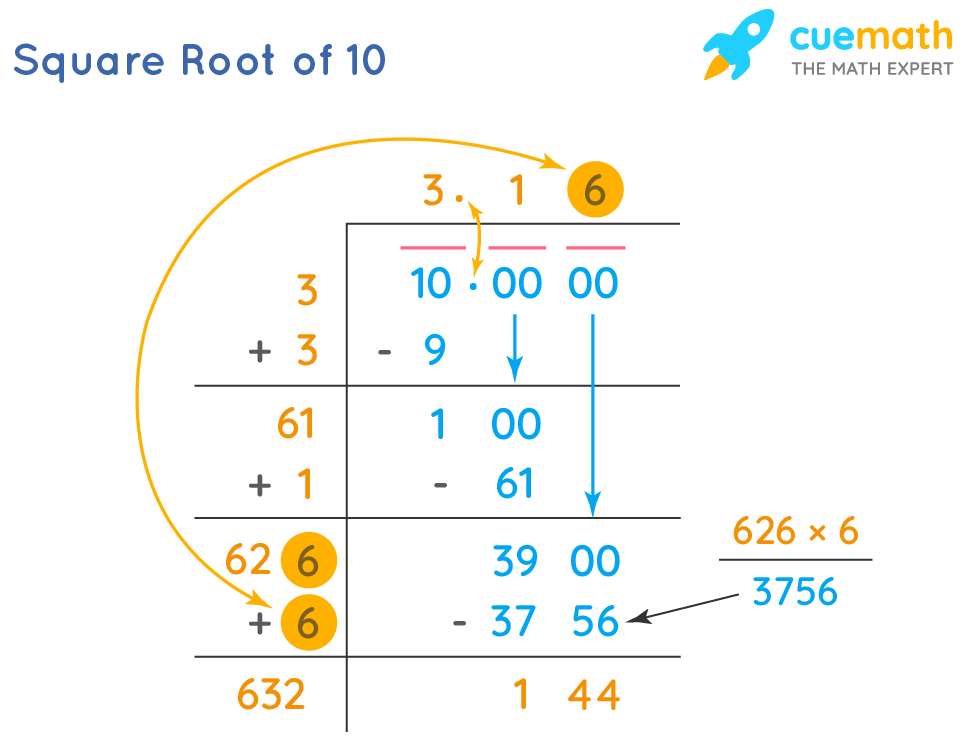
1. Calculate \( \sqrt{10} \) using a calculator or a numerical method. The initial digits are: \[ \sqrt{10} \approx 3.162277660168379... \] This expansion continues infinitely without repeating.
2. To understand why this indicates irrationality, consider the nature of repeating decimal expansions. A rational number, when expressed in decimal form, will either:
   • Terminate (e.g., \( \frac{1}{4} = 0.25 \))
   • Repeat periodically (e.g., \( \frac{1}{3} = 0.3333... \))
   Since \( \sqrt{10} \)'s decimal expansion neither terminates nor repeats, it cannot be a rational number.
3. For further insight, let’s look at a few more digits of \( \sqrt{10} \): \[ \sqrt{10} \approx 3.1622776601683793319988935444327... \] Observing these digits, we see no repeating pattern, reinforcing the fact that \( \sqrt{10} \) is irrational.
4. We can also visualize the non-repeating nature of \( \sqrt{10} \) by examining its expansion graphically or through software that computes more digits. The absence of periodicity confirms the irrationality.

The infinite, non-repeating decimal expansion of \( \sqrt{10} \) is a clear characteristic of irrational numbers. This property is crucial in various mathematical applications, where understanding the behavior of such numbers is essential.

The prime factorization method is a powerful tool in determining whether the square root of a given number is rational or irrational. To illustrate this method, we will apply it to the square root of 10 and show why it is irrational.

1. First, find the prime factorization of 10:
   • 10 can be factorized into primes as: \[ 10 = 2 \times 5 \]
2. Next, consider the square root of 10. If \( \sqrt{10} \) were rational, it could be expressed as \( \frac{a}{b} \), where \( a \) and \( b \) are coprime integers (having no common prime factors other than 1). This implies: \[ \sqrt{10} = \frac{a}{b} \Rightarrow 10 = \frac{a^2}{b^2} \Rightarrow 10b^2 = a^2 \]
3. From the equation \( 10b^2 = a^2 \), we know that \( a^2 \) must be divisible by 10. Thus, \( a \) must also be divisible by 10 (since 10 is not a perfect square, the factors must appear in pairs in \( a^2 \)). Let \( a = 10k \) for some integer \( k \).
4. Substituting \( a = 10k \) into the equation \( 10b^2 = a^2 \): \[ 10b^2 = (10k)^2 \Rightarrow 10b^2 = 100k^2 \Rightarrow b^2 = 10k^2 \] This equation indicates that \( b^2 \) is also divisible by 10, and hence \( b \) must be divisible by 10.
5. We now have that both \( a \) and \( b \) are divisible by 10, which contradicts the assumption that \( a \) and \( b \) are coprime. Therefore, the initial assumption that \( \sqrt{10} \) is rational must be false.
6. Thus, we conclude that \( \sqrt{10} \) cannot be expressed as a fraction of two integers and is therefore irrational.

The prime factorization method effectively demonstrates the irrationality of \( \sqrt{10} \) by showing that its assumed rational representation leads to a contradiction.

The concept of irrational numbers has a rich history dating back to ancient Greece. The discovery of irrational numbers challenged the early Greek mathematicians' understanding of numbers and their properties.

1. Pythagoreans and Early Discoveries:
   • The Pythagoreans, a group of ancient Greek mathematicians, initially believed that all numbers could be expressed as ratios of integers. This belief was shattered when they discovered the irrationality of \( \sqrt{2} \), a fundamental result from the study of right triangles.
   • The legend says that Hippasus, a member of the Pythagorean school, discovered the irrationality of \( \sqrt{2} \). This discovery was so shocking that, according to some accounts, Hippasus was executed or exiled for revealing this "inconvenient truth."
2. Development of Irrational Numbers:
   • Following the discovery of \( \sqrt{2} \), mathematicians began to explore other square roots and their properties. They found that the square roots of non-square numbers, such as \( \sqrt{3} \), \( \sqrt{5} \), and \( \sqrt{10} \), also exhibited irrational characteristics.
   • The concept of irrational numbers expanded over time, with mathematicians like Euclid providing proofs of irrationality for various numbers. Euclid's "Elements" contains a proof of the irrationality of \( \sqrt{2} \), laying the groundwork for future proofs, including that of \( \sqrt{10} \).
3. Modern Understanding:
   • In the modern era, the irrationality of numbers like \( \sqrt{10} \) is well-understood and accepted. Mathematicians have developed numerous methods to prove irrationality, including the prime factorization method and proofs by contradiction.
   • The discovery and study of irrational numbers have profound implications in various fields of mathematics, from number theory to real analysis. Understanding these numbers helps in comprehending the structure and behavior of the real number line.

The historical journey from the initial discovery of irrational numbers to the modern understanding highlights the importance of \( \sqrt{10} \) and similar numbers in the broader mathematical landscape. These discoveries not only challenged ancient beliefs but also paved the way for future advancements in mathematical theory.

The square root of 10, being an irrational number, has significant applications across various fields of mathematics. Its non-repeating and non-terminating decimal expansion makes it useful in several mathematical contexts. Here are some key applications:

• Geometry:

  In geometry, irrational numbers like the square root of 10 are often encountered when calculating distances, areas, and volumes. For example, the length of the diagonal of a rectangle with side lengths of 1 and √10 units involves the square root of 10.

• Algebra:

  In algebra, irrational numbers are crucial when solving quadratic equations. The solutions to these equations frequently involve square roots of non-perfect squares, including √10, particularly in cases where the discriminant is not a perfect square.

• Calculus:

  Calculus extensively uses irrational numbers in limits, integrals, and series expansions. Functions involving √10 can appear in various contexts, such as in the evaluation of integrals and the convergence of series.

• Trigonometry:

  In trigonometry, irrational numbers appear in the exact values of trigonometric functions for certain angles. While √10 itself is less common, the principles apply broadly to similar irrational values.

• Numerical Methods:

  In numerical analysis, approximations of irrational numbers are essential. Methods such as the Newton-Raphson method use successive approximations to find square roots, including that of 10, to a high degree of accuracy.

• Physics and Engineering:

  Irrational numbers, including √10, are used in various physical and engineering calculations. They help describe natural phenomena and are integral to designing systems and structures that require precise measurements.

Overall, the square root of 10, like other irrational numbers, plays a vital role in advancing mathematical understanding and solving complex problems across different domains.

When discussing the irrationality of the square root of 10, several common misconceptions can arise. Understanding these misconceptions helps clarify why the square root of 10 is irrational.

• Misconception 1: Any Non-Integer Result is Rational

  Some people mistakenly believe that if a number's square root is not an integer, it must be a rational number. However, a rational number is defined as a number that can be expressed as a fraction of two integers. The square root of 10, approximately 3.162277660168379, cannot be expressed as such a fraction, thus it is irrational.

• Misconception 2: Square Roots of All Numbers Are Rational

  Another common misconception is that the square root of any number is rational. This is not true. Only the square roots of perfect squares (e.g., 4, 9, 16) are rational. Since 10 is not a perfect square, its square root is irrational.

• Misconception 3: Irrational Numbers Have No Practical Use

  Some might believe that irrational numbers like the square root of 10 are purely theoretical and have no real-world applications. In reality, irrational numbers are essential in various fields of science, engineering, and mathematics, where precise calculations are necessary.

• Misconception 4: All Roots Are Rational

  People sometimes generalize that all roots, such as cube roots or fourth roots, are rational if they are non-repeating. This is incorrect; many roots are irrational, especially when the radicand (the number under the root) is not a perfect power. For example, the cube root of 10 is also irrational.

Addressing these misconceptions helps in better understanding the nature of irrational numbers and the specific case of the square root of 10.

Irrational numbers are real numbers that cannot be expressed as a simple fraction. Their decimal expansions are non-terminating and non-repeating. Here are some well-known examples of irrational numbers:

• √2: The square root of 2 is approximately 1.41421356237. It is the length of the diagonal of a square with sides of length 1.
• π (Pi): Pi is approximately 3.14159265359. It represents the ratio of a circle's circumference to its diameter and is ubiquitous in geometry and trigonometry.
• e (Euler's Number): Euler's number is approximately 2.71828182845. It is the base of the natural logarithm and appears in various fields such as calculus and complex analysis.
• φ (The Golden Ratio): The Golden Ratio is approximately 1.61803398875. It occurs frequently in geometry, art, architecture, and nature.
• √3: The square root of 3 is approximately 1.73205080757. It is the length of the diagonal of a cube with side length 1.
• √5: The square root of 5 is approximately 2.23606797750. It is related to the Golden Ratio and appears in various geometric contexts.
• ln(2) (Natural Logarithm of 2): The natural logarithm of 2 is approximately 0.69314718056. It is used in many areas of mathematics, especially in calculus.
• Γ (Euler-Mascheroni Constant): The Euler-Mascheroni constant is approximately 0.57721566490. It appears in number theory and analysis.
• √7: The square root of 7 is approximately 2.64575131106. It is another example of a non-repeating, non-terminating decimal.
• Chaitin's Constant: This constant is used in algorithmic information theory and is known to be a non-computable real number.