Topic square root of 116 in radical form: Unlock the mystery of the square root of 116 in radical form! Learn how to simplify this intriguing number, explore its properties, and understand its applications in various mathematical contexts. This guide provides a step-by-step process, making it easy for you to grasp and apply these concepts effectively. Dive in and enhance your mathematical skills today!
Table of Content
- Square Root of 116 in Radical Form
- Introduction
- What is the Square Root of 116?
- How to Simplify the Square Root of 116
- Prime Factorization Method
- Steps to Simplify
- Decimal Form of the Square Root of 116
- Properties of the Square Root of 116
- Why 116 is not a Perfect Square
- Visual Representation and Examples
- Application of Square Root of 116 in Geometry
- Comparisons with Other Square Roots
- Common Mistakes to Avoid
- Practice Problems and Solutions
- YOUTUBE: SQUARE ROOT OF 116
Square Root of 116 in Radical Form
The square root of 116 in its simplest radical form is expressed as:
\\( \sqrt{116} = 2\sqrt{29} \\)
Steps to Simplify the Square Root of 116
- Factorize 116: \( 116 = 2 \times 58 = 2 \times 2 \times 29 \).
- Identify and group the perfect squares: \( 2^2 = 4 \).
- Rewrite the square root of 116 using the factors: \( \sqrt{116} = \sqrt{2^2 \times 29} \).
- Pull out the square root of the perfect square: \( \sqrt{2^2 \times 29} = 2\sqrt{29} \).
Calculation of Square Root
The approximate value of the square root of 116 in decimal form is:
\\( \sqrt{116} \approx 10.770329614269 \\)
Visual Representation
 |
 |
| Figure 1: Simplified Radical Form | Figure 2: Decimal Approximation |
Properties of Square Root of 116
- 116 is not a perfect square.
- The square root of 116 is an irrational number.
- The simplest radical form of the square root of 116 is \( 2\sqrt{29} \).
Example Problems
Here are some example problems involving the square root of 116:
- Calculate the length of the hypotenuse in a right triangle with legs measuring 10 inches and 4 inches:
\\( \text{Length} = \sqrt{10^2 + 4^2} = \sqrt{100 + 16} = \sqrt{116} \approx 10.77 \text{ inches} \\)
- Simplify the expression involving the square root of 116:
\\( \frac{\sqrt{116}}{2} = \frac{2\sqrt{29}}{2} = \sqrt{29} \\)
Conclusion
The square root of 116, simplified in radical form, is \( 2\sqrt{29} \), and its decimal approximation is approximately 10.770. Understanding these concepts is essential in various mathematical applications.
READ MORE:
Introduction
Understanding the square root of 116 in radical form involves a few key steps and concepts. The square root of 116, denoted as √116, simplifies to 2√29. This simplification process involves factoring 116 into its prime factors, recognizing pairs of identical factors, and then simplifying under the radical sign.
Here's a step-by-step explanation:
- First, factor 116 into its prime components: 116 = 22 × 29.
- Next, separate the prime factors under the radical sign: √(22 × 29).
- Then, take the square root of the perfect square factor: √(22) = 2.
- Finally, simplify the expression to get the radical form: 2√29.
The value of √116 is approximately 10.7703, but the exact value is best represented in its simplified radical form, 2√29. This form is particularly useful in mathematical proofs and applications where exact values are required.
What is the Square Root of 116?
The square root of 116, written as √116, is a number which, when multiplied by itself, gives the product 116. To express this in its simplest radical form, we start by factoring 116 into its prime factors.
Step-by-step process to simplify √116:
- List the factors of 116: 1, 2, 4, 29, 58, 116.
- Identify the perfect squares from the list of factors: 1 and 4.
- Divide 116 by the largest perfect square identified: 116 ÷ 4 = 29.
- Take the square root of the largest perfect square: √4 = 2.
- Combine the results to express √116 in its simplest radical form: 2√29.
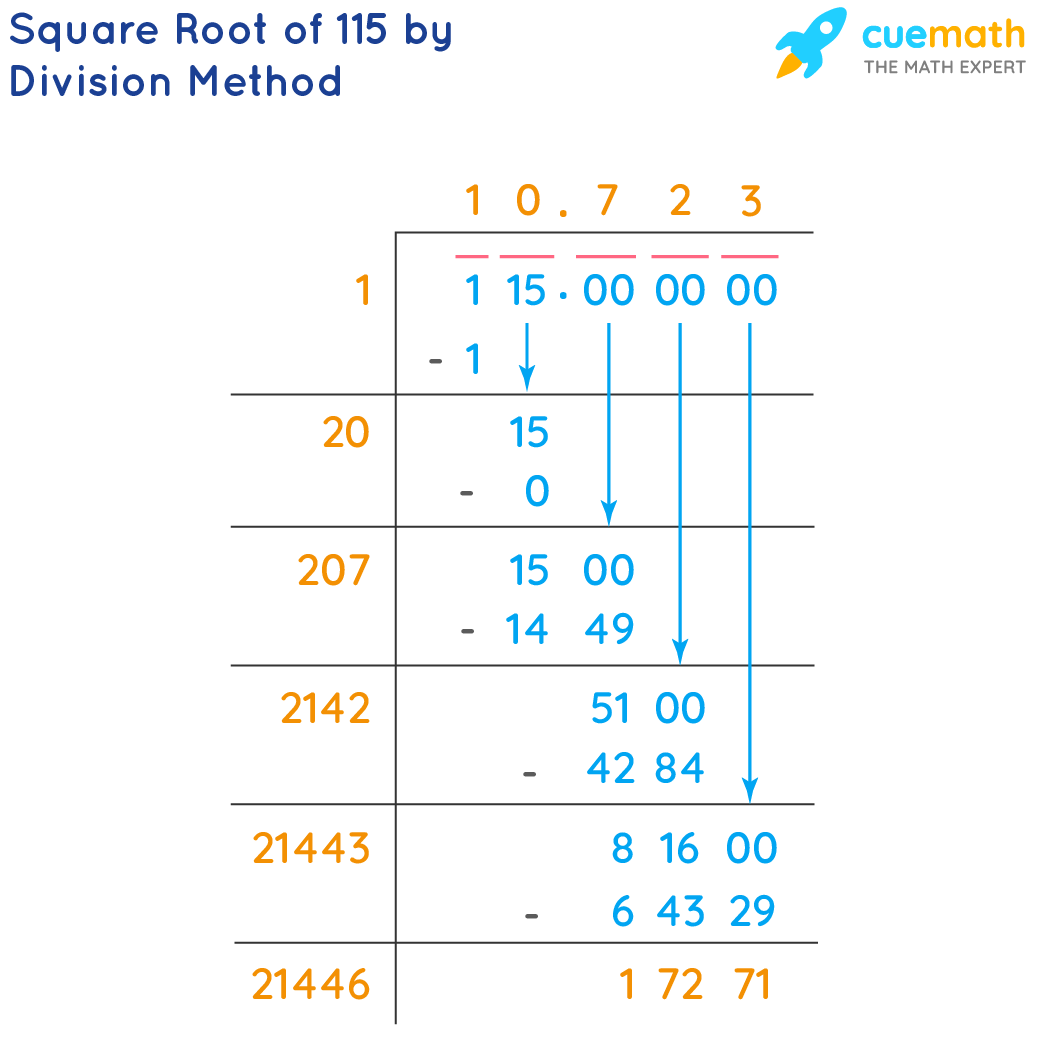
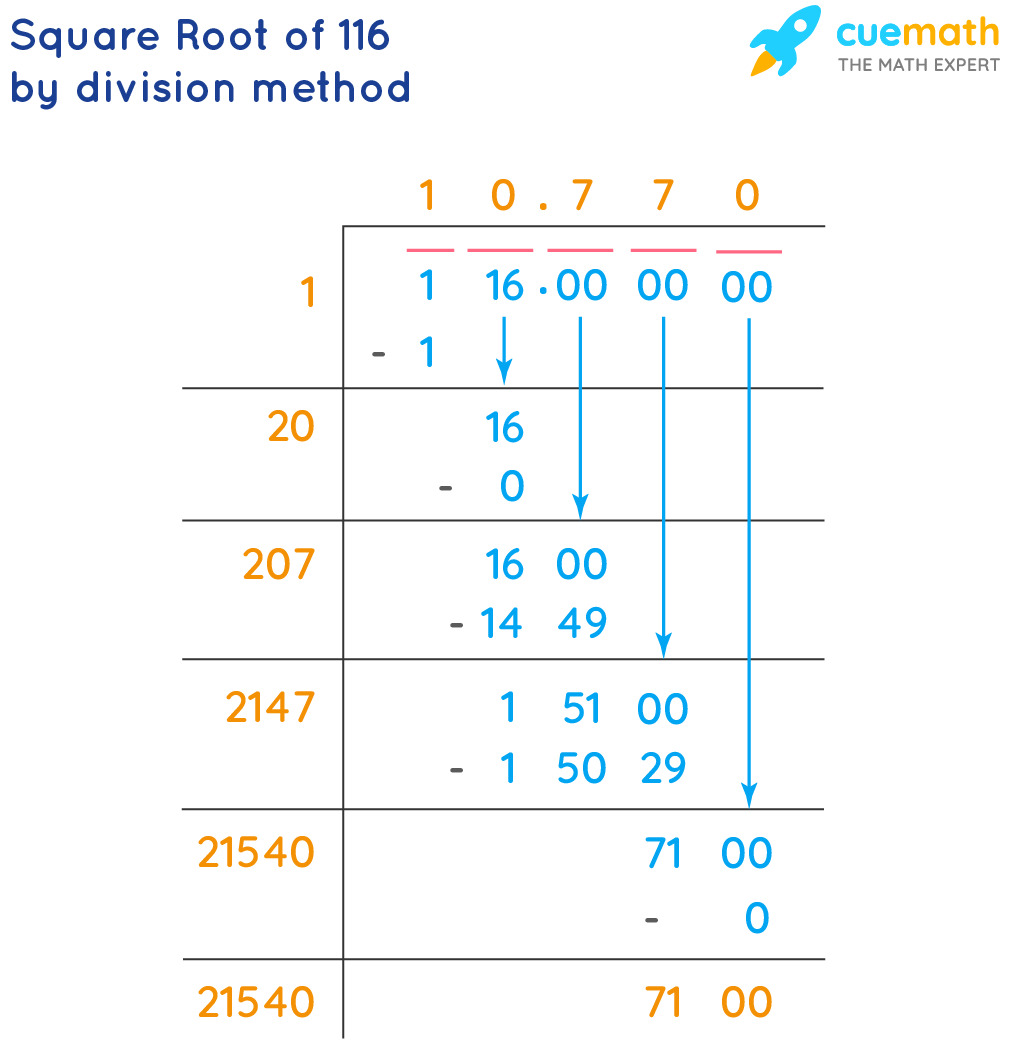
In decimal form, the square root of 116 is approximately 10.770. This value is obtained through methods like the long division method, which involves iterative approximation to achieve a precise result.
Here’s a brief outline of the long division method to find √116:
- Write 116 as 116.000000 and pair the digits from right to left.
- Find the largest number whose square is less than or equal to 1, which is 1 (1x1=1), with a remainder of 0.
- Double the quotient (1) to get 2. Use 20 as the new divisor, and bring down 16 to get the new dividend.
- Find the largest digit to append to 20 that allows multiplication to stay ≤ 1600. Repeat the steps to approximate to the nearest thousandth.
- The final quotient is 10.770.
The square root of 116 is an irrational number because it cannot be expressed as a simple fraction and its decimal form is non-terminating and non-repeating.
How to Simplify the Square Root of 116
To simplify the square root of 116, we follow a step-by-step process to express it in its simplest radical form. Here is a detailed method:
- List the Factors: Identify all factors of 116. These are 1, 2, 4, 29, 58, and 116.
- Identify Perfect Squares: From the list of factors, find the perfect squares. In this case, 4 is a perfect square.
- Divide by the Largest Perfect Square: Divide 116 by the largest perfect square identified. Here, \(116 \div 4 = 29\).
- Calculate the Square Root: Take the square root of the largest perfect square. The square root of 4 is 2.
- Combine the Results: Multiply the result from step 4 by the square root of the remaining factor. Hence, \(\sqrt{116} = \sqrt{4 \times 29} = \sqrt{4} \times \sqrt{29} = 2\sqrt{29}\).
Therefore, the simplified form of the square root of 116 is \(2\sqrt{29}\).
| Square Root | \(\sqrt{116}\) |
| Simplified Form | \(2\sqrt{29}\) |
| Decimal Form | \(10.770\) (approximately) |
This method ensures that the square root is expressed in the simplest radical form, which is more useful in various mathematical contexts.
Prime Factorization Method
To simplify the square root of 116 using the prime factorization method, follow these steps:
- Find the prime factors of 116. Start by dividing 116 by the smallest prime number, which is 2.
- 116 ÷ 2 = 58
- 58 ÷ 2 = 29
- Now we have the prime factors: 2, 2, and 29.
- Group the prime factors into pairs of the same number. Here, we have one pair of 2s.
- 2 × 2 = 4
- 29 remains as a single factor
- Write the square root of 116 as the product of the square roots of these factors: \[ \sqrt{116} = \sqrt{2^2 \times 29} \]
- Simplify by taking the square root of the perfect square (2^2 = 4): \[ \sqrt{116} = \sqrt{2^2} \times \sqrt{29} = 2\sqrt{29} \]
So, the square root of 116 in its simplest radical form is \(2\sqrt{29}\).

Steps to Simplify
Simplifying the square root of 116 involves breaking down the number into its prime factors and simplifying the expression. Here are the detailed steps:
-
Find the prime factorization of 116:
116 can be divided by 2 (which is a prime number). Continue dividing by 2 until it no longer divides evenly:
- \(116 \div 2 = 58\)
- \(58 \div 2 = 29\)
- 29 is a prime number and cannot be divided further.
So, the prime factorization of 116 is \(2^2 \times 29\).
-
Write the square root of 116 using its prime factors:
\(\sqrt{116} = \sqrt{2^2 \times 29}\)
-
Simplify the expression by taking the square root of the perfect square (2 squared):
\(\sqrt{2^2 \times 29} = \sqrt{2^2} \times \sqrt{29}\)
\(\sqrt{2^2} = 2\), so the expression simplifies to:
\(2\sqrt{29}\)
-
Thus, the simplified form of the square root of 116 is:
\(\sqrt{116} = 2\sqrt{29}\)
By following these steps, you can simplify the square root of 116 to its radical form \(2\sqrt{29}\).
Decimal Form of the Square Root of 116
To find the decimal form of the square root of 116, we follow these steps:
-
Understand the square root notation:
The square root of 116 is represented as \(\sqrt{116}\).
-
Use a calculator to find the square root:
Enter 116 and press the square root function (\(\sqrt{}\)) on a scientific calculator to get the decimal value.
-
Obtain the decimal approximation:
The decimal form of \(\sqrt{116}\) is approximately 10.7703.
-
Verify the result (optional):
To ensure accuracy, you can square the decimal value:
\((10.7703)^2 \approx 116\)
Thus, the decimal form of the square root of 116 is approximately 10.7703. This value is rounded to four decimal places for precision.
Properties of the Square Root of 116
The square root of 116 has several interesting properties. Below are some key aspects:
- Non-Perfect Square: 116 is not a perfect square. This means its square root is an irrational number and cannot be expressed as a simple fraction.
- Simplest Radical Form: The simplest radical form of the square root of 116 is \(2\sqrt{29}\). This simplification is achieved by factoring 116 into its prime factors (4 and 29), where 4 is a perfect square.
- Decimal Form: The decimal approximation of the square root of 116 is approximately 10.7703.
- Irrational Number: As an irrational number, the square root of 116 cannot be expressed exactly as a fraction and its decimal form is non-terminating and non-repeating.
- Mathematical Representation: The square root of 116 can also be expressed using exponents as \(116^{1/2}\) or \(2 \times 29^{1/2}\).
In summary, the square root of 116, simplified to \(2\sqrt{29}\), highlights the fascinating nature of irrational numbers and their properties in mathematics.
Why 116 is not a Perfect Square
A perfect square is a number that can be expressed as the product of an integer with itself. In other words, a number \( n \) is a perfect square if there exists an integer \( k \) such that \( n = k^2 \).
To determine if 116 is a perfect square, we need to find if there is an integer \( k \) such that \( k \times k = 116 \). We start by examining the square root of 116.
The square root of 116 is:
\[ \sqrt{116} \approx 10.770329614269 \]
Since 10.770329614269 is not a whole number, 116 cannot be expressed as the product of an integer with itself. Therefore, 116 is not a perfect square.
To further understand why 116 is not a perfect square, let's consider its factors:
\[ 1, 2, 4, 29, 58, 116 \]
Among these factors, the perfect squares are 1 and 4. We notice that 116 is not one of these perfect squares.
We can also look at the prime factorization of 116:
\[ 116 = 2^2 \times 29 \]
For a number to be a perfect square, all the exponents in its prime factorization must be even. Here, 29 is raised to the power of 1, which is not even, hence 116 is not a perfect square.
Lastly, comparing 116 with the closest perfect squares:
\[ 100 = 10^2 \quad \text{and} \quad 121 = 11^2 \]
We can see that 116 lies between 100 and 121, and there is no integer \( k \) such that \( k^2 = 116 \).
In conclusion, because the square root of 116 is not an integer and its prime factorization includes a prime number raised to an odd power, 116 is not a perfect square.
Visual Representation and Examples
The square root of 116 can be visualized and understood through various methods and examples. Let's explore a few of these to gain a better understanding.
Graphical Representation
A graphical representation can help us understand where the square root of 116 lies on the number line. Since is 10 and is 11, the square root of 116 will be between these two values.
On a number line, it can be shown as:

Here, the exact position of can be approximated to 10.77.
Example Problems
Let's go through some practical examples to better understand the application of the square root of 116:
-
Example 1:
If you place a ladder 10 inches high and 4 inches away from the wall, what is the length of the ladder?
Using the Pythagorean theorem: -
Example 2:
What is the least number that should be multiplied to 116 to make it a perfect square?
Prime factorizing 116 gives us .
Multiplying by 29 gives:
Square Root Simplification
Simplifying the square root of 116 gives us . This representation is useful for various mathematical computations and visualizations.
Application of Square Root of 116 in Geometry
The square root of 116 has various applications in geometry, particularly when dealing with measurements that involve irrational numbers. Here are some examples and detailed explanations:
-
Diagonal of a Square:
Consider a square with each side of length \(a\). The length of the diagonal \(d\) can be found using the Pythagorean theorem:
\(d = \sqrt{a^2 + a^2} = \sqrt{2a^2} = a\sqrt{2}\)
If the length of the diagonal is \( \sqrt{116} \), then:
\(a\sqrt{2} = \sqrt{116}\)
Solving for \(a\):
\(a = \sqrt{\frac{116}{2}} = \sqrt{58}\)
Thus, each side of the square would be \( \sqrt{58} \).
-
Right Triangle:
In a right triangle, if one of the legs is known and the hypotenuse is \( \sqrt{116} \), you can find the other leg.
Let \(a\) and \(b\) be the legs, and \(c\) be the hypotenuse:
\(c^2 = a^2 + b^2\)
If \(c = \sqrt{116}\), then:
\(116 = a^2 + b^2\)
Assuming \(a = 10\):
\(116 = 10^2 + b^2\)
\(116 = 100 + b^2\)
\(b^2 = 16\)
\(b = 4\)
Thus, the other leg \(b\) would be 4 units.
-
Circular Segment:
In the context of a circle, the square root of 116 can be used to determine the length of a chord. For example, if a chord is divided into two equal segments by a perpendicular bisector, each segment's length can be found using the Pythagorean theorem.
If the radius \(r\) is \( \sqrt{116} \) and the perpendicular bisector divides the chord into two segments of length \(x\):
\(r^2 = x^2 + \left(\frac{chord}{2}\right)^2\)
Assuming the chord is \(20\) units:
\(\left(\sqrt{116}\right)^2 = x^2 + \left(10\right)^2\)
116 = \(x^2 + 100\)
x^2 = 16
x = 4
Therefore, each segment of the chord is 4 units long.
Comparisons with Other Square Roots
Understanding the square root of 116 in relation to other square roots can provide a clearer picture of its magnitude and characteristics. Here, we will compare
-
Square Root of 100:
The square root of 100 is a perfect square,
\( \sqrt{100} = 10 \) . Compared to 116, 100 is the nearest perfect square less than 116. -
Square Root of 121:
The square root of 121 is another perfect square,
\( \sqrt{121} = 11 \) . This is the closest perfect square greater than 116. -
Decimal Comparisons:
The square root of 116 in decimal form is approximately
\( 10.77 \) . This value lies between 10 and 11, specifically:-
\( 10 < \sqrt{116} < 11 \) -
\( \sqrt{100} < \sqrt{116} < \sqrt{121} \)
-
-
Comparison with Nearby Non-Perfect Squares:
Comparing with non-perfect squares near 116:
-
\( \sqrt{115} \approx 10.72 \) -
\( \sqrt{117} \approx 10.82 \)
-
These comparisons help illustrate the irrational nature of
| Number | Square Root | Type |
|---|---|---|
| 100 | 10 | Perfect Square |
| 115 | 10.72 | Non-Perfect Square |
| 116 | 10.77 | Non-Perfect Square |
| 117 | 10.82 | Non-Perfect Square |
| 121 | 11 | Perfect Square |
In summary, the square root of 116, or
Common Mistakes to Avoid
When working with the square root of 116, students and enthusiasts often make several common mistakes. Here are some pitfalls to be aware of and how to avoid them:
- Incorrect Simplification: A frequent mistake is failing to simplify the square root correctly. The correct simplified form of the square root of 116 is \(2\sqrt{29}\). Ensure you factor 116 properly as \(2^2 \times 29\) and then take the square root of the perfect square.
- Forgetting the Radical Form: Another common error is omitting the radical form entirely and only using the decimal approximation. Remember that while \(\sqrt{116} \approx 10.770\), the exact radical form is important in many mathematical contexts.
- Misidentifying Perfect Squares: When simplifying square roots, it's crucial to correctly identify and use perfect squares. For 116, the largest perfect square factor is 4. Misidentifying factors can lead to incorrect simplification.
- Rounding Errors: When using the decimal form, rounding errors can occur. It’s important to know when and how to round correctly to maintain precision. The square root of 116 is approximately 10.770, and rounding should be done according to the required precision level.
- Confusing Rational and Irrational Numbers: The square root of 116 is an irrational number, meaning it cannot be expressed as a simple fraction. Ensure you recognize the nature of the number to avoid mistakes in rational approximations.
By being aware of these common mistakes, you can approach problems involving the square root of 116 with greater accuracy and confidence.

Practice Problems and Solutions
Below are some practice problems and detailed solutions involving the square root of 116. These problems will help you understand the concept better and ensure you are comfortable with the calculations.
-
Problem 1: Simplify the square root of 116 in radical form.
Solution:
- List the factors of 116: 1, 2, 4, 29, 58, 116.
- Identify the largest perfect square factor: 4.
- Rewrite 116 as a product of 4 and another number: \(116 = 4 \times 29\).
- Simplify: \(\sqrt{116} = \sqrt{4 \times 29} = \sqrt{4} \times \sqrt{29} = 2\sqrt{29}\).
-
Problem 2: Find the length of the hypotenuse in a right triangle if one leg is 10 units and the other leg is 4 units.
Solution:
- Apply the Pythagorean theorem: \(a^2 + b^2 = c^2\).
- Substitute the given values: \(10^2 + 4^2 = c^2\).
- Calculate: \(100 + 16 = c^2\), so \(c^2 = 116\).
- Take the square root of both sides: \(c = \sqrt{116} = 2\sqrt{29} \approx 10.77\) units.
-
Problem 3: Convert the square root of 116 to its decimal form and round to three decimal places.
Solution:
- Using a calculator, find the decimal value of \(\sqrt{116}\).
- The result is approximately \(10.7703\).
- Rounded to three decimal places, \(\sqrt{116} \approx 10.770\).
-
Problem 4: Verify if 116 is a perfect square and explain why or why not.
Solution:
- A number is a perfect square if its square root is an integer.
- Calculate \(\sqrt{116}\).
- \(\sqrt{116} \approx 10.77\), which is not an integer.
- Therefore, 116 is not a perfect square.
-
Problem 5: Determine the least number that must be multiplied to 116 to make it a perfect square.
Solution:
- Prime factorize 116: \(116 = 2^2 \times 29\).
- To make it a perfect square, each prime factor must have an even exponent.
- 29 has an odd exponent, so multiply by 29 to balance it: \(116 \times 29 = 3364\).
- Check: \(\sqrt{3364} = 58\), which is an integer.
- Thus, multiplying 116 by 29 makes it a perfect square.
These practice problems cover various aspects of working with the square root of 116, helping you to master the concept and avoid common mistakes.
SQUARE ROOT OF 116
Saxon Math Algebra 1 - Lesson 116 - Quotient Rule for Square Roots
Saxon Math - Algebra 1: 3rd Edition (Lesson 116 - Quotient Rule for Square Roots)
DeltaMath: Exponential to Radical Form
How to Simplify the Square Root of 112: sqrt(112)
Math 116 Sec 8.2 Part B New
READ MORE: