Simplifying Square Roots of Fractions: A Comprehensive Guide

Simplifying the square roots of fractions involves a series of steps to ensure the fraction is in its simplest form. Here, we break down the process and provide examples for better understanding.

Steps to Simplify Square Roots of Fractions

1. Simplify the Square Root

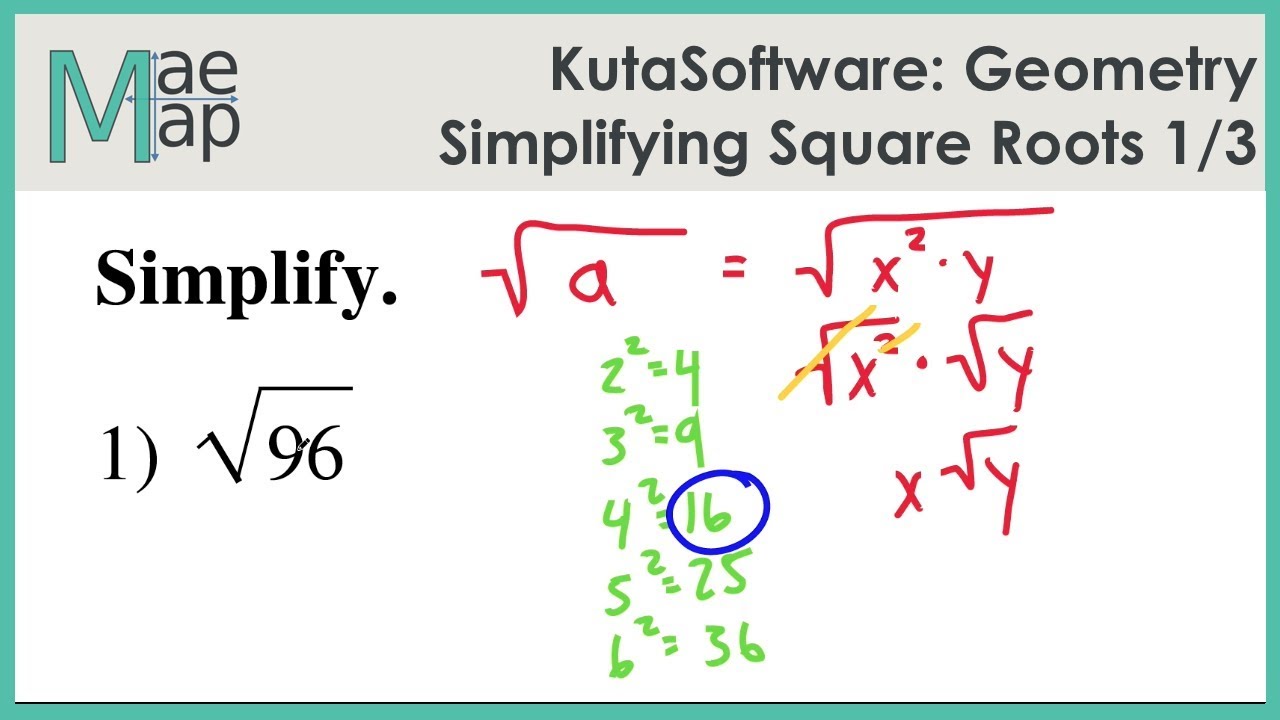
   Find the largest perfect square that can be factored out of the square root.

   Example: \( \sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2} \)

2. Rationalize the Denominator

   Eliminate any square roots in the denominator by multiplying the numerator and the denominator by the conjugate of the denominator.

   Example: \( \frac{5}{\sqrt{3}} = \frac{5}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{5\sqrt{3}}{3} \)

Examples of Simplifying Fractions with Square Roots

Example 1:

Simplify \( \frac{\sqrt{18}}{3} \)

Step 1: \( \sqrt{18} = \sqrt{9 \times 2} = 3\sqrt{2} \)

Step 2: \( \frac{\sqrt{18}}{3} = \frac{3\sqrt{2}}{3} = \sqrt{2} \)

Example 2:

Simplify \( \frac{2}{\sqrt{12}} \)

Step 1: \( \sqrt{12} = \sqrt{4 \times 3} = 2\sqrt{3} \)

Step 2: \( \frac{2}{\sqrt{12}} = \frac{2}{2\sqrt{3}} = \frac{1}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{\sqrt{3}}{3} \)

Example 3:

Simplify \( \frac{\sqrt{6}}{\sqrt{2}} \)

Step 1: \( \sqrt{6} = \sqrt{2 \times 3} = \sqrt{2} \times \sqrt{3} \)

Step 2: \( \frac{\sqrt{6}}{\sqrt{2}} = \frac{\sqrt{2} \times \sqrt{3}}{\sqrt{2}} = \sqrt{3} \)

Additional Practice Examples

• Simplify \( \frac{3\sqrt{5}}{5} \): \( \frac{3\sqrt{5}}{5} = \frac{3}{5} \sqrt{5} \)
• Simplify \( \frac{4}{\sqrt{18}} \): \( \sqrt{18} = 3\sqrt{2} \), so \( \frac{4}{\sqrt{18}} = \frac{4}{3\sqrt{2}} = \frac{4\sqrt{2}}{6} = \frac{2\sqrt{2}}{3} \)

Summary

By simplifying square roots and rationalizing the denominator, you can reduce fractions with square roots to their simplest forms. Regular practice will make the process easier and more intuitive.

Simplifying square roots of fractions is an essential skill in mathematics that helps in making complex expressions more manageable. This process involves breaking down the square roots into simpler forms and rationalizing denominators to eliminate any square roots from the denominator. In this guide, we will explore the step-by-step methods to simplify square roots of fractions, including examples and practical applications. By mastering these techniques, you will enhance your mathematical proficiency and problem-solving abilities.

Simplifying square roots of fractions involves breaking down both the numerator and the denominator into their prime factors, applying the square root to each part separately, and then simplifying the resulting expression.

1. Identify the fraction under the square root, for example, \( \sqrt{\frac{18}{50}} \).
2. Simplify the fraction inside the square root if possible:
   • For example, \( \frac{18}{50} \) can be simplified to \( \frac{9}{25} \).
3. Apply the square root to the numerator and the denominator separately:
   • \( \sqrt{\frac{9}{25}} = \frac{\sqrt{9}}{\sqrt{25}} \).
4. Simplify the square roots:
   • \( \sqrt{9} = 3 \) and \( \sqrt{25} = 5 \).
5. Combine the simplified roots:
   • \( \sqrt{\frac{9}{25}} = \frac{3}{5} \).

This process ensures that the fraction under the square root is simplified to its simplest form. Here are additional steps to consider:

1. If dealing with mixed numbers, convert them to improper fractions before applying the square root.
2. If the fraction has a radical in the denominator, rationalize the denominator by multiplying both the numerator and the denominator by the appropriate value to eliminate the radical.

By following these steps, you can simplify any square root of a fraction efficiently and accurately.

When simplifying square roots of fractions, certain special cases and techniques can make the process easier. Understanding these methods allows for more efficient simplification and accurate results.

1. Simplifying Radicals Involving Variables:
   • Identify and separate perfect square factors from non-perfect square factors within the radicand.
   • Example: \(\sqrt{a^6b^{10}}\) simplifies to \(a^3b^5\).
2. Quotient Property of Square Roots:

   The square root of a quotient is equal to the quotient of the square roots.

   • Example: \(\sqrt{\frac{36}{4}} = \frac{\sqrt{36}}{\sqrt{4}} = 3\).
3. Handling Negative Radicands:
   • When simplifying square roots of expressions involving negative radicands, the result will include an imaginary unit \(i\).
   • Example: \(\sqrt{-16} = 4i\).
4. Simplifying Complex Radicals:
   • Break down complex radicals into simpler components using the product and quotient properties of square roots.
   • Example: \(\sqrt{75}\) can be simplified as \(\sqrt{25 \cdot 3} = 5\sqrt{3}\).

Applying these special cases and techniques helps streamline the process of simplifying square roots of fractions, ensuring accurate and simplified results in mathematical computations.

To master simplifying square roots of fractions, practice is essential. Here are several problems that will help you understand and apply the concepts:

1. Simplify \( \frac{\sqrt{50}}{5} \)
2. Simplify \( \frac{3}{\sqrt{27}} \)
3. Simplify \( \sqrt{\frac{18}{50}} \)
4. Simplify \( \sqrt{1\frac{13}{36}} \)
5. Simplify \( \frac{4}{\sqrt{12}} \)

For each problem, follow these steps:

• Simplify the square root: Break down the number under the square root into its prime factors or perfect squares.
• Rationalize the denominator: If there is a square root in the denominator, multiply the numerator and the denominator by the square root to eliminate it.

Let's work through the first example:

Practice these steps with each problem to improve your skills.

When simplifying square roots of fractions, it's common to encounter a few pitfalls. Here are some mistakes to watch out for and how to steer clear of them:

1. Forgetting to Simplify the Square Root: One of the most frequent errors is neglecting to simplify the square root completely. Always ensure that the square root is simplified as much as possible before proceeding.
2. Missing Rationalizing the Denominator: When dealing with fractions containing square roots, failing to rationalize the denominator can lead to incorrect results. Remember to multiply the numerator and denominator by the conjugate of the denominator to eliminate the square root from the denominator.
3. Overlooking Perfect Square Factors: Sometimes, perfect square factors within the radicand are overlooked, resulting in incomplete simplification. Be thorough in identifying and factoring out perfect square numbers from the radicand.
4. Ignoring Mixed Numbers: If dealing with mixed numbers, it's essential to convert them to improper fractions before simplifying. Failure to do so can lead to errors in the simplification process.
5. Forgetting to Apply Quotient Rule: In cases where the fraction contains a square root in both the numerator and denominator, forgetting to apply the quotient rule can lead to complications. Remember to divide both the numerator and denominator by the square root in the denominator to simplify the expression.
6. Incorrectly Combining Radicals: Combining radicals without proper consideration of their terms can result in incorrect simplifications. Always ensure that the radicals being combined have the same radicand before combining them.
7. Misapplying Advanced Techniques: While advanced techniques can be useful, misapplying them can lead to errors. Make sure to thoroughly understand the principles behind each technique before attempting to use them.

By being aware of these common mistakes and following the corresponding strategies to avoid them, you can streamline the process of simplifying square roots of fractions and minimize errors in your calculations.

When it comes to simplifying square roots of fractions, mastering advanced techniques can greatly enhance your efficiency and accuracy. Here are some advanced methods to consider:

1. Combining Radicals: Look for opportunities to combine like terms under the same radical. This involves adding or subtracting radicals with identical radicands, simplifying the expression while preserving its value.
2. Working with Surds: Surds are expressions containing square roots. Understanding how to manipulate surds can lead to more elegant simplifications. Practice operations such as addition, subtraction, multiplication, and division involving surds to gain proficiency.
3. Applying the Quotient Rule: When faced with a fraction containing a square root in both the numerator and denominator, applying the quotient rule can facilitate simplification. Divide both the numerator and denominator by the square root in the denominator to rationalize the expression.
4. Utilizing Advanced Factoring: Employ advanced factoring techniques, such as difference of squares or perfect square trinomials, to simplify complex expressions involving square roots. Factor out common factors to reveal opportunities for simplification.
5. Employing Conjugates: Utilize conjugates to rationalize denominators efficiently. Multiply both the numerator and denominator by the conjugate of the denominator to eliminate square roots from the denominator, resulting in a simplified expression.
6. Understanding Nested Radicals: Nested radicals contain square roots within other square roots. Develop a clear understanding of how to simplify expressions with nested radicals, unraveling the layers systematically to achieve the simplest form.
7. Considering Binomial Expansion: In some cases, binomial expansion can be employed to simplify expressions involving square roots. Expand binomials using techniques like Pascal's triangle or the binomial theorem to reveal patterns and facilitate simplification.

By mastering these advanced simplification techniques, you can tackle even the most complex fractions containing square roots with confidence and precision, efficiently arriving at simplified forms while avoiding common pitfalls.

In conclusion, mastering the art of simplifying square roots of fractions is a valuable skill that can enhance your mathematical proficiency and problem-solving abilities. By following the step-by-step techniques outlined in this guide, you can confidently tackle fractions containing square roots, streamline the simplification process, and minimize errors.

From understanding the fundamentals of square roots and fractions to employing advanced techniques such as rationalizing the denominator and combining radicals, each step plays a crucial role in achieving accurate and simplified expressions.

Remember to always simplify square roots as much as possible, rationalize the denominator when necessary, and be vigilant of common mistakes to avoid errors. Practice regularly to reinforce your understanding and fluency in simplifying square roots of fractions.

With dedication and perseverance, you can become proficient in simplifying even the most complex expressions involving square roots of fractions, empowering yourself to confidently tackle mathematical challenges with ease.