Topic simplifying fractions with square roots: Simplifying fractions with square roots can seem complex, but with the right steps, it becomes manageable. This article provides a comprehensive guide to help you master this concept. We will cover the process of simplifying square roots, rationalizing the denominator, and working through various examples. By the end of this guide, you'll be simplifying fractions with square roots with confidence.
Table of Content
- Simplifying Fractions with Square Roots
- Introduction
- Table of Contents
- Understanding Square Roots
- Basic Simplification Techniques
- Simplifying Fractions with Square Roots in the Numerator
- Simplifying Fractions with Square Roots in the Denominator
- Rationalizing the Denominator
- Examples and Practice Problems
- Common Mistakes and How to Avoid Them
- Advanced Techniques
- Applications in Algebra and Beyond
- Detailed Topics
- YOUTUBE: Học cách rút gọn phân số có chứa căn dưới dạng hợp pháp và hiệu quả với video 'Simplify a fraction under a square root - Cách rút gọn phân số dưới dấu căn - Phương pháp hợp pháp trong toán học'.
Simplifying Fractions with Square Roots
Simplifying fractions that contain square roots can be broken down into a series of steps. These steps ensure the fraction is in its simplest form by eliminating square roots from the denominator and simplifying the square roots in the numerator and denominator.
Steps to Simplify Fractions with Square Roots
-
Simplify the Square Root
Find the largest perfect square that can be factored out of the square root. For example, for \(\sqrt{72}\), the largest perfect square is 36:
\(\sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2}\)
-
Rationalize the Denominator
To eliminate the square root from the denominator, multiply the numerator and denominator by the conjugate of the denominator. For instance, to simplify \(\frac{5}{\sqrt{3}}\), multiply by \(\frac{\sqrt{3}}{\sqrt{3}}\):
\(\frac{5}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{5\sqrt{3}}{3}\)
Examples
-
Example 1: Simplify \(\frac{\sqrt{18}}{3}\)
First, simplify the square root:
\(\sqrt{18} = \sqrt{9 \times 2} = 3\sqrt{2}\)
Then, simplify the fraction:
\(\frac{3\sqrt{2}}{3} = \sqrt{2}\)
-
Example 2: Simplify \(\frac{2}{\sqrt{12}}\)
\(\sqrt{12} = \sqrt{4 \times 3} = 2\sqrt{3}\)
Then, rationalize the denominator:
\(\frac{2}{\sqrt{12}} \times \frac{\sqrt{12}}{\sqrt{12}} = \frac{2\sqrt{12}}{12} = \frac{\sqrt{3}}{3}\)
-
Example 3: Simplify \(\frac{\sqrt{6}}{\sqrt{2}}\)
Simplify the square roots:
\(\sqrt{6} = \sqrt{2 \times 3} = \sqrt{2} \times \sqrt{3}\)
\(\frac{\sqrt{6}}{\sqrt{2}} = \frac{\sqrt{2} \times \sqrt{3}}{\sqrt{2}} = \sqrt{3}\)
Practice Problems
Here are some additional examples for practice:
- Simplify \(\frac{3\sqrt{5}}{5}\)
- Simplify \(\frac{4}{\sqrt{18}}\)
Summary
Simplifying fractions with square roots involves two main steps: simplifying the square roots and rationalizing the denominator. With practice, these processes become more intuitive, enabling you to simplify any fraction with square roots effectively.

READ MORE:
Introduction
Simplifying fractions with square roots is a fundamental skill in mathematics that helps in reducing complex expressions to their simplest forms. This process involves simplifying the square roots in both the numerator and the denominator, and often requires rationalizing the denominator to eliminate the square root. In this guide, we will walk you through the detailed steps of simplifying fractions that contain square roots, providing examples and tips to make the process clear and easy to understand.
- Simplifying the Square Root
- Rationalizing the Denominator
- Step-by-Step Examples
- Practice Problems
Table of Contents
- Introduction
- Understanding Square Roots in Fractions
- Basic Rules for Simplifying Square Roots
- Product Rule
- Quotient Rule
- Steps to Simplify Fractions with Square Roots
- Simplifying the Square Root
- Rationalizing the Denominator
- Examples of Simplifying Fractions with Square Roots
- Example 1: Simplifying √18/3
- Example 2: Simplifying 2/√12
- Example 3: Simplifying √6/√2
- Practice Problems
- Common Mistakes to Avoid
- Conclusion
Understanding Square Roots
Simplifying fractions with square roots involves reducing the fraction to its simplest form by addressing the square roots present in the numerator or denominator. This process typically requires two main steps: simplifying the square root and rationalizing the denominator.
-
Simplifying the Square Root:
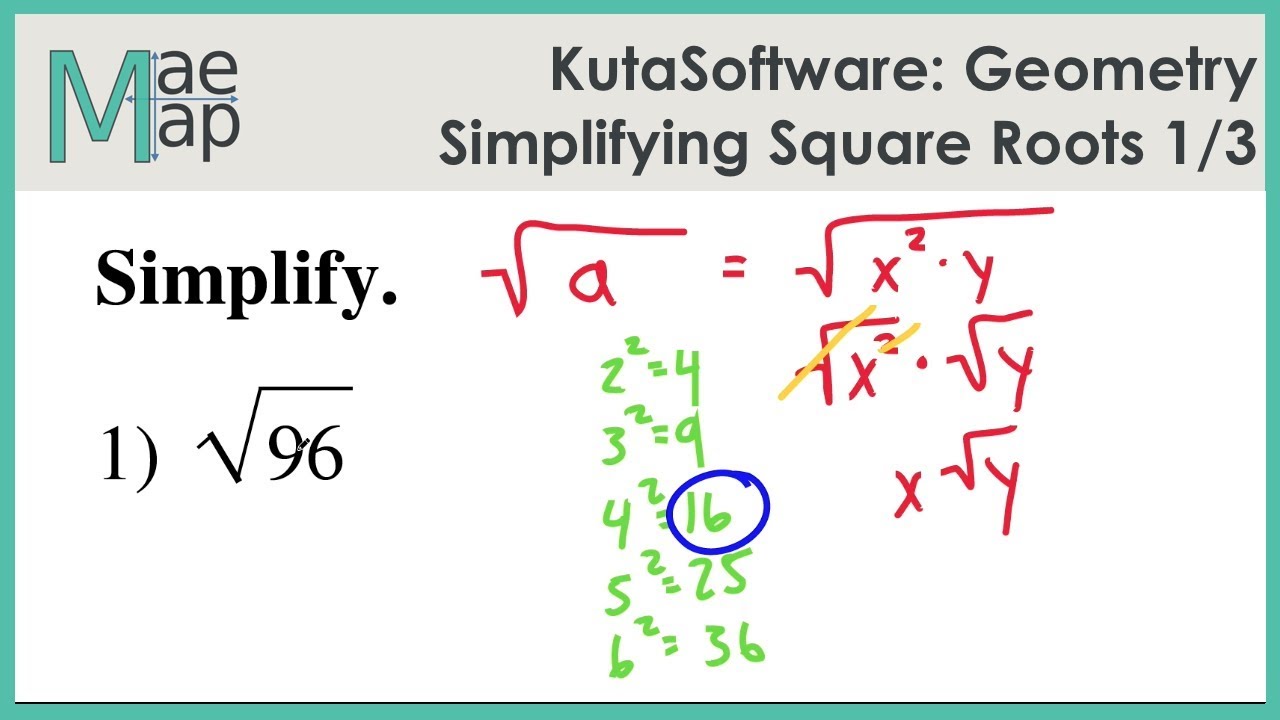
To simplify a square root, factorize the number inside the square root to find the largest perfect square. For example, √72 can be simplified as follows:
- \(\sqrt{72} = \sqrt{36 \times 2} = \sqrt{36} \times \sqrt{2} = 6\sqrt{2}\)
-
Rationalizing the Denominator:
Rationalizing involves eliminating the square root from the denominator. This is done by multiplying both the numerator and the denominator by the conjugate of the denominator. For example, simplifying \(\frac{5}{\sqrt{3}}\) involves the following steps:
- \(\frac{5}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{5\sqrt{3}}{3}\)
With practice, these steps become easier, allowing you to handle various fractions containing square roots confidently. Below are some additional examples:
| Example | Solution |
|---|---|
| Simplify \(\sqrt{18}/3\) | \(\sqrt{18}/3 = (3\sqrt{2})/3 = \sqrt{2}\) |
| Simplify \(2/\sqrt{12}\) | \(2/\sqrt{12} = (2/\sqrt{12}) \times (\sqrt{12}/\sqrt{12}) = 2\sqrt{12}/12 = \sqrt{3}/3\) |
| Simplify \(\sqrt{6}/\sqrt{2}\) | \(\sqrt{6}/\sqrt{2} = (\sqrt{2} \times \sqrt{3})/\sqrt{2} = \sqrt{3}\) |
Basic Simplification Techniques
Simplifying fractions with square roots involves a series of straightforward steps to make the expression easier to work with. Below are the key techniques used in the process:
- Simplify the Square Root:
- Identify and extract the largest perfect square from the square root.
- For example, \(\sqrt{72}\) can be simplified to \(6\sqrt{2}\) because \(72 = 36 \times 2\) and \(\sqrt{36} = 6\).
- Rationalize the Denominator:
- Eliminate the square root from the denominator by multiplying both the numerator and the denominator by the conjugate of the denominator.
- For example, to simplify \(\frac{5}{\sqrt{3}}\), multiply by \(\frac{\sqrt{3}}{\sqrt{3}}\) to get \(\frac{5\sqrt{3}}{3}\).
- Combine Like Terms:
- After rationalizing, combine like terms if possible to simplify further.
- For example, simplifying \(\frac{\sqrt{6}}{\sqrt{2}}\) results in \(\sqrt{3}\) because \(\sqrt{6} = \sqrt{2 \times 3} = \sqrt{2} \times \sqrt{3}\).
By following these steps, you can simplify fractions containing square roots to their simplest forms, making calculations easier and more manageable.

Simplifying Fractions with Square Roots in the Numerator
When simplifying fractions with square roots in the numerator, it is important to follow systematic steps to ensure accurate results. Here is a detailed approach:
-
Simplify the Square Root: Start by simplifying the square root in the numerator, if possible.
Example: \(\frac{\sqrt{54}}{6}\) can be simplified to \(\frac{\sqrt{9} \cdot \sqrt{6}}{6} = \frac{3\sqrt{6}}{6} = \frac{\sqrt{6}}{2}\)
-
Remove Common Factors: Look for common factors in the numerator and the denominator.
Example: \(\frac{\sqrt{32}}{8}\) simplifies to \(\frac{\sqrt{16} \cdot \sqrt{2}}{8} = \frac{4\sqrt{2}}{8} = \frac{\sqrt{2}}{2}\)
-
Use the Quotient Rule: Apply the quotient rule for square roots to simplify expressions.
Example: \(\frac{\sqrt{27}}{\sqrt{75}}\) can be rewritten using the quotient property as \(\sqrt{\frac{27}{75}} = \sqrt{\frac{9}{25}} = \frac{3}{5}\)
-
Rationalize the Denominator (if needed): If the fraction has a square root in the denominator, multiply the numerator and the denominator by the conjugate or an appropriate value to rationalize the denominator.
Example: \(\frac{5}{\sqrt{2}}\) can be rationalized by multiplying both the numerator and denominator by \(\sqrt{2}\) to get \(\frac{5\sqrt{2}}{2}\)
By following these steps, you can systematically simplify fractions with square roots in the numerator, ensuring accurate and simplified results.
Simplifying Fractions with Square Roots in the Denominator
Simplifying fractions with square roots in the denominator is an essential skill in algebra. The goal is to eliminate the square root from the denominator to make the fraction easier to work with. This process is known as rationalizing the denominator.
Here are the detailed steps to simplify fractions with square roots in the denominator:
-
Identify the Square Root:
Find the square root in the denominator that needs to be rationalized. For example, in the fraction \( \frac{1}{\sqrt{2}} \), the square root of 2 is in the denominator.
-
Multiply by the Conjugate:
Multiply both the numerator and the denominator by the conjugate of the denominator. The conjugate of a term like \( \sqrt{a} \) is \( \sqrt{a} \) itself, while the conjugate of a binomial like \( a + \sqrt{b} \) is \( a - \sqrt{b} \).
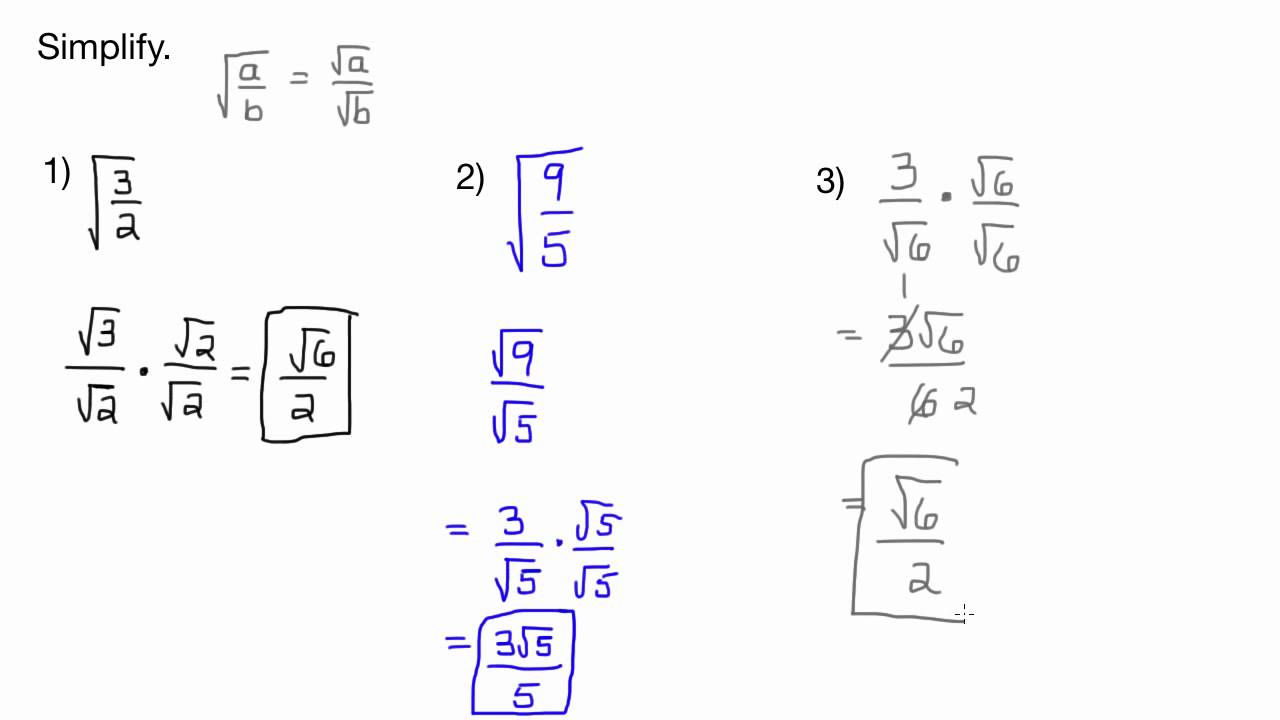
- Example: \( \frac{1}{\sqrt{2}} \times \frac{\sqrt{2}}{\sqrt{2}} = \frac{\sqrt{2}}{2} \)
- Example: \( \frac{3}{2 + \sqrt{5}} \times \frac{2 - \sqrt{5}}{2 - \sqrt{5}} = \frac{3(2 - \sqrt{5})}{(2)^2 - (\sqrt{5})^2} = \frac{3(2 - \sqrt{5})}{4 - 5} = \frac{3(2 - \sqrt{5})}{-1} = -3(2 - \sqrt{5}) \)
-
Simplify the Expression:
After multiplying, simplify the resulting fraction by performing the necessary arithmetic operations in both the numerator and the denominator.
- Example: \( \frac{\sqrt{2}}{2} \) is already in simplest form.
- Example: \( -3(2 - \sqrt{5}) = -6 + 3\sqrt{5} \)
Rationalizing the denominator not only simplifies the fraction but also makes further mathematical operations more straightforward.
Rationalizing the Denominator
Rationalizing the denominator is a technique used to eliminate square roots or other radical expressions from the denominator of a fraction. This process is particularly useful when simplifying fractions involving square roots, as it helps to make the expression easier to work with.
The general idea behind rationalizing the denominator is to multiply both the numerator and denominator of the fraction by a carefully chosen expression in order to remove the radical from the denominator while maintaining the value of the fraction.
There are different methods for rationalizing the denominator, depending on the type of radical expression present. One common method involves multiplying the fraction by a form of 1 that cleverly introduces the conjugate of the denominator, thereby eliminating the radical.
For example, if we have a fraction like \(\frac{1}{\sqrt{2}}\), we can rationalize the denominator by multiplying both the numerator and denominator by the conjugate of the denominator, which in this case is \(\sqrt{2}\). This results in: \(\frac{1 \times \sqrt{2}}{\sqrt{2} \times \sqrt{2}} = \frac{\sqrt{2}}{2}\).
Another method for rationalizing the denominator involves using rational exponents to rewrite the radical expression in a different form, making it easier to simplify.
When dealing with more complex fractions or radical expressions, it may be necessary to apply additional algebraic techniques or properties of radicals to fully rationalize the denominator.
Examples and Practice Problems
Below are some examples and practice problems to help reinforce the concepts of simplifying fractions with square roots:
-
Example 1:
Simplify the fraction \(\frac{\sqrt{18}}{3}\).
Solution:
We can simplify \(\sqrt{18}\) by breaking it down into its prime factors: \(\sqrt{18} = \sqrt{2 \times 9} = \sqrt{2 \times 3^2} = 3\sqrt{2}\).
So, the fraction becomes \(\frac{3\sqrt{2}}{3}\).
Now, we cancel out the common factor of 3 in the numerator and denominator:
\(\frac{3\cancel{\sqrt{2}}}{\cancel{3}} = \sqrt{2}\).
Therefore, the simplified fraction is \(\sqrt{2}\).
-
Example 2:
Simplify the fraction \(\frac{2}{\sqrt{5}}\).
Solution:
To rationalize the denominator, we multiply both the numerator and denominator by \(\sqrt{5}\):
\(\frac{2 \times \sqrt{5}}{\sqrt{5} \times \sqrt{5}} = \frac{2\sqrt{5}}{5}\).
Therefore, the simplified fraction is \(\frac{2\sqrt{5}}{5}\).
-
Practice Problem:
Simplify the fraction \(\frac{\sqrt{50}}{10}\).
-
Practice Problem:
Simplify the fraction \(\frac{3}{\sqrt{12}}\).
Common Mistakes and How to Avoid Them
While simplifying fractions with square roots, it's common to encounter certain mistakes. Here are some of the most common mistakes and how to avoid them:
-
Mistake:
Forgetting to rationalize the denominator.
How to Avoid:Always remember to rationalize the denominator whenever you have a square root or other radical expression in the denominator. This ensures that the fraction is simplified properly and is in its simplest form.
-
Mistake:
Incorrectly simplifying radicals.
How to Avoid:When simplifying radicals, make sure to correctly identify perfect square factors and simplify them. Be careful not to overlook any perfect squares or make errors in simplification.
-
Mistake:
Misunderstanding when to use rational exponents.
How to Avoid:Understand the situations where using rational exponents can simplify the process of rationalizing the denominator. Practice identifying when it's beneficial to rewrite radical expressions using rational exponents.
-
Mistake:
Skipping steps in the simplification process.
How to Avoid:Take your time and ensure that you follow each step carefully when simplifying fractions with square roots. Skipping steps can lead to errors, so it's important to be thorough in your work.
-
Mistake:
Not double-checking the final answer.
How to Avoid:After simplifying a fraction, always double-check your final answer to ensure accuracy. Review each step of the simplification process and verify that the fraction is in its simplest form.
Advanced Techniques
For those looking to delve deeper into simplifying fractions with square roots, here are some advanced techniques to explore:
-
Technique:
Simplifying Complex Fractions
Overview:Complex fractions involve fractions within fractions, and simplifying them requires careful manipulation of numerator and denominator expressions. Advanced techniques may include breaking down complex fractions into simpler components, applying common denominators, and simplifying individual components before reassembling the fraction.
-
Technique:
Working with Variables and Radicals
Overview:When variables are introduced alongside radicals in fractions, the simplification process becomes more intricate. Advanced techniques may involve isolating variables, applying algebraic operations to radicals, and considering the properties of exponents to simplify expressions involving variables and radicals.
-
Technique:
Application in Higher Mathematics
Overview:Simplifying fractions with square roots has applications beyond basic arithmetic and algebra. In higher mathematics, such as calculus, linear algebra, and differential equations, understanding how to simplify and manipulate fractions with square roots is essential for solving complex problems and proving theorems. Advanced techniques may involve applying these concepts in various mathematical contexts to solve advanced problems.
Applications in Algebra and Beyond
The concepts of simplifying fractions with square roots have widespread applications in various fields beyond basic arithmetic and algebra. Here are some examples:
-
Application:
Solving Algebraic Equations
Overview:When solving algebraic equations involving square roots, the ability to simplify fractions with square roots is crucial. Whether it's solving quadratic equations or higher-order polynomial equations, understanding how to simplify and manipulate fractions with square roots helps in isolating variables and finding solutions.
-
Application:
Applications in Geometry
Overview:In geometry, fractions with square roots often arise when dealing with geometric shapes and measurements. Whether it's calculating the lengths of sides in right triangles, finding areas of circles or other curved shapes, or determining volumes of solids, the ability to simplify fractions with square roots is essential for accurate calculations and geometric proofs.
-
Application:
Real-World Problems
Overview:Beyond mathematics, the skills acquired in simplifying fractions with square roots can be applied to real-world problems in various fields such as physics, engineering, finance, and computer science. Whether it's calculating forces in mechanical systems, analyzing data in scientific research, or optimizing algorithms in computer programming, the ability to simplify and manipulate fractions with square roots is invaluable.
Detailed Topics
-
Understanding Square Roots
Definition of Square Roots
Properties of Square Roots
Perfect Squares
-
Basic Simplification Techniques
Prime Factorization
Simplifying Radicals
Combining Like Terms
-
Simplifying Fractions with Square Roots in the Numerator
Basic Examples
Step-by-Step Process
-
Simplifying Fractions with Square Roots in the Denominator
Importance of Rationalizing the Denominator
Techniques for Rationalization
-
Rationalizing the Denominator
Understanding Conjugates
Examples with Simple Denominators
Examples with Complex Denominators
-
Examples and Practice Problems
Basic Problems
Intermediate Problems
Advanced Problems
-
Common Mistakes and How to Avoid Them
Typical Errors in Simplification
Misunderstanding Rationalization
How to Double-Check Your Work
-
Advanced Techniques
Simplifying Complex Fractions
Working with Variables and Radicals
Application in Higher Mathematics
-
Applications in Algebra and Beyond
Solving Algebraic Equations
Applications in Geometry
Real-World Problems

Học cách rút gọn phân số có chứa căn dưới dạng hợp pháp và hiệu quả với video 'Simplify a fraction under a square root - Cách rút gọn phân số dưới dấu căn - Phương pháp hợp pháp trong toán học'.
Simplify a fraction under a square root - Cách rút gọn phân số dưới dấu căn - Phương pháp hợp pháp trong toán học
READ MORE:
Học cách rút gọn căn bậc hai của phân số với video 'Rút gọn căn bậc hai của Phân số: căn bậc hai của (a/b), a/căn bậc hai của b'.
Rút gọn căn bậc hai của Phân số: căn bậc hai của (a/b), a/căn bậc hai của b