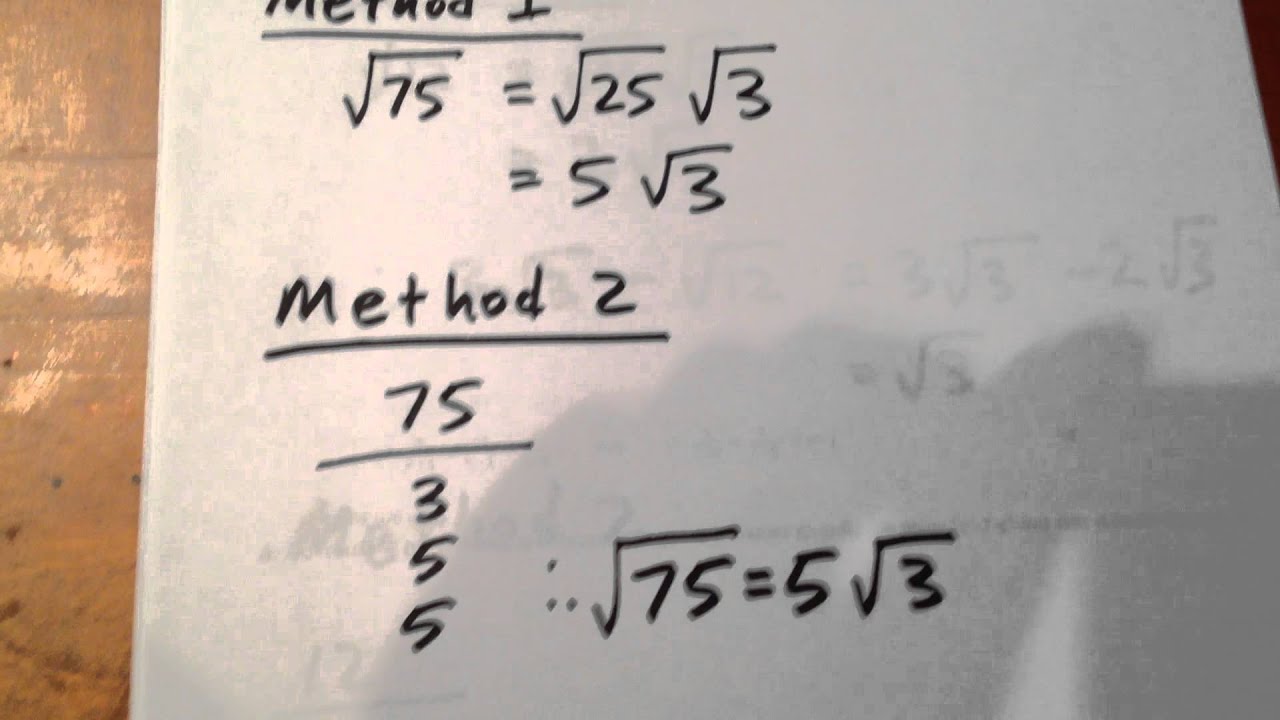Simplify Square Root 108: Easy Step-by-Step Guide

To simplify the square root of 108, we need to factor it into its prime components and look for perfect squares.

Step-by-Step Solution

1. Find the prime factorization of 108.

\[ 108 = 2^2 \times 3^3 \]

2. Rewrite the square root of 108 using its prime factors.

\[ \sqrt{108} = \sqrt{2^2 \times 3^3} \]

3. Separate the factors into two groups: perfect squares and non-perfect squares.

\[ \sqrt{108} = \sqrt{2^2} \times \sqrt{3^3} = 2 \times \sqrt{27} \]

4. Simplify further by factoring out perfect squares from 27.

\[ \sqrt{27} = \sqrt{3^3} = \sqrt{3^2 \times 3} = 3 \times \sqrt{3} \]

5. Combine the results to get the final simplified form.

\[ 2 \times 3 \times \sqrt{3} = 6\sqrt{3} \]

Thus, the simplified form of \(\sqrt{108}\) is \(6\sqrt{3}\).

The concept of square roots is fundamental in mathematics. A square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 9 is 3 because \(3 \times 3 = 9\).

Square roots are denoted by the radical symbol \(\sqrt{}\). The number inside the radical is called the radicand. For instance, in \(\sqrt{108}\), 108 is the radicand.

Square roots can be classified into two categories:

• Perfect Squares: Numbers whose square roots are integers. Examples include 1, 4, 9, 16, and 25.
• Non-Perfect Squares: Numbers whose square roots are not integers. Examples include 2, 3, 5, 10, and 108.

Understanding how to simplify square roots, especially non-perfect squares, is crucial. Simplification involves expressing the square root in its simplest form, often by factoring the radicand into its prime factors.

In this guide, we will focus on simplifying the square root of 108. By following a step-by-step approach, you will learn to break down the number into its prime components and simplify the expression effectively.

Prime factorization is the process of breaking down a composite number into its prime factors. Prime numbers are numbers greater than 1 that have no divisors other than 1 and themselves. Examples of prime numbers include 2, 3, 5, 7, and 11.

To understand prime factorization, follow these steps:

1. Identify the smallest prime number that divides the given number: Start with the smallest prime number, which is 2, and check if it divides the given number without leaving a remainder. If it does, it's a factor.
2. Divide the number by the prime factor: Divide the number by the identified prime factor to get a quotient.
3. Repeat the process with the quotient: Continue the process with the quotient, checking for divisibility by the same or next smallest prime number, until the quotient is a prime number.

Let's apply prime factorization to 108:

• Step 1: 108 is divisible by 2 (the smallest prime number).

  \[ 108 \div 2 = 54 \]

• Step 2: 54 is also divisible by 2.

  \[ 54 \div 2 = 27 \]

• Step 3: 27 is not divisible by 2. The next smallest prime number is 3.

  \[ 27 \div 3 = 9 \]

• Step 4: 9 is also divisible by 3.

  \[ 9 \div 3 = 3 \]

• Step 5: 3 is a prime number.

Thus, the prime factorization of 108 is:
\[ 108 = 2^2 \times 3^3 \]

Understanding prime factorization is crucial for simplifying square roots because it allows us to break down the radicand into smaller, manageable components.

Simplifying the square root of 108 involves breaking it down into its prime factors and then simplifying the expression. Follow these detailed steps to simplify \(\sqrt{108}\):

1. Find the Prime Factorization of 108:

   Prime factorization is the first step. We need to express 108 as a product of its prime factors.

   \[ 108 = 2^2 \times 3^3 \]

2. Express the Square Root Using Prime Factors:

   Rewrite the square root of 108 using its prime factors.

   \[ \sqrt{108} = \sqrt{2^2 \times 3^3} \]

3. Separate Perfect Squares from the Radicand:

   Identify and separate the perfect squares from the radicand.

   \[ \sqrt{2^2 \times 3^3} = \sqrt{2^2} \times \sqrt{3^3} \]

4. Simplify the Perfect Squares:

   Simplify the square root of the perfect squares.

   \[ \sqrt{2^2} = 2 \]

5. Simplify the Remaining Radicand:

   Break down the remaining radicand into manageable parts.

   \[ \sqrt{3^3} = \sqrt{3^2 \times 3} = \sqrt{3^2} \times \sqrt{3} = 3\sqrt{3} \]

6. Combine the Simplified Parts:

   Combine the simplified parts to get the final expression.

   \[ \sqrt{108} = 2 \times 3\sqrt{3} = 6\sqrt{3} \]

Thus, the simplified form of \(\sqrt{108}\) is \(6\sqrt{3}\). By following these steps, you can easily simplify the square root of any non-perfect square.

Prime factorization is the process of expressing a number as the product of its prime factors. To find the prime factorization of 108, we will divide it by the smallest prime numbers step by step.

1. Divide by 2:

   Since 108 is an even number, we start by dividing it by 2, the smallest prime number.

   \[ 108 \div 2 = 54 \]

2. Divide by 2 again:

   54 is also an even number, so we continue dividing by 2.

   \[ 54 \div 2 = 27 \]

3. Divide by 3:

   27 is not divisible by 2, so we move to the next smallest prime number, which is 3.

   \[ 27 \div 3 = 9 \]

4. Divide by 3 again:

   9 is divisible by 3.

   \[ 9 \div 3 = 3 \]

5. Prime number reached:

   The last quotient, 3, is a prime number.

We can now write 108 as the product of these prime factors:

\[ 108 = 2 \times 2 \times 3 \times 3 \times 3 \]

Or, expressed with exponents:

\[ 108 = 2^2 \times 3^3 \]

Prime factorization is a critical step in simplifying square roots, as it allows us to identify and separate perfect squares from the radicand.

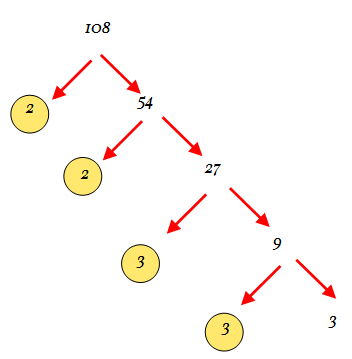
Identifying perfect squares is a crucial step in simplifying square roots. Perfect squares are numbers that can be expressed as the product of an integer with itself. For example, \(1, 4, 9,\) and \(16\) are perfect squares because they can be written as \(1^2, 2^2, 3^2,\) and \(4^2\) respectively.

To simplify the square root of a number like 108, we need to find the perfect squares within its prime factorization. Let's review the prime factorization of 108:

\[ 108 = 2^2 \times 3^3 \]

Next, we identify the perfect squares from these factors. A perfect square has even exponents in its prime factorization:

1. \(<2^2>\) is a perfect square because the exponent is even.
2. \(<3^2>\) is a perfect square because the exponent is even (note that \(3^3\) can be written as \(3^2 \times 3\)).

We now express \(108\) using these perfect squares:

\[ 108 = 2^2 \times 3^2 \times 3 \]

We can then separate the perfect squares from the remaining factors under the square root:

\[ \sqrt{108} = \sqrt{2^2 \times 3^2 \times 3} \]

We can simplify this by taking the square root of each perfect square:

\[ \sqrt{108} = \sqrt{2^2} \times \sqrt{3^2} \times \sqrt{3} = 2 \times 3 \times \sqrt{3} \]

Combining these results, we get:

\[ \sqrt{108} = 6\sqrt{3} \]

By identifying and extracting perfect squares, we simplify the square root of 108 to \(6\sqrt{3}\).

To simplify the square root of 108, we follow these detailed steps:

1. Factor 108 into its prime factors:

   First, we need to factorize 108. The prime factorization of 108 is:

   
   $$
   
   
   2
   2
   
   ×
   
   3
   3
   
   
   

   This means:

   
   $$
   
   108
   =
   2
   ×
   2
   ×
   3
   ×
   3
   ×
   3
   
   

2. Group the factors in pairs of identical numbers:

   We group the factors in pairs to find perfect squares:

   
   $$
   
   
   2
   2
   
   ×
   
   3
   2
   
   ×
   3
   
   

3. Extract the square roots of the perfect squares:

   We take the square root of the grouped factors:

   
   $$
   
   
   
   2
   2
   
   
   ×
   
   
   3
   2
   
   ×
   
   3
   
   
   

   This simplifies to:

   
   $$
   
   2
   ×
   3
   ×
   
   3
   
   
   

4. Combine the simplified terms:

   Multiplying the simplified terms, we get:

   
   $$
   
   6
   ×
   
   3
   
   
   

   So, the simplified form of the square root of 108 is:

   
   $$
   
   6
   
   3
   
   
   

Therefore, the simplified form of $\sqrt{108}$ is $6\sqrt{3}$.

To simplify the square root of 108, we need to combine the perfect square factors and the remaining factors. Here's a detailed step-by-step guide:

1. Identify the perfect square factors of 108. From the prime factorization, we have:

   \( 108 = 2^2 \times 3^3 \)

   The perfect squares here are \( 2^2 = 4 \) and \( 3^2 = 9 \). Multiplying these, we get \( 4 \times 9 = 36 \), which is the largest perfect square factor of 108.

2. Rewrite 108 as a product of the largest perfect square and the remaining factor:

   \( 108 = 36 \times 3 \)

3. Express the square root of 108 using the product rule for radicals:

   \( \sqrt{108} = \sqrt{36 \times 3} \)

4. Separate the square root of the product into the product of the square roots:

   \( \sqrt{108} = \sqrt{36} \times \sqrt{3} \)

5. Simplify the square root of the perfect square:

   \( \sqrt{36} = 6 \)

6. Combine the simplified square root of the perfect square with the remaining factor:

   \( \sqrt{108} = 6 \times \sqrt{3} \)

   So, the simplified form of \( \sqrt{108} \) is:

   \( 6\sqrt{3} \)

By following these steps, we have successfully combined the perfect square factor and the remaining factor to simplify the square root of 108 to \( 6\sqrt{3} \).

In the final steps of simplifying the square root of 108, we combine our previous work to get the simplest form of the expression. Here’s how we can do it step by step:

1. Identify Perfect Square Factors:

   We already know from our prime factorization that 108 can be expressed as \( 36 \times 3 \), where 36 is a perfect square.

2. Separate the Factors:

   Using the property of square roots that \(\sqrt{a \times b} = \sqrt{a} \times \sqrt{b}\), we can write:

   \[ \sqrt{108} = \sqrt{36 \times 3} \]

   Which can be separated into:

   \[ \sqrt{108} = \sqrt{36} \times \sqrt{3} \]

3. Calculate the Square Root of the Perfect Square:

   The square root of 36 is 6, so we can simplify this part as follows:

   \[ \sqrt{36} = 6 \]

4. Combine the Results:

   Now, we combine the square root of 36 with the remaining factor under the square root sign:

   \[ \sqrt{108} = 6 \times \sqrt{3} \]

5. Final Simplified Form:

   The simplest form of the square root of 108 is:

   \[ \sqrt{108} = 6\sqrt{3} \]

By following these steps, we have successfully simplified the square root of 108 to its simplest radical form, \( 6\sqrt{3} \).

Simplifying square roots can be tricky, and there are several common mistakes to be aware of:

• Forgetting to Check for Perfect Squares: Always look for the largest perfect square factor within the number under the square root. This helps simplify the expression significantly.
• Rushing the Process: Simplify step-by-step. Take your time to ensure each factor is correctly identified and simplified.
• Ignoring Prime Factorization: Breaking the number into its prime factors can make simplification easier. Factorize first, then simplify.
• Leaving Unnecessary Factors Inside the Root: Ensure all possible factors are simplified outside the square root to achieve the simplest form.
• Not Simplifying Completely: Sometimes, intermediate steps are simplified, but the final expression isn't fully reduced. Double-check your final answer.
• Mistaking Non-Perfect Squares for Perfect Squares: Be careful with numbers like 48, which isn't a perfect square but can be broken down as 4 * 12.

By avoiding these mistakes and practicing regularly, you can simplify square roots accurately and efficiently.

Here are a few additional examples to help you understand how to simplify square roots using prime factorization and the identification of perfect squares:

Example 1: Simplify \(\sqrt{72}\)

1. Prime factorize 72: \(72 = 2^3 \times 3^2\)
2. Rewrite inside the square root: \(\sqrt{2^3 \times 3^2}\)
3. Identify perfect squares: \(3^2\) is a perfect square
4. Extract perfect squares: \(\sqrt{3^2} = 3\)
5. Combine: \(3 \times \sqrt{2^3} = 3 \sqrt{2^3}\)
6. Simplify further: \(3 \sqrt{8} = 6 \sqrt{2}\)

Example 2: Simplify \(\sqrt{200}\)

1. Prime factorize 200: \(200 = 2^3 \times 5^2\)
2. Rewrite inside the square root: \(\sqrt{2^3 \times 5^2}\)
3. Identify perfect squares: \(5^2\) is a perfect square
4. Extract perfect squares: \(\sqrt{5^2} = 5\)
5. Combine: \(5 \times \sqrt{2^3} = 5 \sqrt{2^3}\)
6. Simplify further: \(5 \sqrt{8} = 10 \sqrt{2}\)

Example 3: Simplify \(\sqrt{245}\)

1. Prime factorize 245: \(245 = 5 \times 7^2\)
2. Rewrite inside the square root: \(\sqrt{5 \times 7^2}\)
3. Identify perfect squares: \(7^2\) is a perfect square
4. Extract perfect squares: \(\sqrt{7^2} = 7\)
5. Combine: \(7 \times \sqrt{5} = 7 \sqrt{5}\)

Example 4: Simplify \(\sqrt{180}\)

1. Prime factorize 180: \(180 = 2^2 \times 3^2 \times 5\)
2. Rewrite inside the square root: \(\sqrt{2^2 \times 3^2 \times 5}\)
3. Identify perfect squares: \(2^2\) and \(3^2\) are perfect squares
4. Extract perfect squares: \(\sqrt{2^2} = 2\) and \(\sqrt{3^2} = 3\)
5. Combine: \(2 \times 3 \times \sqrt{5} = 6 \sqrt{5}\)

These examples illustrate the method of simplifying square roots by breaking them down into their prime factors and extracting perfect squares to simplify the expression.

Practice simplifying square roots with these problems. Use the steps outlined in this guide to ensure you simplify each square root correctly.

1. Simplify \( \sqrt{72} \)

   1. Prime factorize 72: \( 72 = 2^3 \times 3^2 \)
   2. Identify perfect squares: \( 2^2 \) and \( 3^2 \)
   3. Rewrite as \( \sqrt{(2^2 \times 3^2) \times 2} \)
   4. Extract the perfect squares: \( 2 \times 3 \times \sqrt{2} = 6\sqrt{2} \)

2. Simplify \( \sqrt{180} \)

   1. Prime factorize 180: \( 180 = 2^2 \times 3^2 \times 5 \)
   2. Identify perfect squares: \( 2^2 \) and \( 3^2 \)
   3. Rewrite as \( \sqrt{(2^2 \times 3^2) \times 5} \)
   4. Extract the perfect squares: \( 2 \times 3 \times \sqrt{5} = 6\sqrt{5} \)

3. Simplify \( \sqrt{200} \)

   1. Prime factorize 200: \( 200 = 2^3 \times 5^2 \)
   2. Identify perfect squares: \( 2^2 \) and \( 5^2 \)
   3. Rewrite as \( \sqrt{(2^2 \times 5^2) \times 2} \)
   4. Extract the perfect squares: \( 2 \times 5 \times \sqrt{2} = 10\sqrt{2} \)

4. Simplify \( \sqrt{245} \)

   1. Prime factorize 245: \( 245 = 5 \times 7^2 \)
   2. Identify perfect squares: \( 7^2 \)
   3. Rewrite as \( \sqrt{(7^2) \times 5} \)
   4. Extract the perfect squares: \( 7 \times \sqrt{5} = 7\sqrt{5} \)

These practice problems will help you become proficient in simplifying square roots. Remember to always factorize the number completely and identify perfect squares for simplification.

• What is the square root of 108?

  The square root of 108 is \( \sqrt{108} = \pm 10.392 \).

• How is the square root of 108 simplified?

  The simplified form of the square root of 108 is \( 6\sqrt{3} \). This is obtained by recognizing that \( 108 = 36 \times 3 \), where 36 is a perfect square.

• Is \( \sqrt{108} \) a rational number?

  No, \( \sqrt{108} \) is an irrational number because its decimal form is non-terminating and non-repeating.

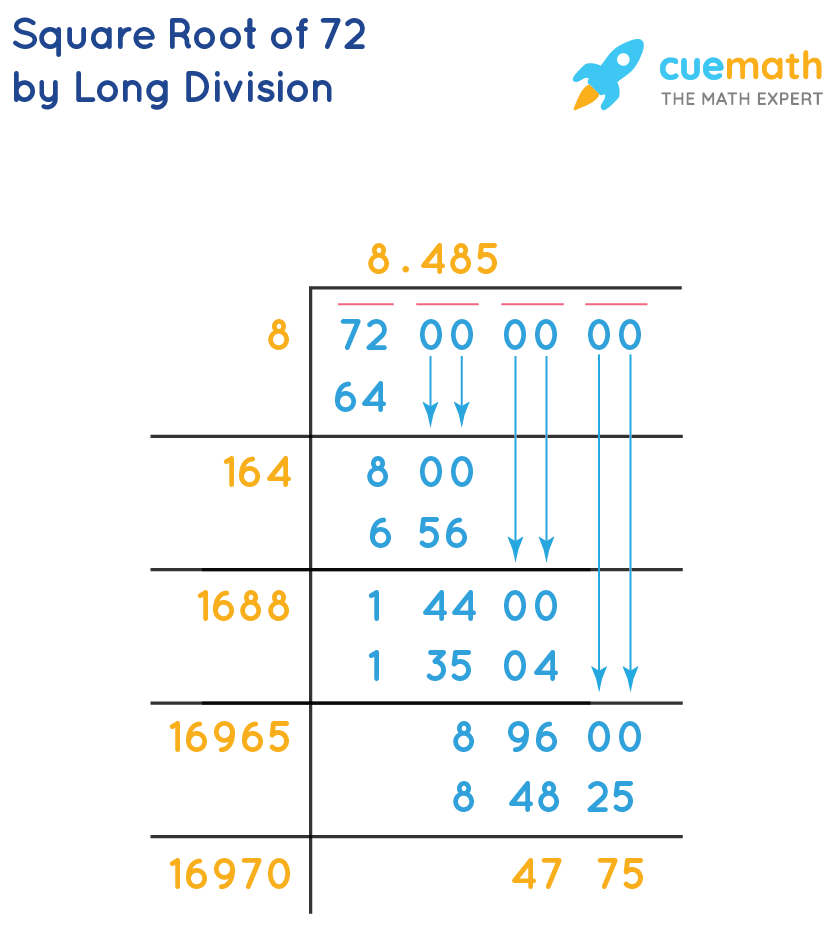
• How do you calculate the square root of 108 using the long division method?

  The long division method involves dividing 108 into pairs of digits and finding the square root step by step, resulting in \( \sqrt{108} \approx 10.392 \) rounded to three decimal places.

• Can the square root of 108 be expressed in exponential form?

  Yes, the square root of 108 can be written in exponential form as \( 108^{\frac{1}{2}} = 6 \times 3^{\frac{1}{2}} \).

Simplifying the square root of 108 can be approached methodically by breaking it down into steps. The process involves prime factorization, identifying perfect squares, and simplifying the expression by extracting these perfect squares.

In summary:

1. Prime factorize 108: \( 108 = 2^2 \times 3^3 \).
2. Identify the perfect squares: \( 4 \) (from \( 2^2 \)) and \( 9 \) (from \( 3^2 \)).
3. Rewrite the square root: \( \sqrt{108} = \sqrt{36 \times 3} = \sqrt{36} \times \sqrt{3} \).
4. Simplify the square root: \( 6\sqrt{3} \).

This method ensures accuracy and clarity in simplifying square roots, and it highlights the importance of understanding the fundamental concepts of prime factorization and perfect squares. By mastering these steps, you can simplify any square root efficiently.