Simplified Square Root of 48: A Comprehensive Guide

To simplify the square root of 48, follow these steps:

Step-by-Step Simplification

1. List the factors of 48: 1, 2, 3, 4, 6, 8, 12, 16, 24, 48
2. Identify the perfect squares from the list: 1, 4, 16
3. Divide 48 by the largest perfect square: \( \frac{48}{16} = 3 \)
4. Calculate the square root of the largest perfect square: \( \sqrt{16} = 4 \)
5. Combine the results to get the simplified form: \( \sqrt{48} = 4\sqrt{3} \)

Mathematical Form

In simplest radical form, the square root of 48 is expressed as:

\[
\sqrt{48} = 4\sqrt{3}
\]

Alternative Representations

In decimal form, the square root of 48 is approximately:

\[
\sqrt{48} \approx 6.928
\]

In exponent form, it is written as:

\[
48^{\frac{1}{2}} = 4 \cdot 3^{\frac{1}{2}}
\]

Conclusion

The square root of 48 can be simplified to \( 4\sqrt{3} \). This is the simplest form and can be used in various mathematical calculations.

Square roots are a fundamental concept in mathematics, particularly in algebra and geometry. They are the inverse operation of squaring a number. For the number 48, the square root represents a value which, when multiplied by itself, equals 48. Understanding how to simplify square roots, such as √48, involves methods like prime factorization, long division, and recognizing perfect squares.

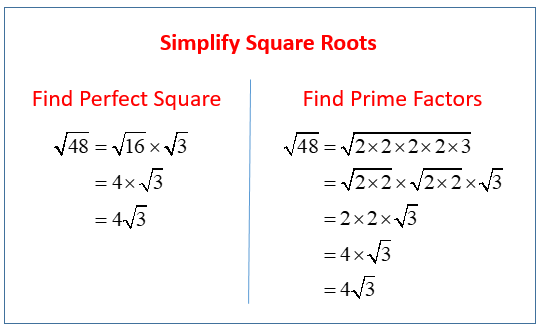
The square root of 48, denoted as √48, can be simplified to 4√3. This simplification is achieved by breaking down 48 into its prime factors: 48 = 16 × 3 = (4 × 4) × 3. Taking the square root of each factor separately gives √(16 × 3) = √16 × √3 = 4√3. Therefore, √48 simplifies to 4√3 in radical form.

To simplify the square root of 48, we follow a structured process involving prime factorization and the identification of perfect squares. Below are the detailed steps:

1. Prime Factorization:

   First, we find the prime factors of 48.

   • 48 can be divided by 2 (the smallest prime number):
   • \( 48 \div 2 = 24 \)
   • 24 can also be divided by 2:
   • \( 24 \div 2 = 12 \)
   • 12 can also be divided by 2:
   • \( 12 \div 2 = 6 \)
   • 6 can also be divided by 2:
   • \( 6 \div 2 = 3 \)
   • Finally, 3 is a prime number.

   Thus, the prime factorization of 48 is:

   \( 48 = 2 \times 2 \times 2 \times 2 \times 3 \) or \( 48 = 2^4 \times 3 \).

2. Group the Prime Factors:

   Next, we group the prime factors into pairs:

   • \( 48 = (2 \times 2) \times (2 \times 2) \times 3 \)
   • Or, we can write it as:
   • \( 48 = (2^2) \times (2^2) \times 3 \)

3. Extract the Square Roots:

   We take the square root of each group of prime factors:

   • \( \sqrt{48} = \sqrt{(2^2) \times (2^2) \times 3} \)
   • \( \sqrt{48} = \sqrt{2^2} \times \sqrt{2^2} \times \sqrt{3} \)
   • \( \sqrt{48} = 2 \times 2 \times \sqrt{3} \)

4. Simplify the Expression:

   Finally, we multiply the simplified factors:

   • \( \sqrt{48} = 4\sqrt{3} \)

   Thus, the simplified form of \( \sqrt{48} \) is \( 4\sqrt{3} \).

The prime factorization method is a straightforward way to simplify the square root of 48. This method involves breaking down the number 48 into its prime factors and then simplifying the radical expression by pairing the factors.

1. First, determine the prime factors of 48. We do this by continuously dividing 48 by the smallest prime numbers:
• 48 ÷ 2 = 24
• 24 ÷ 2 = 12
• 12 ÷ 2 = 6
• 6 ÷ 2 = 3
• 3 ÷ 3 = 1
2. Now, we can write 48 as a product of its prime factors:

\(48 = 2 \times 2 \times 2 \times 2 \times 3\)

3. Group the prime factors into pairs:

\(48 = (2 \times 2) \times (2 \times 2) \times 3\)

4. Take the square root of each pair of prime factors and move them outside the square root sign:

\(\sqrt{48} = \sqrt{(2 \times 2) \times (2 \times 2) \times 3}\)

\(\sqrt{48} = 2 \times 2 \times \sqrt{3}\)

5. Simplify the expression:

\(\sqrt{48} = 4 \sqrt{3}\)

Therefore, the simplified form of the square root of 48 is \(4 \sqrt{3}\).

The long division method is a systematic approach to finding the square root of a number. Here’s a step-by-step guide to finding the square root of 48 using this method:

1. Step 1: Set up the number 48 under the long division symbol.

   	6.	9	2	8	2	0	3	2	7	5	5
   48	√

2. Step 2: Find the largest number whose square is less than or equal to 48. This number is 6 because \(6^2 = 36\).

   Write 6 as the first digit of the square root and subtract 36 from 48 to get the remainder 12.

   6
   48	36
   12

3. Step 3: Bring down a pair of zeros to make the new dividend 1200.

4. Step 4: Double the divisor (6), which gives us 12. Determine how many times 1200 can be divided by 120 to get a digit, which in this case is 9. Write 9 as the next digit of the square root.

   	6.	9
   48	36
   1200

5. Step 5: Subtract 1080 (9 × 120) from 1200 to get the remainder 120. Bring down another pair of zeros to make the new dividend 12000.

6. Step 6: Adjust the divisor by adding the digit used (9), making it 129. Determine how many times 12000 can be divided by 1290, which is 9 again. Write 9 as the next digit.

   	6.	9	2
   48	36
   1200	1080
   12000

7. Step 7: Repeat the process until you reach the desired level of accuracy. The square root of 48 is approximately 6.928.

Identifying perfect squares is a crucial step in simplifying square roots. Perfect squares are numbers that can be expressed as the product of an integer with itself. Let's go through the process step-by-step:

1. List Factors of 48:

   Start by listing all the factors of 48:

   • 1, 2, 3, 4, 6, 8, 12, 16, 24, 48

2. Identify Perfect Squares:

   From the list of factors, identify which are perfect squares. A perfect square is a number that can be expressed as \(n^2\), where \(n\) is an integer:

   • 1 (\(1^2\))
   • 4 (\(2^2\))
   • 16 (\(4^2\))

3. Select the Largest Perfect Square:

   Choose the largest perfect square from the identified factors. In this case, it is 16.

4. Divide and Simplify:

   Divide 48 by the largest perfect square identified:

   \[
   \frac{48}{16} = 3
   \]

   Then, express the square root of 48 using the product of the square root of 16 and the square root of 3:

   \[
   \sqrt{48} = \sqrt{16 \times 3} = \sqrt{16} \times \sqrt{3} = 4\sqrt{3}
   \]

Therefore, the simplified form of \(\sqrt{48}\) is \(4\sqrt{3}\).

To calculate the simplified radical form of the square root of 48, follow these steps:

1. Factorize 48: Start by finding the prime factors of 48. The prime factorization of 48 is:

   \[
   48 = 2 \times 2 \times 2 \times 2 \times 3 = 2^4 \times 3
   \]

2. Group the factors: Group the factors in pairs. Each pair of identical factors can be taken out of the square root:

   \[
   \sqrt{48} = \sqrt{2^4 \times 3} = \sqrt{(2^2)^2 \times 3} = \sqrt{(4)^2 \times 3}
   \]

3. Simplify: Take the square root of the perfect square (which is outside the square root) and leave the remaining factor inside:

   \[
   \sqrt{48} = 4\sqrt{3}
   \]

4. Result: The simplified form of \(\sqrt{48}\) is:

   \[
   \sqrt{48} = 4\sqrt{3}
   \]

By following these steps, you can simplify the square root of any number. For \(\sqrt{48}\), the simplest radical form is \(4\sqrt{3}\).

The square root of 48 can be represented in various forms, each providing a different perspective on the number. Here are some alternative representations:

• Exact Form: The simplified radical form of √48 is \( 4\sqrt{3} \). This is achieved by breaking down 48 into its prime factors and simplifying.
• Decimal Form: By calculating the square root of 48, we get an approximate decimal value of 6.928203230275509, which provides a numerical representation.
• Fractional Exponent Form: Another way to express the square root of 48 is using fractional exponents. This can be written as \( 48^{1/2} \).
• Irrational Form: Since the decimal form of √48 is non-repeating and non-terminating, it is an irrational number. This indicates that it cannot be expressed exactly as a fraction of two integers.
• Product Form: Expressing the square root of 48 as the product of its factors, we get \( 4\sqrt{3} \). Here, 4 is the square root of 16, which is a perfect square factor of 48, and √3 remains under the radical.

These representations illustrate different aspects of the square root of 48, making it easier to understand and utilize in various mathematical contexts.

The square root of 48 can also be represented in its decimal form. This is useful for practical calculations where a more precise value is required.

Here is the step-by-step process to find the decimal form of the square root of 48:

1. First, note that the square root of 48 in its simplest radical form is \(4\sqrt{3}\).
2. To find the decimal value, we approximate the value of \(\sqrt{3}\).
3. The approximate value of \(\sqrt{3}\) is 1.732.
4. Multiply this value by 4 to get the decimal form of the square root of 48.

Therefore, the decimal form of \(\sqrt{48}\) is approximately:

\[
4 \times 1.732 = 6.928
\]

Hence, the square root of 48 in decimal form is \(6.928\).

This value is rounded to three decimal places and provides a practical approximation for various applications.

The square root of 48 can also be expressed in exponent form. This is particularly useful in higher mathematics and certain scientific applications. Here's how we do it:

• Step 1: Understand the Relationship

  Recall that the square root of a number is the same as raising that number to the power of 1/2. Therefore, we can express the square root of 48 as:

  \[
  \sqrt{48} = 48^{1/2}
  \]

• Step 2: Simplify the Exponent

  We have already simplified the square root of 48 to \( 4\sqrt{3} \). We can now express this in exponent form. First, express 4 and \( \sqrt{3} \) in exponent form:

  \[
  4 = 2^2
  \]

  \[
  \sqrt{3} = 3^{1/2}
  \]

  Therefore, \( 4\sqrt{3} \) can be written as:

  \[
  4\sqrt{3} = 2^2 \cdot 3^{1/2}
  \]

• Step 3: Combine Exponents

  When we combine these, we keep the exponents as they are:

  \[
  2^2 \cdot 3^{1/2}
  \]

  Or more generally, we can write:

  \[
  \sqrt{48} = 2^2 \cdot 3^{1/2}
  \]

• Step 4: General Exponent Form

  We can write this in a generalized exponent form as:

  \[
  48^{1/2} = 2^2 \cdot 3^{1/2}
  \]

This form is particularly useful in various mathematical transformations and calculations, allowing for more flexibility and understanding in complex operations.

The simplified form of square roots has numerous practical applications in various fields. Understanding how to simplify square roots can make complex calculations easier and more intuitive. Here are some key applications:

• Geometry: Simplified square roots are frequently used in geometry to find distances, lengths, and areas. For example, the distance between two points in a coordinate plane can be calculated using the Pythagorean theorem, which often involves square roots.
• Physics: In physics, square roots are used to solve problems related to motion, energy, and waves. For instance, the formula for the period of a pendulum involves the square root of the length of the pendulum divided by the acceleration due to gravity.
• Engineering: Engineers use square roots in various calculations, such as determining the stress and strain on materials, electrical circuit analysis, and fluid dynamics.
• Statistics: Simplified square roots are essential in statistics for calculating standard deviations and variances, which measure the dispersion of data points in a dataset.
• Computer Science: Algorithms in computer science, particularly those involving computational geometry and graphics, often use simplified square roots for efficiency.

By mastering the simplification of square roots, you can enhance your problem-solving skills and apply these concepts effectively in both academic and real-world scenarios.

The following are frequently asked questions about the square root of 48:

• What is the simplified form of the square root of 48?

  The simplified form of √48 is 4√3.

• How do you simplify the square root of 48?

  To simplify √48, follow these steps:

  1. Find the prime factorization of 48: 48 = 2 × 2 × 2 × 2 × 3.
  2. Group the prime factors into pairs: (2 × 2) × (2 × 2) × 3.
  3. Take the square root of each pair: √(2 × 2) = 2 and √(2 × 2) = 2.
  4. Multiply the results outside the radical: 2 × 2 = 4.
  5. Combine the result with the remaining factor inside the radical: 4√3.
• Is the square root of 48 a rational number?

  No, the square root of 48 is an irrational number because it cannot be expressed as a simple fraction.

• What is the decimal form of the square root of 48?

  The approximate decimal form of √48 is 6.928.

• How do you represent the square root of 48 in exponent form?

  The square root of 48 can be represented in exponent form as 48^1/2.

• Can the square root of 48 be simplified further?

  No, 4√3 is the simplest radical form of √48.

• What are some applications of the square root of 48?

  The square root of 48 can be used in various mathematical and scientific calculations, including geometry, physics, and engineering, where simplification of square roots is needed for problem-solving and analysis.

• What are common mistakes to avoid when simplifying the square root of 48?

  Common mistakes include:

  • Incorrectly identifying the prime factors.
  • Not properly pairing the factors.
  • Failing to multiply the factors outside the radical correctly.

When simplifying square roots, such as √48, it's easy to make errors if you're not careful. Understanding and avoiding these common mistakes can help improve your mathematical skills and ensure accurate results. Here are some of the most frequent pitfalls to watch out for:

• Overlooking Prime Factorization:

  One common mistake is not fully breaking down the number into its prime factors. Ensure you factorize the number completely to simplify the square root accurately.

• Miscounting the Pairs of Prime Factors:

  Another error occurs when pairs of prime factors are miscounted. Each pair allows one factor to move outside the square root, so accurate counting is crucial.

• Ignoring the Remainder:

  When simplifying √48 to 4√3, it's essential not to forget any remaining numbers under the square root. Every prime factor must be accounted for in the simplified expression.

• Incorrect Multiplication:

  After simplifying, some may incorrectly multiply the factors, leading to the wrong answer. Remember, 4√3 means 4 times the square root of 3, not 4 plus √3.

• Rounding Errors:

  When approximating the square root, avoid rounding too early in your calculations. This can lead to significant inaccuracies in the final answer.

• Skipping Steps:

  Rushing through the simplification process can cause mistakes. Take your time to ensure each step is done correctly and methodically.

By being mindful of these common mistakes, you can improve your accuracy and efficiency in simplifying square roots, leading to better mathematical understanding and performance.

Practicing the simplification of square roots can help solidify your understanding and improve your mathematical skills. Below are some practice problems designed to challenge and enhance your ability to simplify square roots, including √48. Follow the steps carefully and check your answers to ensure accuracy.

1. Problem 1: Simplify √75

   Steps:

   1. Find the prime factorization of 75: \( 75 = 3 \times 5 \times 5 \)
   2. Pair the prime factors: \( (5 \times 5) \times 3 \)
   3. Take the square root of each pair: \( \sqrt{5 \times 5} = 5 \)
   4. Combine the result with the remaining factor inside the radical: \( 5\sqrt{3} \)

   Answer: \( 5\sqrt{3} \)

2. Problem 2: Simplify √32

   Steps:

   1. Find the prime factorization of 32: \( 32 = 2 \times 2 \times 2 \times 2 \times 2 \)
   2. Pair the prime factors: \( (2 \times 2) \times (2 \times 2) \times 2 \)
   3. Take the square root of each pair: \( \sqrt{2 \times 2} = 2 \) and \( \sqrt{2 \times 2} = 2 \)
   4. Combine the results with the remaining factor inside the radical: \( 2 \times 2 \sqrt{2} = 4\sqrt{2} \)

   Answer: \( 4\sqrt{2} \)

3. Problem 3: Simplify √50

   Steps:

   1. Find the prime factorization of 50: \( 50 = 2 \times 5 \times 5 \)
   2. Pair the prime factors: \( (5 \times 5) \times 2 \)
   3. Take the square root of each pair: \( \sqrt{5 \times 5} = 5 \)
   4. Combine the result with the remaining factor inside the radical: \( 5\sqrt{2} \)

   Answer: \( 5\sqrt{2} \)

4. Problem 4: Simplify √48

   Steps:

   1. Find the prime factorization of 48: \( 48 = 2 \times 2 \times 2 \times 2 \times 3 \)
   2. Pair the prime factors: \( (2 \times 2) \times (2 \times 2) \times 3 \)
   3. Take the square root of each pair: \( \sqrt{2 \times 2} = 2 \) and \( \sqrt{2 \times 2} = 2 \)
   4. Combine the results with the remaining factor inside the radical: \( 2 \times 2 \sqrt{3} = 4\sqrt{3} \)

   Answer: \( 4\sqrt{3} \)

5. Problem 5: Simplify √72

   Steps:

   1. Find the prime factorization of 72: \( 72 = 2 \times 2 \times 2 \times 3 \times 3 \)
   2. Pair the prime factors: \( (2 \times 2) \times (3 \times 3) \times 2 \)
   3. Take the square root of each pair: \( \sqrt{2 \times 2} = 2 \) and \( \sqrt{3 \times 3} = 3 \)
   4. Combine the results with the remaining factor inside the radical: \( 2 \times 3 \sqrt{2} = 6\sqrt{2} \)

   Answer: \( 6\sqrt{2} \)

Practice these problems to get a better understanding of simplifying square roots. By following the step-by-step method, you will improve your skills and become more confident in handling similar mathematical problems.