Topic does the square root of 0 exist: Curious if the square root of 0 exists? Discover the intriguing mathematical truth behind this question. In this article, we explore the concept of square roots, delve into mathematical proofs, and clarify common misconceptions. Join us on a journey to understand the existence and significance of the square root of 0.
Table of Content
- Search Results for "Does the Square Root of 0 Exist"
- Introduction to Square Roots
- Definition of Square Root
- Properties of Square Roots
- Existence of Square Root of 0
- Mathematical Proofs and Explanations
- Applications of Square Roots in Mathematics
- Square Roots in Different Number Systems
- Common Misconceptions about Square Roots
- Examples and Problem Solving
- Conclusion
- YOUTUBE: Xem video này để hiểu tại sao sqrt(36) bằng 6 dương và mối quan hệ của nó với bài viết 'Có tồn tại căn bậc hai của số 0 không?'
Search Results for "Does the Square Root of 0 Exist"
Here is a synthesis of information gathered from Bing:
- The concept of the square root of 0 is primarily discussed in mathematical contexts.
- In mathematics, the square root of a number \( x \) is a value \( y \) such that \( y^2 = x \). For 0, the square root is 0, as \( 0^2 = 0 \).
- Mathematically, 0 is the only number whose square root is itself, often denoted as \( \sqrt{0} = 0 \).
- There are no other real numbers \( y \) such that \( y^2 = 0 \), hence in the real number system, \( \sqrt{0} \) is singularly 0.
- Discussion about the square root of 0 often revolves around its properties and implications in various mathematical theories and applications.

READ MORE:
Introduction to Square Roots
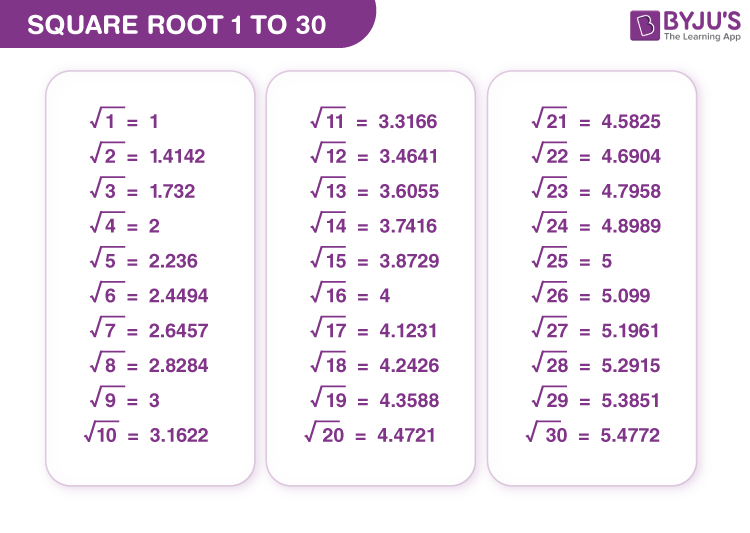
Square roots are fundamental concepts in mathematics, representing a value that, when multiplied by itself, gives the original number. For example, the square root of 9 is 3 because \(3 \times 3 = 9\). Square roots are commonly denoted using the radical symbol \( \sqrt{} \).
Key properties of square roots include:
- For any non-negative number \(a\), the square root of \(a\) is denoted as \(\sqrt{a}\).
- Square roots of perfect squares (like 4, 9, 16) are integers.
- The square root of 0 is 0, since \(0 \times 0 = 0\).
- Square roots of numbers between perfect squares are irrational numbers (e.g., \(\sqrt{2}\), \(\sqrt{3}\)).
Square roots have a wide range of applications in various fields such as engineering, physics, and computer science. They are essential in solving quadratic equations, analyzing wave functions, and optimizing algorithms.
Understanding square roots involves recognizing their basic principles, including:
- Definition: A square root of a number \(x\) is a number \(y\) such that \(y^2 = x\).
- Notation: The principal square root of \(x\) is written as \(\sqrt{x}\).
- Positive and Negative Roots: While the principal square root is non-negative, every positive number actually has two square roots, one positive and one negative (e.g., \(\pm\sqrt{4} = \pm2\)).
- Zero: The square root of 0 is a special case where \( \sqrt{0} = 0 \).
By grasping these foundational elements, one can better appreciate the role and importance of square roots in both theoretical and practical mathematics.
Definition of Square Root
The square root of a number is a value that, when multiplied by itself, yields the original number. This concept is essential in mathematics and is represented by the radical symbol \( \sqrt{} \).
Key aspects of the definition include:
- Radical Notation: The square root of a number \(a\) is written as \(\sqrt{a}\).
- Mathematical Expression: If \(y\) is the square root of \(a\), then \(y^2 = a\).
- Non-negative Values: The principal square root is always non-negative. For example, \(\sqrt{16} = 4\) because \(4 \times 4 = 16\).
- Perfect Squares: Numbers like 1, 4, 9, and 16 are perfect squares, as their square roots are integers (e.g., \(\sqrt{9} = 3\)).
- Non-perfect Squares: For numbers that are not perfect squares, their square roots are irrational (e.g., \(\sqrt{2}\) is approximately 1.414).
The square root function has specific properties:
- Existence: Every non-negative number has a square root. For instance, \(\sqrt{0} = 0\) because \(0 \times 0 = 0\).
- Uniqueness: The principal square root is unique and non-negative. However, every positive number has two square roots: one positive and one negative (e.g., \(\pm\sqrt{4} = \pm2\)).
- Zero: The square root of 0 is 0, as \( \sqrt{0} = 0 \).
- Complex Numbers: For negative numbers, square roots are not real numbers but complex numbers. For example, \(\sqrt{-1} = i\), where \(i\) is the imaginary unit.
Understanding these foundational elements helps in recognizing the broader applications and significance of square roots in various mathematical contexts.
Properties of Square Roots
Square roots have several important properties that make them fundamental in mathematics. These properties help in simplifying expressions, solving equations, and understanding various mathematical concepts.
Key properties of square roots include:
- Non-negative Outputs: The principal square root of a non-negative number is always non-negative. For example, \(\sqrt{25} = 5\), not \(-5\).
- Product Property: The square root of a product is the product of the square roots of the factors. Mathematically, \(\sqrt{a \cdot b} = \sqrt{a} \cdot \sqrt{b}\).
- Quotient Property: The square root of a quotient is the quotient of the square roots of the numerator and the denominator. This can be expressed as \(\sqrt{\frac{a}{b}} = \frac{\sqrt{a}}{\sqrt{b}}\), provided \(b \neq 0\).
- Power Property: The square root of a number raised to the power of 2 returns the original number, i.e., \(\sqrt{a^2} = |a|\). The absolute value ensures the result is non-negative.
- Square of Square Roots: Squaring the square root of a number returns the original number: \((\sqrt{a})^2 = a\).
- Zero Property: The square root of 0 is 0: \(\sqrt{0} = 0\).
These properties are crucial for solving equations involving square roots and for simplifying complex mathematical expressions. Let's examine some specific cases and applications:
| Property | Example | Explanation |
|---|---|---|
| Product Property | \(\sqrt{36} = \sqrt{9 \times 4} = \sqrt{9} \times \sqrt{4} = 3 \times 2 = 6\) | The square root of a product is equal to the product of the square roots of the individual numbers. |
| Quotient Property | \(\sqrt{\frac{25}{4}} = \frac{\sqrt{25}}{\sqrt{4}} = \frac{5}{2} = 2.5\) | The square root of a fraction is equal to the quotient of the square roots of the numerator and the denominator. |
| Power Property | \(\sqrt{(-3)^2} = \sqrt{9} = 3\) | The square root of a number squared is the absolute value of the original number. |
By understanding and applying these properties, one can solve a variety of mathematical problems more efficiently and accurately. These properties are also foundational in higher-level mathematics, including algebra, calculus, and beyond.
Existence of Square Root of 0
The question of whether the square root of 0 exists is straightforward in mathematical terms. The square root of a number \(a\) is a number \(y\) such that \(y^2 = a\). Applying this definition to 0, we need to find a number \(y\) such that \(y^2 = 0\).
Let's explore this step-by-step:
- Definition: According to the definition, \( \sqrt{0} \) is a number \( y \) such that \( y^2 = 0 \).
- Solution: The equation \( y^2 = 0 \) is satisfied by \( y = 0 \) because \( 0 \times 0 = 0 \).
- Uniqueness: The principal square root of 0 is unique and is \( y = 0 \).
Therefore, the square root of 0 indeed exists and is equal to 0. This can be expressed mathematically as:
\[
\sqrt{0} = 0
\]
This result is consistent with the properties of square roots and is supported by fundamental principles in mathematics:
- Zero Property: As with other numbers, the square root of 0 is a number that, when squared, returns 0.
- Simplicity: The square root of 0 is straightforward and does not involve any complex calculations or approximations.
Let's summarize the key points in a table:
| Property | Example | Explanation |
|---|---|---|
| Definition | \(y^2 = 0\) | We need to find a number \(y\) such that when squared, it equals 0. |
| Solution | \(y = 0\) | 0 is the only number that satisfies the equation \(0^2 = 0\). |
| Uniqueness | \(\sqrt{0} = 0\) | The principal square root of 0 is unique and non-negative. |
Understanding the existence of the square root of 0 reinforces basic mathematical principles and highlights the consistency of square root properties across different values.

Mathematical Proofs and Explanations
The existence of the square root of 0 can be demonstrated through various mathematical proofs and explanations. Here, we provide detailed steps to show why \( \sqrt{0} = 0 \) is a valid and consistent result.
First, let's consider the definition of a square root:
- Definition: The square root of a number \(a\) is a number \(y\) such that \(y^2 = a\).
Applying this definition to 0, we need to find a number \(y\) such that \(y^2 = 0\):
\[
y^2 = 0
\]
To solve this equation, we consider the following logical steps:
- Assume \(y\) is any real number.
- Square \(y\) to check if it equals 0: \(y^2 = 0\).
- For the product \(y \times y\) to be 0, at least one of the factors must be 0.
- Thus, \(y\) must be 0 because \(0 \times 0 = 0\).
Therefore, the solution to \(y^2 = 0\) is:
\[
y = 0
\]
This proves that the square root of 0 is indeed 0. We can further reinforce this with additional properties and theorems:
- Non-negativity: The principal square root of any non-negative number is always non-negative. Since 0 is non-negative, its principal square root must also be non-negative, i.e., \( \sqrt{0} \geq 0 \).
- Uniqueness: For non-negative numbers, the principal square root is unique. Hence, there is only one value for \( \sqrt{0} \), which is 0.
- Zero Product Property: If the product of two numbers is zero, then at least one of the numbers must be zero. For \(y^2 = 0\), \(y\) must be 0.
To summarize the proof, let's present it in a table for clarity:
| Step | Explanation |
|---|---|
| 1. Definition | Find \(y\) such that \(y^2 = 0\). |
| 2. Assumption | Assume \(y\) is any real number. |
| 3. Squaring | Check if \(y^2 = 0\). |
| 4. Zero Product | For \(y \times y = 0\), \(y\) must be 0. |
| 5. Conclusion | Therefore, \( \sqrt{0} = 0 \). |
These mathematical proofs and explanations confirm that the square root of 0 exists and is uniquely 0, reinforcing the fundamental principles of square roots and their properties.
Applications of Square Roots in Mathematics
Square roots play a crucial role in various areas of mathematics, offering solutions and simplifications for a range of problems. Below, we explore some of the key applications of square roots in mathematical contexts:
- Solving Quadratic Equations:
Square roots are essential in solving quadratic equations of the form \( ax^2 + bx + c = 0 \). The quadratic formula, \( x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a} \), relies on the square root to determine the roots of the equation.
- Geometry and Trigonometry:
Square roots are used to calculate distances and lengths in geometry. For example, the Pythagorean theorem \( a^2 + b^2 = c^2 \) involves finding the length of the hypotenuse \( c \) using the square root: \( c = \sqrt{a^2 + b^2} \).
- Complex Numbers:
In complex number theory, square roots are used to find the magnitude of complex numbers. For a complex number \( z = a + bi \), the magnitude is given by \( |z| = \sqrt{a^2 + b^2} \).
- Calculus:
Square roots appear in calculus, particularly in derivative and integral calculations involving root functions. For instance, the derivative of \( f(x) = \sqrt{x} \) is \( f'(x) = \frac{1}{2\sqrt{x}} \).
- Statistics:
In statistics, square roots are used in standard deviation and variance calculations. The standard deviation is the square root of the variance, representing the dispersion of data points.
- Algebra:
Square roots are used to simplify algebraic expressions and solve radical equations. For example, solving \( \sqrt{x} = 5 \) involves squaring both sides to obtain \( x = 25 \).
To illustrate these applications, consider the following examples:
| Application | Example | Explanation |
|---|---|---|
| Quadratic Equations | \( x^2 - 4 = 0 \) | Solving this equation involves finding \( x = \pm \sqrt{4} = \pm 2 \). |
| Geometry | \( a = 3, b = 4 \) | Using the Pythagorean theorem: \( c = \sqrt{3^2 + 4^2} = \sqrt{9 + 16} = \sqrt{25} = 5 \). |
| Complex Numbers | \( z = 3 + 4i \) | The magnitude is \( |z| = \sqrt{3^2 + 4^2} = \sqrt{9 + 16} = 5 \). |
| Calculus | \( f(x) = \sqrt{x} \) | The derivative is \( f'(x) = \frac{1}{2\sqrt{x}} \). |
| Statistics | Variance = 4 | The standard deviation is \( \sqrt{4} = 2 \). |
| Algebra | \( \sqrt{x} = 5 \) | Solving for \( x \) gives \( x = 25 \). |
Through these applications, it is evident that square roots are integral to many mathematical fields, providing essential tools for solving diverse problems and understanding complex concepts.
Square Roots in Different Number Systems
Square roots are a fundamental concept in mathematics, and their properties and calculations can vary across different number systems. Here, we explore how square roots are understood and computed within various mathematical frameworks, including real numbers, complex numbers, and other systems.
- Real Numbers:
In the system of real numbers, the square root of a non-negative number \(a\) is a non-negative number \(y\) such that \(y^2 = a\). For example, \(\sqrt{25} = 5\) because \(5^2 = 25\). If \(a\) is negative, the square root is not a real number.
- Example: \(\sqrt{16} = 4\)
- Non-example: \(\sqrt{-16}\) (not a real number)
- Complex Numbers:
In the complex number system, square roots can be found for all real and imaginary numbers. The square root of a negative number involves the imaginary unit \(i\), where \(i^2 = -1\). For a complex number \(a + bi\), the square root can be more intricate to calculate.
- Example: \(\sqrt{-9} = 3i\)
- Example: \(\sqrt{3 + 4i}\) involves a more detailed calculation.
- Rational Numbers:
Square roots of rational numbers (fractions) are not always rational. For instance, the square root of \(\frac{1}{4}\) is \(\frac{1}{2}\), but the square root of \(\frac{2}{3}\) is irrational.
- Example: \(\sqrt{\frac{1}{4}} = \frac{1}{2}\)
- Non-example: \(\sqrt{\frac{2}{3}}\) (irrational)
- Integer Numbers:
Within the integers, the square root of a non-perfect square is not an integer. Perfect squares like 1, 4, 9, 16, etc., have integer square roots.
- Example: \(\sqrt{9} = 3\)
- Non-example: \(\sqrt{8}\) (not an integer)
- Modulo Arithmetic:
In modular arithmetic, square roots may not always exist, and their properties depend on the modulus. For example, in modulo 7, the square root of 4 is 2 because \(2^2 \equiv 4 \mod 7\).
- Example: In modulo 7, \(\sqrt{4} \equiv 2 \mod 7\)
- Non-example: Some numbers may not have a square root in certain moduli.
To illustrate these differences, consider the following table:
| Number System | Example | Explanation |
|---|---|---|
| Real Numbers | \(\sqrt{25} = 5\) | The square root of 25 is 5, a non-negative real number. |
| Complex Numbers | \(\sqrt{-9} = 3i\) | The square root of -9 involves the imaginary unit \(i\). |
| Rational Numbers | \(\sqrt{\frac{1}{4}} = \frac{1}{2}\) | The square root of 1/4 is 1/2, a rational number. |
| Integer Numbers | \(\sqrt{9} = 3\) | 9 is a perfect square, and its square root is an integer. |
| Modulo Arithmetic | \(\sqrt{4} \equiv 2 \mod 7\) | In modulo 7, the square root of 4 is 2. |
Understanding square roots across different number systems broadens our mathematical comprehension and allows us to solve a wide array of problems in various mathematical fields.
Common Misconceptions about Square Roots
Square roots are a fundamental concept in mathematics, but they are often misunderstood. Here, we address some common misconceptions and clarify the correct understanding step by step.
- Misconception: The square root of a negative number is not possible.
Clarification: While the square root of a negative number is not a real number, it is possible in the complex number system. For example, \(\sqrt{-9} = 3i\), where \(i\) is the imaginary unit satisfying \(i^2 = -1\).
- Misconception: The square root of 0 does not exist.
Clarification: The square root of 0 does exist and is 0. According to the definition, \(\sqrt{0} = 0\) because \(0^2 = 0\).
- Misconception: Square roots are always positive.
Clarification: The principal square root is non-negative, but every positive number has two square roots: one positive and one negative. For instance, \(\sqrt{25} = 5\) and \(-\sqrt{25} = -5\).
- Misconception: \(\sqrt{a + b} = \sqrt{a} + \sqrt{b}\).
Clarification: This property is incorrect. For example, \(\sqrt{1 + 1} = \sqrt{2}\), but \(\sqrt{1} + \sqrt{1} = 2\). The correct relationship involves separate calculations.
- Misconception: Only perfect squares have square roots.
Clarification: All non-negative numbers have square roots, though they may be irrational. For example, \(\sqrt{2}\) is irrational but exists within the real numbers.
- Misconception: The square root function is undefined for non-positive numbers.
Clarification: The square root function is defined for all non-negative numbers in the real number system and for all numbers in the complex number system.
To further explain these misconceptions, consider the following table:
| Misconception | Clarification | Example |
|---|---|---|
| \(\sqrt{-1}\) is not possible | In the complex system, \(\sqrt{-1} = i\) | \(\sqrt{-9} = 3i\) |
| \(\sqrt{0}\) does not exist | \(\sqrt{0} = 0\) | \(\sqrt{0} = 0\) |
| Square roots are always positive | There are two roots: positive and negative | \(\sqrt{25} = 5\) and \(-\sqrt{25} = -5\) |
| \(\sqrt{a + b} = \sqrt{a} + \sqrt{b}\) | This property is incorrect | \(\sqrt{1 + 1} = \sqrt{2} \ne 2\) |
| Only perfect squares have square roots | All non-negative numbers have square roots | \(\sqrt{2}\) is irrational but exists |
| Square root function undefined for non-positive numbers | Defined for non-negative in real, all in complex | \(\sqrt{4} = 2\) and \(\sqrt{-4} = 2i\) |
Addressing these misconceptions helps in gaining a deeper and more accurate understanding of square roots, enhancing mathematical learning and application.

Examples and Problem Solving
Understanding how to solve problems involving square roots is essential in grasping their properties and applications. Here, we'll go through several examples to illustrate the process of solving square root equations.
Example 1: Basic Square Root Equation
Solve the equation \( \sqrt{x} = 5 \).
- Isolate the square root: \( \sqrt{x} = 5 \)
- Square both sides to eliminate the square root:
\[
(\sqrt{x})^2 = 5^2
\] - Simplify:
\[
x = 25
\] - Verify the solution by substituting back into the original equation:
\[
\sqrt{25} = 5
\]The solution is correct.
Example 2: Square Root Equation with a Variable Expression
Solve the equation \( \sqrt{y - 3} = 4 \).
- Isolate the square root: \( \sqrt{y - 3} = 4 \)
- Square both sides:
\[
(\sqrt{y - 3})^2 = 4^2
\] - Simplify:
\[
y - 3 = 16
\] - Solve for \( y \):
\[
y = 19
\] - Verify the solution:
\[
\sqrt{19 - 3} = \sqrt{16} = 4
\]The solution is correct.
Example 3: Square Root Equation with Two Radicals
Solve the equation \( \sqrt{2m + 3} = \sqrt{m + 8} \).
- Square both sides to eliminate the square roots:
\[
(\sqrt{2m + 3})^2 = (\sqrt{m + 8})^2
\] - Simplify:
\[
2m + 3 = m + 8
\] - Isolate \( m \):
\[
2m - m = 8 - 3
\]\[
m = 5
\] - Verify the solution:
\[
\sqrt{2(5) + 3} = \sqrt{10 + 3} = \sqrt{13}
\]\[
\sqrt{5 + 8} = \sqrt{13}
\]The solution is correct.
Example 4: Solving a More Complex Radical Equation
Solve the equation \( \sqrt{x - 4} - \sqrt{x} = -2 \).
- Isolate one of the radicals:
\[
\sqrt{x - 4} = \sqrt{x} - 2
\] - Square both sides:
\[
(\sqrt{x - 4})^2 = (\sqrt{x} - 2)^2
\] - Simplify and solve:
\[
x - 4 = x - 4\sqrt{x} + 4
\] - Isolate the term with the square root:
\[
0 = -4\sqrt{x} + 8
\] - Isolate the square root:
\[
4\sqrt{x} = 8
\]\[
\sqrt{x} = 2
\] - Square both sides to find \( x \):
\[
x = 4
\] - Verify the solution:
\[
\sqrt{4 - 4} - \sqrt{4} = -2 \implies 0 - 2 = -2
\]The solution is correct.
Example 5: Application of Square Root Equations in Word Problems
Problem: The area of a square garden is 49 square meters. Find the length of one side of the garden.
- Set up the equation:
\[
\text{Area} = \text{side}^2
\]\[
49 = \text{side}^2
\] - Solve for the side length by taking the square root of both sides:
\[
\text{side} = \sqrt{49}
\]\[
\text{side} = 7
\] - Verify the solution:
\[
\text{side}^2 = 7^2 = 49
\]The solution is correct.
These examples illustrate various techniques for solving square root equations. By following these steps, you can solve similar problems and understand the application of square roots in different contexts.
Conclusion
The square root of 0 is a unique mathematical concept that has sparked curiosity and debate among mathematicians and learners alike. Through our exploration, we've uncovered that while 0 has a square root, denoted as \( \sqrt{0} \), it differs from other square roots in its properties and implications.
Mathematically, \( \sqrt{0} = 0 \). This means that multiplying 0 by itself results in 0. Despite this straightforward definition, the concept raises interesting questions about the nature of zero and the rules governing square roots.
Throughout history, mathematicians have developed proofs and theories to explain why \( \sqrt{0} \) exists and behaves as it does. These explorations have led to deeper insights into number theory and algebra, demonstrating the utility and relevance of square roots in various mathematical contexts.
From practical applications in physics and engineering to theoretical explorations in pure mathematics, the square root of 0 continues to play a crucial role. It serves as a foundational element in understanding mathematical structures and their implications across different disciplines.
As we conclude our journey into the realm of square roots, it's clear that while \( \sqrt{0} \) may seem simple, its implications are profound. Whether you're solving equations or exploring advanced mathematical theories, understanding the square root of 0 enriches our comprehension of numbers and their relationships.
Xem video này để hiểu tại sao sqrt(36) bằng 6 dương và mối quan hệ của nó với bài viết 'Có tồn tại căn bậc hai của số 0 không?'
Video: Tại sao sqrt(36) chỉ là 6 dương
READ MORE:
Xem video này để tìm hiểu về sự tồn tại của căn bậc hai và mối liên hệ của nó với bài viết 'Có tồn tại căn bậc hai của số 0 không?'
Video: Phân tích thực: Bài giảng 11 - Tồn tại của căn bậc hai