8 Times Square Root of 3: Understanding This Mathematical Marvel

The expression 8 \times \sqrt{3} involves the multiplication of the number 8 by the square root of 3. Below, we detail various aspects and applications of this mathematical operation.

Expression and Calculation

The square root of 3 is an irrational number approximately equal to 1.732. Therefore, multiplying this value by 8 gives:

\[
8 \times \sqrt{3} \approx 8 \times 1.732 = 13.856
\]

Properties and Characteristics

• Irrationality: Since \(\sqrt{3}\) is irrational, the product \(8 \times \sqrt{3}\) is also irrational.
• Decimal Representation: The value of \(8 \times \sqrt{3}\) is approximately 13.856, but its decimal representation is non-terminating and non-repeating.
• Symbolic Form: It is often represented in its exact form \(8 \times \sqrt{3}\) in mathematical expressions to maintain precision.

Applications

The product \(8 \times \sqrt{3}\) can appear in various contexts, including geometry, trigonometry, and physics. Here are a few examples:

1. Geometry: Involving lengths of sides in special right triangles or the height of equilateral triangles.
2. Trigonometry: Related to certain trigonometric identities and expressions.
3. Physics: Appearing in calculations involving forces, distances, or other quantities where the square root of 3 is a factor.

Conclusion

The expression 8 \times \sqrt{3} represents a common mathematical operation that yields an irrational number with various applications in different fields. Understanding and using this expression effectively requires familiarity with the properties of irrational numbers and their implications in real-world problems.

The expression \( 8 \sqrt{3} \) represents a fascinating mathematical concept that arises in various contexts. By multiplying the square root of 3 by 8, we get a number with unique properties and significant applications. This guide aims to delve into the importance and utility of \( 8 \sqrt{3} \), providing a thorough understanding of its role in different mathematical and physical scenarios.

To appreciate the significance of \( 8 \sqrt{3} \), it is essential to understand the basics of square roots and their multiplication:

• Square Root Definition: The square root of a number is a value that, when multiplied by itself, gives the original number. For example, the square root of 3 (\( \sqrt{3} \)) is approximately 1.732.
• Multiplication of Square Roots: Multiplying a square root by an integer scales the value while retaining its root properties. In this case, multiplying \( \sqrt{3} \) by 8 results in \( 8 \sqrt{3} \).

Here is the step-by-step calculation for \( 8 \sqrt{3} \):

1. Calculate the square root of 3: \( \sqrt{3} \approx 1.732 \).
2. Multiply this value by 8: \( 8 \times 1.732 \approx 13.856 \).

Thus, \( 8 \sqrt{3} \approx 13.856 \).

In this guide, we will explore the mathematical definition, properties, symbolic representation, and various applications of \( 8 \sqrt{3} \) across different fields such as geometry, trigonometry, and physics. Let's embark on this mathematical journey to uncover the wonders of \( 8 \sqrt{3} \).

The expression \( 8 \sqrt{3} \) combines the concepts of multiplication and square roots in mathematics. To understand its significance, we need to break down its components and their mathematical properties.

Square Root: The square root of a number \( x \), denoted as \( \sqrt{x} \), is a value that, when multiplied by itself, yields \( x \). Mathematically, this can be written as:

\[ \sqrt{x} \times \sqrt{x} = x \]

In the case of 3:

\[ \sqrt{3} \approx 1.732 \]

Multiplication: Multiplying a number by an integer scales it linearly. Here, we multiply the square root of 3 by 8:

\[ 8 \times \sqrt{3} \]

Substituting the approximate value of \( \sqrt{3} \):

\[ 8 \times 1.732 \approx 13.856 \]

Thus, \( 8 \sqrt{3} \approx 13.856 \).

Properties of \( 8 \sqrt{3} \):

• Irrational Number: The square root of 3 is an irrational number, meaning it cannot be expressed as a simple fraction. Consequently, \( 8 \sqrt{3} \) is also irrational.
• Exact and Approximate Values: While the exact value of \( 8 \sqrt{3} \) is \( 8 \times \sqrt{3} \), its approximate value is often used in practical applications.
• Scalability: The expression can be scaled further by multiplying with other numbers, preserving its root properties.

To summarize, \( 8 \sqrt{3} \) is a product of the square root of 3 and the integer 8, resulting in an irrational number approximately equal to 13.856. Understanding this mathematical expression requires a grasp of square roots, multiplication, and their combined properties.

Calculating \( 8 \sqrt{3} \) involves understanding both the exact mathematical expression and its numerical approximation. Here, we will explore the detailed steps to calculate and approximate this value.

Step-by-Step Calculation:

1. Identify the square root component:

   The square root of 3 is represented as \( \sqrt{3} \). This value is irrational, meaning it cannot be precisely expressed as a fraction. However, it can be approximated.

2. Approximate the square root of 3:

   The approximate value of \( \sqrt{3} \) is 1.732. This approximation is typically used in calculations where an exact value is not required.

3. Multiply by 8:

   Now, multiply the approximate value of \( \sqrt{3} \) by 8 to find \( 8 \sqrt{3} \).

   \[ 8 \times 1.732 = 13.856 \]

Using a Calculator:

For more precision, a scientific calculator can be used to find the value of \( 8 \sqrt{3} \). Simply enter the expression directly into the calculator to obtain a more accurate result:

• Enter 3 and press the square root (√) button to get \( \sqrt{3} \).
• Multiply the result by 8.

Mathematical Representation:

The exact value of \( 8 \sqrt{3} \) remains \( 8 \times \sqrt{3} \), an irrational number. For practical purposes, the approximate value is often used:

\[ 8 \sqrt{3} \approx 13.856 \]

Tabular Comparison:

Understanding both the exact and approximate values of \( 8 \sqrt{3} \) is essential for various mathematical applications. Whether using a calculator for precision or approximating for simplicity, recognizing the nature of this expression helps in accurately solving problems and appreciating its mathematical beauty.

The symbolic representation of mathematical expressions like \( 8 \sqrt{3} \) plays a crucial role in simplifying and understanding complex calculations. This section will break down the components and notations used in representing \( 8 \sqrt{3} \).

Components of the Expression:

• 8: The integer multiplier, which scales the value of the square root of 3.
• \( \sqrt{3} \): The square root of 3, an irrational number approximately equal to 1.732.

Combined Expression:

The multiplication of these components is represented as:

\[ 8 \sqrt{3} \]

Mathematical Notation:

In mathematical notation, \( 8 \sqrt{3} \) is expressed as:

\[ 8 \times \sqrt{3} \]

Where:

• \( 8 \) is the multiplier.
• \( \sqrt{3} \) denotes the square root of 3.

LaTeX Representation:

In LaTeX, a typesetting system commonly used for mathematical and scientific documents, the expression is written as:

\[ 8 \sqrt{3} \]

The LaTeX code for this expression is:

8 \sqrt{3}

Symbolic Manipulations:

This expression can be manipulated symbolically in various ways, including addition, subtraction, multiplication, and division:

• Addition: \( 8 \sqrt{3} + 4 \sqrt{3} = 12 \sqrt{3} \)
• Subtraction: \( 8 \sqrt{3} - 2 \sqrt{3} = 6 \sqrt{3} \)
• Multiplication: \( 8 \sqrt{3} \times 2 = 16 \sqrt{3} \)
• Division: \( \frac{8 \sqrt{3}}{4} = 2 \sqrt{3} \)

Geometric Interpretation:

In geometric contexts, \( 8 \sqrt{3} \) can represent lengths and distances, such as the height of an equilateral triangle with a side length of 8. This geometric interpretation often aids in visualizing and solving problems.

In conclusion, the symbolic representation of \( 8 \sqrt{3} \) involves understanding its components, mathematical notation, and the ways it can be manipulated and interpreted. This foundational knowledge is essential for leveraging \( 8 \sqrt{3} \) in various mathematical and practical applications.

The expression \( 8 \sqrt{3} \) has numerous applications in mathematics, making it a valuable tool in various fields. Here, we explore some of the most significant applications, detailing how this expression is utilized in different mathematical contexts.

1. Geometry:

• Equilateral Triangles: In an equilateral triangle with side length 8, the height can be calculated using \( 8 \sqrt{3} \). The formula for the height (h) of an equilateral triangle is:

  \[ h = \frac{\sqrt{3}}{2} \times \text{side length} \]

  For a side length of 8:

  \[ h = \frac{\sqrt{3}}{2} \times 8 = 4 \sqrt{3} \]

  Doubling this height results in \( 8 \sqrt{3} \).

• Hexagons: The distance between opposite vertices in a regular hexagon with a side length of 4 can be expressed as \( 8 \sqrt{3} \), highlighting its geometric significance.

2. Trigonometry:

• Trigonometric Ratios: In trigonometry, \( 8 \sqrt{3} \) can appear in various problems involving 30-60-90 triangles, where the sides are in the ratio 1 : \( \sqrt{3} \) : 2. For example, if one side of the triangle is 4, then the longer leg would be \( 4 \sqrt{3} \), and doubling it gives \( 8 \sqrt{3} \).
• Sine and Cosine Values: Understanding the properties of \( 8 \sqrt{3} \) can help in solving problems involving sine and cosine values for angles that are multiples of 30 degrees or \( \pi/6 \) radians.

3. Algebra:

• Simplifying Expressions: Algebraic expressions often require the simplification of terms involving square roots. Knowing how to handle \( 8 \sqrt{3} \) helps in simplifying and solving such expressions efficiently.
• Equations: Solving equations involving square roots, such as quadratic equations, can benefit from understanding the manipulation and properties of \( 8 \sqrt{3} \).

4. Calculus:

• Integration and Differentiation: In calculus, expressions like \( 8 \sqrt{3} \) appear in integrals and derivatives, especially when dealing with functions involving square roots. For example, integrating or differentiating functions like \( f(x) = 8 \sqrt{3} x \) requires familiarity with such expressions.
• Limits: Limits involving irrational numbers, such as \( 8 \sqrt{3} \), often arise in calculus problems, and understanding their properties is crucial for solving these problems accurately.

5. Number Theory:

• Irrational Numbers: The study of irrational numbers in number theory includes exploring expressions like \( 8 \sqrt{3} \), understanding their properties, and proving their irrationality.
• Diophantine Equations: In number theory, solving Diophantine equations, which involve integer solutions, sometimes requires understanding and manipulating expressions involving square roots.

In summary, \( 8 \sqrt{3} \) is a versatile expression with applications in geometry, trigonometry, algebra, calculus, and number theory. Its unique properties and characteristics make it an essential tool for solving a wide range of mathematical problems.

The expression \( 8 \sqrt{3} \) finds significant utility in geometric contexts, particularly involving special triangles and polygon properties.

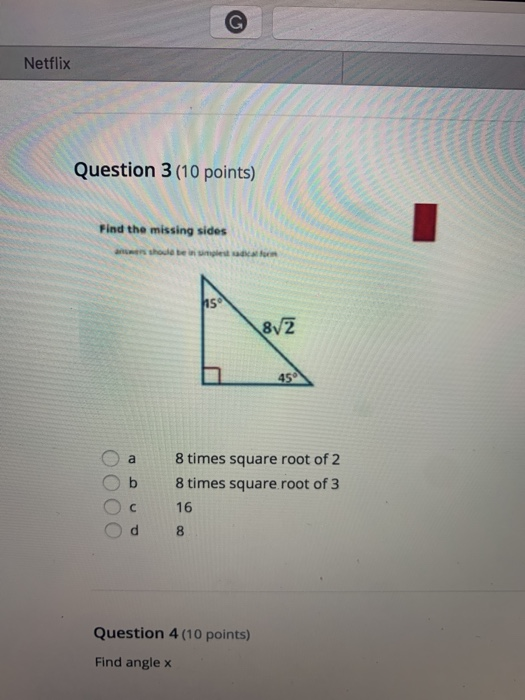
One common geometric application is in 30-60-90 triangles. The side lengths of these triangles are in the ratio \( 1 : \sqrt{3} : 2 \). This means:

• The length of the side opposite the 30° angle is \( x \).
• The length of the side opposite the 60° angle is \( x \sqrt{3} \).
• The hypotenuse is \( 2x \).

If we set \( x = 8 \), then:

• The side opposite the 30° angle is \( 8 \).
• The side opposite the 60° angle is \( 8 \sqrt{3} \).
• The hypotenuse is \( 16 \).

This geometric configuration is useful in various problems involving right triangles, trigonometric ratios, and properties of polygons.

Additionally, the value \( 8 \sqrt{3} \) appears in the context of hexagons. A regular hexagon can be divided into six equilateral triangles. If each side of the hexagon is \( 8 \), the height of each triangle (which is \( 8 \sqrt{3} \)) helps calculate the area of the hexagon and its related properties.

In summary, understanding the geometric implications of \( 8 \sqrt{3} \) enhances the ability to solve complex geometric problems involving special triangles and regular polygons.

The expression \(8 \sqrt{3}\) has various applications in trigonometry, particularly in solving problems involving angles and lengths in right triangles and in the unit circle. Here, we explore some key applications:

• Right Triangle Problems:

  In right triangles, the expression \(8 \sqrt{3}\) can represent a leg or the hypotenuse. Consider a right triangle where one leg is \(8 \sqrt{3}\) units long:

  • Finding the other leg: If one leg is \(8 \sqrt{3}\) and the hypotenuse is \(16\), you can use the Pythagorean theorem:

    \[
    \text{Let } a = 8 \sqrt{3}, \text{ and } c = 16. \text{ Find } b.
    \]
    \[
    a^2 + b^2 = c^2 \implies (8 \sqrt{3})^2 + b^2 = 16^2 \implies 192 + b^2 = 256 \implies b^2 = 64 \implies b = 8.
    \]

  • Finding angles using trigonometric ratios: With one leg and the hypotenuse known, the sine and cosine of the angles can be calculated. For example, for angle \(\theta\) opposite to the leg \(8 \sqrt{3}\):
    \[
    \sin \theta = \frac{8 \sqrt{3}}{16} = \frac{\sqrt{3}}{2}, \quad \cos \theta = \frac{8}{16} = \frac{1}{2}.
    \]
    Thus, \(\theta = \frac{\pi}{3}\) or 60 degrees.

• Unit Circle:

  In the unit circle, the coordinates of points corresponding to certain angles can involve multiples of \(\sqrt{3}\). For example, the point at \(60^\circ\) or \(\frac{\pi}{3}\) radians has coordinates \((\frac{1}{2}, \frac{\sqrt{3}}{2})\). Scaling these coordinates by 16 results in:
  \[
  (8, 8\sqrt{3})
  \]
  This scaling is useful in problems involving circles and ellipses.

• Law of Sines:

  The Law of Sines relates the sides of a triangle to its angles:
  \[
  \frac{a}{\sin A} = \frac{b}{\sin B} = \frac{c}{\sin C}.
  \]
  For a triangle with sides \(a = 8 \sqrt{3}\), \(b = 8\), and an angle \(A = 60^\circ\), the other angles and sides can be found using this law.

Understanding these applications helps in solving a wide range of trigonometric problems, demonstrating the versatility of the expression \(8 \sqrt{3}\) in mathematical contexts.

The expression \(8 \sqrt{3}\) finds its relevance in various physical contexts. Here, we explore some significant applications:

• Wave Mechanics:

  In wave mechanics, the amplitude of waves can sometimes be expressed in terms of \(8 \sqrt{3}\). For instance, in certain interference patterns or resonance phenomena, the amplitude relationship might involve this expression.

• Electrical Engineering:

  The root mean square (RMS) value of an alternating current (AC) waveform can be related to expressions involving square roots. If the peak voltage is \(8 \sqrt{3}\), the RMS voltage \(V_{RMS}\) is calculated as:

  \[
  V_{RMS} = \frac{V_{peak}}{\sqrt{2}} = \frac{8 \sqrt{3}}{\sqrt{2}} = 4 \sqrt{6} \approx 9.798
  \]

• Optics:

  In optics, particularly in the study of light paths through different media, expressions involving \(8 \sqrt{3}\) can emerge when calculating critical angles and refractive indices. For example, when dealing with a prism where the path of light must be calculated using trigonometric functions involving square roots.

• Structural Engineering:

  When analyzing stress and strain in materials, certain force or displacement vectors might be expressed as \(8 \sqrt{3}\) units, especially in composite materials where directional forces are resolved into components.

These examples highlight the versatility and importance of \(8 \sqrt{3}\) in practical physical applications, demonstrating its utility in simplifying complex calculations and enhancing our understanding of various physical phenomena.

Understanding the expression \(8 \sqrt{3}\) is crucial, but several common misconceptions can lead to confusion. Here are some of the most frequent misunderstandings:

• Misinterpreting the Operation:

  Some may mistakenly interpret \(8 \sqrt{3}\) as \(8 \cdot 3\), which results in \(24\). The correct interpretation is \(8\) times the square root of \(3\), which is approximately \(8 \times 1.732 = 13.856\).

• Distribution Error:

  Another common mistake is the incorrect distribution of the square root over addition. For example, incorrectly simplifying \( \sqrt{a + b}\) as \( \sqrt{a} + \sqrt{b}\). This error can also manifest in more complex expressions involving \( \sqrt{3}\).

• Incorrect Simplification:

  Some learners incorrectly simplify \(8 \sqrt{3}\) by trying to combine the terms under the square root. The correct simplification keeps the number outside, as in \(8 \sqrt{3}\).

• Confusion with Units:

  When applying \(8 \sqrt{3}\) in physical contexts, such as physics problems involving distance or force, it is essential to maintain the proper units throughout the calculation. Mismanagement of units can lead to significant errors.

• Misapplication in Formulas:

  In trigonometry and geometry, specific values are required in formulas. Misapplying \(8 \sqrt{3}\) instead of another term can yield incorrect results, especially in formulas for areas and volumes where precise values are critical.

Recognizing and addressing these misconceptions is vital for correct mathematical understanding and application.

Visual representations play a crucial role in understanding mathematical concepts, including the expression \(8\sqrt{3}\). These representations can help students and educators visualize complex numbers and their relationships, facilitating deeper comprehension. Here are some common visual methods used to represent \(8\sqrt{3}\) and its applications:

• Number Line: Representing \(8\sqrt{3}\) on a number line helps in understanding its magnitude and position relative to other numbers. It is approximately 13.856, so it would be placed between 13 and 14.
• Geometric Shapes: Using triangles or rectangles can illustrate the properties of \(8\sqrt{3}\). For instance, in an equilateral triangle with side length 8, the height can be shown as \(8\sqrt{3}\).
• Graphs: Plotting \(8\sqrt{3}\) on a graph can help visualize its value in relation to other functions and numbers. This can be particularly useful in trigonometric and geometric applications where visualizing the height or distance is necessary.

Below are detailed steps and visual aids for representing \(8\sqrt{3}\) geometrically:

1. Number Line Representation

To represent \(8\sqrt{3}\) on a number line:

1. Draw a horizontal line and mark equal intervals representing integers.
2. Identify the approximate position of 13.856 on the number line, placing it between 13 and 14.

2. Geometric Shape Representation

Using an equilateral triangle:

1. Draw an equilateral triangle with each side of length 8 units.
2. Draw a perpendicular line from one vertex to the midpoint of the opposite side, creating two 30-60-90 triangles.
3. The height of these triangles is \(8\sqrt{3}\).

3. Graphical Representation

To plot \(8\sqrt{3}\) on a Cartesian plane:

1. Set up the Cartesian coordinate system with appropriate scaling on both axes.
2. Plot the point corresponding to \(8\sqrt{3}\), which is approximately (13.856, 0).
3. Alternatively, use a function that includes \(8\sqrt{3}\) as a key value to demonstrate its application.

These visual aids help in understanding the magnitude and application of \(8\sqrt{3}\), making abstract concepts more concrete and accessible.

The mathematical constant \( \sqrt{3} \), also known as the square root of 3, has a rich historical background that dates back to ancient civilizations. This irrational number, approximately equal to 1.732, appears in various mathematical contexts and has been studied for centuries.

One of the earliest known uses of \( \sqrt{3} \) is in the work of the ancient Greek mathematician Euclid, who used it in his famous treatise "Elements." Euclid explored the properties of numbers and geometric shapes, laying the groundwork for much of modern mathematics.

The constant also appears in the geometry of regular polygons, particularly in the equilateral triangle. The height of an equilateral triangle with side length 2 is \( \sqrt{3} \), demonstrating its geometric significance. This relationship was likely known to Greek mathematicians and has been used in various geometric constructions since antiquity.

In the context of ancient Indian mathematics, \( \sqrt{3} \) is prominently featured in the Sulba Sutras, ancient Hindu texts that provide rules for constructing altars and other geometric shapes. These texts, dating back to around 800 BCE, show the advanced understanding of geometry and mathematics in ancient India.

During the Middle Ages, Islamic mathematicians and scholars further explored the properties of \( \sqrt{3} \). Al-Khwarizmi, a Persian mathematician, included discussions of square roots in his works, which were later translated into Latin and influenced European mathematics during the Renaissance.

In modern times, \( 8\sqrt{3} \) has been utilized in various fields such as engineering, architecture, and physics. Its applications range from calculating distances in triangular formations to analyzing wave functions and vibrations in physical systems. Understanding the historical context of \( \sqrt{3} \) provides insight into its enduring importance in mathematics and its continuous influence on various scientific disciplines.

The expression \(8 \times \sqrt{3}\) holds significant importance in various educational contexts. Understanding and working with this mathematical concept can provide students with a solid foundation in algebra, geometry, and trigonometry. Here are several ways in which \(8 \times \sqrt{3}\) is important in education:

• Algebraic Skills:

  Learning to manipulate and simplify expressions involving square roots, such as \(8 \times \sqrt{3}\), helps students develop strong algebraic skills. It encourages critical thinking and problem-solving abilities, which are essential for advanced mathematics.

• Geometric Understanding:

  The expression \(8 \times \sqrt{3}\) frequently appears in geometric contexts, particularly in relation to equilateral triangles and other regular polygons. For example, the height of an equilateral triangle with side length \(8\) can be calculated using \(8 \times \sqrt{3} / 2\). This reinforces students' understanding of geometric properties and the Pythagorean theorem.

• Trigonometric Applications:

  In trigonometry, the value of \(8 \times \sqrt{3}\) can be related to specific angles and their sine or cosine values. Understanding these relationships enhances students' comprehension of trigonometric functions and their applications in real-world problems.

• Real-World Applications:

  Practical examples of \(8 \times \sqrt{3}\) can be found in various fields such as engineering, architecture, and physics. By studying this expression, students can appreciate the relevance of mathematics in everyday life and professional settings.

• Problem-Solving Skills:

  Working with \(8 \times \sqrt{3}\) requires students to apply multiple mathematical concepts simultaneously, fostering their ability to tackle complex problems. This skill is crucial for success in higher education and STEM careers.

• Preparation for Advanced Topics:

  Understanding expressions like \(8 \times \sqrt{3}\) prepares students for more advanced mathematical concepts, such as calculus and linear algebra, where they will encounter similar expressions and need to manipulate them efficiently.

Incorporating \(8 \times \sqrt{3}\) into the curriculum not only enriches students' mathematical knowledge but also prepares them for future academic and professional challenges. By mastering such expressions, students gain confidence and competence in their mathematical abilities.

Practical applications of the mathematical concept "8 times square root of 3" span various fields:

1. Engineering: In structural engineering, calculations involving dimensions and forces often utilize precise mathematical constants like "8 times square root of 3" to ensure stability and safety of constructions.
2. Physics: When analyzing wave frequencies or resonance phenomena, this mathematical value appears in formulas describing natural frequencies of systems.
3. Computer Graphics: Algorithms for rendering 3D shapes or simulating physical phenomena in computer graphics rely on accurate mathematical constants, including "8 times square root of 3," to ensure realistic simulations.
4. Mathematical Modeling: In mathematical modeling of complex systems such as population dynamics or economic growth, this value can be crucial in formulating differential equations and predicting behaviors over time.
5. Chemistry: Quantum mechanical calculations in chemistry often involve precise mathematical constants to describe molecular structures and predict chemical behaviors accurately.

Advanced mathematical concepts related to "8 times square root of 3" include:

1. Trigonometric Identities: It appears in various trigonometric identities and formulas, such as the tangent of 75 degrees or pi/5 radians.
2. Geometric Applications: In geometry, it relates to specific geometric configurations and calculations involving angles and side lengths.
3. Number Theory: As an irrational number, its properties in number theory involve continued fractions and approximations.
4. Algebraic Expressions: It appears in algebraic equations and expressions involving radicals and powers.
5. Complex Analysis: In complex analysis, it plays a role in understanding the behavior of functions and their mappings in the complex plane.