Topic 3 divided by the square root of 3: Discover the simplicity and elegance of dividing 3 by the square root of 3 in this comprehensive guide. We break down the steps, explain the mathematical background, and explore real-world applications, making this concept easy to understand and apply. Perfect for students, educators, and math enthusiasts looking to deepen their knowledge.
Table of Content
- Calculation of 3 Divided by the Square Root of 3
- Introduction to the Concept
- Mathematical Background
- Simplifying 3 Divided by the Square Root of 3
- Step-by-Step Simplification Process
- Rationalizing the Denominator
- Common Mistakes and Misconceptions
- Applications in Real-World Problems
- Advanced Mathematical Implications
- Frequently Asked Questions
- Conclusion and Summary
- YOUTUBE:
Calculation of 3 Divided by the Square Root of 3
To calculate \( \frac{3}{\sqrt{3}} \), follow these steps:
- Identify the given expression: \( \frac{3}{\sqrt{3}} \).
- To rationalize the denominator, multiply both the numerator and the denominator by \( \sqrt{3} \).
- This gives \( \frac{3 \cdot \sqrt{3}}{\sqrt{3} \cdot \sqrt{3}} \).
- Simplify the denominator: \( \sqrt{3} \cdot \sqrt{3} = 3 \).
- This results in \( \frac{3 \cdot \sqrt{3}}{3} \).
- Cancel out the 3s in the numerator and the denominator: \( \frac{\cancel{3} \cdot \sqrt{3}}{\cancel{3}} = \sqrt{3} \).
Therefore, \( \frac{3}{\sqrt{3}} = \sqrt{3} \).
In summary:
- Given expression: \( \frac{3}{\sqrt{3}} \)
- Rationalized form: \( \sqrt{3} \)
This calculation demonstrates the process of rationalizing the denominator and simplifying the expression.
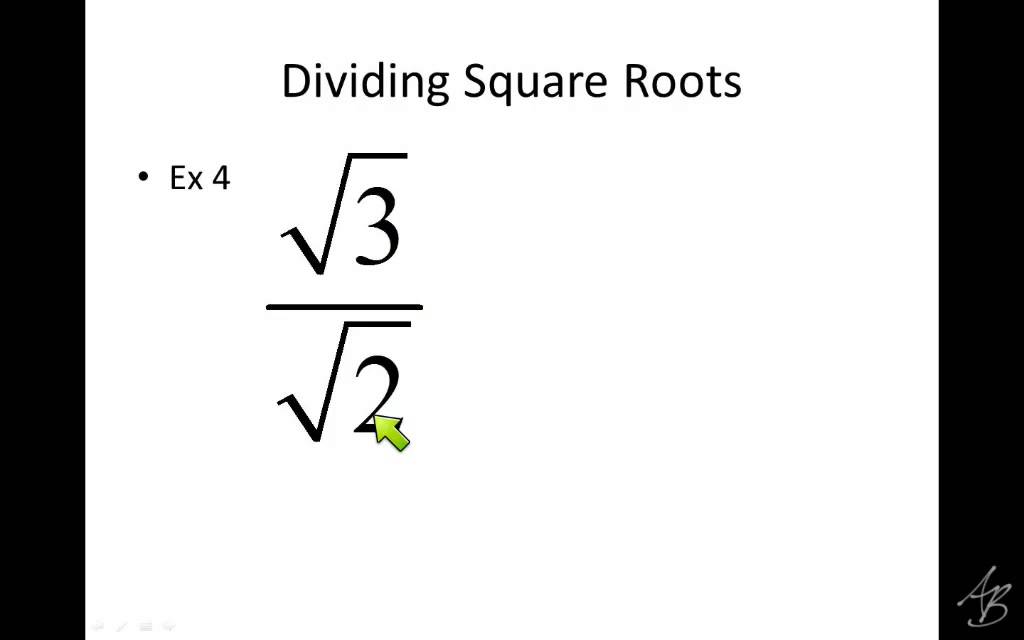
READ MORE:
Introduction to the Concept
Understanding how to divide 3 by the square root of 3 involves basic principles of algebra and rationalization. This concept is a fundamental part of simplifying expressions in mathematics. Below, we will break down the process step-by-step to ensure clarity and comprehension.
Firstly, consider the expression:
\[ \frac{3}{\sqrt{3}} \]
To simplify this, we need to eliminate the square root from the denominator. We do this by multiplying both the numerator and the denominator by the square root of 3:
\[ \frac{3}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} \]
When we multiply these expressions, we get:
\[ \frac{3 \cdot \sqrt{3}}{\sqrt{3} \cdot \sqrt{3}} \]
This simplifies to:
\[ \frac{3 \sqrt{3}}{3} \]
Since the 3 in the numerator and the 3 in the denominator cancel each other out, we are left with:
\[ \sqrt{3} \]
Therefore, the simplified form of \(\frac{3}{\sqrt{3}}\) is \(\sqrt{3}\).
- This process is known as rationalizing the denominator.
- It helps in simplifying expressions and making them easier to work with in further calculations.
By understanding and applying this process, you can simplify similar expressions and enhance your mathematical skills.
Mathematical Background
To fully grasp the concept of dividing 3 by the square root of 3, it's essential to understand some foundational mathematical principles. This section will explore these principles in detail.
- Square Roots: The square root of a number \( x \) is a value that, when multiplied by itself, gives \( x \). For example, \( \sqrt{3} \times \sqrt{3} = 3 \).
- Rationalizing the Denominator: This is the process of eliminating the square root or other irrational numbers from the denominator of a fraction by multiplying both the numerator and the denominator by a suitable value.
Consider the expression:
\[ \frac{3}{\sqrt{3}} \]
To simplify this, we rationalize the denominator by multiplying the numerator and the denominator by \( \sqrt{3} \):
\[ \frac{3}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{3 \cdot \sqrt{3}}{\sqrt{3} \cdot \sqrt{3}} \]
The denominator simplifies as follows:
\[ \sqrt{3} \cdot \sqrt{3} = 3 \]
Thus, the expression becomes:
\[ \frac{3 \cdot \sqrt{3}}{3} \]
Here, the 3 in the numerator and the 3 in the denominator cancel each other out:
\[ \frac{3 \sqrt{3}}{3} = \sqrt{3} \]
Therefore, we find that:
\[ \frac{3}{\sqrt{3}} = \sqrt{3} \]
Understanding this process is crucial for simplifying mathematical expressions and solving algebraic equations. Rationalizing the denominator is a common technique used in various mathematical fields, including algebra, calculus, and beyond.
In summary, the mathematical background behind dividing 3 by the square root of 3 involves understanding square roots and the method of rationalizing the denominator, which simplifies the expression to a more manageable form.
Simplifying 3 Divided by the Square Root of 3
To simplify the expression \( \frac{3}{\sqrt{3}} \), we follow a systematic approach involving rationalization of the denominator. Here's a detailed step-by-step guide:
-
Identify the expression:
The expression we need to simplify is:
\[ \frac{3}{\sqrt{3}} \] -
Rationalize the denominator:
To eliminate the square root in the denominator, multiply both the numerator and the denominator by \( \sqrt{3} \):
\[ \frac{3}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} \] -
Perform the multiplication:
Multiply the numerators together and the denominators together:
\[ \frac{3 \cdot \sqrt{3}}{\sqrt{3} \cdot \sqrt{3}} \] -
Simplify the denominator:
The denominator \( \sqrt{3} \cdot \sqrt{3} \) simplifies to 3:
\[ \sqrt{3} \cdot \sqrt{3} = 3 \] -
Combine the results:
The expression now looks like this:
\[ \frac{3 \sqrt{3}}{3} \] -
Simplify the fraction:
The 3 in the numerator and the 3 in the denominator cancel each other out:
\[ \frac{3 \sqrt{3}}{3} = \sqrt{3} \]
Thus, the simplified form of \( \frac{3}{\sqrt{3}} \) is \( \sqrt{3} \). This process of rationalizing the denominator is a valuable technique in algebra, ensuring expressions are in their simplest form and making further mathematical operations more straightforward.
Step-by-Step Simplification Process
The process of simplifying \( \frac{3}{\sqrt{3}} \) involves rationalizing the denominator and ensuring the expression is in its simplest form. Here is a detailed, step-by-step guide:
-
Write down the original expression:
The expression to be simplified is:
\[ \frac{3}{\sqrt{3}} \] -
Multiply by a form of 1:
To rationalize the denominator, multiply both the numerator and the denominator by \( \sqrt{3} \). This step eliminates the square root from the denominator:
\[ \frac{3}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} \] -
Multiply the numerators:
Perform the multiplication in the numerator:
\[ 3 \times \sqrt{3} = 3\sqrt{3} \] -
Multiply the denominators:
Perform the multiplication in the denominator:
\[ \sqrt{3} \times \sqrt{3} = 3 \] -
Form the new fraction:
Combine the results from the numerator and the denominator:
\[ \frac{3\sqrt{3}}{3} \] -
Cancel common factors:
The 3 in the numerator and the 3 in the denominator cancel each other out:
\[ \frac{3\sqrt{3}}{3} = \sqrt{3} \]
After following these steps, we find that the simplified form of \( \frac{3}{\sqrt{3}} \) is \( \sqrt{3} \). This rationalization process ensures that the expression is in a more manageable form, free of irrational denominators.
Rationalizing the Denominator
Rationalizing the denominator is a technique used to eliminate irrational numbers, such as square roots, from the denominator of a fraction. This process makes expressions easier to work with in further calculations. Let's go through the steps to rationalize the denominator of \( \frac{3}{\sqrt{3}} \).
-
Identify the original expression:
The expression we need to simplify is:
\[ \frac{3}{\sqrt{3}} \] -
Multiply by the conjugate:
In this case, since we have a simple square root, we multiply both the numerator and the denominator by \( \sqrt{3} \) to rationalize the denominator:
\[ \frac{3}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} \]This fraction is equivalent to multiplying by 1, so the value of the expression does not change.
-
Multiply the numerators:
Carry out the multiplication in the numerator:
\[ 3 \times \sqrt{3} = 3\sqrt{3} \] -
Multiply the denominators:
Perform the multiplication in the denominator:
\[ \sqrt{3} \times \sqrt{3} = 3 \] -
Combine the results:
Form the new fraction with the results from the multiplication:
\[ \frac{3\sqrt{3}}{3} \] -
Simplify the fraction:
Cancel out the common factor of 3 in the numerator and the denominator:
\[ \frac{3\sqrt{3}}{3} = \sqrt{3} \]
Thus, the rationalized form of \( \frac{3}{\sqrt{3}} \) is \( \sqrt{3} \). By rationalizing the denominator, we have transformed the expression into a simpler and more convenient form for further calculations.
Common Mistakes and Misconceptions
When simplifying expressions like \( \frac{3}{\sqrt{3}} \), several common mistakes and misconceptions can occur. Being aware of these can help avoid errors and ensure accurate results. Here are some frequent pitfalls:
-
Forgetting to Rationalize the Denominator:
One of the most common mistakes is neglecting to rationalize the denominator. Leaving the expression as \( \frac{3}{\sqrt{3}} \) without rationalizing can lead to complications in further calculations.
-
Incorrect Multiplication:
Another common error occurs during the multiplication step. It's crucial to multiply both the numerator and the denominator by the same value, \( \sqrt{3} \), to maintain the equality of the expression:
\[ \frac{3}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} \] -
Misinterpreting the Square Root Multiplication:
Students often misinterpret the multiplication of square roots. Remember, \( \sqrt{3} \times \sqrt{3} \) simplifies to 3, not \( \sqrt{9} \):
\[ \sqrt{3} \times \sqrt{3} = 3 \] -
Not Simplifying Completely:
After rationalizing, the expression should be simplified completely. For instance, in \( \frac{3\sqrt{3}}{3} \), the 3s cancel out, leaving:
\[ \frac{3\sqrt{3}}{3} = \sqrt{3} \] -
Misunderstanding Rationalization Purpose:
Some may not understand why we rationalize the denominator. The purpose is to convert the expression into a simpler form that is easier to work with in subsequent calculations.
By being aware of these common mistakes and misconceptions, students can approach the process of rationalizing denominators with greater confidence and accuracy. Correctly simplifying \( \frac{3}{\sqrt{3}} \) to \( \sqrt{3} \) enhances mathematical problem-solving skills and lays a solid foundation for more advanced topics.
Applications in Real-World Problems
Understanding how to simplify \( \frac{3}{\sqrt{3}} \) is not just an academic exercise; it has practical applications in various real-world problems. Here are some examples of how this mathematical concept is used:
-
Physics and Engineering:
In physics and engineering, simplifying expressions involving square roots is crucial for solving problems related to wave functions, electrical circuits, and mechanical vibrations. For instance, calculating the impedance in AC circuits often requires rationalizing the denominator to find a more workable solution.
-
Architecture and Construction:
Architects and construction engineers use these simplifications when dealing with geometric designs and structural calculations. Simplifying the ratios helps in determining the precise measurements and scaling of components.
-
Computer Graphics:
In computer graphics, rationalizing denominators helps in algorithms for rendering images and transformations. Simplified expressions allow for more efficient calculations, leading to faster processing times and smoother graphics.
-
Statistics and Probability:
In statistics, expressions involving square roots are common in standard deviation and variance calculations. Rationalizing these expressions simplifies the formulas, making it easier to interpret statistical data and derive meaningful conclusions.
-
Finance and Economics:
Financial analysts use simplified expressions to model economic scenarios and predict market trends. For example, calculating the volatility of stock prices often involves square root expressions that need to be simplified for accurate analysis.
By mastering the technique of rationalizing the denominator and simplifying expressions like \( \frac{3}{\sqrt{3}} \), you can enhance your problem-solving skills across various fields. This mathematical skill is not only essential for academic success but also for practical applications in diverse professional disciplines.
Advanced Mathematical Implications
The expression \( \frac{3}{\sqrt{3}} \) has significant implications in various advanced mathematical concepts. Below, we explore some of these implications in detail.
- Normalization in Vector Calculus:
In vector calculus, normalizing a vector involves dividing it by its magnitude to produce a unit vector. This process often includes expressions similar to \( \frac{3}{\sqrt{3}} \). For example, given a vector \( \mathbf{v} = [3, 3, 3] \), its magnitude is \( \sqrt{3^2 + 3^2 + 3^2} = 3\sqrt{3} \). The normalized vector is \( \mathbf{u} = \left[\frac{3}{3\sqrt{3}}, \frac{3}{3\sqrt{3}}, \frac{3}{3\sqrt{3}}\right] = \left[\frac{1}{\sqrt{3}}, \frac{1}{\sqrt{3}}, \frac{1}{\sqrt{3}}\right] \).
- Trigonometric Identities:
Expressions involving \( \frac{3}{\sqrt{3}} \) frequently appear in trigonometric identities. For instance, simplifying the expression aids in solving complex trigonometric equations and can be used in conjunction with identities such as \( \sin^2 \theta + \cos^2 \theta = 1 \).
- Probability and Statistics:
In probability theory, standard deviations and variances often involve square roots. Simplifying fractions like \( \frac{3}{\sqrt{3}} \) can be crucial when dealing with normal distributions and other statistical computations.
- Quantum Mechanics:
In quantum mechanics, wave functions and probabilities often require normalization. The process involves expressions similar to \( \frac{3}{\sqrt{3}} \), especially when dealing with spherical harmonics and angular momentum states.
To delve deeper into these advanced topics, let's consider some detailed mathematical derivations and their implications:
- Normalization of Eigenvectors:
When normalizing eigenvectors in linear algebra, expressions like \( \frac{3}{\sqrt{3}} \) often emerge. For an eigenvector \( \mathbf{v} \) with components proportional to 3, normalizing it requires dividing each component by \( \sqrt{3} \). This results in components of \( \frac{3}{\sqrt{3}} = \sqrt{3} \), simplifying to consistent eigenvalues.
- Application in Fourier Transforms:
Fourier transforms in signal processing often involve root-based simplifications. Converting between time and frequency domains requires precise calculations, where \( \frac{3}{\sqrt{3}} \) simplifies the computation of harmonic frequencies.
- Geometry and Lattice Structures:
In crystallography and geometry, lattice structures often involve ratios of lengths and diagonals. Simplifying expressions like \( \frac{3}{\sqrt{3}} \) helps in determining lattice parameters and angles between crystallographic planes.
In summary, the simplification of \( \frac{3}{\sqrt{3}} \) is not just a mathematical exercise but a foundational tool across various advanced mathematical fields, enhancing precision and clarity in complex computations.

Frequently Asked Questions
Below are some common questions related to the division of 3 by the square root of 3, along with detailed answers to help clarify the concept:
-
What is 3 divided by the square root of 3?
The division of 3 by the square root of 3 can be expressed as \( \frac{3}{\sqrt{3}} \). To simplify, you multiply both the numerator and the denominator by \( \sqrt{3} \) to rationalize the denominator:
\[ \frac{3}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{3\sqrt{3}}{3} = \sqrt{3} \]
So, \( \frac{3}{\sqrt{3}} = \sqrt{3} \approx 1.732 \).
-
Why do we rationalize the denominator?
Rationalizing the denominator is a common practice in mathematics to make expressions easier to work with and understand. It involves eliminating any irrational numbers from the denominator of a fraction. This is done by multiplying both the numerator and the denominator by a conjugate or the appropriate form that will cancel out the irrational part in the denominator.
-
What are the steps to rationalize the denominator for \( \frac{3}{\sqrt{3}} \)?
- Identify the irrational part of the denominator, which is \( \sqrt{3} \).
- Multiply both the numerator and the denominator by \( \sqrt{3} \):
- \[ \frac{3}{\sqrt{3}} \times \frac{\sqrt{3}}{\sqrt{3}} = \frac{3\sqrt{3}}{3} \]
- Simplify the expression: \[ \frac{3\sqrt{3}}{3} = \sqrt{3} \]
Thus, the simplified form is \( \sqrt{3} \).
-
What is the decimal equivalent of \( \sqrt{3} \)?
The decimal equivalent of \( \sqrt{3} \) is approximately 1.732050807569. This value is often rounded to 1.732 for simplicity.
-
Can \( \frac{3}{\sqrt{3}} \) be simplified further without a calculator?
Yes, \( \frac{3}{\sqrt{3}} \) simplifies to \( \sqrt{3} \), which is the simplest form of the expression. This can be done without a calculator by following the rationalization steps outlined above.
Conclusion and Summary
The process of simplifying the expression \( \frac{3}{\sqrt{3}} \) reveals interesting aspects of mathematical operations involving radicals and rationalization. By multiplying the numerator and the denominator by the conjugate of the denominator, we simplify the expression to \( \sqrt{3} \). This result underscores the importance of understanding and applying the concept of rationalization in simplifying expressions.
In this guide, we have explored the step-by-step process of simplifying \( \frac{3}{\sqrt{3}} \):
- Multiplying by the conjugate to remove the square root from the denominator.
- Simplifying the resulting expression to its simplest radical form.
- Understanding the importance of rationalizing the denominator in various mathematical contexts.
This simplification process is not only a fundamental skill in algebra but also has implications in higher-level mathematics, including calculus and complex number theory. Rationalizing denominators helps in performing operations that involve radicals more efficiently and accurately.
In summary, mastering the technique of rationalization and simplification is crucial for solving algebraic expressions and understanding more complex mathematical concepts. This fundamental skill bridges basic arithmetic operations and advanced mathematical theories, demonstrating its significance in various applications.
By following the methods outlined in this guide, one can confidently simplify similar expressions and apply these principles to solve more complex mathematical problems.
Cách tìm Căn bậc hai của số 3 - Hướng dẫn chi tiết
READ MORE:
Học cách chia các căn bậc hai