Topic what is 2 square root of 3: Discover the intriguing world of mathematics with our comprehensive guide to understanding "what is 2 square root of 3." This article delves into the mathematical definition, practical applications, and visualization techniques to make this concept clear and fascinating. Unlock the secrets of this fundamental expression and see its significance in various fields.
Table of Content
Understanding 2√3
The expression 2\sqrt{3} represents a mathematical value involving the square root of 3, multiplied by 2. To fully understand this expression, let's break it down:
What is the Square Root of 3?
The square root of 3, denoted as \sqrt{3}, is an irrational number. Its approximate decimal value is 1.732. This means:
\sqrt{3} \approx 1.732
Multiplying by 2
When we multiply the square root of 3 by 2, we get:
2 \times \sqrt{3} \approx 2 \times 1.732 = 3.464
Applications of 2√3
The value 2\sqrt{3} appears frequently in various mathematical and scientific contexts, such as:
- Geometry: In calculations involving equilateral triangles, where the height is given by
\sqrt{3}/2times the side length, and certain other geometric shapes. - Trigonometry: In solving trigonometric equations and functions, especially in the context of 30-60-90 triangles.
- Physics: In formulas involving wave mechanics and other physical phenomena where square roots of numbers are used.
Visual Representation
For a better understanding, let's look at a simple example:
Consider a right triangle where the sides are in the ratio 1:√3:2. The hypotenuse of such a triangle is twice the length of the shorter leg:
Hypotenuse = 2 \times \sqrt{3} \times (shorter\ leg)
Conclusion
The expression 2\sqrt{3} is an important mathematical constant that is useful in various branches of mathematics and science. Understanding how to manipulate and apply this expression can be beneficial in solving complex problems.
Mathematical Notation
Using MathJax, we can clearly represent the value as:
2\sqrt{3} or approximately 3.464.
READ MORE:
Introduction to 2√3
The expression 2√3 represents a mathematical term involving the square root of 3, multiplied by 2. This form appears frequently in algebra and geometry, where simplifying square roots is a common task. Understanding 2√3 involves breaking down the square root and recognizing its role in various mathematical operations.
- Definition: The term
2√3means 2 times the square root of 3. In mathematical notation, it is written as \(2 \sqrt{3}\). - Exact Form: The exact form of this expression remains as
2√3, which is an irrational number since √3 cannot be simplified to a rational number. - Decimal Form: By evaluating the square root of 3 (approximately 1.732), the decimal form of
2√3can be calculated as: \[2 \times 1.732 = 3.464\] - Simplification: The expression
2√3is already in its simplest form, as the square root of 3 cannot be simplified further.
Knowing how to manipulate and understand 2√3 can aid in solving more complex algebraic equations and understanding geometric properties. This term showcases the elegance of combining integers with irrational numbers to represent precise mathematical concepts.
Mathematical Definition
The mathematical expression \( 2\sqrt{3} \) represents a number that is approximately equal to \( 3.4641016151377544 \).
Decimal Approximation
The decimal approximation of \( 2\sqrt{3} \) is \( 3.4641016151377544 \).
Geometric Applications
The number \(2\sqrt{3}\) has various geometric applications due to its occurrence in different shapes and structures. Here are a few examples:
-
Equilateral Triangle:
In an equilateral triangle with side length \(a\), the height can be expressed as \( \frac{\sqrt{3}}{2}a \). For an equilateral triangle with side length \(4\), the height would be:
\[
h = \frac{\sqrt{3}}{2} \times 4 = 2\sqrt{3}
\]This height divides the triangle into two 30-60-90 right triangles.
-
Regular Hexagon:
A regular hexagon can be divided into six equilateral triangles. If each side of the hexagon is \(2\), then the distance between two opposite sides (the height of the hexagon) is:
\[
h = 2 \times \sqrt{3}
\]Thus, the height of the hexagon is \(2\sqrt{3}\).
-
Rectangular Prism:
Consider a rectangular prism with sides of length \(2\), \(2\sqrt{3}\), and \(4\). The diagonal \(d\) of one of the faces measuring \(2\) by \(2\sqrt{3}\) can be found using the Pythagorean theorem:
\[
d = \sqrt{(2)^2 + (2\sqrt{3})^2} = \sqrt{4 + 12} = \sqrt{16} = 4
\] -
Circle and Chord:
In a circle, the length of a chord that is \(2\sqrt{3}\) units away from the center can be calculated. For a circle with radius \(4\), the chord length \(c\) is given by:
\[
c = 2 \sqrt{4^2 - (2\sqrt{3})^2} = 2 \sqrt{16 - 12} = 2 \sqrt{4} = 4
\]

Trigonometric Relevance
The expression \(2\sqrt{3}\) has significant relevance in trigonometry, particularly in relation to specific angles and their trigonometric functions. Here are a few key points:
-
30-60-90 Triangle:
In a 30-60-90 triangle, the sides are in the ratio \(1 : \sqrt{3} : 2\). If the shortest side (opposite the 30° angle) is \(2\), the side opposite the 60° angle is:
\[
2 \times \sqrt{3} = 2\sqrt{3}
\]This demonstrates that \(2\sqrt{3}\) frequently appears in the context of these special triangles.
-
Sine and Cosine Functions:
The sine and cosine of 60° and 30° angles are often expressed using \(\sqrt{3}\). For example:
\[
\sin(60^\circ) = \cos(30^\circ) = \frac{\sqrt{3}}{2}
\]By multiplying by 2, we get:
\[
2 \times \frac{\sqrt{3}}{2} = \sqrt{3}
\]This highlights how \(2\sqrt{3}\) can relate to standard trigonometric values.
-
Unit Circle:
On the unit circle, the coordinates of points corresponding to common angles often involve \(\sqrt{3}\). For example, the coordinates for 30° (π/6) and 60° (π/3) are:
- 30°: \(\left(\frac{\sqrt{3}}{2}, \frac{1}{2}\right)\)
- 60°: \(\left(\frac{1}{2}, \frac{\sqrt{3}}{2}\right)\)
These coordinates can be scaled by 2 to yield values involving \(2\sqrt{3}\).
-
Law of Sines:
The Law of Sines states that for any triangle:
\[
\frac{a}{\sin(A)} = \frac{b}{\sin(B)} = \frac{c}{\sin(C)}
\]If one side of the triangle is \(2\sqrt{3}\) and the corresponding angle is 60°, then:
\[
\frac{2\sqrt{3}}{\sin(60^\circ)} = 2\sqrt{3} \times \frac{2}{\sqrt{3}} = 4
\]
Physics and Engineering Uses
The mathematical constant \(2\sqrt{3}\) has several significant applications in physics and engineering. Below are some detailed examples of its relevance:
1. Electrical Engineering
In electrical engineering, the root mean square (RMS) value is essential for analyzing AC (alternating current) circuits. RMS values are crucial because they provide a measure of the effective power of an AC signal, which is essential in designing and evaluating electrical systems.
For instance, in a sinusoidal waveform, the peak voltage (Vpeak) can be related to the RMS voltage (VRMS) using the square root of 2:
\[
V_{RMS} = \frac{V_{peak}}{\sqrt{2}}
\]
While this specific example uses \(\sqrt{2}\), the principles behind RMS calculations and waveform analysis apply similarly to other square roots, such as \(\sqrt{3}\) for different waveform analyses and calculations.
2. Mechanical Engineering
In mechanical engineering, particularly in the analysis of vibrations and waves, the term \(2\sqrt{3}\) appears in various contexts. For example, when analyzing the natural frequencies of systems or in the context of dynamic response analyses, such constants might be used in specific equations derived from the system's physical properties.
Another application is in stress and strain analysis where certain geometric configurations of materials result in stress distributions described by expressions involving square roots.
3. Signal Processing
In signal processing, constants like \(2\sqrt{3}\) can be relevant in filter design and analysis. For example, certain filter coefficients might involve such terms, especially in higher-order filters where the polynomial roots are critical in determining the filter's behavior.
4. Structural Engineering
Structural engineering often involves calculations related to load distributions and material strength. When dealing with trusses and beams, the angles and resulting forces can sometimes be represented with expressions involving \(\sqrt{3}\). For example, in the analysis of equilateral triangle trusses, the ratio of forces can involve such terms.
The relationship between the sides of a 30°-60°-90° triangle, which are in the ratio \(1:\sqrt{3}:2\), is particularly significant in structural designs that utilize these angles for stability and load distribution.
5. Civil Engineering
Civil engineers use radical expressions in various design and analysis tasks. For example, in road design, the super-elevation and banking angles for curves often involve calculations with square roots to ensure safe vehicle handling.
In hydraulic engineering, the flow rate of water in open channels and pipes may involve terms with \(\sqrt{3}\) when deriving certain empirical formulas for flow dynamics.
6. Optics and Wave Mechanics
In optics, the refractive index calculations and light wave propagation through different media can sometimes involve expressions with \(2\sqrt{3}\), particularly in complex lens designs and wave interference patterns.
Overall, the constant \(2\sqrt{3}\) finds its use in diverse fields of physics and engineering, often appearing in equations that describe natural phenomena, optimize designs, and ensure the accuracy of various technological applications.
Visualization and Examples
The value \(2\sqrt{3}\) can be visualized and exemplified in various ways to enhance understanding. Here are some detailed visualizations and examples:
Example 1: Using Geometry
Consider an equilateral triangle where each side is of length \(2\sqrt{3}\). To visualize this, draw an equilateral triangle and mark each side as \(2\sqrt{3}\). The height of this triangle, which can be calculated using the Pythagorean theorem, is 3 units, illustrating how \(2\sqrt{3}\) fits into geometric shapes.
Example 2: Cartesian Plane
On a Cartesian plane, plot a right triangle with one leg on the x-axis extending from the origin to \(2\sqrt{3}\) (approximately 3.464). The height of this triangle, determined by the other leg, could be a different number for various applications. Using the distance formula, the hypotenuse can be checked to validate the \(2\sqrt{3}\) side length.
Example 3: Real-world Application
In real-world applications, consider a hexagon where the distance from the center to any vertex is \(2\sqrt{3}\). This distance can be used in architecture and engineering to determine structural dimensions and properties.
Visualization with Desmos
Utilize online tools such as the Desmos graphing calculator to plot and manipulate the value \(2\sqrt{3}\) in various functions and geometrical shapes. This interactive approach can deepen comprehension and provide a tangible aspect to the abstract value.
Example 4: Calculating Areas
For a regular hexagon with a side length of \(2\sqrt{3}\), the area can be calculated using the formula for the area of a hexagon:
\[
\text{Area} = \frac{3\sqrt{3}}{2} \times (\text{side length})^2
\]
By substituting \(2\sqrt{3}\) for the side length, the calculation becomes:
\[
\text{Area} = \frac{3\sqrt{3}}{2} \times (2\sqrt{3})^2 = 54
\]
This provides a concrete example of how \(2\sqrt{3}\) is applied in geometric calculations.
Step-by-Step Calculation
- Draw a right triangle with legs of lengths 1 and \(2\sqrt{3}\).
- Calculate the hypotenuse using the Pythagorean theorem: \[ \text{Hypotenuse} = \sqrt{1^2 + (2\sqrt{3})^2} = \sqrt{1 + 12} = \sqrt{13} \]
- Verify the calculations using a calculator or graphing tool for accuracy.
These examples and visualizations demonstrate the versatile applications and understanding of \(2\sqrt{3}\) in both theoretical and practical contexts.
Historical Context
The concept of square roots has been significant throughout mathematical history, and the value of \(2\sqrt{3}\) has its place within this context. Ancient civilizations, including the Babylonians, Egyptians, Indians, Greeks, and Chinese, explored square roots for various applications in geometry, astronomy, and algebra.
One of the earliest recorded approximations of square roots comes from the Babylonians around 1800-1600 BC, who used a sophisticated numerical system to estimate values such as \(\sqrt{2}\). Their work, illustrated on clay tablets like the YBC 7289, demonstrates a remarkable level of mathematical understanding for the time.
In ancient Egypt, the Rhind Mathematical Papyrus (circa 1650 BC) reveals that the Egyptians used methods involving inverse proportions to find square roots. This indicates that the concept of extracting square roots was known and applied in practical ways, such as in the construction of pyramids and other architectural endeavors.
In India, significant contributions were made by mathematicians such as Baudhayana and Aryabhata. The Sulba Sutras (circa 800-500 BC) contain procedures for approximating square roots, including \(\sqrt{2}\) and \(\sqrt{3}\), which were crucial for their work in geometry. Aryabhata, in particular, provided methods for finding square roots that were highly accurate and influential in later mathematical developments.
The Greeks, notably through the work of Euclid and the Pythagoreans, also explored irrational numbers, understanding that the square roots of non-square numbers are irrational. Theaetetus is credited with the theorem stating that square roots of non-square integers are irrational, a fundamental insight in the study of numbers.
In China, the Writings on Reckoning from the Han Dynasty (202 BC - 186 BC) describe methods for approximating square roots using a form of excess and deficiency method, highlighting the global nature of mathematical discovery and the exchange of knowledge across cultures.
The symbol for the square root, an elongated 'R', was introduced by Regiomontanus in the 15th century, and the current symbol (√) was popularized in the 16th century by Christoph Rudolff in his publication "Coss". These advancements in notation made it easier to communicate and standardize mathematical procedures across different regions and eras.
Understanding the historical context of square roots, including specific values like \(2\sqrt{3}\), allows us to appreciate the depth and continuity of mathematical thought and its practical applications throughout human history.
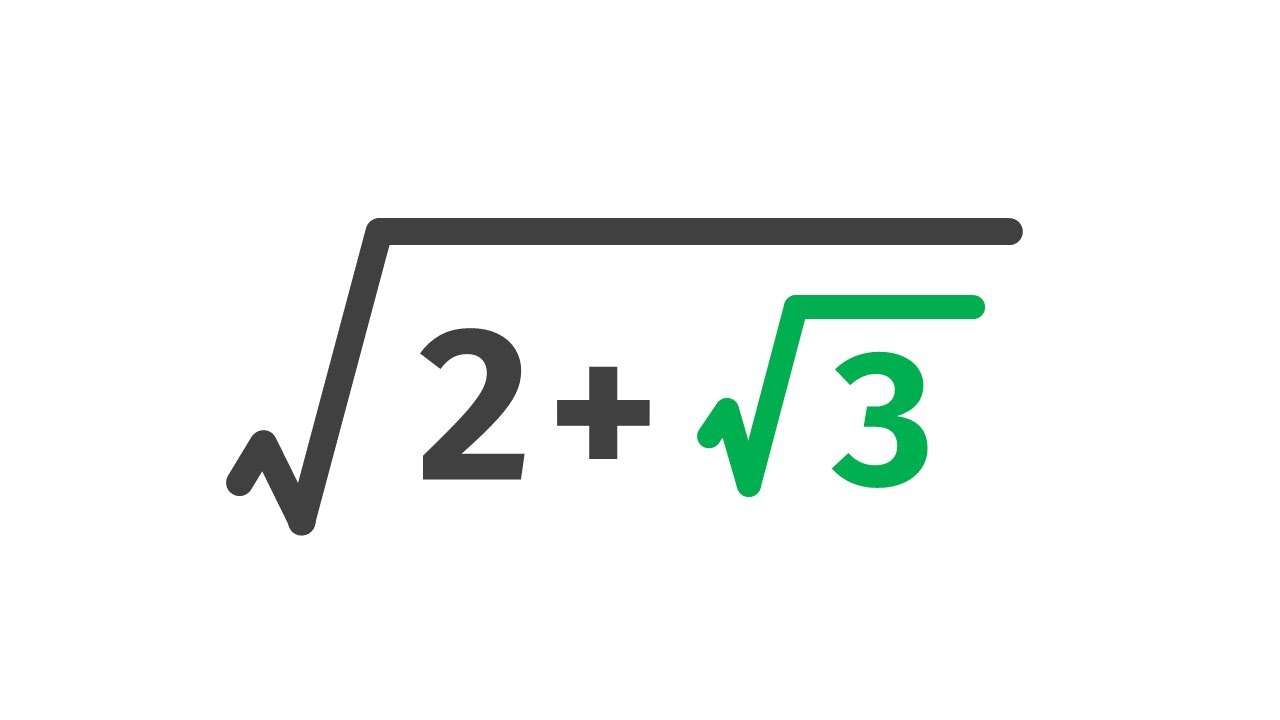
Calculation Methods
There are several methods to calculate the value of \(2\sqrt{3}\), each with varying levels of complexity and precision. Below, we outline a few common methods:
-
Prime Factorization Method:
Prime factorization involves breaking down the number into its prime factors. For \(\sqrt{3}\), since 3 is already a prime number, it remains as is.
- Identify the prime factors of the number under the square root. Here, \(\sqrt{3}\) has the prime factor 3.
- Since 2 is a constant multiplier, the calculation is \(2 \times \sqrt{3}\).
- The value of \(\sqrt{3}\) is approximately 1.732. Therefore, \(2 \times 1.732 \approx 3.464\).
-
Estimation Method:
This method involves estimating the value by considering nearby perfect squares.
- Identify the nearest perfect squares around 3, which are 1 (\(1^2\)) and 4 (\(2^2\)).
- Since 3 is closer to 4, \(\sqrt{3}\) is slightly less than 2.
- Multiply the approximate value of \(\sqrt{3}\) by 2: \(2 \times 1.732 \approx 3.464\).
-
Long Division Method:
This method provides a more accurate result through a step-by-step division process.
- Start with the number 3.00 and find the largest square less than or equal to 3, which is 1 (since \(1^2 = 1\)).
- Divide 3 by 1, giving a quotient of 1 and a remainder of 2.
- Bring down a pair of zeros, making it 200. Double the quotient (1) to get 2 and find a digit that, when added to 20 and multiplied by the same digit, is less than or equal to 200.
- Refine the process to achieve the desired precision. The final approximate value of \(\sqrt{3}\) is 1.732.
- Multiply \(2 \times 1.732 \approx 3.464\).
-
Using a Calculator:
Most scientific calculators have a square root function that simplifies the process.
- Enter the number 3 and press the square root button to get 1.732.
- Multiply the result by 2 to get \(2 \times 1.732 \approx 3.464\).
Each of these methods provides a way to calculate \(2\sqrt{3}\), with the final approximate value being 3.464. The choice of method depends on the desired level of precision and the tools available.
Common Mistakes to Avoid
When dealing with \( 2\sqrt{3} \), it's essential to be aware of common mistakes that can lead to errors in calculations and understanding. Below are some frequent errors and tips to avoid them:
-
Misinterpreting the Square Root Symbol: The square root symbol \( \sqrt{} \) always represents the principal (positive) square root. For instance, \( \sqrt{9} = 3 \), not \( \pm 3 \). When dealing with expressions like \( 2\sqrt{3} \), ensure you only consider the positive root of 3.
-
Incorrect Simplification: Avoid incorrectly simplifying expressions involving square roots. For example, \( \sqrt{a^2} = |a| \), not \( a \). Thus, \( \sqrt{4} = 2 \), not \( -2 \). For \( 2\sqrt{3} \), ensure no further incorrect simplifications are made.
-
Errors in Multiplication: Be careful when multiplying square roots and other numbers. For example, \( 2 \cdot \sqrt{3} \) should be calculated as \( 2\sqrt{3} \), not \( \sqrt{6} \). The multiplication rule \( a\sqrt{b} = a \cdot \sqrt{b} \) should be followed accurately.
-
Misinterpretation in Context: In physics or engineering contexts, ensure the correct application of \( 2\sqrt{3} \). Misinterpreting its value or misuse in equations can lead to significant errors. Double-check unit conversions and context-specific rules.
-
Calculator Errors: When using calculators, ensure the correct entry of \( 2\sqrt{3} \). Many calculators have a dedicated square root button (√). Ensure proper usage to avoid syntax errors or misinterpretations.
-
Forgetting to Rationalize: In some cases, it’s necessary to rationalize the denominator. For instance, if you have \( \frac{2\sqrt{3}}{3} \), check if rationalizing is required based on the context.
-
Neglecting Decimal Approximation: While \( 2\sqrt{3} \approx 3.464 \), ensure precision in applications requiring decimal approximations. Always round to the required decimal places as per the context.
By keeping these common mistakes in mind, you can ensure accurate calculations and a better understanding of expressions involving \( 2\sqrt{3} \).
FAQs about 2√3
Here are some frequently asked questions about the value 2√3:
-
What is 2√3 approximately equal to in decimal form?
2√3 is approximately equal to 3.464. This is calculated by multiplying 2 by the square root of 3, which is approximately 1.732.
-
How can 2√3 be used in geometry?
2√3 often appears in problems involving 30-60-90 triangles. For instance, in such a triangle, the length of the side opposite the 60° angle is 2√3 times the length of the shortest side.
-
What is the significance of 2√3 in trigonometry?
In trigonometry, 2√3 can represent the length of the side opposite the 60° angle in a 30-60-90 triangle when the shorter side is 1. It is also used in various trigonometric identities and equations.
-
Can 2√3 be simplified further?
No, 2√3 is already in its simplest form. It is the product of a rational number (2) and an irrational number (√3).
-
Is 2√3 a rational number?
No, 2√3 is not a rational number because it includes the square root of 3, which is an irrational number. Multiplying an irrational number by a rational number still results in an irrational number.
2√3 + √3 || Cách cộng 2√3 và √3
READ MORE:
Sản Phẩm Của Căn Bậc Ba Của 2 Và Căn Bậc Hai Của 3 Là Gì?