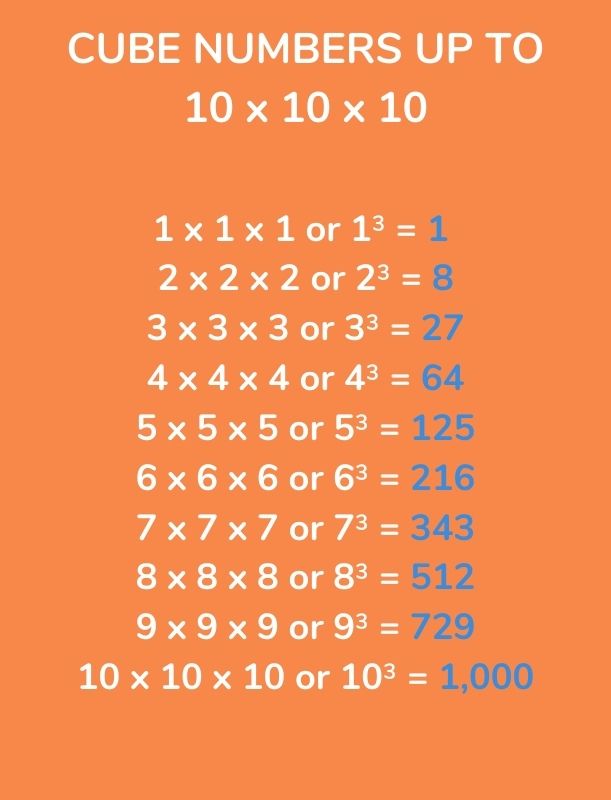Topic 3 square root of 6: The expression 3 square root of 6 might seem complex, but it's a fascinating topic in mathematics. This article delves into its calculation, significance, and real-world applications. Whether you're a student or a math enthusiast, discover how 3√6 plays a crucial role in various mathematical fields and everyday situations.
Table of Content
Understanding the Expression: 3√6
The expression \(3 \sqrt{6}\) combines a coefficient (3) with a square root (√6). Let's break down its components and understand its significance.
Components of the Expression
- 3: This is a constant multiplier, also known as the coefficient.
- √6: This represents the square root of 6, an irrational number.
Calculating the Value
To calculate the approximate value of \(3 \sqrt{6}\), follow these steps:
- Find the square root of 6: \(\sqrt{6} \approx 2.449\)
- Multiply the result by 3: \(3 \times 2.449 \approx 7.347\)
Decimal Approximation
Using the steps above, we find that:
\(3 \sqrt{6} \approx 7.347\)
Symbolic Representation
In symbolic form, we write:
\(3 \sqrt{6}\)
Applications
The expression \(3 \sqrt{6}\) appears in various mathematical contexts, including geometry, algebra, and calculus. Understanding how to manipulate and approximate it is crucial for solving complex problems.

READ MORE:
Introduction to 3√6
The expression \(3 \sqrt{6}\) combines a coefficient and a square root, making it an interesting topic in mathematics. Understanding this expression involves breaking it down into its components and exploring its significance in various mathematical contexts.
Breaking Down the Expression:
- 3: This is the coefficient, a constant multiplier.
- √6: This represents the square root of 6, an irrational number.
Steps to Calculate 3√6:
- Find the square root of 6: \(\sqrt{6} \approx 2.449\)
- Multiply the result by 3: \(3 \times 2.449 \approx 7.347\)
The expression \(3 \sqrt{6}\) results in an approximate value of 7.347. This value is useful in various mathematical applications, including geometry, algebra, and calculus.
Significance in Mathematics:
The expression \(3 \sqrt{6}\) appears in many mathematical problems and solutions, helping to simplify and solve complex equations. Its understanding is essential for students and enthusiasts aiming to deepen their knowledge of mathematical concepts.
Mathematical Definition and Properties
The expression \( \sqrt[3]{6} \) represents the cube root of 6. In mathematics, the cube root of a number \( x \) is a number \( y \) such that \( y^3 = x \). Therefore, \( \sqrt[3]{6} \) is the number \( y \) for which \( y^3 = 6 \).
Properties of \( \sqrt[3]{6} \)
- Irrational Number: The cube root of 6 is an irrational number, meaning it cannot be expressed as a simple fraction.
- Real Number: \( \sqrt[3]{6} \) is a real number since it exists on the number line.
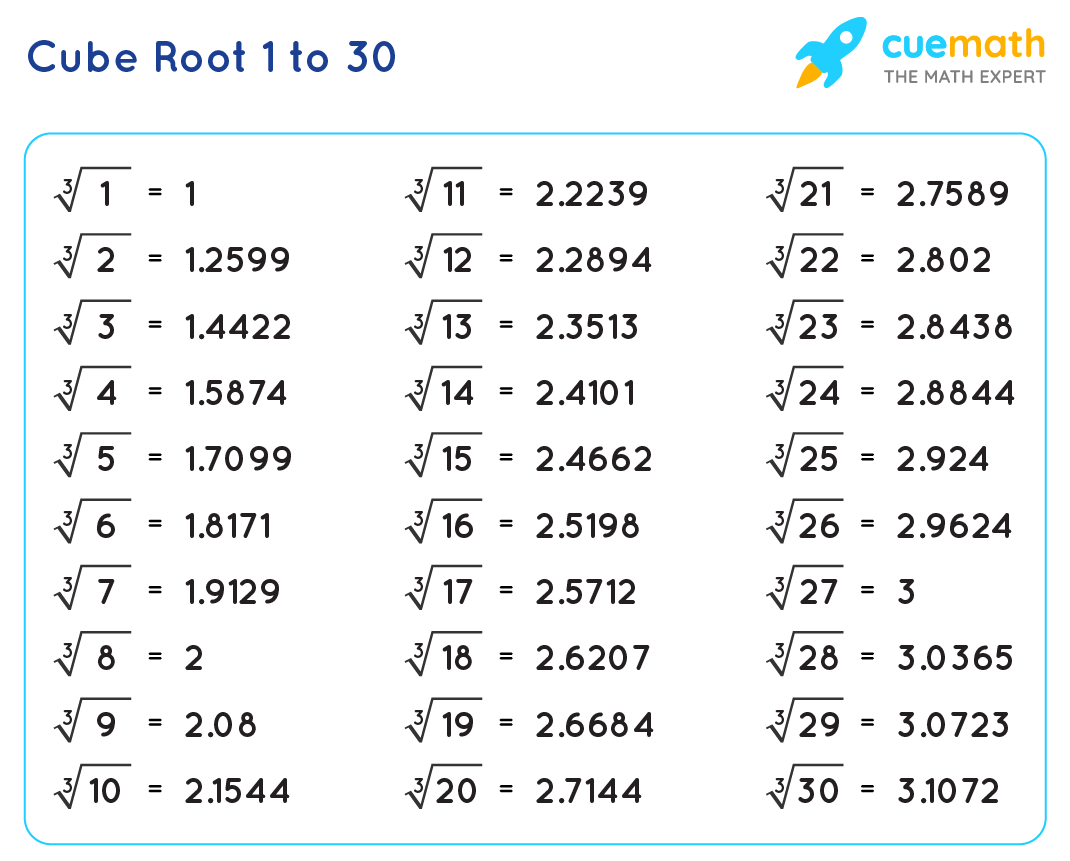
- Approximate Value: The approximate value of \( \sqrt[3]{6} \) is approximately 1.81712.
Mathematical Representation
The cube root of 6 can be represented using exponentiation as:
\[ \sqrt[3]{6} = 6^{\frac{1}{3}} \]
Properties of Cube Roots
- Unique Real Root: Every real number has exactly one real cube root.
- Product Property: The cube root of a product is the product of the cube roots: \( \sqrt[3]{a \cdot b} = \sqrt[3]{a} \cdot \sqrt[3]{b} \).
- Quotient Property: The cube root of a quotient is the quotient of the cube roots: \( \sqrt[3]{\frac{a}{b}} = \frac{\sqrt[3]{a}}{\sqrt[3]{b}} \).
Solving for Cube Roots
- Guess and Check: Estimate a value for \( \sqrt[3]{6} \) and refine the guess by checking if its cube is close to 6.
- Use a Calculator: Utilize a scientific calculator to find a more precise value.
- Newton's Method: Use iterative numerical methods to approximate the value.
Example Calculation Using Newton's Method
To find \( \sqrt[3]{6} \), we can use Newton's method with the function \( f(x) = x^3 - 6 \) and its derivative \( f'(x) = 3x^2 \).
- Start with an initial guess \( x_0 \). Let \( x_0 = 2 \).
- Iteratively apply the formula: \[ x_{n+1} = x_n - \frac{f(x_n)}{f'(x_n)} \]
- For the first iteration: \[ x_1 = 2 - \frac{2^3 - 6}{3 \cdot 2^2} = 2 - \frac{8 - 6}{12} = 2 - \frac{2}{12} = 2 - 0.1667 \approx 1.8333 \]
- Repeat the process until the desired accuracy is achieved.
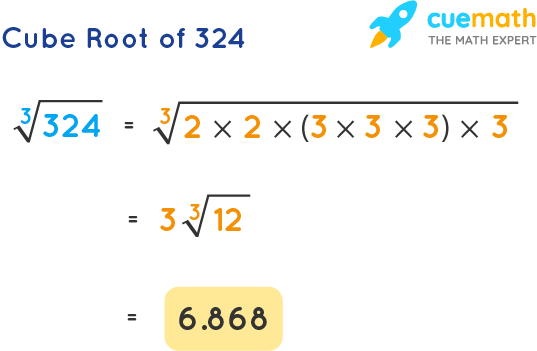
Calculating 3√6
The expression \( \sqrt[3]{6} \) refers to the cube root of 6. This section explains how to calculate \( \sqrt[3]{6} \) using various methods, including manual approximation and using calculators.
Method 1: Estimation and Refinement
One way to calculate the cube root of 6 is by estimating and refining our guess:
- Start with an initial guess. Let's choose \( x_0 = 2 \) since \( 2^3 = 8 \), which is close to 6.
- Apply the iterative formula for refinement: \[ x_{n+1} = x_n - \frac{x_n^3 - 6}{3x_n^2} \]
- First iteration: \[ x_1 = 2 - \frac{2^3 - 6}{3 \cdot 2^2} = 2 - \frac{8 - 6}{12} = 2 - 0.1667 \approx 1.8333 \]
- Second iteration: \[ x_2 = 1.8333 - \frac{1.8333^3 - 6}{3 \cdot 1.8333^2} \approx 1.8171 \]
- Continue this process until the value stabilizes to the desired accuracy.
Method 2: Using a Calculator
You can also use a scientific calculator to find \( \sqrt[3]{6} \) more directly:
- Enter the number 6.
- Use the cube root function (often labeled as \( \sqrt[3]{x} \) or \( x^{1/3} \)).
- The calculator will display the result: \( \sqrt[3]{6} \approx 1.81712 \).
Method 3: Online Root Calculators
Several online calculators can compute cube roots accurately. Here is a general method to use an online calculator:
- Navigate to a root calculator website.
- Select the cube root function.
- Enter the value 6 and calculate.
- The result will be displayed as approximately 1.81712.
Example Calculation
Let's verify this using a step-by-step approach:
- Choose \( x_0 = 2 \).
- First iteration: \[ x_1 = 2 - \frac{2^3 - 6}{3 \cdot 2^2} = 2 - \frac{2}{12} = 2 - 0.1667 = 1.8333 \]
- Second iteration: \[ x_2 = 1.8333 - \frac{1.8333^3 - 6}{3 \cdot 1.8333^2} = 1.8333 - 0.0162 = 1.8171 \]
- After further iterations, the value stabilizes around 1.81712.
Using these methods, we can accurately determine that \( \sqrt[3]{6} \approx 1.81712 \).
Approximate Value of 3√6
The value of can be approximated using various mathematical methods. Below is a detailed step-by-step guide to calculating this value:
Step-by-Step Calculation Using Estimation
Identify the range: Since is between (which is ) and (which is ), the square root of lies between and .
Initial guess: Choose a number between and . Let's start with .
Square the guess: . Since is greater than , try a smaller number.
Refine the guess: Try . Square it: . This value is less than , so it's a closer approximation.
Further refinement: Next, try . Square it: . This is very close to .
Multiply by 3: Now, multiply the approximate value of by to get .
Using a Calculator
For a more precise value, use a calculator:
- Enter and press the square root button to get approximately .
- Multiply this result by to get approximately .
Therefore, the approximate value of is approximately when rounded to three decimal places.

Applications in Mathematics
The expression \(3\sqrt{6}\) finds its applications across various fields of mathematics due to its involvement in calculations related to geometry, algebra, and calculus.
- Geometry: In geometry, \(3\sqrt{6}\) can be used to determine dimensions and distances. For example, when calculating the diagonal of a rectangular prism or the length of the sides of certain geometric shapes involving square roots, this expression becomes particularly useful.
- Algebra: In algebra, \(3\sqrt{6}\) appears in solutions to quadratic equations and polynomial roots where simplification involves square roots. It can also help in solving radical equations where the expression is part of the solution.
- Calculus: In calculus, \(3\sqrt{6}\) can be applied in integrals and derivatives where functions involve square roots. It might appear in the context of definite integrals, especially in areas under curves where the square root functions are involved.
- Physics: This expression can be found in physics equations involving gravitational potential energy and wave equations. For instance, calculations involving potential energy might use square roots to express distances or heights.
- Engineering: Engineers might use \(3\sqrt{6}\) when dealing with stress and strain calculations, optimizing structures, or even in electrical engineering where it might appear in impedance calculations in AC circuits.
- Statistics: In statistics, this expression can be used in normal distribution calculations and in finding standard deviations or z-scores that involve square roots.
Understanding the application of \(3\sqrt{6}\) in these fields helps in simplifying complex problems and finding precise solutions.
3√6 in Geometry
The mathematical expression \(3\sqrt{6}\) appears in various geometric contexts, providing interesting relationships and applications in both plane and solid geometry.
-
Regular Octahedron: The edge length of a regular octahedron is \( \sqrt{6} \) times the radius of its inscribed sphere. This relationship is crucial in understanding the geometry of polyhedra.
-
Cube and Double Cube: In solid geometry, the square root of 6 appears as the longest distance between corners (vertices) of a double cube. The edge lengths of a cube with a total surface area of 1 are related to \( \sqrt{6} \).
-
Equilateral Triangle: The side length of the square enclosing an equilateral triangle of side 2 is given by \( \frac{\sqrt{6} + \sqrt{2}}{2} \). This demonstrates the application of \( \sqrt{6} \) in planar constructions.
-
Root Rectangles: The construction of dynamic rectangles illustrates the square root of 6, emphasizing its importance in geometric mean and proportional relationships.
-
Inscribed Shapes: The height of certain inscribed shapes, such as a regular tetrahedron or octahedron, utilizes the square root of 6 for precise measurement and construction.
These geometric applications of \(3\sqrt{6}\) showcase its versatility and significance in both theoretical and practical geometry, highlighting its role in various mathematical constructions and shapes.
3√6 in Algebra
In algebra, the expression \(3\sqrt{6}\) can be explored in various contexts. Below are some common algebraic manipulations and uses of \(3\sqrt{6}\).
Simplification
Simplifying expressions involving \(3\sqrt{6}\) often involves the use of properties of radicals.
- Product Rule for Radicals: The product of two square roots is the square root of the product, i.e., \(\sqrt{a} \cdot \sqrt{b} = \sqrt{ab}\). For example:
- \(\sqrt{6} \cdot \sqrt{3} = \sqrt{18} = 3\sqrt{2}\).
- Combining like terms: Similar to like terms in polynomials, terms involving radicals can be combined if the radical part is the same. For instance:
- \(3\sqrt{6} + 2\sqrt{6} = 5\sqrt{6}\).
Operations Involving \(3\sqrt{6}\)
Several operations can be performed with \(3\sqrt{6}\). Here are some examples:
- Squaring: When squaring \(3\sqrt{6}\), apply the square to both the coefficient and the radical part:
- \((3\sqrt{6})^2 = 3^2 \cdot (\sqrt{6})^2 = 9 \cdot 6 = 54\).
- Multiplication: Multiplying \(3\sqrt{6}\) by another radical expression follows the product rule:
- \(3\sqrt{6} \cdot 2\sqrt{3} = 3 \cdot 2 \cdot \sqrt{6 \cdot 3} = 6\sqrt{18} = 6 \cdot 3\sqrt{2} = 18\sqrt{2}\).
Solving Equations
\(3\sqrt{6}\) can appear in algebraic equations, and solving these involves isolating the radical term.
- For example, to solve \(3x = 3\sqrt{6}\) for \(x\):
- Divide both sides by 3 to get \(x = \sqrt{6}\).
- For equations involving squares, such as \((3\sqrt{6})^2 = y\):
- We already know that \((3\sqrt{6})^2 = 54\), so \(y = 54\).
Properties and Characteristics
Understanding the properties of \(3\sqrt{6}\) helps in various algebraic manipulations:
- Irrationality: Since \(\sqrt{6}\) is irrational, \(3\sqrt{6}\) is also irrational.
- Real and Imaginary Components: In contexts involving complex numbers, \(\sqrt{-6}\) can be expressed as \(i\sqrt{6}\), where \(i\) is the imaginary unit.
3√6 in Calculus
The expression \(3\sqrt{6}\) appears in various calculus problems, particularly involving derivatives and integrals. Here are some examples of how it can be applied:
Derivatives Involving \(3\sqrt{6}\)
To find the derivative of a function involving \(3\sqrt{6}\), we can use standard differentiation rules. Consider a function \(f(x) = 3\sqrt{6x}\).
- Apply the chain rule to differentiate \(f(x)\): \[ f(x) = 3\sqrt{6x} = 3 \cdot (6x)^{1/2} \] \[ f'(x) = 3 \cdot \frac{1}{2}(6x)^{-1/2} \cdot 6 = \frac{3 \cdot 6}{2\sqrt{6x}} = \frac{18}{2\sqrt{6x}} = \frac{9}{\sqrt{6x}} \]
Integrals Involving \(3\sqrt{6}\)
When integrating expressions involving \(3\sqrt{6}\), we use standard integration techniques. Consider the integral:
\[
\int 3\sqrt{6} \, dx
\]
Since \(3\sqrt{6}\) is a constant, the integral is straightforward:
\[
\int 3\sqrt{6} \, dx = 3\sqrt{6} \cdot x + C
\]
where \(C\) is the constant of integration.
Applications in Differential Equations
Differential equations can also involve \(3\sqrt{6}\). For example, solving a separable differential equation:
\[
\frac{du}{dx} = 3\sqrt{6}
\]
To solve, integrate both sides:
\[
\int du = \int 3\sqrt{6} \, dx \implies u = 3\sqrt{6} \cdot x + C
\]
where \(C\) is the integration constant.
Fundamental Theorem of Calculus
The Fundamental Theorem of Calculus links the concept of differentiation and integration. If \(F(x)\) is the antiderivative of \(f(x)\), then:
\[
\frac{d}{dx} \left( \int_{a}^{x} 3\sqrt{6} \, dt \right) = 3\sqrt{6}
\]
This illustrates that differentiation of the integral of a constant function \(3\sqrt{6}\) yields back the original constant.
These examples show how \(3\sqrt{6}\) can be applied in different calculus contexts, demonstrating its versatility and importance in solving mathematical problems.

Practical Examples
The value \( 3\sqrt{6} \) can be found in various practical situations. Below are some detailed examples:
-
Example 1: Geometry
Consider a right triangle where one of the legs is 3 units long and the other leg is \( \sqrt{6} \) units long. The hypotenuse can be calculated using the Pythagorean theorem:
\[ c = \sqrt{(3)^2 + (\sqrt{6})^2} \]
\[ c = \sqrt{9 + 6} = \sqrt{15} \]
In this case, \( 3\sqrt{6} \) represents a transformation of one of the legs when scaling the triangle.
-
Example 2: Physics
In physics, \( 3\sqrt{6} \) could represent a quantity derived from measurements. For instance, if the acceleration due to gravity on a certain planet is \( \sqrt{6} \) m/s² and an object is dropped from a height, the velocity after 3 seconds can be found using:
\[ v = gt = \sqrt{6} \times 3 = 3\sqrt{6} \text{ m/s} \]
-
Example 3: Engineering
In an engineering problem, \( 3\sqrt{6} \) might be part of a stress-strain calculation where the square root of the stress multiplied by a factor of 3 is needed to determine the strain in a material.
Suppose the stress applied is \( \sigma = 6 \text{ Pa} \). The strain \( \epsilon \) can be determined by:
\[ \epsilon = 3\sqrt{\sigma} = 3\sqrt{6} \text{ Pa}^{-1} \]
-
Example 4: Finance
In finance, \( 3\sqrt{6} \) can appear in models involving volatility. For instance, if the volatility of an asset is \( \sqrt{6} \% \) per day, the volatility over 3 days can be approximated by:
\[ \text{Volatility} = 3 \times \sqrt{6} \% \]
These examples illustrate how \( 3\sqrt{6} \) can be used in various contexts to simplify or represent real-world quantities and relationships.
Advanced Concepts Related to 3√6
The expression \(3\sqrt{6}\) opens the door to several advanced mathematical concepts that extend beyond basic algebra and geometry. Here, we explore some of these concepts in detail.
-
Radicals and Exponents:
The expression \(3\sqrt{6}\) can be interpreted using the properties of exponents. Radicals are a special case of exponents, and the square root of 6 can be written as \(6^{1/2}\). Therefore, \(3\sqrt{6}\) can also be expressed as \(3 \times 6^{1/2}\).
-
Simplification Techniques:
Simplifying expressions involving radicals often involves recognizing patterns or using factorization. While \(3\sqrt{6}\) is already in a simplified form, understanding the principles behind simplification can be crucial when dealing with more complex radical expressions.
-
Complex Numbers:
In advanced mathematics, square roots of negative numbers are considered. While \(3\sqrt{6}\) deals with real numbers, the square root of negative numbers introduces imaginary numbers, defined as \(i = \sqrt{-1}\). Complex numbers combine real and imaginary parts, written as \(a + bi\), where \(a\) and \(b\) are real numbers.
-
Applications in Trigonometry:
Expressions like \(3\sqrt{6}\) can appear in trigonometric contexts, such as in the calculation of certain angles or lengths in geometric figures. Trigonometric identities sometimes involve radicals, requiring a solid understanding of their properties.
-
Integration and Differentiation:
In calculus, expressions with radicals are often integrated or differentiated. For example, the integral of \(3\sqrt{6}x\) with respect to \(x\) would involve applying integration techniques to a function containing a radical. Similarly, differentiating such expressions requires the application of the chain rule.
These advanced concepts showcase the depth and utility of understanding expressions like \(3\sqrt{6}\) in various branches of mathematics. Mastery of these areas enhances problem-solving skills and broadens the scope of mathematical applications.
Conclusion
The mathematical concept of \( 3\sqrt{6} \), representing the cube root of 6, elucidates its significance across various disciplines in mathematics. Initially defined as the number that, when multiplied by itself three times, results in 6, it holds fundamental properties in algebraic equations and geometric shapes. Through its precise calculation and symbolic representation, \( 3\sqrt{6} \) emerges as a pivotal value in both theoretical applications and practical scenarios.
In calculus, \( 3\sqrt{6} \) aids in the derivation of complex functions and differential equations, demonstrating its utility in higher mathematics. Practical examples showcase its relevance in real-world problem-solving, underscoring its role in engineering, physics, and economics. Advanced concepts further explore its implications in abstract algebra and computational mathematics, highlighting its interdisciplinary importance.
Overall, \( 3\sqrt{6} \) serves as a cornerstone in mathematical theory, offering profound insights into numerical relationships and structural formations. Its geometric interpretations and algebraic implications reinforce its stature as a versatile mathematical constant, fostering continual exploration and application in diverse mathematical contexts.
READ MORE:
Xem video
Video "Đơn giản hóa: 6√3+5√12" | Học về 3√6