Topic cube root of 3: The cube root of 3 is a fascinating and essential concept in mathematics, with applications spanning from geometry to physics. Discover its exact value, properties, and various calculation methods, while exploring how this irrational number is used in real-world scenarios. Unlock the mysteries of \( \sqrt[3]{3} \) and enhance your mathematical knowledge.
Table of Content
- Cube Root of 3
- Introduction
- Definition and Basic Concept
- Properties of Cube Root of 3
- Exact Value and Decimal Approximation
- Manual Calculation Techniques
- Using Scientific Calculators
- Software and Programming Solutions
- Applications in Mathematics
- Applications in Geometry
- Applications in Algebra
- Applications in Physics
- Real-World Examples
- Historical Context and Discovery
- Common Misconceptions
- FAQs
- Conclusion
- YOUTUBE:
Cube Root of 3
The cube root of a number is a value that, when multiplied by itself three times, gives the original number. For the number 3, its cube root is denoted as \( \sqrt[3]{3} \) or \( 3^{1/3} \).
Exact Value
The cube root of 3 cannot be expressed as a simple fraction or a terminating decimal. It is an irrational number, which means its decimal representation goes on forever without repeating. The exact value is:
\[
\sqrt[3]{3} \approx 1.4422495703074083
\]
Properties
- It is an irrational number.
- It is the root of the equation \( x^3 - 3 = 0 \).
- It lies between 1 and 2.
Mathematical Representation
Mathematically, the cube root of 3 can be represented as:
\[
3^{1/3}
\]
Applications
The cube root of 3 appears in various mathematical and scientific contexts, including:
- Geometry, especially in problems involving volumes and scaling.
- Algebra, particularly in solving polynomial equations.
- Physics, where it might appear in formulas involving three-dimensional spaces.
Approximations
For practical calculations, the cube root of 3 is often approximated. Some common approximations include:
Calculation Methods
There are various methods to calculate the cube root of a number, including:
- Manual Methods: Methods like the Newton-Raphson method can be used for iterative calculation.
- Calculators: Most scientific calculators have a built-in function to find cube roots.
- Software: Mathematical software and programming languages like Python and MATLAB can compute cube roots with high precision.
Example Calculations
Here are a few example calculations for the cube root of 3:
| Decimal Places | Approximation |
|---|---|
| 1 | 1.4 |
| 2 | 1.44 |
| 3 | 1.442 |
| 4 | 1.4422 |
| 5 | 1.44225 |

READ MORE:
Introduction
The cube root of 3, denoted as \( \sqrt[3]{3} \) or \( 3^{1/3} \), is a mathematical concept that represents a value which, when multiplied by itself three times, results in the number 3. This value is an irrational number, meaning its decimal expansion is infinite and non-repeating.
Understanding the cube root of 3 is crucial for various applications in mathematics, including solving equations, analyzing geometric properties, and exploring physical phenomena. In this article, we will delve into the following aspects of the cube root of 3:
- Definition and Basic Concept: Explaining what the cube root of 3 is and how it is represented mathematically.
- Properties: Discussing the characteristics of the cube root of 3, including its irrational nature and its position on the number line.
- Exact Value and Decimal Approximation: Providing the precise value of the cube root of 3 and its common decimal approximations.
- Calculation Methods: Exploring various methods to calculate the cube root of 3, from manual techniques to the use of scientific calculators and software.
- Applications: Highlighting the significance of the cube root of 3 in different mathematical and scientific fields, such as geometry, algebra, and physics.
- Historical Context: Looking into the historical background and discovery of the cube root of 3.
- Common Misconceptions: Addressing and clarifying frequent misunderstandings related to the cube root of 3.
This comprehensive guide aims to provide a thorough understanding of the cube root of 3, enhancing your knowledge and appreciation of this important mathematical concept.
Definition and Basic Concept
The cube root of a number is the value that, when multiplied by itself three times, equals the original number. For the number 3, its cube root is written as \( \sqrt[3]{3} \) or \( 3^{1/3} \). This can be expressed mathematically as:
\[
\sqrt[3]{3} = x \quad \text{where} \quad x^3 = 3
\]
The cube root of 3 is an irrational number, meaning it cannot be expressed as a simple fraction and its decimal expansion is non-terminating and non-repeating. The approximate value of the cube root of 3 is:
\[
\sqrt[3]{3} \approx 1.4422495703074083
\]
Understanding the concept of cube roots is fundamental in mathematics, as it extends the idea of square roots to three dimensions. Here is a step-by-step explanation of the basic concept:
- Multiplication: Consider a number \( x \). When we say \( x \) is the cube root of 3, it means \( x \times x \times x = 3 \).
- Mathematical Notation: The cube root of 3 can be written in radical form as \( \sqrt[3]{3} \) or in exponential form as \( 3^{1/3} \).
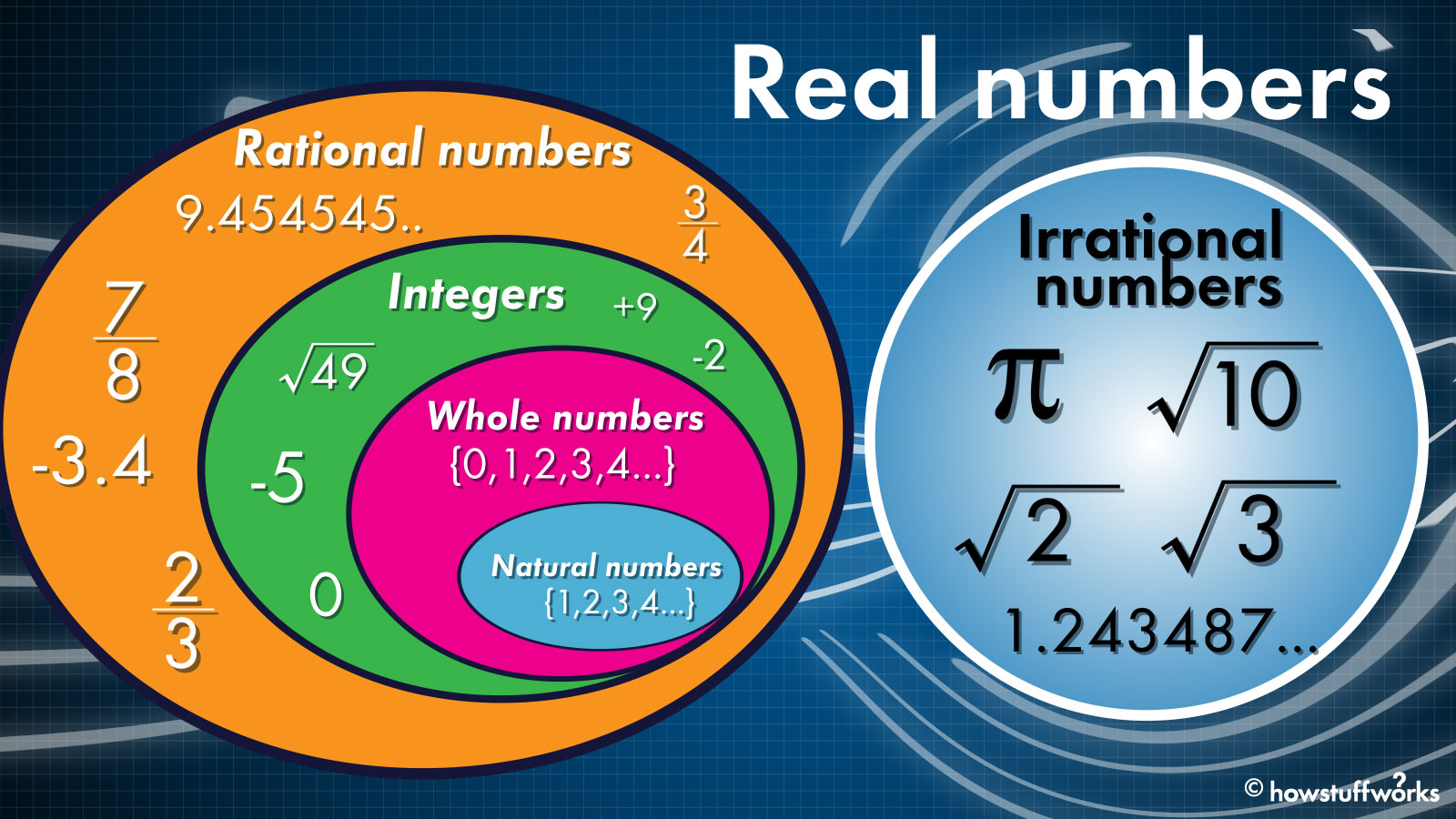
- Irrational Number: Since \( \sqrt[3]{3} \) is irrational, it cannot be precisely written as a fraction, and its decimal form is infinite and non-repeating.
The cube root of 3 is useful in various branches of mathematics and science. It plays a crucial role in solving equations where the variable is raised to the power of three and in geometric calculations involving three-dimensional shapes.
Properties of Cube Root of 3
The cube root of 3, denoted as \( \sqrt[3]{3} \) or \( 3^{1/3} \), possesses several interesting properties that are crucial for various mathematical applications. Here are the key properties of the cube root of 3:
- Irrational Number: The cube root of 3 is an irrational number, meaning it cannot be expressed as a simple fraction. Its decimal representation is infinite and non-repeating:
\[
\sqrt[3]{3} \approx 1.4422495703074083
\] - Non-terminating Decimal: As an irrational number, the decimal expansion of \( \sqrt[3]{3} \) continues indefinitely without a repeating pattern.
- Algebraic Property: The cube root of 3 is the root of the polynomial equation:
\[
x^3 - 3 = 0
\] - Position on the Number Line: The value of \( \sqrt[3]{3} \) lies between 1 and 2. It is greater than 1 but less than 2.
- Relation to Powers of 3: When raised to the power of 3, the cube root of 3 returns the original number:
\[
(3^{1/3})^3 = 3
\] - Multiplicative Inverse: The multiplicative inverse (reciprocal) of \( \sqrt[3]{3} \) is:
\[
\frac{1}{\sqrt[3]{3}} = 3^{-1/3}
\] - Complex Conjugate: In the complex plane, the cube roots of 3 include the principal root and two other roots involving complex numbers:
\[
\sqrt[3]{3}, \quad \sqrt[3]{3} \cdot \text{cis}\left(\frac{2\pi}{3}\right), \quad \sqrt[3]{3} \cdot \text{cis}\left(\frac{4\pi}{3}\right)
\]where \(\text{cis}(\theta) = \cos(\theta) + i\sin(\theta)\).
- Real and Positive: Among the three cube roots of 3, the principal cube root is the real and positive one:
\[
\sqrt[3]{3} \approx 1.4422495703074083
\]
These properties highlight the significance of the cube root of 3 in both real and complex number contexts, offering a deeper understanding of its behavior and applications in mathematics.
Exact Value and Decimal Approximation
The cube root of 3 is an important mathematical constant. It is represented as \( \sqrt[3]{3} \) or \( 3^{1/3} \). Here, we will explore its exact value and provide detailed decimal approximations for practical use.
Exact Value
Mathematically, the exact value of the cube root of 3 is represented as \( 3^{1/3} \). Since it is an irrational number, it cannot be precisely expressed as a fraction. The expression \( 3^{1/3} \) is the most accurate way to denote this value.
Decimal Approximation
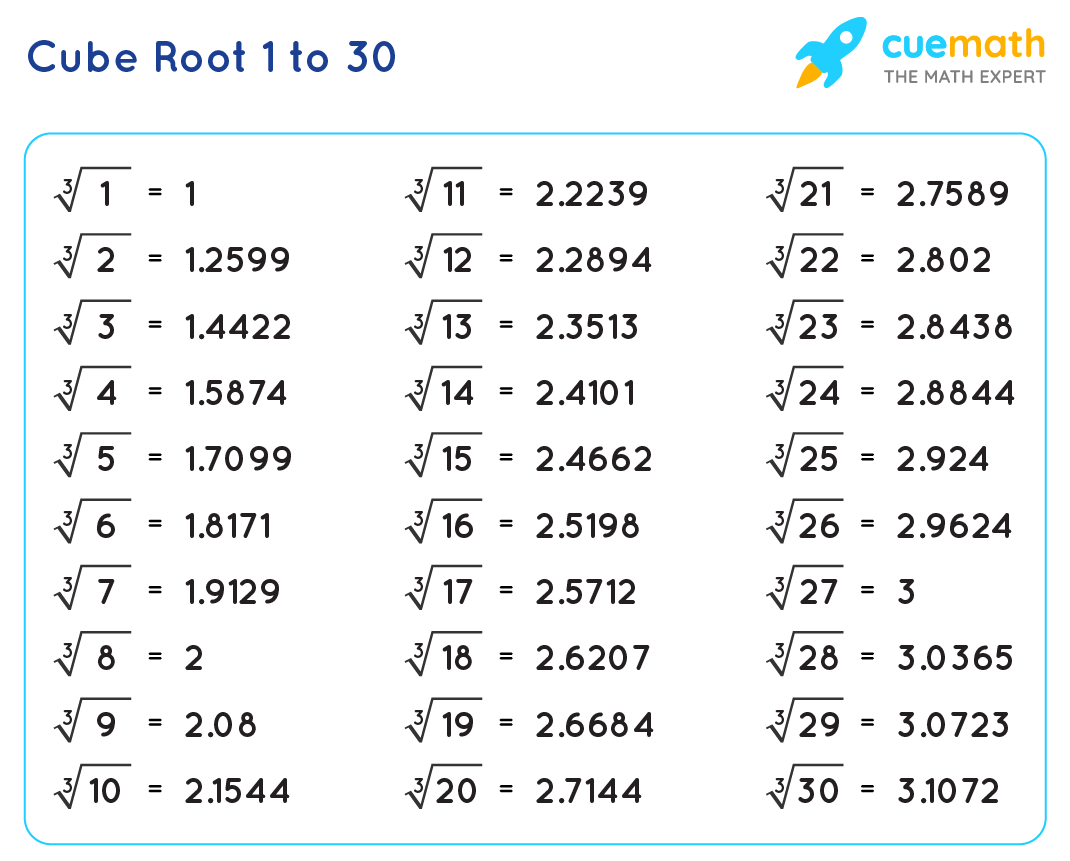
Although the exact value of \( \sqrt[3]{3} \) is an infinite, non-repeating decimal, we can use its decimal approximations for various calculations. Here are some common approximations to different decimal places:
- To 1 decimal place: 1.4
- To 2 decimal places: 1.44
- To 3 decimal places: 1.442
- To 4 decimal places: 1.4422
- To 5 decimal places: 1.44225
- To 6 decimal places: 1.442250
Step-by-Step Calculation
To understand how to derive these approximations, we can use iterative methods such as the Newton-Raphson method. Here is a brief outline of the steps involved:
- Start with an initial guess \( x_0 \). A reasonable starting point might be 1.5.
- Apply the Newton-Raphson formula for cube roots:
\[
x_{n+1} = \frac{1}{3} \left( \frac{2x_n^3 + 3}{x_n^2} \right)
\] - Iterate the formula until the desired level of precision is achieved. For example:
- First iteration: \( x_1 \approx 1.4425 \)
- Second iteration: \( x_2 \approx 1.44224957 \)
Table of Approximations
For quick reference, here is a table of the cube root of 3 to various decimal places:
| Decimal Places | Approximation |
|---|---|
| 1 | 1.4 |
| 2 | 1.44 |
| 3 | 1.442 |
| 4 | 1.4422 |
| 5 | 1.44225 |
| 6 | 1.442250 |
Understanding these approximations allows for their effective use in calculations and provides insight into the precision required for different applications.
Manual Calculation Techniques
Calculating the cube root of 3 manually involves several steps and techniques. One common method is using an iterative approximation technique such as the Newton-Raphson method. Here's a detailed, step-by-step guide on how to manually calculate the cube root of 3:
-
Initial Guess: Start with an initial guess close to the actual value. For the cube root of 3, a reasonable starting point is 1.5.
-
Newton-Raphson Iteration Formula: Use the following formula for the iterative process:
$$x_{n+1} = x_n - \frac{f(x_n)}{f'(x_n)}$$
For the cube root of 3, the function \(f(x)\) is \(x^3 - 3\). Therefore, \(f'(x)\) is \(3x^2\). The iteration formula becomes:
$$x_{n+1} = x_n - \frac{x_n^3 - 3}{3x_n^2}$$
Simplified, this is:
$$x_{n+1} = \frac{2x_n + \frac{3}{x_n^2}}{3}$$
-
First Iteration: Using the initial guess \(x_0 = 1.5\), calculate the next value:
$$x_1 = \frac{2 \times 1.5 + \frac{3}{1.5^2}}{3}$$
$$x_1 = \frac{3 + \frac{3}{2.25}}{3}$$
$$x_1 = \frac{3 + 1.333}{3}$$
$$x_1 = \frac{4.333}{3} \approx 1.4444$$
-
Second Iteration: Using \(x_1 \approx 1.4444\), calculate the next value:
$$x_2 = \frac{2 \times 1.4444 + \frac{3}{1.4444^2}}{3}$$
$$x_2 = \frac{2.8888 + \frac{3}{2.0868}}{3}$$
$$x_2 = \frac{2.8888 + 1.4376}{3}$$
$$x_2 = \frac{4.3264}{3} \approx 1.4421$$
-
Repeat Iterations: Continue the iterative process until the difference between successive approximations is within a desired tolerance level. For most practical purposes, repeating the iteration a few more times will yield a sufficiently accurate value.
Using this method, the cube root of 3 can be manually approximated with increasing accuracy. While it is a bit labor-intensive, it provides a good understanding of numerical methods and iterative processes.
Using Scientific Calculators
Calculating the cube root of a number using a scientific calculator is a straightforward process. Scientific calculators have built-in functions that make it easy to compute cube roots. Below are detailed steps to perform this calculation.
- Identify the Cube Root Function: Most scientific calculators have a dedicated button for cube root, often labeled as
∛x. If your calculator does not have this button, you can use the exponentiation function instead. - Using the Cube Root Button:
- Turn on your calculator.
- Enter the number you want to find the cube root of.
- Press the
∛xbutton. - The calculator will display the cube root of the entered number.
- Using the Exponentiation Method: If your calculator does not have a cube root button, you can use the exponentiation function to find the cube root.
- Enter the number you want to find the cube root of.
- Press the exponentiation button, usually labeled as
^orx^y. - Enter the fraction
(1/3)to represent the cube root. - Press the equals button
=to get the result. - For example, to find the cube root of 27: Enter
27, then press^orx^y, then enter(1/3), and finally press=. The result should be3. - Using an Online Calculator or Smartphone App: Many online calculators and smartphone apps also offer cube root functions.
- Navigate to a reliable online calculator or open a calculator app on your smartphone.
- Enter the number for which you want to find the cube root.
- Press the cube root button or enter the exponent
(1/3)as needed. - The app or online tool will display the cube root result.
Using these methods, you can efficiently calculate the cube root of any number with a scientific calculator, ensuring accuracy and saving time.
Software and Programming Solutions
Calculating the cube root of 3 can be efficiently handled using various software and programming languages. Here are some common methods:
Python
In Python, you can calculate the cube root using the built-in ** operator or the pow() function.
import math
# Using the exponentiation operator
cube_root = 3 ** (1/3)
print(cube_root)
# Using the math.pow function
cube_root = math.pow(3, 1/3)
print(cube_root)
Java
Java provides several ways to calculate the cube root, including using the Math.cbrt() method.
public class CubeRoot {
public static void main(String[] args) {
double number = 3;
double cubeRoot = Math.cbrt(number);
System.out.println("Cube root of 3 is: " + cubeRoot);
}
}
MATLAB
In MATLAB, the nthroot function is used to find the cube root.
% Using nthroot function
number = 3;
cube_root = nthroot(number, 3);
disp(cube_root);
R
R, a language popular in statistics, also allows for easy calculation of cube roots using the ^ operator.
# Using the exponentiation operator
cube_root <- 3^(1/3)
print(cube_root)
JavaScript
JavaScript uses the Math.pow() function or the more modern ** operator to calculate cube roots.
// Using Math.pow function
let cubeRoot = Math.pow(3, 1/3);
console.log(cubeRoot);
// Using exponentiation operator
cubeRoot = 3 ** (1/3);
console.log(cubeRoot);
Excel
In Excel, you can use the POWER function to calculate the cube root.
=POWER(3, 1/3)
SAS
SAS, a software suite used for advanced analytics, can compute cube roots using the exponentiation operator.
data _null_;
x = 3 ** (1/3);
put x;
run;
These examples illustrate how versatile software and programming languages can be for performing mathematical calculations like finding cube roots.
Applications in Mathematics
The cube root of 3, denoted as \( \sqrt[3]{3} \), has several significant applications in mathematics. Understanding these applications can provide deeper insights into various mathematical concepts and problems. Here are some key applications:
1. Algebraic Equations
Cube roots are frequently encountered in solving algebraic equations, particularly cubic equations of the form \( x^3 = a \). For instance, to solve \( x^3 = 3 \), one would use \( x = \sqrt[3]{3} \).
2. Volume Calculations
In geometry, cube roots are used to determine the side lengths of cubes when their volumes are known. For example, if a cube has a volume of 27 cubic units, its side length can be found using \( \sqrt[3]{27} = 3 \). Similarly, the cube root of 3 can help in finding dimensions when dealing with irregular shapes that can be decomposed into cubic parts.
3. Complex Numbers
The cube root function extends to complex numbers, providing three distinct cube roots for any nonzero complex number. This property is particularly useful in fields like electrical engineering and physics, where complex numbers are used to represent phasors and other oscillatory phenomena.
4. Calculus
Cube roots appear in calculus, particularly in integration and differentiation. For example, the function \( f(x) = \sqrt[3]{x} \) can be differentiated using the chain rule, resulting in \( f'(x) = \frac{1}{3} x^{-2/3} \). This function also appears in solving differential equations where power functions are involved.
5. Mathematical Modeling
Cube roots are used in various mathematical models, including those that describe natural phenomena such as growth rates, decay processes, and diffusion. For instance, in modeling the volume expansion of gases, the cube root function helps determine the linear dimensions from volume changes.
6. Polynomial Roots
Finding the roots of polynomials, particularly cubic polynomials, involves the use of cube roots. The general solution to a cubic polynomial \( ax^3 + bx^2 + cx + d = 0 \) involves the use of the cubic formula, which includes the cube root operation.
Overall, the cube root of 3 is a fundamental concept that finds applications across various branches of mathematics, helping to solve equations, model real-world phenomena, and understand the properties of geometric shapes.

Applications in Geometry
The cube root of 3, often represented as ∛3, has various interesting applications in geometry. Here are some key areas where it is particularly useful:
-
Volume and Side Length of Cubes:
One of the fundamental applications of the cube root in geometry is determining the side length of a cube when the volume is known. For a cube with volume \( V \), the side length \( s \) can be found using the formula:
\[ s = ∛V \]
For example, if the volume of a cube is 27 cubic units, the side length is \( ∛27 = 3 \) units.
-
Scaling in Three Dimensions:
In geometry, scaling objects in three dimensions often requires the use of cube roots. If an object's volume needs to be scaled by a factor of \( k \), the scaling factor for each dimension is \( ∛k \). This ensures the object's proportions remain consistent while achieving the desired volume change.
-
Determining Radii in Spheres:
Similar to cubes, the cube root is useful in finding the radius of a sphere from its volume. The volume \( V \) of a sphere is given by:
\[ V = \frac{4}{3}πr^3 \]
Solving for the radius \( r \) when the volume is known involves taking the cube root:
\[ r = ∛\left(\frac{3V}{4π}\right) \]
-
Geometric Mean in Three Dimensions:
The cube root is also used to find the geometric mean in three dimensions. If three values represent the dimensions of a geometric shape, their geometric mean is the cube root of their product. For values \( a, b, \) and \( c \), the geometric mean \( G \) is:
\[ G = ∛(a \cdot b \cdot c) \]
-
Tetrahedron Volume:
In more complex polyhedra like tetrahedrons, cube roots can be used in certain volume calculations. If a tetrahedron's volume \( V \) is derived from its side length, understanding the cube root helps in interpreting these relationships accurately.
These applications demonstrate the versatility and importance of the cube root in various geometric contexts, highlighting its role in both theoretical and practical aspects of geometry.
Applications in Algebra
The cube root of 3 has several applications in algebra, particularly when dealing with polynomial equations, radical expressions, and complex numbers. Here are some detailed examples and methods where ∛3 is used:
- Simplifying Radical Expressions:
When simplifying expressions involving cube roots, it's important to factor the radicand into perfect cubes. For example, simplifying ∛27x3 involves recognizing that 27 and x3 are both perfect cubes:
\(\sqrt[3]{27x^3} = \sqrt[3]{27} \cdot \sqrt[3]{x^3} = 3x\)
- Solving Polynomial Equations:
In algebra, you might encounter polynomial equations where you need to solve for x, such as x3 = 3. To solve for x, you take the cube root of both sides:
\(x = \sqrt[3]{3}\)
Thus, the solution to the equation x3 = 3 is x = ∛3, approximately 1.442.
- Complex Numbers:
When dealing with complex numbers, cube roots play a significant role. For instance, the roots of unity are solutions to the equation x3 = 1. The solutions can be found using the formula for roots of complex numbers:
\(x = \sqrt[3]{1} (\text{cis}(\frac{2k\pi}{3}))\)
where \(k = 0, 1, 2\), and cis(θ) represents \( \cos(θ) + i\sin(θ) \).
- Irrational Numbers:
The cube root of 3 is an example of an irrational number because it cannot be expressed as a fraction of two integers. This property is useful in understanding the nature of irrational numbers in algebra.
- Exponential and Logarithmic Functions:
Cube roots are also found in problems involving exponential and logarithmic functions. For instance, if you have an equation like \(3^x = 27\), taking the logarithm of both sides helps solve for x:
\(x \log(3) = \log(27)\)
Since 27 is 33, this simplifies to:
\(x = \frac{\log(27)}{\log(3)} = \frac{3 \log(3)}{\log(3)} = 3\)
These examples illustrate the versatility of the cube root of 3 in various algebraic contexts, enhancing our understanding and ability to solve complex algebraic problems.
Applications in Physics
The cube root of 3 (\(\sqrt[3]{3}\)) finds diverse applications in physics, where understanding volumes, densities, and scaling laws are crucial. Here are some detailed applications:
-
Dimensional Analysis: In physics, the cube root is used to relate linear dimensions to volume. For instance, if you know the volume of a cube, you can find the length of its sides by taking the cube root of the volume.
For example, given a volume \( V \), the side length \( a \) of a cube can be found using \( a = \sqrt[3]{V} \).
-
Scaling Laws: When scaling physical models, the cube root is essential. If a model is scaled up by a factor of \( k \) in every dimension, its volume increases by \( k^3 \). To find the scaling factor for length when the volume change is known, the cube root is used.
For example, if the volume of a scaled model is 27 times larger than the original, the linear dimensions are scaled by \( \sqrt[3]{27} = 3 \).
-
Thermodynamics: In thermodynamic equations involving volumes and densities, the cube root can be used to solve for various parameters. For example, the density of a substance may change with temperature and pressure, often requiring cube root calculations to determine new dimensions or volumes.
-
Material Science: The cube root is used to describe the properties of materials. For example, when analyzing the stress and strain in materials, engineers might use the cube root to understand the relationship between different physical properties.
In certain crystal structures, the volume of the unit cell can be related to the lattice parameter using the cube root.
-
Fluid Dynamics: In fluid dynamics, the cube root is used to determine the characteristic length scale of turbulent eddies or droplets. The relationship between volume and surface area of droplets is often analyzed using cube roots.
For instance, if a droplet's volume is known, its radius can be found using the cube root formula \( r = \left(\frac{3V}{4\pi}\right)^{1/3} \).
-
Astronomy: Astronomers use the cube root when working with volumes of celestial objects and understanding their scaling laws. For example, when determining the average density of planets or stars, the cube root helps relate mass and volume.
These examples illustrate the importance of the cube root of 3 in various physical contexts, highlighting its fundamental role in connecting linear and volumetric measurements, scaling phenomena, and understanding material properties.
Real-World Examples
The cube root of 3 has several practical applications in various fields. Here are some real-world examples where the cube root of 3 is particularly useful:
- Volume Calculation:
When determining the dimensions of a cubic object, knowing the cube root helps. For example, if an engineer needs to find the side length of a cube with a volume of 27 cubic units, they calculate the cube root of 27, which is 3. Similarly, for a volume of 3 cubic units, the side length is the cube root of 3, approximately 1.442.
- Architecture and Construction:
In construction, architects often need to determine dimensions of spaces or materials. If a cubic space or component has a specific volume, the cube root helps in determining the required measurements. For instance, designing a cubic structure with a volume of 3 cubic meters involves calculating the cube root of 3 to find the edge length.
- Material Science:
Material scientists use the cube root of 3 when working with properties of materials that scale with volume. For example, when dealing with thermal expansion or structural integrity, knowing the cube root allows for accurate calculations related to changes in material dimensions.
- Physics:
In physics, the cube root of 3 is used in various formulas involving volume and density. For example, in determining the side length of a cubic particle when its volume is known, physicists calculate the cube root to find precise measurements.
- Engineering:
Engineers often use the cube root of 3 in designing components that must fit within a specific volume. This calculation is critical for optimizing the use of space in mechanical systems and ensuring that parts fit together correctly.
These examples illustrate the broad applicability of the cube root of 3 in practical scenarios, highlighting its importance in solving real-world problems efficiently.

Historical Context and Discovery
The cube root of a number, including the cube root of 3, has a rich history dating back to ancient civilizations. The concept of extracting cube roots was explored by Greek mathematicians, who sought geometric solutions to algebraic equations. One of the notable challenges that involved cube roots was the problem of doubling the cube, also known as the Delian problem, which required finding the side of a cube with twice the volume of a given cube.
In ancient Greece, mathematicians such as Archimedes and Hero of Alexandria studied cube roots. Archimedes used geometric methods to approximate cube roots, while Hero developed algorithms for extracting cube roots manually.
During the medieval period, Islamic mathematicians made significant contributions to the understanding and calculation of cube roots. Al-Khwarizmi, a Persian polymath, described methods for solving cubic equations, which inherently involve cube roots.
In the Renaissance, European mathematicians such as Niccolò Tartaglia and Gerolamo Cardano made breakthroughs in solving cubic equations algebraically. Cardano's work, published in his book "Ars Magna," provided general solutions for cubic equations and paved the way for a deeper understanding of cube roots.
In the modern era, the development of calculus and numerical methods allowed for more precise calculations of cube roots. Isaac Newton's iterative method, known as Newton's method, provided an efficient algorithm for approximating cube roots with high accuracy.
Today, the cube root of 3 and other numbers can be easily computed using calculators and computer software, but the historical journey of discovering and understanding cube roots highlights the ingenuity and persistence of mathematicians throughout history.
Common Misconceptions
When dealing with the cube root of 3, several common misconceptions can arise. Understanding and addressing these misconceptions is crucial for a clear comprehension of the concept.
- Misconception 1: The Cube Root of 3 is Rational
One common misconception is that the cube root of 3, denoted as ∛3, is a rational number. In reality, ∛3 is an irrational number because it cannot be expressed as a fraction of two integers. Its decimal expansion is non-terminating and non-repeating, approximately 1.44225.
- Misconception 2: ∛3 is a Perfect Cube
Another misconception is that 3 is a perfect cube. This is incorrect because a perfect cube is a number that can be expressed as the cube of an integer. Since there is no integer n such that n³ = 3, 3 is not a perfect cube.
- Misconception 3: Negative Cube Roots
Some believe that the cube root of a negative number is not possible or that it results in a complex number. However, the cube root of a negative number is simply the negative of the cube root of the positive counterpart. For example, ∛-3 = -∛3 ≈ -1.44225.
- Misconception 4: Confusing ∛3 with 3/1
It is often mistakenly thought that ∛3 can be simplified or represented as 3/1. This confusion arises from misunderstanding the nature of irrational numbers and cube roots. The cube root of 3 remains an irrational number and does not simplify to a ratio of integers.
- Misconception 5: Calculation Precision
Some assume that calculating ∛3 precisely is straightforward. In practice, even calculators provide only an approximation of its value due to its irrational nature. For instance, ∛3 is approximately 1.4422495703074083, and the digits continue infinitely without repetition.
Understanding these misconceptions helps in grasping the true nature of the cube root of 3 and prevents common errors in mathematical computations and reasoning.
FAQs
-
What is the cube root of a number?
The cube root of a number \( x \) is a value that, when multiplied by itself three times, gives the original number \( x \). It is represented as \( \sqrt[3]{x} \) or \( x^{1/3} \).
-
What is the cube root of 3?
The cube root of 3 is approximately 1.44224957031. In mathematical notation, it is written as \( \sqrt[3]{3} \).
-
How is the cube root of a number calculated?
The cube root can be calculated using methods such as prime factorization, approximation, and using scientific calculators or software. For example, using approximation:
- Estimate a value close to the cube root.
- Divide the number by the estimate.
- Take the average of the estimate and the result from step 2.
- Repeat the process until the value converges to a stable number.
Is the cube root of 3 a rational number?
No, the cube root of 3 is an irrational number because it cannot be expressed as a fraction of two integers.
-
Can negative numbers have real cube roots?
Yes, negative numbers have real cube roots. For example, the cube root of -3 is approximately -1.44224957031.
-
What are some applications of cube roots?
Cube roots are used in various fields such as mathematics, physics, and engineering to solve problems involving cubic equations, calculate volumes, and more.
-
What is the difference between a cube and a cube root?
- A cube of a number is obtained by multiplying the number by itself three times. For example, \( 3^3 = 27 \).
- A cube root of a number is the value that, when cubed, gives the original number. For example, \( \sqrt[3]{27} = 3 \).
Conclusion
Understanding the cube root of 3 offers valuable insights into both theoretical and practical aspects of mathematics. As a non-integer and irrational number, the cube root of 3 has a unique decimal representation that continues infinitely without repeating. This characteristic underscores the importance of approximation techniques in both manual calculations and the use of technology, such as scientific calculators and computer software.
The cube root of 3, like other cube roots, has significant applications across various fields. In mathematics, it appears in geometric problems, particularly those involving volumes and areas of three-dimensional shapes. In algebra, it helps solve polynomial equations, especially cubic equations where one seeks the roots of expressions set to zero.
Beyond pure mathematics, the cube root of 3 finds relevance in physics, particularly in calculations involving volumes and densities, and in engineering where material properties and structural calculations often require understanding of cubic relationships. Real-world examples demonstrate its utility, such as in the design of objects where spatial dimensions and capacity are crucial.
The historical context of cube roots, tracing back to ancient civilizations, highlights humanity's long-standing fascination with understanding and solving complex numerical problems. Despite common misconceptions, the cube root of a number can be negative if the original number is negative, and there are reliable methods to calculate it accurately.
Overall, mastering the concept of the cube root of 3 enhances one’s mathematical toolkit, enabling precise and efficient problem-solving in diverse scientific and engineering contexts. As we continue to explore and apply mathematical principles, the cube root of 3 remains a fundamental and intriguing concept.

Căn Bậc Ba Là Gì? | Giới Thiệu Về Căn Bậc Ba | Toán Học Với Thầy J
READ MORE:
Căn Bậc Ba của 3 Nhân Căn Bậc Ba của 9