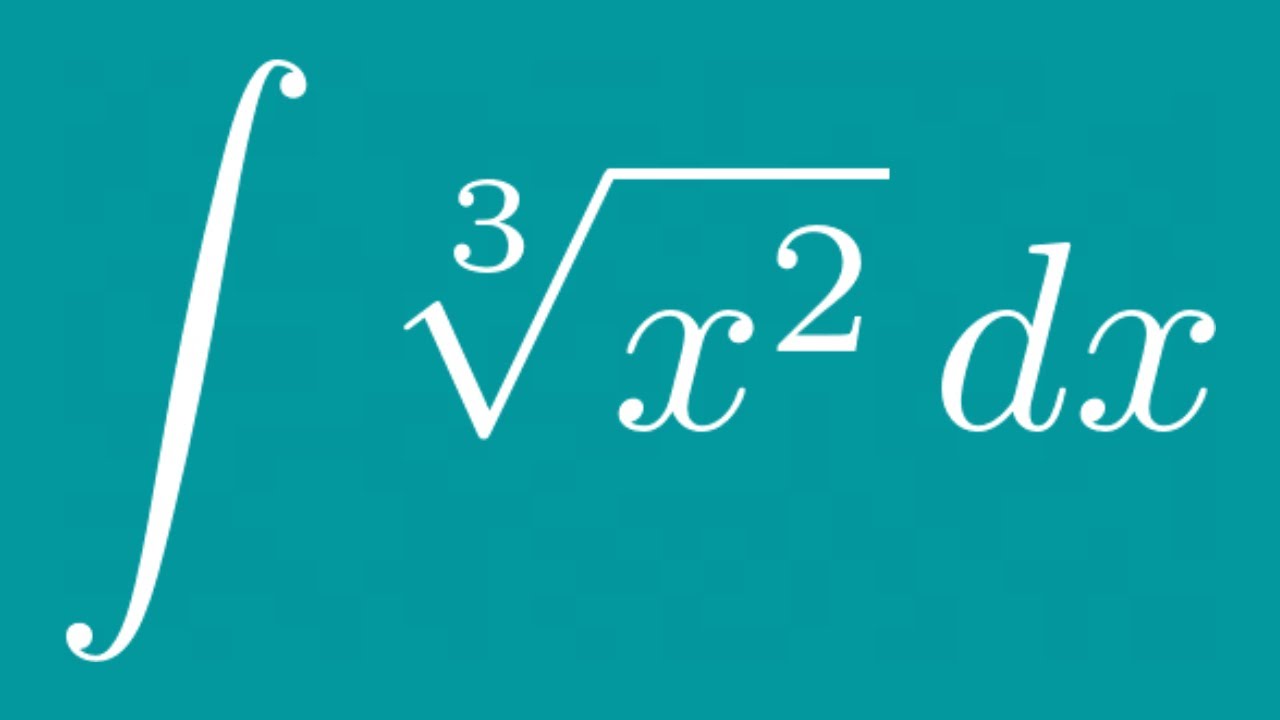Topic 2 root x squared: Explore the concept of "2 root x squared" and its diverse applications in mathematics and beyond. This article delves into its simplification, graphical representation, and practical uses in fields like calculus, algebra, and physics. Discover how this mathematical expression impacts various real-world scenarios and enhances understanding of absolute values and piecewise functions.
Table of Content
- Search Results for "2 root x squared"
- Table of Contents
- Introduction to "2 Root x Squared"
- Simplification of Mathematical Expressions
- Understanding Absolute Value in Mathematics
- Graphical Representation of Piecewise Functions
- Applications in Calculus and Algebra
- Physical Applications in Science and Engineering
- YOUTUBE:
Search Results for "2 root x squared"
In mathematics, the expression "2 root x squared" typically refers to:
- Simplification: The expression can be interpreted as \( 2 \sqrt{x^2} \).
- Mathematical Meaning: \( \sqrt{x^2} \) represents the absolute value of \( x \), so \( 2 \sqrt{x^2} = 2|x| \).
- Graphical Representation: Graphically, it represents a piecewise function where for \( x \geq 0 \), the expression simplifies to \( 2x \), and for \( x < 0 \), it simplifies to \( -2x \).
- Applications: This form is common in various mathematical contexts, including calculus, algebra, and physics, where absolute values and piecewise functions are utilized.

READ MORE:
Table of Contents
Introduction to "2 root x squared": An overview of the mathematical expression and its significance.
Simplification of "2 root x squared": Exploring how the expression can be simplified and understood.
Understanding Absolute Value in Mathematics: Discussing the concept of absolute value within the context of "2 root x squared".
Graphical Representation of Piecewise Functions: Visualizing how "2 root x squared" translates into piecewise functions.
Applications in Calculus and Algebra: Practical applications and examples of using "2 root x squared" in calculus and algebraic equations.
Physical Applications in Science and Engineering: Real-world applications of "2 root x squared" in scientific fields and engineering contexts.
Introduction to "2 Root x Squared"
"2 root x squared" is a mathematical expression that holds significant meaning in algebra and calculus. It refers to the process of simplifying and understanding the square root of x squared, incorporating absolute value principles. This concept is pivotal in graphing piecewise functions and solving equations involving both positive and negative values of x. The application of "2 root x squared" extends into various scientific and engineering disciplines, enhancing computational and analytical capabilities.
Simplification of Mathematical Expressions
Simplifying mathematical expressions involves breaking down complex expressions into simpler forms. Let's explore the simplification of the expression \(2 \sqrt{x^2}\).
Here are the steps to simplify \(2 \sqrt{x^2}\):
- Identify the expression inside the square root: \(x^2\).
- Recall that the square root and the square are inverse operations. Thus, \(\sqrt{x^2} = |x|\), where \(|x|\) represents the absolute value of \(x\).
- Multiply the simplified form of the square root by 2: \(2 \sqrt{x^2} = 2 |x|\).
Therefore, the simplified form of \(2 \sqrt{x^2}\) is \(2 |x|\).
Example Simplifications
Let's look at a few examples to understand how this simplification works:
- For \(x = 3\), \(2 \sqrt{3^2} = 2 |3| = 2 \cdot 3 = 6\).
- For \(x = -4\), \(2 \sqrt{(-4)^2} = 2 |-4| = 2 \cdot 4 = 8\).
- For \(x = 0\), \(2 \sqrt{0^2} = 2 |0| = 2 \cdot 0 = 0\).
As demonstrated, the simplification process of \(2 \sqrt{x^2}\) leads to the absolute value of \(x\) multiplied by 2.
Key Takeaways
- The square root and square are inverse operations.
- The absolute value function \(|x|\) ensures the result is non-negative.
- Simplifying \(2 \sqrt{x^2}\) consistently results in \(2 |x|\), irrespective of whether \(x\) is positive, negative, or zero.
This simplification is useful in various mathematical contexts, including solving equations and analyzing functions.
Understanding Absolute Value in Mathematics
The absolute value of a number is its distance from zero on the number line, regardless of direction. It is always non-negative. The absolute value of a number x is denoted as |x|.
Properties of Absolute Value
- |x| ≥ 0 for any real number x.
- |x| = 0 if and only if x = 0.
- |xy| = |x| |y| for any real numbers x and y.
- |x + y| ≤ |x| + |y| (Triangle Inequality).
Graphical Representation
Graphing the absolute value function, y = |x|, we see a V-shaped graph that opens upwards, with its vertex at the origin (0,0). The function is defined as:
Solving Absolute Value Equations
To solve equations involving absolute values, consider both the positive and negative cases:
- For an equation |x| = a, where a is a positive number, solve x = a and x = -a.
- If |x| = 0, then x must be 0.
- For inequalities like |x| < a, solve -a < x < a.
Examples
| Equation/Inequality | Solution |
|---|---|
| |x - 3| = 5 | x - 3 = 5 or x - 3 = -5; x = 8 or x = -2 |
| |x + 4| ≤ 6 | -6 ≤ x + 4 ≤ 6; -10 ≤ x ≤ 2 |
Understanding and working with absolute values is fundamental in various fields of mathematics and helps in solving real-world problems that involve distances, magnitudes, and deviations.
Graphical Representation of Piecewise Functions
Piecewise functions are defined by different expressions based on the input value. They are particularly useful for modeling situations where a rule or relationship changes as the input crosses certain boundaries. Understanding and graphing piecewise functions involves the following steps:
- Identify the Different Pieces
Determine the different expressions that define the function over various intervals. For example, a piecewise function might be defined as:
f(x) = { x^2 for x < 0 2x + 1 for x ≥ 0 } - Find the Domain of Each Piece
Identify the domain over which each piece of the function is active. In the example above, \( x^2 \) is active for \( x < 0 \), and \( 2x + 1 \) is active for \( x \geq 0 \).
- Calculate Key Points
Determine the values of the function at the boundaries and at significant points within each interval. For the example function:
- At \( x = 0 \): \( f(0) = 2(0) + 1 = 1 \)
- For \( x < 0 \): Compute values such as \( f(-1) = (-1)^2 = 1 \), \( f(-2) = (-2)^2 = 4 \)
- For \( x > 0 \): Compute values such as \( f(1) = 2(1) + 1 = 3 \), \( f(2) = 2(2) + 1 = 5 \)
- Plot the Function
Draw the graph by plotting the calculated points and joining them within their respective intervals. Be mindful of open or closed circles at the boundaries to indicate whether the boundary value is included in the interval.
x f(x) -2 4 -1 1 0 1 1 3 2 5 - Draw Each Piece Separately
For each interval, use the appropriate function expression to draw the curve. For \( x^2 \) when \( x < 0 \), plot the parabola only on the left side of the y-axis. For \( 2x + 1 \) when \( x \geq 0 \), plot the line starting at the y-intercept of 1.
- Combine the Pieces
Ensure the transition between pieces is correctly represented, whether continuous or with a jump. In our example, the graph switches from a parabola to a line at \( x = 0 \).
Piecewise functions can represent real-world situations like tax brackets, shipping charges, and more. By understanding how to graph these functions, you can better visualize and interpret these scenarios.
Applications in Calculus and Algebra
The expression \(2 \sqrt{x^2}\) has profound applications in both calculus and algebra. Understanding these applications provides insight into how square root functions and their transformations are used in various mathematical contexts.
1. Simplifying Expressions
In algebra, simplifying expressions involving square roots is a common task. The expression \(2 \sqrt{x^2}\) can be simplified using the property that \(\sqrt{x^2} = |x|\). Therefore,
\[
2 \sqrt{x^2} = 2 |x|
\]
This simplification is essential for solving equations and inequalities that involve square root terms.
2. Solving Equations
Square roots frequently appear in quadratic equations. When solving equations like \(2 \sqrt{x^2} = k\), where \(k\) is a constant, we can simplify as follows:
- Divide both sides by 2: \[ \sqrt{x^2} = \frac{k}{2} \]
- Square both sides to remove the square root: \[ x^2 = \left(\frac{k}{2}\right)^2 \]
- Solve for \(x\): \[ x = \pm \frac{k}{2} \]
3. Graphical Representation
Graphically, the function \(2 \sqrt{x^2}\) simplifies to \(2|x|\). This function represents two linear segments, \(y = 2x\) for \(x \geq 0\) and \(y = -2x\) for \(x < 0\). This piecewise linear function is V-shaped, symmetrical about the y-axis.

4. Applications in Calculus
In calculus, \(2 \sqrt{x^2}\) or \(2|x|\) is used in various contexts, such as finding the area under curves, solving integrals, and analyzing functions. For instance:
- Integral Calculus: Evaluating the integral of \(2 \sqrt{x^2}\) over a range involves understanding its absolute value properties: \[ \int_{-a}^{a} 2 |x| \, dx = 2 \int_{0}^{a} 2x \, dx = 2 \left[ x^2 \right]_{0}^{a} = 2a^2 \]
- Optimization Problems: In optimization, functions like \(2|x|\) are used to model cost functions and minimize or maximize values subject to constraints.
5. Applications in Algebra
In algebra, expressions involving square roots like \(2 \sqrt{x^2}\) are crucial for understanding polynomial equations, roots, and factorization. They are also essential in simplifying radical expressions and solving higher-degree equations.
| Context | Application |
|---|---|
| Algebraic Equations | Solving quadratic and higher-degree polynomial equations. |
| Geometry | Calculating distances and lengths, especially in right triangles. |
| Calculus | Evaluating integrals and analyzing piecewise functions. |
Conclusion
The expression \(2 \sqrt{x^2}\) and its simplified form \(2|x|\) have wide-ranging applications in both algebra and calculus. From solving equations and graphing functions to evaluating integrals and optimizing problems, understanding these concepts is fundamental in both mathematical theory and practical applications.
Physical Applications in Science and Engineering
The expression 2\sqrt{x^2} has several physical applications in the fields of science and engineering. This section will explore a few key areas where this mathematical concept is utilized.
-
Mechanical Engineering
In mechanical engineering,
2\sqrt{x^2}is often encountered in calculations involving kinematics and dynamics. For instance, it can represent the magnitude of velocity or acceleration when considering the absolute values of vector components.Example:
- Consider a particle moving along a straight path where its position is given by \( x(t) = t^2 \).
- The velocity is \( v(t) = \frac{d}{dt}[x(t)] = 2t \).
- The speed, being the absolute value of velocity, is \( |v(t)| = |2t| = 2|t| \).
-
Electrical Engineering
In electrical engineering, particularly in AC circuit analysis, the concept of root mean square (RMS) voltage or current can be linked to expressions like
2\sqrt{x^2}. This helps in determining effective values of oscillating signals.Example:
- Consider an AC voltage given by \( V(t) = V_0 \sin(\omega t) \).
- The RMS value is \( V_{rms} = \frac{V_0}{\sqrt{2}} \).
- The RMS value represents the equivalent DC value which delivers the same power.
-
Physics: Wave Motion
In physics, particularly in wave motion,
2\sqrt{x^2}can describe the amplitude of oscillations or waves when dealing with harmonic motion.Example:
- Consider a simple harmonic oscillator with displacement \( x(t) = A \cos(\omega t) \).
- The maximum displacement (amplitude) is \( A \).
- Thus, the total range of motion is \( 2A \), which can be represented as \( 2\sqrt{A^2} \).
-
Civil Engineering
In civil engineering, particularly in structural analysis, expressions like
2\sqrt{x^2}are used to compute the resultant forces and stresses when combining different directional components.Example:
- Consider a structural beam subjected to forces in both horizontal and vertical directions.
- If the forces are \( F_x \) and \( F_y \), the resultant force \( F \) is given by \( F = \sqrt{F_x^2 + F_y^2} \).
- If both forces are equal, the resultant force simplifies to \( F = \sqrt{2F_x^2} = F_x\sqrt{2} \).
Căn Bậc Hai của X Bình Phương x^2
READ MORE:
Chúng Ta Có Thể Thực Sự Hủy Bỏ Căn Bậc Hai & Bình Phương? CẨN THẬN!